Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-28T11:08:51.711Z Has data issue: false hasContentIssue false
  • English
  • Français

Intermittency and inertial particle entrainment at a turbulent interface: the effect of the large-scale eddies

Published online by Cambridge University Press:  03 February 2012

G. H. Good ,
S. Gerashchenko  and
Z. Warhaft
G. H. Good
Affiliation:
Sibley School of Mechanical and Aerospace Engineering, Cornell University, Ithaca, NY 14853, USA and International Collaboration for Turbulence Research
S. Gerashchenko
Affiliation:
Sibley School of Mechanical and Aerospace Engineering, Cornell University, Ithaca, NY 14853, USA and International Collaboration for Turbulence Research
Z. Warhaft*
Affiliation:
Sibley School of Mechanical and Aerospace Engineering, Cornell University, Ithaca, NY 14853, USA and International Collaboration for Turbulence Research Atkinson Center for a Sustainable Future, Cornell University, Ithaca, NY 14853, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We present measurements of mean and conditional number densities, radial distribution functions (r.d.f.s), velocities and accelerations of sub-Kolmogorov-scale water droplets entraining at a shearless turbulence–turbulence interface (TTI) and a turbulence–non-turbulence interface (TNI). We thus look at statistics of an inhomogeneous inertial particle field in both homogeneous and inhomogeneous turbulence. As in a previous communication (Gerashchenko, Good & Warhaft J. Fluid Mech., vol. 818, 2011, pp. 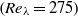293–303), an active grid produces high-Reynolds number turbulence on either one or both sides of a splitter plate in a wind tunnel. Sprays seed droplets on one side of the splitter plate, while screens dampen turbulence in the adjacent flow for the TNI. Gravitational and inertial effects are isolated by turning of the apparatus with respect to gravity. We parameterize the droplets under homogeneous conditions, where it is demonstrated that both the sweeping and loitering effects on the droplet settling velocities are present. In the inhomogeneous conditions, we show that the droplets are entrained in bulk, resulting in large-scale clusters and preserving the droplet-ambient conditions of the seeded side of the flows.

JFM classification

Turbulent Flows: Intermittency Mixing: Turbulent mixing
Type
Papers
Information
Journal of Fluid Mechanics , Volume 694 , 10 March 2012 , pp. 371 - 398
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

‡

Present address: Now with the P-23 Extreme Fluids Team, Los Alamos National Laboratory, Los Alamos, NM 87545, USA.

References

1. Aliseda, A., Cartellier, A., Hainaux, F. & Lasheras, J. C. 2002 Effect of preferential concentration on the settling velocity of heavy particles in homogeneous isotropic turbulence. J. Fluid Mech. 468, 77–105.Google Scholar
2. Andrejczuk, M., Grabowski, W. W., Malinowski, S. P. & Smolarkiewicz, P. K. 2004 Numerical simulation of cloud clear air interfacial mixing. Atmos. Sci. 61, 17261739.Google Scholar
3. Ayyalasomayajula, S., Gylfason, A., Collins, L. R., Bodenschatz, E. & Warhaft, Z. 2006 Lagrangian measurements of inertial particle accelerations in grid generated wind tunnel turbulence. Phys. Rev. Lett. 97, 144507.Google Scholar
4. Bec, J., Biferale, L., Cencini, M., Lanotte, A., Musacchi, S. & Toschi, F. 2007 Heavy particle concentration in turbulence at dissipative and inertial scales. Phys. Rev. Lett. 98, 084502.Google Scholar
5. Broadwell, J. E. & Breidenthal, R. E. 1982 A simple model of mixing and chemical reaction in a turbulent shear layer. J. Fluid Mech. 125, 397–410.Google Scholar
6. Comte-Bellot, G. & Corrsin, S. 1971 Simple eulerian time correlation of full and narrow-band velocity signals in grid-generated ‘isotropic’ turbulence. J. Fluid Mech. 48, 273–337.CrossRefGoogle Scholar
7. Dávila, J. & Hunt, J. C. R. 2001 Settling of small particles near vortices and in turbulence. J. Fluid Mech. 440, 117–145.Google Scholar
8. Deardorff, J. W. 1974 Three-dimensional numerical study of turbulence in an entraining mixed layer. Boundary-Layer Meteorol. 7 (2), 199–226.Google Scholar
9. Friedman, P. D. & Katz, J. 2002 Mean rise rate of droplets in isotropic turbulence. Phys. Fluids 14, 3059–3073.Google Scholar
10. Gerashchenko, S., Good, G. & Warhaft, Z. 2011 Entrainment and mixing of water droplets across a shearless turbulent interface with and without gravitational effects. J. Fluid Mech. 668, 293–303.Google Scholar
11. Gerashchenko, S., Sharp, N. S., Neuscamman, S. & Warhaft, Z. 2008 Lagrangian measurements of inertial particle accelerations in a turbulent boundary layer. J. Fluid Mech. 617, 255–281.CrossRefGoogle Scholar
12. Hill, R. J. 2005 Geometric collision rates and trajectories of cloud droplets falling into a burgers vortex. Phys. Fluids 17, 037103.Google Scholar
13. Holtzer, G. L. & Collins, L. R. 2002 Relationship between the intrinsic radial distribution function for an isotropic field of particles and lower-dimensional measurements. J. Fluid Mech. 459, 93–102.Google Scholar
14. Ireland, P. J. & Collins, L. R. 2011 Direct numerical simulation of inertial particle entrainment in a shearless mixing layer. J. Fluid Mech. (submitted).Google Scholar
15. Jayesh, & Warhaft, Z. 1994 Turbulent penetration of a thermally stratified interfacial layer in a wind tunnel. J. Fluid Mech. 277, 23–54.Google Scholar
16. Kang, H. S. & Meneveau, C. 2008 Experimental study of an active grid-generated shearless mixing layer and comparisons with large-eddy simulation. J. Fluid Mech. 20, 125102.Google Scholar
17. Kantha, L. H., Phillips, O. M. & Azad, R. 1977 On turbulent entrainment at a stable density interface. J. Fluid Mech. 79, 753–768.Google Scholar
18. Kawanisi, K. & Shiozaki, R. 2008 Turbulent effects on the settling velocity of suspended sediment. J. Hydraul. Engng ASCE 134 (2), 261–266.CrossRefGoogle Scholar
19. Lázaro, B. J. & Lasheras, J. C. 1989 Particle dispersion in a turbulent, plane, free shear layer. Phys. Fluids 1, 1035–1044.Google Scholar
20. Longmire, E. K. & Eaton, J. K. 1992 Structure of a particle-laden round jet. J. Fluid Mech. 236, 217–257.CrossRefGoogle Scholar
21. Manton, M. J. 1974 On the motion of a small particle in the atmosphere. Boundary-Layer Meteorol. 6, 487–504.CrossRefGoogle Scholar
22. McQuarrie, D. A. 1976 Statistical Mechanics. Harper Row.Google Scholar
23. Mei, R. 1994 Effect of turbulence on the particle settling velocity in the nonlinear drag range. Intl J. Multiphase Flow 20, 273–284.CrossRefGoogle Scholar
24. Mordant, N., Crawford, A. & Bodenschatz, E. 2004 Experimental Lagrangian acceleration probability density function measurements. Physica D 193, 245–251.CrossRefGoogle Scholar
25. Murray, S. P. 1970 Settling velocities and vertical diffusion of particles in turbulent water. J. Geophys. Res. 75, 1647–1654.Google Scholar
26. Mydlarski, L. & Warhaft, Z. 1996 On the onset of high-Reynolds-number grid-generated wind tunnel turbulence. J. Fluid Mech. 320, 331–368.Google Scholar
27. Nielsen, P. 1993 Turbulence effects on the settling of suspended particles. J. Sedim. Petrol. 63 (5), 835–838.Google Scholar
28. Ouellette, N. T., Xu, H., Bourgoin, M. & Bodenschatz, E. 2006 A quantitative study of three-dimensional Lagrangian particle tracking algorithms. Exp. Fluids 40, 301–313.CrossRefGoogle Scholar
29. Salazar, J. P. L. C., de Jong, J., Cao, L., Woodward, S. H., Meng, H. & Collins, L. R. 2008 Experimental and numerical investigations of inertial particle clustering in isotropic turbulence. J. Fluid Mech. 600, 245–256.Google Scholar
30. Saw, E. W., Shaw, R. A., Ayyalasomayayajula, S., Chuang, P. Y. & Gylfason, Á. 2008 Inertial clustering of particles in high-Reynolds-number turbulence. Phys. Rev. Lett. 100, 214501.CrossRefGoogle ScholarPubMed
31. Shaw, R. A. 2003 Particle-turbulence interactions in atmospheric clouds. Annu. Rev. Fluid Mech. 35, 183–227.CrossRefGoogle Scholar
32. Shaw, R. A., Kostinski, A. B. & Larsen, M. L. 2002 Towards quantifying droplet clustering in clouds. Q. J. R. Meteorol. Soc. 128 (582), 1043–1057.Google Scholar
33. Shaw, R. A., Reade, W. C., Collins, L. R. & Verlinde, J. 1998 Preferential concentration of cloud droplets by turbulence: effects on the early evolution of cumulus cloud droplet spectra. J. Atmos. Sci. 55, 1965–1976.Google Scholar
34. Siebert, H., Gerashchenko, S., Gylfason, A., Lehmann, K., Collins, L. R., Shaw, R. A. & Warhaft, Z. 2010 Towards understanding the role of turbulence on droplets in clouds: in situ and laboratory measurements. Atmos. Res. 97, 426–437.CrossRefGoogle Scholar
35. Squires, K. D. & Eaton, J. K. 1991 Preferential concentration of particles by turbulence. Phys. Fluids A 3, 1169–1178.Google Scholar
36. Sundaram, S. & Collins, L. R. 1997 Collision statistics in an isotropic particle-laden turbulent suspension. Part 1. Direct numerical simulations. J. Fluid Mech. 335, 75–109.Google Scholar
37. Tooby, P. F., Gerald, L. W. & John, D. I. 1977 The motion of a small sphere in a rotating velocity field: a possible mechanism for suspending particles in turbulence. J. Geophys. Res. 82 (15), 2096–2100.Google Scholar
38. Townsend, A. A. 1976 The Structure of Turbulent Shear Flow. Cambridge University Press.Google Scholar
39. Turner, J. S. 1986 Turbulent entrainment: the development of the entrainment assumption, and its applications to geophysical flows. J. Fluid Mech. 173, 431–471.Google Scholar
40. Veeravalli, S. & Warhaft, Z. 1989 The shearless turbulence mixing layer. J. Fluid Mech. 207, 194–229.CrossRefGoogle Scholar
41. Voth, G. A., Porta, A. La, Crawford, A. M., Alexander, J. & Bodenschatz, E. 2002 Measurement of particle accelerations in fully developed turbulence. J. Fluid Mech. 469, 121–160.CrossRefGoogle Scholar
42. Wang, L. P. & Maxey, M. R. 1993 Settling velocity and concentration distribution of heavy particles in homogeneous isotropic turbulence. J. Fluid Mech. 256, 26–68.Google Scholar
43. Wood, A. M., Hwang, W. & Eaton, J. K. 2005 Preferential concentration of particles in homogeneous and isotropic turbulence. Intl J. Multiphase Flow 31, 1220–1230.Google Scholar
44. Yang, C. Y. & Lei, U. 1998 The role of turbulent scales in the settling velocity of heavy particles in homogeneous isotropic turbulence. J. Fluid Mech. 371, 179–205.Google Scholar
45. Yang, T. S. & Shy, S. S. 2005 Two-way interactions between solid particles and homogeneous air turbulence: particle settling rate and turbulence modification measurements. J. Fluid Mech. 526, 171–216.CrossRefGoogle Scholar