Published online by Cambridge University Press: 06 March 2017
Mountain-generated inertia–gravity waves (IGWs) affect the dynamics of both the atmosphere and the ocean through the mean force they exert as they interact with the flow. A key to this interaction is the presence of critical-level singularities or, when planetary rotation is taken into account, inertial-level singularities, where the Doppler-shifted wave frequency matches the local Coriolis frequency. We examine the role of the latter singularities by studying the steady wavepacket generated by a multiscale mountain in a rotating linear shear flow at low Rossby number. Using a combination of Wentzel–Kramers–Brillouin (WKB) and saddle-point approximations, we provide an explicit description of the form of the wavepacket, of the mean forcing it induces and of the mean-flow response. We identify two distinguished regimes of wave propagation: Regime I applies far enough from a dominant inertial level for the standard ray-tracing approximation to be valid; Regime II applies to a thin region where the wavepacket structure is controlled by the inertial-level singularities. The wave–mean-flow interaction is governed by the change in Eliassen–Palm (or pseudomomentum) flux. This change is localised in a thin inertial layer where the wavepacket takes a limiting form of that found in Regime II. We solve a quasi-geostrophic potential-vorticity equation forced by the divergence of the Eliassen–Palm flux to compute the wave-induced mean flow. Our results, obtained in an inviscid limit, show that the wavepacket reaches a large-but-finite distance downstream of the mountain (specifically, a distance of order  $(k_{\ast }\unicode[STIX]{x1D6E5})^{1/2}\unicode[STIX]{x1D6E5}$, where
$(k_{\ast }\unicode[STIX]{x1D6E5})^{1/2}\unicode[STIX]{x1D6E5}$, where  $k_{\ast }^{-1}$ and
$k_{\ast }^{-1}$ and 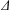 $\unicode[STIX]{x1D6E5}$ measure the wave and envelope scales of the mountain) and extends horizontally over a similar scale.
$\unicode[STIX]{x1D6E5}$ measure the wave and envelope scales of the mountain) and extends horizontally over a similar scale.