3.1. Interface perturbations

Given the known sensitivity of the behaviour of the RMI to the perturbations on the interface (Zhou Reference Zhou2017b), it is important to quantify the initial interface perturbations in these experiments, including the determination of whether the perturbations are single mode, narrowband or broadband in a spectral sense. These definitions have previously been introduced by Thornber et al. (Reference Thornber, Drikakis, Youngs and Williams2011, Reference Thornber, Drikakis, Youngs and Williams2012) to define an initial interface perturbation spectrum with a single wavelength, a narrow spectral bandwidth or a wide spectral bandwidth, respectively. The spectrum of the initial perturbation in these experiments is quantified by choosing 12 representative experiments that had a relatively large difference in particle seeding density between the heavy and light gases, making the interface between them possible to detect. Ideally, the seeding between the two gases should be similar in order to produce the best PIV data, and so most experiments had well-matched particle seeding densities such that visual interface detection was not possible, limiting the number of experiments that could be analysed in this fashion. Nonetheless, the initial interface spectra from these experiments with a larger difference in seeding density between the two gases should be similar to those with well-matched seeding density. The last image captured prior to the arrival of the incident shock wave is selected from each experiment to best capture the state of the interface at incident shock arrival. A single representative image from this set is shown in figure 3. The initial interface profile is detected utilizing the process described by Sewell et al. (Reference Sewell, Ferguson, Krivets and Jacobs2021) in which a strong Gaussian blurring operation is utilized to make the image intensity in each gas more uniform, and a Sobel edge-detection algorithm is used to identify the interface between the two gases in each column of pixels.

Figure 3. Experimental image depicting the initial perturbations just prior to incident shock arrival. Note that the contrast on this image has been increased to make the initial interface shape easier to identify. The distorted regions on the edge of the image are caused by the exhaust holes in the test section walls.

The spectrum of the initial perturbation for each of the initial interface profiles is found by applying a Hanning window to the profile and then taking the fast Fourier transform (FFT) of each profile, yielding one initial perturbation spectrum per experiment. The magnitude of each Fourier coefficient is then averaged across all 12 chosen experiments to produce a single average initial perturbation spectrum. This averaged spectrum is presented in figure 4 plotted as a function of wavenumber, $k$. Analysis of this spectrum reveals that ${\approx }60\,\%$ of the energy in the initial perturbation spectrum is located at wavenumbers of $k \lessapprox 0.7\,2{\rm \pi} \,\mathrm {mm}^{-1}$, with the spectrum being relatively constant in magnitude over this wavenumber range. This range is indicated by the red dashed line in figure 4. The wavenumber range of $0.7 \lessapprox k \lessapprox 6.9\,2{\rm \pi} \,\mathrm {mm}^{-1}$ contains another 39 % of the total energy, and the spectrum decays approximately according to $k^{-2}$ in this range. This range is indicated by the blue dashed line in figure 4. These two wavenumber ranges therefore contain 99 % of the total energy in this spectrum. A significantly steeper rate of decay is observed for $k \gtrapprox 6.9\,2{\rm \pi} \,\mathrm {mm}^{-1}$, with the remaining 1 % of the spectrum energy contained in these scales. Additionally, while this method of quantification of the interface does not capture the diffusion thickness of the two gases, work by Morgan (Reference Morgan2014) in a configuration similar to this one suggests that the diffusion layer should be approximately $6$ mm in thickness based on Rayleigh scattering measurements of a flat air–SF$_6$ interface.

Figure 4. The averaged spectrum of the initial perturbation of the interface just prior to the arrival of the incident shock. This average is taken across 12 experiments.

This spectrum is slightly steeper than the $\sim k^{-1.5}$ spectrum found in the experiments of Sewell et al. (Reference Sewell, Ferguson, Krivets and Jacobs2021) that was also used as the initial conditions for the simulations of Groom & Thornber (Reference Groom and Thornber2023). Those simulations also considered initial spectra of the form $k^{-0.5}$ and $k^{-1}$. The present perturbation spectrum is also steeper than the $k^{-1}$ utilized for the simulations of Thornber et al. (Reference Thornber, Drikakis, Youngs and Williams2011). The jet-based initial condition of Weber et al. (Reference Weber, Haehn, Oakley, Rothamer and Bonazza2014) (and others in that facility (Reese et al. Reference Reese, Oakley, Navarro-Nunez, Rothamer, Weber and Bonazza2014, Reference Reese, Ames, Noble, Oakley, Rothamer and Bonazza2018; Noble et al. Reference Noble, Herzog, Ames, Oakley, Rothamer and Bonazza2020a,Reference Noble, Herzog, Rothamer, Ames, Oakley and Bonazzab, Reference Noble, Ames, McConnell, Oakley, Rothamer and Bonazza2023)) had a spectrum based on scalar variance that was proportional to $k^{-1}$ at low wavenumbers and transitions to a $k^{-3}$ and later $k^{-5}$ decay at middle and high wavenumbers in their experiments. A relatively flat low wavenumber component and a $\sim k^{-2}$ spectrum at higher wavenumbers was also observed in the (tilt-compensated) initial condition of Mohaghar et al. (Reference Mohaghar, Carter, Musci, Reilly, McFarland and Ranjan2017, Reference Mohaghar, Carter, Pathikonda and Ranjan2019), with this spectrum similarly based on scalar fluctuations. Finally, gas curtain experiments have a much more regular and single-mode interface owing to the method of their formation (Jacobs Reference Jacobs1993; Jacobs et al. Reference Jacobs, Klein, Jenkins and Benjamin1993, Reference Jacobs, Jenkins, Klein and Benjamin1995; Balakumar et al. Reference Balakumar, Orlicz, Tomkins and Prestridge2008a,Reference Balakumar, Orlicz, Tomkins and Prestridgeb; Orlicz et al. Reference Orlicz, Balakumar, Tomkins and Prestridge2009; Balakumar et al. Reference Balakumar, Orlicz, Ristorcelli, Balasubramanian, Prestridge and Tomkins2012; Balasubramanian et al. Reference Balasubramanian, Orlicz, Prestridge and Balakumar2012; Orlicz et al. Reference Orlicz, Balasubramanian, Vorobieff and Prestridge2015).

3.2. Experimental progression

As discussed in previous sections, the PIV technique utilized in this work only resolves the velocity field of this flow as opposed to a concentration or density field. Therefore, methods of assessing the state and evolution of the RMI must be similarly velocity based. One useful metric for a velocity-based analysis is the magnitude of the component of vorticity normal to the image plane, $\omega = ( \boldsymbol {\nabla } \times \boldsymbol {u} ) \boldsymbol {\cdot } \hat {n}$. Considering that the passage of a shock wave through the interface results in deposited vorticity, and that there is no mean vorticity elsewhere in the test section, this metric will naturally highlight the regions where RMI-induced vorticity is present. Vorticity is calculated in the present work using the DaVis software package in which the gradients are calculated using a central difference scheme involving the four closest neighbours (i.e. the vectors above, below, left and right) of a given vector.

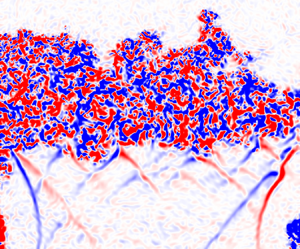
A montage of vorticity pseudocolour plots from an example experiment is shown in figure 5. The experiment presented in this montage is a heavy shock first experiment from experimental group 2. These composite images are the result of merging the individual PIV vector fields from four cameras oriented in a vertical stack to form one composite vector field. The experimental images used to generate these fields are captured at a rate of 1500 image pairs per second. Therefore, the time between each image in this montage is $1/1500 \approx 0.66$ ms.

Figure 5. A montage of PIV fields resulting from images captured during a single heavy shock first experiment from experimental group 2. The colour scale is shown at the bottom of the figure and is fixed across all images. Times of each image relative to reshock are (a) $-$2.90 ms, (b) $-$2.23 ms, (c) $-$1.56 ms, (d) $-$0.90 ms, (e) $-$0.23 ms, ( f) 0.43 ms, (g) 1.10 ms, (h) 1.76 ms, (i) 2.43 ms, ( j) 3.10 ms, (k) 3.76 ms, (l) 4.43 ms, (m) 5.10 ms, (n) 5.76 ms, (o) 6.43 ms, (p) 7.10 ms, (q) 7.76 ms, (r) 8.43 ms.

The interface location is initially at the bottom of the viewable area, which is indicated by the dashed line in figure 5(a). The interface is then impacted by the incident shock wave in figure 5(b), travelling upward from the heavy gas into the light gas, causing the interface to travel upward and initiating the RMI in the incident shock regime. The RMI then develops in figure 5(b–e) as the interface continues to travel upwards in the tube. The interface is impacted a second time by the shock wave arriving from the light gas travelling downwards into the heavy gas in figure 5( f), with this impact taking place approximately $\Delta t_{s \to rs} = 3.0$ ms after the incident shock arrival. The second shock wave arrival initiates the RMI in the reshock regime, as indicated by the increased intensity of vorticity on this fixed colour scale and the development of finer scales of turbulent motion as compared with the pre-reshock flow. This rapid transition from relatively ordered structures pre-reshock to a more disorganized structure has been observed in previous experiments (Balakumar et al. Reference Balakumar, Orlicz, Tomkins and Prestridge2008a, Reference Balakumar, Orlicz, Ristorcelli, Balasubramanian, Prestridge and Tomkins2012; Balasubramanian et al. Reference Balasubramanian, Orlicz, Prestridge and Balakumar2012; Mohaghar et al. Reference Mohaghar, Carter, Musci, Reilly, McFarland and Ranjan2017, Reference Mohaghar, Carter, Pathikonda and Ranjan2019; Sewell et al. Reference Sewell, Ferguson, Krivets and Jacobs2021). The RMI is then allowed to evolve in the reshock regime in figure 5(g–r). It can be observed that the bulk interface velocity is approximately halted, and the mixing layer is stationary in space as it evolves following the second shock interaction. Some large-scale structures may be observed in these images, though the randomness in the initial perturbation results in the spatial distribution of these larger structures being different for each experiment. The RMI continues to evolve in reshock for approximately 8 ms in this experiment. During this time, viscosity acts to dissipate energy in the mixing layer, indicated by the decreasing colour intensity on the fixed colour scale used here.

Aside from the RMI itself, there are a number of other features observable in figure 5 that are interesting to note. One immediately noticeable feature is the apparent thickness of the shock waves observed in the images. This occurs due to the transit of the shock wave between the first and second images in the PIV image pair. A similar phenomenon was also observed by Sewell et al. (Reference Sewell, Ferguson, Krivets and Jacobs2021).

Another feature worth pointing out in these images are the noticeable lines of vorticity lying below the mixing layer following reshock. There are both larger diagonal lines approximately the width of the test section, as well as smaller wedge-like structures visible. These lines of vorticity represent vortex sheets produced by velocity shear across the slip lines connected to triple points formed as the refracted shock wave passes through the mixing layer. The largest structures that span the test section width as well as the dome-shaped structures near the left and right walls are likely caused by the interaction of the incident shock wave with the boundary layers along the shock tube walls. These vortex sheets persist for the remainder of the experiment observation time. The smaller vortex sheets appear to be correlated with the location of the largest structures in the mixing layer at the time of reshock, suggesting that they are formed by the refraction of the shock wave as it transits the mixing layer. These structures are quickly obscured by the growing mixing layer shortly following reshock.

A final observation of importance in these images is the presence and growth of boundary layers on the side walls of the test section. The boundary layers are visible in these images as the camera fields of view capture the flow all the way to the test section walls. The boundary layers are initially very thin such that they are not visible in the incident shock regime and become significantly thicker, as well as potentially transitioning to turbulence, following reshock. This is more noticeable in the heavy gas region owing to the significantly lower kinematic viscosity there, resulting in a larger Reynolds number.

3.3. Mixing layer width

An important measurement in the study of the RMI is the growth of the width of the mixing layer versus time. Normally, the mixing layer width is defined by a concentration distribution of the two gases. However, as discussed above, the PIV diagnostic only resolves the velocity field and so methods for detecting the mixing layer must similarly be velocity based.

Sewell et al. (Reference Sewell, Ferguson, Krivets and Jacobs2021) used a method that identified the mixing layer as the region where spanwise-averaged TKE is greater than 5 % of its maximum value. A similar approach is utilized here to determine the extent of the mixing layer using elevated levels of spanwise-averaged plane normal enstrophy instead of TKE. Enstrophy was chosen for this work over TKE as the fact that there is no mean shear in this flow makes the separation of the fluctuating part of the field unnecessary and simplifies this stage of analysis. Spanwise averaging is required for this stage of the analysis as the changing shock-to-reshock times of these experiments, the run-to-run variation in shock wave strengths and the lack of density or concentration measurements make the methods for spatially aligning the results across different experiments for ensemble averaging imprecise. Previous simulations by Groom & Thornber (Reference Groom and Thornber2023) have shown that the mixing layer width defined using TKE will tend to be larger than the volume fraction based mixing layer width. Given that the enstrophy-based metric used in this work is similarly based on velocity, it is reasonable to expect that these mixing layer widths are also greater than the volume fraction based mixing layer width.

The mixing layer is identified through a series of steps. Figure 6 illustrates this automated process of identifying the mixing layer for a single frame in a single experiment. Figure 6(a) shows the vorticity field output from PIV processing. The left and right $2$-$3$ mm of the field is cropped to remove edge effects near the shock tube walls (black dashed line), with the cropped area set by the specific camera configuration used for the experiment. The image-plane normal component of vorticity is then squared to obtain enstrophy ($\omega ^2$), shown in figure 6(b). This enstrophy field is then averaged along the $x$ axis to generate a one-dimensional spanwise-averaged profile as shown in figure 6(c). Note that this profile has elevated values where the mixing layer is located and low values elsewhere. A boxcar moving average with a window size of 10 points (${\approx }3$ mm) of the spanwise-averaged profile is then taken to smooth the fluctuations in the data, shown as the red line in figure 6(d). This step is done to reduce the influence of noise in the data on the detection of the edge of the mixing layer, and provides smoother overall results. The maximum value of this smoothed profile and its corresponding position is then found, indicated by the green vertical line in figure 6(c). The search for the maximum value is restricted to a region visually identified as the mixing layer in the vorticity pseudocolour images, with this step performed to increase the robustness of the automated algorithm against random imperfections in the data far away from the mixing layer location (e.g. a small pocket of unseeded gas drifting into the camera view). Finally, the edges of the mixing layer are found by starting at the location of this maximum value and marching outwards until the value of the smoothed profile is less than 5 % of the maximum value. These locations, indicated by the pink and blue vertical lines in figure 6(c), are used to define the edges of the mixing layer, and the mixing layer width is defined as the distance between these edges. The influence of the choice of the 5 % threshold used here on the mixing layer width results is discussed in Appendix A.

Figure 6. (a) A single vorticity field from a PIV experiment. (b) Enstrophy field calculated from the vorticity field in (a). (c) The resulting row-averaged enstrophy profile. (d) Moving average profile (red) and locations of maximum value and mixing layer edges associated with moving average dropping below 5 % of the maximum value.

The result of this width finding process for a single experiment is shown as the black dots in figure 7. Note that this data appears to follow a power law of the form $h(t) \propto t^\theta$ (Alon et al. Reference Alon, Hecht, Mukamel and Shvarts1994). The parameter $\theta$ is found in the present work by plotting the logarithm of width versus the logarithm of time and fitting a line to the result. It should be acknowledged that utilizing a fit of this form implicitly assumes that $h=0$ at $t=0$, which is clearly not the case here, but is necessary due to complications that arise from attempting to fit a more general model equation that accounts for this discrepancy (e.g. $(h-h_0) = a (t - t_0)^\theta$) where unique solutions are often difficult to obtain. As a consequence, these fits focus on late-time data where the influence of this assumption is small. The result of this fit is shown in figure 7 using the red dashed line, which yields a value of $\theta = 0.314 \pm 0.025$ at 95 % confidence for this particular experiment. Points early in the experiment were excluded from this fit as these points do not appear to be well described by the model equation.

Figure 7. Measured interface mixing layer width from a single PIV experiment with a shock-to-reshock time of $\Delta t_{s\to rs}=2.0\,{\rm ms}$ on (a) linear and (b) log–log axes. The red line indicates the fit of $h(t) \propto t^\theta$ to the data, where $\theta = 0.314 \pm 0.025$ (95 %) is found for this experiment. Data points indicated by a black cross were excluded from the fit and points indicated by a blue cross in (a) correspond to the incident shock regime.

Prior studies have suggested a wide range of values for $\theta$, from as high as 2/3 (Barenblatt Reference Barenblatt1983) to as low as 0.18 (Zhou Reference Zhou2017a), though many of these studies did not consider the reshock case. As noted in § 1, a great deal of previous experimental work, models and simulations have observed or predicted linear growth of the RMI in reshock, making the observation of power law growth behaviour of the RMI in reshock in these experiments noteworthy. Other work has found power law growth of the RMI in reshock. Simulations by Tritschler et al. (Reference Tritschler, Olson, Lele, Hickel, Hu and Adams2014) found power law growth of the RMI in reshock where $\theta = 2/7 \approx 0.285$, while Thornber et al. (Reference Thornber, Drikakis, Youngs and Williams2011) found $\theta$ in the range of 0.28 for narrow bandwidth initial conditions to 0.36 for broad bandwidth initial conditions. Power law growth of the RMI in reshock in an experiment was observed by Sewell et al. (Reference Sewell, Ferguson, Krivets and Jacobs2021), who found values of $\theta$ between 0.33 and 0.5 in reshock for high and low amplitude experiments, respectively. Power law growth of the reshocked RMI has also been predicted by models (Mikaelian Reference Mikaelian2011; Morán-López & Schilling Reference Morán-López and Schilling2013, Reference Morán-López and Schilling2014; Mikaelian Reference Mikaelian2015; Mikaelian & Olson Reference Mikaelian and Olson2020; Schilling Reference Schilling2024). The present experiments generally agree well with this previous work, particularly the broad bandwidth simulations of Thornber et al. (Reference Thornber, Drikakis, Youngs and Williams2011), as well as the high amplitude initial perturbation experiments of Sewell et al. (Reference Sewell, Ferguson, Krivets and Jacobs2021). The simulations of Groom & Thornber (Reference Groom and Thornber2023), which were based on the shock tube experiments of Sewell et al., have found that $\theta$ found from a velocity-based mixing layer width agreed well with those based on integral or volume fraction based measurements, particularly for relatively broadband initial conditions as in these experiments.

Given the capabilities of the DDVST it is interesting to consider how the value of $\theta$ varies with shock-to-reshock time. The result of this process is shown in figure 8. The black dots shown are the values of $\theta$ found from fits of individual experiments, and the error bars show the 95 % confidence interval of the fits. Only fits that have $N>4$ points and $r^2 > 0.75$ are included in this plot to remove experiments with too little data to properly fit the model equation and which would yield large confidence intervals, as well as cases where the fit poorly describes the data. These fits show a significant amount of scatter owing to the sensitivity of the curve fitting process. Overall, despite this scatter, the average values of $\theta$ obtained from the heavy shock first experiments appear to be very similar to values obtained from the light shock first experiments. Furthermore, if it is assumed that the value of $\theta$ is, in an average sense, constant for each set of experiments, but that it has a different value for heavy shock first and light shock first experiments, a comparison can be made of the two cases. An average value of $\theta _{avg,H} = 0.365 \pm 0.018$ (95 %) is found for the heavy shock first experiments and $\theta _{avg,L} = 0.381 \pm 0.020$ (95 %) is found for the light shock first experiments. The standard deviation of the values of $\theta$ is $0.082$ and $0.057$ for the heavy shock first and light shock first experiments, respectively. In this comparison, the 95 % confidence interval is the uncertainty of the mean owing to data scatter, and does not include the uncertainty in the fits of each constituent measurement. The average value of $\theta$ for the heavy shock first cases is indicated by the red dashed line in figure 8, and for the light shock first cases by the blue dashed lines on figure 8. The shaded areas indicate the 95 % confidence bound of the respective mean values. The average value of these two cases are similar, and both averages lie within the 95 % confidence interval of the other average. This result is qualitatively in agreement with the results of Brouillette & Sturtevant (Reference Brouillette and Sturtevant1989), who found that the heavy and light shock first experiments had slightly different linear growth rates but that these differences were also within the uncertainty bounds. Previous experiments by Sewell et al. (Reference Sewell, Ferguson, Krivets and Jacobs2021) found $\theta =0.33\pm 0.07$ and $\theta =0.50\pm 0.07$ in reshock for their high and low amplitude initial perturbation, respectively. The present work agrees well with the high amplitude results, though the value of $\theta$ is slightly lower than the low amplitude initial perturbation results. Additionally, the relatively short post-reshock observational period in those results could also influence this comparison. The simulations of Thornber et al. (Reference Thornber, Drikakis, Youngs and Williams2011, Reference Thornber, Drikakis, Youngs and Williams2012) found $\theta =0.28$ to $\theta =0.36$ for narrowband and broadband initial conditions, respectively, with the latter case agreeing well with the relatively broadband perturbations in these experiments.

Figure 8. Values of $\theta$ found via a linear least-squares fit of enstrophy width for each experiment versus that experiment's shock-to-reshock time. The horizontal lines indicate average values of $\theta$, separated by heavy shock first and light shock first experiments, and the shaded areas represent the 95 % confidence interval of the associated mean.

3.4. Rate of decay of TKE

Many turbulent flows have a constant source of energy input such that the amount of TKE per unit mass will grow with time until eventually the rate of energy addition is matched by the rate that it is dissipated by viscosity. This is not the case with RMI, in which the entirety of the energy is added as the shock wave passes through the interface. The energy then freely decays in time until it is entirely dissipated by viscosity. For this reason, it is useful to examine the total amount of TKE within the mixing layer as it decreases with time and the rate at which this energy decays.

The TKE per unit mass is calculated from the fluctuating components of the turbulent velocity. Variables in turbulent flows can be decomposed in to a mean component plus a component representing fluctuations about that mean. Therefore, the total velocity resolved by the PIV diagnostic must be decomposed into its mean and fluctuating components in order to calculate TKE. In uniform density flows this is performed using the Reynolds decomposition, defined as $f = \bar {f} + f'$, where $\bar {f}$ is the mean and $f'$ is the fluctuations of $f$ about $\bar {f}$. For non-uniform density flows, such as the RMI, this decomposition is instead commonly performed using the Favre, or mass-weighted, decomposition to account for spatial variations in density. Importantly, however, the Favre decomposition requires the density field that can not be obtained using the PIV diagnostic, and so the Reynolds decomposition must be used for the present analysis. It should be noted that variable density flows introduce a number of complexities to a turbulent flow stemming from the non-uniform density field (Charonko & Prestridge Reference Charonko and Prestridge2017; Lai, Charonko & Prestridge Reference Lai, Charonko and Prestridge2018). As a consequence, the influence of Reynolds averaging on these results should be considered. Work by Mohaghar et al. (Reference Mohaghar, Carter, Musci, Reilly, McFarland and Ranjan2017) as well as Charonko & Prestridge (Reference Charonko and Prestridge2017) has shown that while a Reynolds averaging approximation does introduce a small error versus a Favre average, this difference is only a few percent of the total value. The TKE is defined here as one half of the trace of the Reynolds stress tensor (Pope Reference Pope2000). The TKE corresponding to the three components of the flow velocity is

(3.1a–c)\begin{equation} k_u = \tfrac{1}{2}(u - \bar{u})^2 = u^{'2},\quad k_v = \tfrac{1}{2}(v - \bar{v})^2 = v^{'2},\quad k_w = \tfrac{1}{2}(w - \bar{w})^2 = w^{'2}, \end{equation}

where the mean part of each velocity component is defined as the average value of that velocity component within the mixing layer. This method of determining the mean flow was chosen due to the difficulties associated with ensemble averaging noted in § 3.3. The TKE field is defined in terms of these components as

(3.2)\begin{equation} K = k_u + k_v + k_w. \end{equation}

Another aspect to consider in this analysis is that the two-dimensional PIV technique used in this study does not resolve the out-of-plane horizontal component of velocity, $w$. To address this, an assumption is made that, in a statistical sense, the in-plane (resolved) horizontal component of fluctuating velocity is similar to the out-of-plane (unresolved) horizontal component of fluctuating velocity, i.e. $u' \approx w'$. This assumption has been used in other experiments with a two-dimensional PIV diagnostic to account for the out-of-plane component of velocity (Mohaghar et al. Reference Mohaghar, Carter, Musci, Reilly, McFarland and Ranjan2017, Reference Mohaghar, Carter, Pathikonda and Ranjan2019; Sewell et al. Reference Sewell, Ferguson, Krivets and Jacobs2021). The assumption of statistical homogeneity along the horizontal axes in RMI flows is common in simulations (for example, Thornber et al. Reference Thornber, Drikakis, Youngs and Williams2011, Reference Thornber, Drikakis, Youngs and Williams2012; Tritschler et al. Reference Tritschler, Olson, Lele, Hickel, Hu and Adams2014; Oggian et al. Reference Oggian, Drikakis, Youngs and Williams2015; Groom & Thornber Reference Groom and Thornber2018, Reference Groom and Thornber2019, Reference Groom and Thornber2023). Therefore, TKE in this study is defined as

(3.3)\begin{equation} K = 2 k_u + k_v. \end{equation}

Volume integrated specific TKE is then calculated by integrating $K$ over the mixing layer,

(3.4)\begin{equation} \frac{E_{tot}}{\bar{\rho}} = L_z \iint K\,{{\rm d}\kern0.06em x}\,{{\rm d} y}, \end{equation}

where $L_z=88.9$ mm is the out-of-plane depth of the test section and $x$ and $y$ are the cross-stream and streamwise directions as indicated in figure 6. Volume integrated specific TKE is used here due to the lack of density measurements in these experiments. The decay of volume integrated specific TKE is presented in log–log form for a single experiment in figure 9. Here the black dots represent the value of $E_{tot}$ at each time instance for this particular experiment. The experimental data appears to fall in a straight line on this log–log plot, suggesting that the volume integrated specific TKE in the mixing layer decays according to a power law. Thus, a form for this decay of $E_{tot} \propto t^p$ will be assumed, where $a$ and $p$ are coefficients to be found from a fit to the data. The fit is performed identically to the fit of mixing layer width versus time described above, with the data plotted as $\log (E_{tot})$ vs $\log (t)$ and a straight line fit to the result. The fit for this particular experiment is indicated by the red dashed line, and has a slope of $p = -0.834$ with a 95 % confidence interval of the fitted value of $\pm 0.077$.

Figure 9. Volume integrated specific TKE versus time for a single PIV experiment on (a) linear and (b) log–log axes. The red dashed line indicates a fit of $E_{tot} \propto t^p$ to the data, where $p=-0.834 \pm 0.077$ at 95 % confidence for this experiment. Black crosses indicate data points that were excluded from the fit.

Thornber et al. (Reference Thornber, Drikakis, Youngs and Williams2010) has shown that the decay exponent of TKE, $p$, for RMI can be related to the growth exponent, $\theta$, deriving the expression $p = 3 \theta _{tke} - 2$, where the subscript in $\theta _{tke}$ is meant to differentiate this value of $\theta$ from the one found from the mixing layer width. A similar result for volume integrated TKE was also found by Schilling (Reference Schilling2021). This allows a comparison between these results and those in § 3.3, though it should be noted that the definition of TKE used by Thornber et al. (Reference Thornber, Drikakis, Youngs and Williams2010) to find $\theta$ from TKE decay contains a spatially varying density profile, whereas the present results do not have this density data available. The experiment shown in figure 9, for example, has a fitted slope of TKE decay of $p = -0.834\pm 0.077$, which corresponds to $\theta _{tke} = (-0.834\pm 0.077 + 2)/3 \approx 0.388 \pm 0.026$.

As with the mixing layer width measurements, it is interesting to consider the effect of shock-to-reshock time on the volume integrated specific TKE decay rate. Figure 10 shows values of the slope of volume integrated specific TKE decay, $p$, versus shock-to-reshock time indicated by the black dots along with the equivalent values of $\theta _{tke} = (p + 2)/3$ on the right axis. As in figure 8, the 95 % confidence interval of each fit is indicated by the error bars. There is again a significant amount of scatter that can be observed in this data. However, the amount of scatter along with the size of the error bars are noticeably smaller than was observed for the width-based measurements in figure 8. A major source of uncertainty in the mixing layer width measurements is believed to arise from the emergence of large-scale flow structures, such as a single large mushroom ejected from the mixing layer. The mixing layer width finding process will detect the emergence of these larger structures as the edge of the mixing layer and, consequently, the measurement of mixing layer width will be overly influenced by these structures. Thus, one might expect a larger run-to-run variation in $\theta$ measurements depending on the presence of the larger structures. On the other hand, the total amount of TKE, and the rate at which it decays, is arguably less influenced by the emergence of new structures in the flow. This results in less run-to-run variation in the volume integrated specific TKE decay measurements, as well as less uncertainty in the fit of the data from each experiment.

Figure 10. Values of the rate of TKE decay and corresponding values of $\theta _{tke}$ found via a linear least-squares fit of volume integrated specific TKE in the mixing layer versus time for each experiment versus that experiment's shock-to-reshock time. The horizontal lines indicate average values of $p$, separated by heavy shock first and light shock first experiments, and the shaded areas represent the 95 % confidence interval of the associated mean.

Similar to as was done for the mixing layer growth exponent, the assumption may be made that $p$ has a constant value for the heavy shock first and light shock first experiments, but that these values may be different for the two cases. This allows a mean, and associated 95 % confidence interval of that mean, to be found for each of the two groups of experiments. The mean value for the heavy shock first experiments is indicated by the red dashed line and for the light shock first experiments is indicated by the blue dashed line in figure 10. The 95 % confidence intervals of each mean are indicated by the shaded areas. The rate of decay of volume integrated specific TKE for the heavy shock first experiments is found to be $p_{avg,H} = -0.823 \pm 0.06$ (95 %) and, for the light shock first experiments, $p_{avg,L} = -1.061 \pm 0.032$ (95 %). The standard deviation in the values of $p$ is $0.175$ and $0.127$ for the heavy shock first and light shock first experiments, respectively. As with the average values of $\theta$ from mixing layer width measurements, the 95 % confidence interval here arises from the 95 % confidence of the mean and does not include the uncertainty of the fit of the constituent points. These average values of $p$ correspond to values of $\theta _{tke}$ of $\theta _{tke,avg,H} = 0.392 \pm 0.02$ (95 %) and $\theta _{tke,avg,L} = 0.313 \pm 0.011$ (95 %) for the heavy and light shock first experiments, respectively. Notably, the 95 % confidence interval of $\theta _{tke,avg,H}$ overlaps with the 95 % confidence band of $\theta _{avg,H} = 0.365 \pm 0.018$ from the mixing layer width in the heavy shock first case, though a similar overlap is not observed in the light shock first case. Note that the average values of $p$ found here are more negative than the values of $p_{high} = -0.62$ and $p_{low} = -0.14$ for high and low amplitude initial conditions found by Sewell et al. (Reference Sewell, Ferguson, Krivets and Jacobs2021), though this is likely at least in part due to the much longer post-reshock observational window in these experiments. One important difference between figures 10 and 8 is that there is now a significant difference between the heavy shock first and light shock first volume integrated specific TKE decay measurements and that this difference exceeds the 95 % confidence values of the mean.

It is important to recognize that the difference in the values of $p$ between the two groups could be the result of the lack of density/concentration measurements in these experiments. Recall in (3.4) that (1) Favre averaging was not used when calculating the TKE field, and (2) a density field is not used in calculating the TKE. As a consequence, there is an implicit assumption that the relationship between the distributions of density and velocity over time is the same for the light shock first and heavy shock first cases. It should also be noted that the influence of variable density effects may also produce different behaviours in the heavy shock first and light shock first cases and contribute to this observed behaviour (Charonko & Prestridge Reference Charonko and Prestridge2017; Lai et al. Reference Lai, Charonko and Prestridge2018). Further examination of the rate of decay of TKE in this configuration, particularly where the first shock arrives from the heavy gas, via simulation, or experiment with the addition of density measurements, would help clarify this.

3.5. Reynolds number

The Reynolds number is a dimensionless parameter defined as the ratio of inertial to viscous forces, commonly defined in RMI type flows as ${\textit {Re}} = h \dot {h} / \nu$, though alternative definitions have been proposed for different phases of RMI growth (Orlicz et al. Reference Orlicz, Balasubramanian, Vorobieff and Prestridge2015). Here, $h$ is the mixing layer width, $\dot {h}$ is the growth rate of the mixing layer and $\nu = \nu _{avg}$ is the average kinematic viscosity of the two pure fluids on either side of the mixing layer. Each of these quantities can be found through known properties of the two pure fluids ($\rho$ and $\mu$, $\therefore \nu _{avg}$) or from direct measurements of the data ($h$, $\dot {h}$). The determination of the growth rate, $\dot {h}$ is complicated slightly by the fact that it requires taking a derivative of noisy experimental data, which in turn tends to result in still noisier derivatives. This is somewhat mitigated in this work by using a line fit to multiple neighbouring points to determine $\dot {h}$, which will smooth the high frequency noise in the Reynolds number while retaining the low frequency behaviour, though noisy results remain a consistent issue.

Figure 11 shows a plot of Reynolds number versus time for a single experiment. Note that there are large fluctuations in the value of the Reynolds number observed in this plot that is due to the use of a numerical derivative of noisy data as discussed above. The Reynolds number is ${\textit {Re}} \approx 6 \times 10^4$ shortly after reshock in this case, and decays to a somewhat smaller value of ${\textit {Re}} \approx 3\times 10^4$ towards the end of the experiment, though the scatter in the data makes a precise value difficult to determine. Two reference lines are also indicated on this plot. The red dashed line arises from a fit of a power law of the form ${\textit {Re}} \propto t^r$ to this data, where $r=-0.20$ is the fitted value. The blue dotted line arises from the expected decay of Reynolds number with time arising from the assumption of self-similarity (i.e. $h \propto t^\theta$, ${\textit {Re}} = h \dot {h}/\nu _{avg} \therefore {\textit {Re}}_\theta \propto t^{2\theta -1}$), where a value of $\theta =0.37$ is used based on the average value of $\theta$ found in figure 8.

Figure 11. Reynolds number versus time for a single experiment with a shock-to-reshock time of $\Delta t_{s \to rs} = -0.76$ ms. The red dashed line indicates a fit of a power law of the form $a t^r$ to the data, where $a=5.79\times 10^4$ and $r=-0.20$. The blue dotted line indicates the expected decay of the Reynolds number assuming self-similarity (i.e. $h \propto t^\theta$, ${\textit {Re}} = h \dot {h}/\nu _{avg} \therefore {\textit {Re}}_\theta \propto t^{2\theta -1}$), where $\theta =0.37$.

In order to assess the effect of shock-to-reshock time, the value of the Reynolds number may also be examined in a similar manner to as how the influence of the shock-to-reshock time on mixing layer growth and volume integrated specific TKE decay were analysed. An average Reynolds number may be found for each experiment by taking the average of all data points in the first $5$ ms following reshock. An uncertainty in this value can also be calculated from the uncertainty of the mean, which captures the influence of the level of noise present in the underlying data as well as the decay in the value of the Reynolds number over the 5 ms window. Figure 12 shows this average value of the Reynolds number for each experiment versus that experiment's shock-to-reshock time. The error bars indicate the associated 95 % confidence interval of the value of the average. It can be observed in this figure that experiments with shorter shock-to-reshock times of $|\Delta t_{s \to rs}| \lessapprox 1.0$ ms demonstrate a sharp decrease in average Reynolds number as shock-to-reshock time decreases, approaching a minimum at simultaneous shock and reshock arrival, i.e. when $\Delta t_{s \to rs}$ approaches zero. Some experiments in this limit were observed to demonstrate a very low growth of the mixing layer, with this behaviour attributed to the cancellation of vorticity owing to the short period of time between the arrival of the incident and secondary shock waves (Mikaelian Reference Mikaelian1985, Reference Mikaelian1989). An example of such an experiment in the present dataset is presented in Appendix B. In contrast, experiments with a shock-to-reshock time of $|\Delta t_{s \to rs}| \gtrapprox 1.0$ ms achieve a similar average value of the Reynolds number of ${\textit {Re}}_{avg} \approx 1 \times 10^5$, with this value observed across a range of shock-to-reshock times. While the observed trend towards a minimum value of average Reynolds number for small shock-to-reshock times is unsurprising as one may expect less growth of the mixing layer in this limit, it is interesting to note that the Reynolds number does not continue to increase with increasing shock-to-reshock time for $|\Delta t_{s \to rs}| > 1.0$ ms. A possible explanation for this behaviour is that the growth of short wavelength perturbations in the incident shock regime saturate relatively quickly. This would result in the amplitudes of the perturbations just prior to reshock changing relatively little beyond a certain shock-to-reshock time, and this may in turn result in relatively little change of the post-reshock Reynolds number as well. Finally, the post-reshock outer-scale Reynolds number appears to be insensitive to the order of arrival of the two shock waves, with experiments having both positive and negative values of shock-to-reshock time achieving approximately the same value of average Reynolds number.

Figure 12. Average value of Reynolds number versus shock-to-reshock time for the first $5$ ms post-reshock. Error bars represent the 95 % confidence interval of the mean. The blue and red lines indicate the suggested critical Reynolds numbers for transition to turbulence from Dimotakis (Reference Dimotakis2000) and Zhou (Reference Zhou2007), respectively.

The Reynolds number is a useful metric to establish whether these experiments have achieved a fully turbulent state. Dimotakis (Reference Dimotakis2000) indicates that an outer-scale Reynolds number with a value greater than ${\textit {Re}} > 1 \times 10^4$ is required in order to achieve the transition to turbulent mixing, with this relationship having been found by considering a number of different turbulent flows, not just the RMI. Zhou (Reference Zhou2007) suggests a critical Reynolds number for the transition to turbulent mixing to be $1.6 \times 10^5$ for time-dependent laboratory-scale flows. This value was determined by calculating the minimum Reynolds number required to generate an inertial range with sufficient separation between the large and small scales such that some part of the developing inertial range would be unaffected by either the largest or smallest scales of the flow. These critical values suggested by Dimotakis and Zhou are indicated in figure 12 by the blue and red lines, respectively. The Reynolds numbers observed in the present study are in excess of the Reynolds number suggested by Dimotakis. On the other hand, the average Reynolds number for most experiments in the present study fall below the value suggested by Zhou. This suggests that while the present experiments may be approaching a turbulent state, they are not ‘fully turbulent’ according to the definition provided by Zhou (Reference Zhou2007).

3.6. Turbulent length scales

Another method to assess the degree to which a flow is turbulent involves estimating the size of the largest and smallest scales in the inertial range. The present work considers the scales defined by Dimotakis (Reference Dimotakis2000) and Zhou, Robey & Buckingham (Reference Zhou, Robey and Buckingham2003), which are given by

(3.5)\begin{equation} \left. \begin{aligned} &\lambda_L \simeq 5 \textit{Re}^{{-}1/2} h ,\\ &\lambda_D \simeq C (\nu t)^{1/2} ,\\ &\lambda_\nu \simeq 50 \textit{Re}^{{-}3/4} h ,\\ &\lambda_K \simeq \textit{Re}^{{-}3/4} h ,\\ \end{aligned} \right\} \end{equation}

where these scales are in order of decreasing size for a fully turbulent flow. Here, $\lambda _L$ is the Liepmann–Taylor scale, $\lambda _D$ is the time-dependent outer-scale viscous shear layer scale, $\lambda _\nu$ is the inner viscous scale and $\lambda _K$ is the Kolmogorov scale.

These length scales may be utilized to quantify the transition to turbulence in several ways. Three possible criteria will be considered here. The first criterion is $\lambda _L > \lambda _\nu$, suggested by Dimotakis (Reference Dimotakis2000), and is arrived at by noting that the largest scales in the inertial range, $\lambda _L$, must be greater than the smallest scales in the inertial range, $\lambda _\nu$, in order to have an inertial range and, thus, fully developed turbulence. The second criterion is the Zhou–Robey criterion, $\lambda _{L,min} \equiv \min (\lambda _L, \lambda _D) > \lambda _\nu$, defined by noting that the time-dependent outer-scale viscous shear layer scale, $\lambda _D$, introduced by Zhou et al. (Reference Zhou, Robey and Buckingham2003) adds an additional scale by which the upper bound of the inertial range may be specified. Therefore, Zhou et al. defined the upper bound of the inertial range to be the smaller of the two scales. The time dependence of $\lambda _D$ and, therefore, of this transition criterion, is of particular note as it suggests that a transition to turbulence can still occur even after the shock wave has passed through the interface and the RMI has begun to decay due to the time needed to form these scales of motion. Third, Lombardini, Pullin & Meiron (Reference Lombardini, Pullin and Meiron2012) suggests that the ratio required for a full separation of scales and transition to turbulence is $\lambda _L / \lambda _\nu \geq 10$, and notes that this threshold was only met for $M \geq 3$ in their simulations.

Estimates of the turbulent length scales given in (3.5) were computed for all experiments presented in this work. The length scale size estimates for experiments with a shock-to-reshock time of $|\Delta t_{s \to rs}| > 1.0$ ms are shown pointwise in figure 13(a) and in a moving average sense in figure 13(b). The length scale size estimates for experiments with a shock-to-reshock time of $|\Delta t_{s\to rs}| > 1.0$ ms are shown pointwise in figure 13(c) and in a moving average sense in figure 13(d). Also shown in figure 13(b,d) are estimated length scale sizes based on the assumption of self-similarity (i.e. $h \propto t^\theta$, ${\textit {Re}} = h \dot {h}/\nu _{avg} \therefore {\textit {Re}}_\theta \propto t^{2\theta -1}$) in (3.5), where a value of $\theta =0.37$ is used based on the average value of $\theta$ found in figure 8. Notably, the average length scales do appear to be well described by the self-similar growth assumption, particularly at middle to late times in the experiment.

Figure 13. Length scale size versus time for experiments where (a,b) $1.0 \geq |\Delta t_{s \to rs}| > 0.3$ ms and (c,d) $|\Delta t_{s \to rs}| > 1.0$ ms, in a (a,c) pointwise and (b,d) moving average sense. Colours correspond to $h$ (black), $\lambda _L$ (red), $\lambda _\nu$ (blue), $\lambda _D$ (green) and $\lambda _K$ (cyan). The window size of the moving average is $1/1500$ s. The coloured dashed lines in (b) and (d) indicate predicted length scales based on self-similarity (i.e. $h\propto t^\theta, {\textit {Re}}={\textit {Re}}_\theta$) where $\theta =0.37$, and the horizontal black dashed line indicates the average experimental resolution, $\Delta x_{avg} = 0.27\,\textrm {mm}\,\textrm {vector}^{-1}$.

As described above, there are three transition criteria to consider when viewing this data. It is clearly observable for both groups of experiments in figure 13, particularly in the moving average sense, that the transition criterion of Dimotakis is satisfied for the duration of the experiment, though the experiments with a short shock-to-reshock time have noticeably less separation between these two scales than the experiments with a long shock-to-reshock time. The moving average data for the experiments with a short shock-to-reshock time suggests that the Zhou–Robey transition criteria is not satisfied for this group of experiments. In contrast, the experiments with a long shock-to-reshock time do satisfy the Zhou–Robey transition criteria for a short period of time, approximately 3–4 ms after reshock. The pointwise data, however, shows that individual experiments in both groups may satisfy this transition criteria for a longer period of time than the moving average data would suggest. It is important to note, however, that even though the Zhou–Robey transition criteria is satisfied, there is only minimal separation between the largest and smallest scales in the inertial range. The third criterion is $\lambda _L / \lambda _\nu > 10$ as suggested by Lombardini et al. (Reference Lombardini, Pullin and Meiron2012). This ratio is found to be between approximately 1.5 and 1.8 for experiments with a short and long shock-to-reshock time, respectively, and so does not satisfy this criterion. It should be noted, however, that Orlicz et al. (Reference Orlicz, Balasubramanian, Vorobieff and Prestridge2015) finds almost an order of magnitude difference in estimates of $\lambda _L / \lambda _\nu$ in their gas curtain experiments depending on whether these length scales were estimated from the outer-scale Reynolds number or from measured values of the Taylor and Kolmogorov length scales and relationships between these scales and $\lambda _L$ and $\lambda _\nu$. They note that this ambiguity could lead to different conclusions depending on the scaling used, indicating ambiguity in the definition of a transition to turbulence using only Reynolds numbers. Thus, further analysis via the spectra of flow scales is useful to examine if, and to what degree, this flow has formed an inertial range of scales.

3.7. Spectrum of TKE

The spectrum of TKE is a tool by which the energy content of a turbulent flow as a function of scale size may be examined. One method by which the TKE spectrum may be calculated is through the use of the FFT algorithm. The procedure used here for calculating this spectrum for a single time instance follows the one described by Latini, Schilling & Don (Reference Latini, Schilling and Don2007), except that the calculations in this case are not weighted by density. This is also similar to the calculation performed by Sewell et al. (Reference Sewell, Ferguson, Krivets and Jacobs2021). The FFT of the fluctuating part of each component of velocity is taken in the $x$ direction, across the test section, at each $y$ position in the mixing layer. The component FFTs are then multiplied by their complex conjugates to obtain the spectrum of each component of TKE,

(3.6a,b)\begin{equation} \widehat{k_u} = \widehat{u^{'}}\widehat{u^{'}}^*,\quad \widehat{k_v} = \widehat{v^{'}}\widehat{v^{'}}^*, \end{equation}

where $\widehat {k_u}$ is the spectrum of TKE magnitude of the horizontal component of velocity, $u$, $\widehat {({\cdot })}$ indicates a Fourier transform and $({\cdot })^*$ indicates a complex conjugate. A similar definition for the vertical component of velocity, $\widehat {k_v}$ is also defined. The values from each velocity component are then combined to form the TKE spectrum as

(3.7)\begin{equation} \hat{K} = \left( 2 \widehat{k_u} + \widehat{k_v} \right), \end{equation}

where the factor of $2$ on $\widehat {k_u}$ is used to account for the out-of-plane component of horizontal velocity, which is assumed to be similar to the in-plane component. This follows similar logic as that used for adding the factor of $2$ to the $u$ component of TKE in the calculation of volume integrated specific TKE in § 3.4. This process is then repeated for all $y$ positions in the mixing layer to obtain one TKE spectrum per $y$ position. The magnitude of each Fourier coefficient is then averaged at each wavenumber across the mixing layer, resulting in an average one-dimensional TKE spectrum for each time instant,

(3.8)\begin{equation} \bar{\hat{K}} = \left(\overline{ 2 \widehat{k_u} + \widehat{k_v} } \right), \end{equation}

where $\overline {( {\cdot } )}$ indicates an average over the mixing layer. These spectra are further ensemble averaged across groups of experiments, with ensemble averages indicated using angle brackets, $\langle {\cdot } \rangle$. In order to ascertain the effects of the shock-to-reshock time and the order of arrival of the two shock waves on the spectrum of TKE, the present experimental results are divided into four groups. These groups correspond to a large negative shock-to-reshock time ($\Delta t_{s \to rs} \leq -1.0$ ms), a small negative shock-to-reshock time ($-1.0 < \Delta t_{s \to rs} \leq -0.3$ ms), a small positive shock-to-reshock time ($0.3 \leq \Delta t_{s \to rs} < 1.0$ ms) and a large positive shock-to-reshock time ($\Delta t_{s \to rs} \geq 1.0$ ms). An ensemble average of the anisotropy ratio of the experiments in each group are then taken, with ensemble averages indicated using angle brackets, $\langle {\cdot } \rangle$. The ensemble average is calculated using a boxcar moving average to translate all experimental data points on to a common set of time and wavenumber coordinates. In addition, this process only averages the data that falls within a specified window of time and wavenumber about a given point, and no interpolation of the data is performed. The half-width of the temporal window is $1/3000$ s, corresponding to a total temporal window size equivalent to the lowest imaging frame rate of 1500 frames per second. The half-width of the wavenumber window is ${\rm \pi} 115 / 85\,\textrm {mm}^{-1}$, corresponding to half of the spectral resolution of the lowest resolution experiments. The range of wavenumbers used in these averages is likewise limited to the resolvable range of the lowest resolution experiments. Each TKE spectrum from each experiment is normalized by the total energy of the spectrum prior to averaging.

The ensemble-averaged spectrum of TKE, compensated by the Kolmogorov $k^{-5/3}$ scaling, over the four ranges of shock-to-reshock time are presented in figure 14. This compensation results in regions of the spectra that follow a Kolmogorov-type $k^{-5/3}$ scaling with wavenumber appearing as a flat, horizontal line. A fiducial representing a $k^{-3/2}$ type spectrum as suggested by Zhou (Reference Zhou2001) is also shown in each plot as a red dashed line. Each greyscale line represents the ensemble-averaged TKE amplitude spectrum for a single time instant in the ensemble. The grey value of each line represents the length of time elapsed past reshock, with black representing the latest time in the experiment.

Figure 14. Ensemble-averaged compensated spectra for four groups of experiments. These four groups correspond to (a) large negative, (b) small negative, (c) large positive and (d) small positive shock-to-reshock times. The red dashed lines indicate a $-3/2$ slope of decay of TKE with wavenumber as suggested by Zhou (Reference Zhou2001), and the blue dashed lines indicate a Kolmogorov $-5/3$ slope of decay.

There are several aspects of note in the plots shown in figure 14. First, all four groups of experiments exhibit a $\approx -3/2$ type scaling at early times after reshock, in agreement with the suggested decay rate for an RMI-induced turbulent flow of Zhou (Reference Zhou2001). The experiments with a longer shock-to-reshock time (plots a,c) then shift towards a $\approx -5/3$ decay rate at later times in the experiment as indicated by the TKE spectrum flattening and becoming horizontal over time in these compensated plots. Interestingly, such a shift in the spectral content of the mixing layer is not observed for experiments with a shorter shock-to-reshock time (plots b,d), where a $\approx -3/2$ spectrum is maintained for the duration of the experiment. These observations are consistent with the observation from figure 13 that shows the scales representing the upper and lower bounds of the inertial range are beginning to separate in the experiments with longer shock-to-reshock times, while such a separation is not as evident in experiments with shorter shock-to-reshock times. The appearance of nearly a decade of wavenumbers following a $k^{-5/3}$ decay at later times in the long shock-to-reshock time experiments is interesting to note, as this indicates the formation of an inertial range of scales and a transition to turbulence that was not suggested by the Reynolds number or length scale analyses in §§ 3.5 and 3.6. This echoes the ambiguity noted by Orlicz et al. (Reference Orlicz, Balasubramanian, Vorobieff and Prestridge2015) who suggested that an RMI flow may not be ‘fully turbulent’ as defined by a Reynolds number-based analysis while at the same time the turbulent spectra indicate the formation of an inertial range of scales, suggesting the flow is in fact ‘fully turbulent.’ Similar results depicting a $k^{-5/3}$ decay of TKE have been noted in other experiments (Mohaghar et al. Reference Mohaghar, Carter, Musci, Reilly, McFarland and Ranjan2017; Sewell et al. Reference Sewell, Ferguson, Krivets and Jacobs2021), though a slightly steeper rate of decay of $-1.8$ to $-2.2$ was found by Mohaghar et al. (Reference Mohaghar, Carter, Pathikonda and Ranjan2019). A $k^{-3/2}$ to $k^{-5/3}$ decay has also been observed in simulation (Thornber et al. Reference Thornber, Drikakis, Youngs and Williams2011; Tritschler et al. Reference Tritschler, Olson, Lele, Hickel, Hu and Adams2014; Oggian et al. Reference Oggian, Drikakis, Youngs and Williams2015).

3.8. Anisotropy

Isotropy is often considered of importance in many turbulent flows, and turbulence is often studied under the idealization of a homogeneous, isotropic turbulent flow (Kolmogorov Reference Kolmogorov1991a,Reference Kolmogorovb). However, many flow phenomena, such as the RMI, are inherently anisotropic by virtue of their physical configuration, having mean density, pressure or velocity gradients that exist in only one direction. The degree of anisotropy in a reshocked RMI mixing layer has been studied in a number of simulations and experiments as noted in § 1. There is some disagreement in the overall trends of anisotropy versus time in these studies. Simulations by Thornber et al. (Reference Thornber, Drikakis, Youngs and Williams2011) found that the mixing layer remains anisotropic at late times after reshock, with a different asymptotic degree of anisotropy for broadband and narrowband initial conditions. Groom & Thornber (Reference Groom and Thornber2018) observed a trend towards isotropy in their simulations, but found the flow nonetheless remains anisotropic for a long time following reshock. Sewell et al. (Reference Sewell, Ferguson, Krivets and Jacobs2021) found the mixing layer to be initially strongly anisotropic following reshock and decreasing anisotropy over time, though the experiment did not extend late enough following reshock to observe steady-state behaviour. Other studies, such as those of Balakumar et al. (Reference Balakumar, Orlicz, Ristorcelli, Balasubramanian, Prestridge and Tomkins2012), Balasubramanian et al. (Reference Balasubramanian, Orlicz, Prestridge and Balakumar2012) and Orlicz et al. (Reference Orlicz, Balasubramanian, Vorobieff and Prestridge2015) found a late-time trend towards isotropy for the RMI mixing layer. It is therefore of interest in the present work to examine the temporal trends of anisotropy and how these trends change with shock-to-reshock time.

The anisotropy of the RMI may be quantified in more than one way. One common measure of anisotropy is the anisotropy ratio, which is defined as the ratio of the sum of the vertical and horizontal components of TKE. This may be defined in three dimensions in cases where all three components of velocity are available (Cook, Cabot & Miller Reference Cook, Cabot and Miller2004); however, the present experiments resolve only two components of velocity, and so the two-dimensional definition of Thornber et al. (Reference Thornber, Drikakis, Youngs and Williams2011) will be used instead,

(3.9)\begin{equation} A_R = \frac{\sum k_v }{ \sum k_u }, \end{equation}

where the summations are over all values within the mixing layer. An isotropic state is represented by $A_R=1$, with $A_R>1$ indicating more energy in the vertical direction than the horizontal and $A_R<1$ indicating more energy in the horizontal direction than the vertical.

In order to ascertain the effects of the shock-to-reshock time and the order of arrival of the two shock waves on anisotropy, the present experimental results are divided in to the same four groups based on shock-to-reshock time as presented in figure 14. The ensemble average is calculated using a boxcar moving average to translate all experimental data points on to a common set of coordinates. The moving average window has a half-width of $1/3000$ s, corresponding to a total window size equivalent to the slowest imaging frame rate across all of the present experiments. In addition, this process only averages the data that falls within a specified window of time about a given point, and no interpolation of the data is performed. The resulting ensemble-averaged anisotropy ratio of the four groups is shown in figure 15. Also shown for comparison are horizontal bands representing asymptotic values of $A_R = 1.8 \pm 0.2$ and $A_R = 1.7 \pm 0.1$ found from the simulations by Thornber et al. (Reference Thornber, Drikakis, Youngs and Williams2011) for broadband and narrowband initial conditions, respectively.

Figure 15. Anisotropy ratio versus time for four groupings of experiments corresponding to large negative, small negative, small positive and large positive shock-to-reshock times. Shaded areas indicate the range of anisotropy ratio found from simulation (Thornber et al. Reference Thornber, Drikakis, Youngs and Williams2011).

Several trends may be observed in the data in figure 15. The mixing layer for all four groups begins initially very anisotropic with vertical TKE significantly exceeding horizontal TKE. The anisotropy for all four groups then trends towards, although does not reach, an isotropic state at early times following reshock. The experimental groups with the longest shock-to-reshock times demonstrate a more rapid trend towards isotropy than those with a shorter shock-to-reshock time. No significant difference is observed between the heavy shock first and light shock first cases with similar shock-to-reshock times. All four groups reach a minimum anisotropy ratio of approximately 1.1. The four groups all reach a nearly equal degree of anisotropy at around 3-4 ms after reshock, and demonstrate increasing anisotropy for the remainder of the experiment. The experiments approach a quasi-steady value of $1.6 \lessapprox A_R \lessapprox 1.8$ at the latest times, although the length of time that this quasi-steady value is observable is relatively short. The experiments with the longest positive shock-to-reshock time approach a quasi-steady value of $A_R \approx 1.8$, and the experiments with the shortest shock-to-reshock time approach a quasi-steady value of $A_R \approx 1.6$ for both the heavy and light shock first cases. The heavy shock first experiments with the longest shock-to-reshock times do not appear to approach a quasi-steady value by the end of these experiments.

The data presented in figure 15 suggest that, while experiments with a longer shock-to-reshock time approach an isotropic state more rapidly than those with a shorter shock-to-reshock time, there does not appear to be an influence of the order of arrival of the two shock waves. This result suggests that the degree of anisotropy of the mixing layer is primarily influenced by the length of the shock-to-reshock time, and the direction of traversal of reshock does not appear to have a significant influence on the post-reshock degree of anisotropy. The tendency for experiments with a long shock-to-reshock time to more rapidly approach an isotropic state after reshock compared with those with a short shock-to-reshock time is consistent with the simulations of Ristorcelli et al. (Reference Ristorcelli, Gowardhan and Grinstein2013), who found that an increase in mixing layer width prior to reshock, which is equivalent to an increased shock-to-reshock time here, would result in a decrease in the degree of anisotropy in a reshocked mixing layer, although they did not examine the heavy shock first case. Additionally, at later times ($t \gtrapprox 3.0$ ms) in the present experiments there appears to be only limited influence of the shock-to-reshock time and the order of arrival of the two shock waves, with all four groups demonstrating a similar degree of anisotropy with the primary difference between the four groups being the quasi-steady value obtained at the end of the experiments.

There is a notable qualitative departure in behaviour of anisotropy ratio versus time between the data presented in figure 15 and data presented by Thornber et al. (Reference Thornber, Drikakis, Youngs and Williams2011) as well as summarized by Zhou (Reference Zhou2017b) (and others presented within that work). The quasi-steady values of $A_R \approx 1.6-1.8$ obtained from the present experiments at the latest times generally agree with the results found in simulations by Thornber. However, those simulations show qualitatively different temporal behaviour of anisotropy, with the value of the anisotropy ratio approaching its final value monotonically without the initial dip and subsequent increase observed in the present experiments. There has been other work that shows a qualitatively similar behaviour of anisotropy versus time as is observed here, with anisotropy ratio approaching a minimum value at early times, followed by an increase at later times (Ristorcelli et al. Reference Ristorcelli, Gowardhan and Grinstein2013; Oggian et al. Reference Oggian, Drikakis, Youngs and Williams2015; Soulard et al. Reference Soulard, Guillois, Griffond, Sabelnikov and Simoëns2018). The reasons for this behaviour may be related to changes in the dominance of small and large scales of the flow over time. Kolmogorov (Reference Kolmogorov1991b) suggests that a turbulent flow should become isotropic at small scales. Mohaghar et al. (Reference Mohaghar, Carter, Musci, Reilly, McFarland and Ranjan2017) finds that, at late time, the small-scale components of the flow do indeed trend towards isotropy. Soulard et al. (Reference Soulard, Guillois, Griffond, Sabelnikov and Simoëns2018) suggests further that an RMI flow with a decreasing amount of energy in the large scales should become increasingly isotropic, and that a trend towards isotropy in the RMI appears to be driven by the emergence of small-scale flow structures. It may be argued from these suggestions that the behaviour observed in the present experiments is the result of a change in the energy content of the smallest relative to the largest scales in the flow, and the difference in the isotropy of those scales, as the RMI develops in reshock. To further develop this idea, it is useful to consider the spectrum of anisotropy to examine anisotropy as a function of scale size.

The spectrum of anisotropy is calculated in a similar manner to the spectrum of TKE discussed previously in § 3.7. Equation (3.6a,b) is again used to calculate the spectrum of TKE for each component of velocity. In this case, however, each component is treated separately instead of being combined to form a single TKE spectrum. The spectra $\overline {\widehat {k_u}}$ and $\overline {\widehat {k_v}}$, corresponding to the spectrum of TKE in the horizontal and vertical directions from (3.6a,b), are calculated and the average spectrum across the mixing layer is found for each component. The ratio of these two average spectra is then calculated on a per-wavenumber basis to obtain a value of the anisotropy ratio as a function of wavenumber,

(3.10)\begin{equation} \widehat{A_R} = \frac{\overline{\widehat{k_v}}}{\overline{\widehat{k_u}}}, \end{equation}

where $\hat {A}_R$ is the spectrum of the anisotropy ratio. This can be viewed as the equivalent of (3.9) in a spectral sense. The anisotropy ratio spectra are then ensemble averaged in the same manner as the TKE spectra. Plots of this ensemble-averaged anisotropy ratio spectrum versus wavenumber for the same four groups of experiments shown in figure 14 is presented in figure 16. Again, each curve in the plot has a grey value corresponding to the time of the curve relative to reshock, with black representing the latest times in the experiment.

Figure 16. Anisotropy versus wavenumber for four groups of experiments: (a) large negative, (b) small negative, (c) large positive and (d) small positive shock-to-reshock times. The red dashed line in each plot is a reference line indicating isotropy.

It is observed in these plots that, in agreement with the results of Mohaghar et al. (Reference Mohaghar, Carter, Musci, Reilly, McFarland and Ranjan2017) as well as Soulard et al. (Reference Soulard, Guillois, Griffond, Sabelnikov and Simoëns2018), the lowest wavenumber contributions to this flow appear to be anisotropic, while the higher wavenumber contributions trend towards isotropy. Experiments with a long shock-to-reshock time (plots a,c) appear to show a time dependence of the anisotropy at low wavenumbers, with the longer wavelength components initially isotropic, but becoming increasingly anisotropic with time. There does not appear to be any similar temporal trend at high wavenumber, however. Very little time dependence is observed over all wavelengths of the spectrum in the short shock-to-reshock time groups (plots b,d). Additionally, only the length of the shock-to-reshock time, and not the order of shock wave arrival, appears to influence these observed behaviours.

Finally, it is worth considering whether the observed scale dependence of the anisotropy ratio as shown in figure 16, together with the shift in scale sizes as a function of time shown in figures 13 and 14, can be utilized to explain the change in anisotropy ratio as a function of time shown in figure 15. To do this, the centroid wavenumber of the ensemble-averaged TKE spectra are calculated at each time in the ensemble. The value of the anisotropy ratio at the centroid wavenumber is found from the spectrum of anisotropy at the same time in the ensemble. This quantity will be termed the spectral centroid anisotropy ratio and written as $A_{R,c}$ to differentiate it from the anisotropy ratio defined in (3.9). The plot of the spectral centroid anisotropy ratio versus time calculated using this approach is shown in figure 17. It is notable that, although the values of the spectral centroid anisotropy ratio found using this method are slightly less than those found in figure 15, the temporal trends are similar. This indicates that the trends in the anisotropy ratio versus time observed in figure 15 do appear to be at least partially explained by the change in the dominance of different scales of the flow, as well as the anisotropy of those scales, over time.

Figure 17. Spectral centroid anisotropy ratio versus time for four groups of experiments. This is calculated by finding the centroid of the ensemble-averaged TKE spectrum in figure 14 at each time instant, and then finding the corresponding value of anisotropy ratio for that time instant and centroid location from the ensemble-averaged anisotropy spectrum in figure 16.