Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-28T22:31:41.243Z Has data issue: false hasContentIssue false
  • English
  • Français

The influence of inertia on the rheology of a periodic suspension of neutrally buoyant rigid ellipsoids

Published online by Cambridge University Press:  24 September 2015

Mohsen Daghooghi  and
Iman Borazjani
Mohsen Daghooghi
Affiliation:
Department of Mechanical and Aerospace Engineering, State University of New York at Buffalo, Buffalo, NY 14260, USA
Iman Borazjani*
Affiliation:
Department of Mechanical and Aerospace Engineering, State University of New York at Buffalo, Buffalo, NY 14260, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We investigate the rheological properties of a suspension of neutrally buoyant rigid ellipsoids by fluid–structure interaction simulations of a particle in a periodic domain under simple shear using the curvilinear immersed-boundary (CURVIB) method along with a quaternion–angular velocity technique to calculate the dynamics of the particle’s motion. We calculate all the different terms of particle stress for the first time for non-spherical particles, i.e. in addition to the stresslet, we calculate the acceleration and Reynolds stress, which are typically ignored in previous similar works. Furthermore, we derive analytical expressions for all these terms to verify the numerical results and deduce the effect of inertia by comparing our numerical results with the analytical solution. The effect of particle Reynolds number (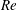$\mathit{Re}$), volume fraction (${\it\phi}$), and the shape of particles has been studied on all mechanisms of stress generation, the intrinsic viscosity, and normal stress differences of the suspension for the range 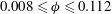$0.008\leqslant {\it\phi}\leqslant 0.112$ and 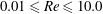$0.01\leqslant \mathit{Re}\leqslant 10.0$. We found that inertia increases the shear and the second normal difference of the stresslet (dominant term of the particle stress), and decreases the first normal difference that is generated due to the strain field. The contribution of acceleration stress to the total stress is found to be important in the second normal stress difference, with a cycle-average comparable to the stresslet component. We also discovered that the contribution of Reynolds stress in the first normal stress difference becomes important even when inertia is as low as 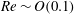$\mathit{Re}\sim O(0.1)$, and its value can be even greater than the stresslet when inertia increases, i.e. Reynolds stresses cannot be ignored for non-spherical particles. For concentrations in the range from dilute to semi-dilute, the effect of inertia on the intrinsic viscosity of a suspension is found to be comparable to the volume fraction. Furthermore, our calculations show that for a dilute concentration and the low-inertia regime (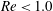$\mathit{Re}<1.0$), the intrinsic viscosity of a suspension consisting of ellipsoids with an aspect ratio of five can be 20 % higher than its Stokesian analytical value.

JFM classification

Complex Fluids: Colloids Complex Fluids: Complex Fluids Complex Fluids: Suspensions
Type
Papers
Information
Journal of Fluid Mechanics , Volume 781 , 25 October 2015 , pp. 506 - 549
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aidun, C. K. & Clausen, J. R. 2010 Lattice–Boltzmann method for complex flows. Annu. Rev. Fluid Mech. 42, 439–472.Google Scholar
Aidun, C. K., Lu, Y. & Ding, E.-J. 1998 Direct analysis of particulate suspensions with inertia using the discrete Boltzmann equation. J. Fluid Mech. 373, 287–311.CrossRefGoogle Scholar
Ancey, C., Coussot, P. & Evesque, P. 1999 A theoretical framework for granular suspensions in a steady simple shear flow. J. Rheol. 43 (6), 1673–1699.CrossRefGoogle Scholar
Ausias, G., Fan, X. J. & Tanner, R. I. 2006 Direct simulation for concentrated fibre suspensions in transient and steady state shear flows. J. Non-Newtonian Fluid Mech. 135 (1), 46–57.Google Scholar
Balay, S., Abhyankar, S., Adams, M. F., Brown, J., Brune, P., Buschelman, K., Dalcin, L., Eijkhout, V., Gropp, W. D., Kaushik, D., Knepley, M. G., McInnes, L. C., Rupp, K., Smith, B. F., Zampini, S. & Zhang, H.2015 PETSc Web page http://www.mcs.anl.gov/petsc.Google Scholar
Baraff, D.1997 An introduction to physically based modeling: rigid body simulation I – unconstrained rigid body dynamics. SIGGRAPH Course Notes. http://excelsior.cs.ucsb.edu/courses/cs290n_cg_modeling/notes/cmu_notes/rigid1.pdf.Google Scholar
Batchelor, G. K. 1970 The stress system in a suspension of force-free particles. J. Fluid Mech. 41 (03), 545–570.Google Scholar
Batchelor, G. K. & Green, J. T. 1972 The determination of the bulk stress in a suspension of spherical particles to order c2. J. Fluid Mech. 56 (03), 401–427.Google Scholar
Berlyand, L., Borcea, L. & Panchenko, A. 2005 Network approximation for effective viscosity of concentrated suspensions with complex geometry. SIAM J. Math. Anal. 36 (5), 1580–1628.Google Scholar
Blakeney, W. R. 1966 The viscosity of suspensions of straight, rigid rods. J. Colloid Interface Sci. 22 (4), 324–330.CrossRefGoogle Scholar
Borazjani, I. 2013a Fluid-structure interaction, immersed boundary-finite element method simulations of bio-prosthetic heart valves. Comput. Meth. Appl. Mech. Engng 257, 103–116.CrossRefGoogle Scholar
Borazjani, I. 2013b The functional role of caudal and anal/dorsal fins during the c-start of a bluegill sunfish. J. Expl Biol. 216, 1658–1669.Google ScholarPubMed
Borazjani, I. & Daghooghi, M. 2013 The fish tail motion forms an attached leading edge vortex. Proc. R. Soc. Lond. B 280, 20122071.Google ScholarPubMed
Borazjani, I., Ge, L., Le, T. & Sotiropoulos, F. 2013 A parallel overset-curvilinear-immersed boundary framework for simulating complex 3d incompressible flows. Comput. Fluids 77, 76–96.CrossRefGoogle ScholarPubMed
Borazjani, I., Ge, L. & Sotiropoulos, F. 2008 Curvilinear immersed boundary method for simulating fluid structure interaction with complex 3d rigid bodies. J. Comput. Phys. 227 (16), 7587–7620.Google Scholar
Borazjani, I., Ge, L. & Sotiropoulos, F. 2010a High-resolution fluid–structure interaction simulations of flow through a bi-leaflet mechanical heart valve in an anatomic aorta. Ann. Biomed. Engng 38, 326–344.CrossRefGoogle Scholar
Borazjani, I. & Sotiropoulos, F. 2009 Vortex-induced vibrations of two cylinders in tandem arrangement in the proximity – wake interference region. J. Fluid Mech. 621, 321–364.Google Scholar
Borazjani, I. & Sotiropoulos, F. 2010a The effect of implantation orientation of a bi-leaflet mechanical heart valve on kinematics and hemodynamics in an anatomic aorta. Trans. ASME. J. Biomech. Engng 132 (11), 111005.CrossRefGoogle Scholar
Borazjani, I. & Sotiropoulos, F. 2010b On the role of form and kinematics on the hydrodynamics of self-propelled body/caudal fin swimming. J. Expl Biol. 213 (1), 89–107.Google Scholar
Borazjani, I., Sotiropoulos, F., Malkiel, E. & Katz, J. 2010c On the role of copepod antenna in the production of hydrodynamic force during hopping. J. Expl Biol. 213, 3019–3035.Google Scholar
Boyer, F., Guazzelli, É. & Pouliquen, O. 2011 Unifying suspension and granular rheology. Phys. Rev. Lett. 107 (18), 188301.CrossRefGoogle ScholarPubMed
Brenner, H. 1974 Rheology of a dilute suspension of axisymmetric Brownian particles. Intl J. Multiphase Flow 1 (2), 195–341.Google Scholar
Burgers, J. M. 1995 On the Motion of Small Particles of Elongated Form. Suspended in a Viscous Liquid. Springer.Google Scholar
Cheng, X., McCoy, J. H., Israelachvili, J. N. & Cohen, I. 2011 Imaging the microscopic structure of shear thinning and thickening colloidal suspensions. Science 333 (6047), 1276–1279.Google Scholar
Claeys, I. L. & Brady, J. F. 1993 Suspensions of prolate spheroids in stokes flow. Part 2. Statistically homogeneous dispersions. J. Fluid Mech. 251, 443–477.Google Scholar
Clausen, J. R. & Aidun, C. K. 2010 Capsule dynamics and rheology in shear flow: particle pressure and normal stress. Phys. Fluids 22 (12), 123302.CrossRefGoogle Scholar
Davis, R. H., Serayssol, J.-M. & Hinch, E. J. 1986 The elastohydrodynamic collision of two spheres. J. Fluid Mech. 163, 479–497.CrossRefGoogle Scholar
Dealy, J. M. & Wang, J. 2013 Melt Rheology and its Applications in the Plastics Industry. Springer.Google Scholar
Doi, M. & Edwards, S. F. 1978 Dynamics of rod-like macromolecules in concentrated solution. Part 1. J. Chem. Soc. Faraday Trans. 2 74, 560–570.Google Scholar
Doostmohammadi, A., Stocker, R. & Ardekani, A. M. 2012 Low-Reynolds-number swimming at pycnoclines. Proc. Natl Acad. Sci. USA 109 (10), 3856–3861.Google Scholar
Eilers, H. 1949 The colloidal structure of asphalt. J. Phys. Chem. 53 (8), 1195–1211.CrossRefGoogle Scholar
Einstein, A. 1906 Eine neue bestimmung der moleküldimensionen. Ann. Phys. 324 (2), 289–306.Google Scholar
Einstein, A. 1956 Investigations on the Theory of the Brownian Movement. Courier Dover.Google Scholar
Fall, A., Lemaître, A., Bertrand, F., Bonn, D. & Ovarlez, G. 2010 Shear thickening and migration in granular suspensions. Phys. Rev. Lett. 105 (26), 268303.Google Scholar
Forgacs, O. L. & Mason, S. G. 1959 Particle motions in sheared suspensions X: orbits of flexible threadlike particles. J. Colloid Sci. 14 (5), 473–491.Google Scholar
Ge, L. & Sotiropoulos, F. 2007 A numerical method for solving the 3D unsteady incompressible Navier–Stokes equations in curvilinear domains with complex immersed boundaries. J. Comput. Phys. 225 (2), 1782–1809.CrossRefGoogle ScholarPubMed
Ghanavati, M., Shojaei, M.-J. & Ahmad, R. S. A. 2013 Effects of asphaltene content and temperature on viscosity of iranian heavy crude oil: experimental and modeling study. Energy Fuels 27 (12), 7217–7232.Google Scholar
Gilmanov, A. & Sotiropoulos, F. 2005 A hybrid cartesian/immersed boundary method for simulating flows with 3D, geometrically complex, moving bodies. J. Comput. Phys. 207 (2), 457–492.CrossRefGoogle Scholar
Gunes, D. Z., Scirocco, R., Mewis, J. & Vermant, J. 2008 Flow-induced orientation of non-spherical particles: effect of aspect ratio and medium rheology. J. Non-Newtonian Fluid Mech. 155 (1), 39–50.Google Scholar
Guth, E. & Gold, O. 1938 On the hydrodynamical theory of the viscosity of suspensions. Phys. Rev. 53 (322), 2–15.Google Scholar
Haber, S. & Brenner, H. 1984 Rheological properties of dilute suspensions of centrally symmetric Brownian particles at small shear rates. J. Colloid Interface Sci. 97 (2), 496–514.CrossRefGoogle Scholar
Haddadi, H. & Morris, J. F. 2014 Microstructure and rheology of finite inertia neutrally buoyant suspensions. J. Fluid Mech. 749, 431–459.CrossRefGoogle Scholar
Hinch, E. J. & Leal, L. G. 1972 The effect of Brownian motion on the rheological properties of a suspension of non-spherical particles. J. Fluid Mech. 52 (04), 683–712.Google Scholar
Hinch, E. J. & Leal, L. G. 1976 Constitutive equations in suspension mechanics. Part 2. Approximate forms for a suspension of rigid particles affected by Brownian rotations. J. Fluid Mech. 76 (01), 187–208.Google Scholar
a-Holek, S. I. & Mendoza, C. I. 2010 The rheology of concentrated suspensions of arbitrarily-shaped particles. J. Colloid Interface Sci. 346 (1), 118–126.Google Scholar
Huggins, M. L. 1942 The viscosity of dilute solutions of long-chain molecules IV: dependence on concentration. J. Am. Chem. Soc. 64 (11), 2716–2718.Google Scholar
Ishikawa, T. & Pedley, T. J. 2007 The rheology of a semi-dilute suspension of swimming model micro-organisms. J. Fluid Mech. 588, 399–435.Google Scholar
Jeffery, G. B. 1922 The motion of ellipsoidal particles immersed in a viscous fluid. Proc. R. Soc. Lond. A 102 (715), 161–179.Google Scholar
Jeffrey, D. J. & Acrivos, A. 1976 The rheological properties of suspensions of rigid particles. AIChE J. 22 (3), 417–432.Google Scholar
Joseph, G. G., Zenit, R., Hunt, M. L. & Rosenwinkel, A. M. 2001 Particle–wall collisions in a viscous fluid. J. Fluid Mech. 433, 329–346.Google Scholar
Joung, C. G., Phan-Thien, N. & Fan, X. J. 2002 Viscosity of curved fibers in suspension. J. Non-Newtonian Fluid Mech. 102 (1), 1–17.Google Scholar
Kim, S. & Karrila, S. J. 2013 Microhydrodynamics: Principles and Selected Applications. Courier Dover.Google Scholar
Kossack, C. A. & Acrivos, A. 1974 Steady simple shear flow past a circular cylinder at moderate Reynolds numbers: a numerical solution. J. Fluid Mech. 66 (02), 353–376.Google Scholar
Krieger, I. M. & Dougherty, T. J. 1959 A mechanism for non-Newtonian flow in suspensions of rigid spheres. Trans. Soc. Rheol. 3 (1), 137–152.Google Scholar
Kuipers, J. B. 1999 Quaternions and Rotation Sequences. vol. 66. Princeton University Press.Google Scholar
Kulkarni, P. M. & Morris, J. F. 2008 Suspension properties at finite Reynolds number from simulated shear flow. Phys. Fluids 20 (4), 040602.CrossRefGoogle Scholar
Kumar, A. & Higdon, J. J. L. 2011a Particle mesh Ewald Stokesian dynamics simulations for suspensions of non-spherical particles. J. Fluid Mech. 675, 297–335.Google Scholar
Kumar, A. & Higdon, J. J. L. 2011b Dynamics of the orientation behavior and its connection with rheology in sheared non-Brownian suspensions of anisotropic dicolloidal particles. J. Rheol. 55 (3), 581–626.Google Scholar
Leal, L. G. & Hinch, E. J. 1971 The effect of weak Brownian rotations on particles in shear flow. J. Fluid Mech. 46 (04), 685–703.Google Scholar
Lin, C.-J., Peery, J. H. & Schowalter, W. R. 1970 Simple shear flow round a rigid sphere: inertial effects and suspension rheology. J. Fluid Mech. 44 (01), 1–17.Google Scholar
Lindström, S. B. & Uesaka, T. 2009 A numerical investigation of the rheology of sheared fiber suspensions. Phys. Fluids 21 (8), 083301.Google Scholar
Lipscomb, G. G., Denn, M. M., Hur, D. U. & Boger, D. V. 1988 The flow of fiber suspensions in complex geometries. J. Non-Newtonian Fluid Mech. 26 (3), 297–325.Google Scholar
MacMeccan, R. M., Clausen, J. R., Neitzel, G. P. & Aidun, C. K. 2009 Simulating deformable particle suspensions using a coupled lattice–Boltzmann and finite-element method. J. Fluid Mech. 618, 13–39.Google Scholar
Maron, S. H. & Pierce, P. E. 1956 Application of Ree–Eyring generalized flow theory to suspensions of spherical particles. J. Colloid Sci. 11 (1), 80–95.Google Scholar
Meng, Q. J. & Higdon, J. J. L. 2008 Large scale dynamic simulation of plate-like particle suspensions. Part i: Non-Brownian simulation. J. Rheol. 52 (1), 1–36.Google Scholar
Metzger, B., Guazzelli, É. & Butler, J. E. 2005 Large-scale streamers in the sedimentation of a dilute fiber suspension. Phys. Rev. Lett. 95 (16), 164506.Google Scholar
Mikulencak, D. R. & Morris, J. F. 2004 Stationary shear flow around fixed and free bodies at finite Reynolds number. J. Fluid Mech. 520, 215–242.Google Scholar
Mooney, M. 1951 The viscosity of a concentrated suspension of spherical particles. J. Colloid Sci. 6 (2), 162–170.Google Scholar
Mueller, S., Llewellin, E. W. & Mader, H. M. 2010 The rheology of suspensions of solid particles. Proc. R. Soc. Lond. A 466 (2116), 1201–1228.Google Scholar
Nguyen, N.-Q. & Ladd, A. J. C. 2002 Lubrication corrections for lattice–Boltzmann simulations of particle suspensions. Phys. Rev. E 66 (4), 046708.Google Scholar
Nielloud, F. 2000 Pharmaceutical Emulsions and Suspensions: Revised and Expanded. CRC Press.Google Scholar
Omori, T., Ishikawa, T., Imai, Y. & Yamaguchi, T. 2014 Hydrodynamic interaction between two red blood cells in simple shear flow: its impact on the rheology of a semi-dilute suspension. Comput. Mech. 54 (4), 933–941.Google Scholar
Pabst, W., Gregorová, E. & Berthold, C. 2006 Particle shape and suspension rheology of short-fiber systems. J. Eur. Ceram. Soc. 26 (1), 149–160.CrossRefGoogle Scholar
Pal, R. & Rhodes, E. 1989 Viscosity/concentration relationships for emulsions. J. Rheol. 33 (7), 1021–1045.Google Scholar
Petrie, C. J. S. 1999 The rheology of fibre suspensions. J. Non-Newtonian Fluid Mech. 87 (2), 369–402.Google Scholar
Poe, G. G. & Acrivos, A. 1975 Closed-streamline flows past rotating single cylinders and spheres: inertia effects. J. Fluid Mech. 72 (04), 605–623.Google Scholar
Rallison, J. M. 1978 The effects of Brownian rotations in a dilute suspension of rigid particles of arbitrary shape. J. Fluid Mech. 84 (02), 237–263.CrossRefGoogle Scholar
Robertson, C. R. & Acrivos, A. 1970 Low Reynolds number shear flow past a rotating circular cylinder. Part 1. Momentum transfer. J. Fluid Mech. 40 (04), 685–703.Google Scholar
Ross, R. F. & Klingenberg, D. J. 1997 Dynamic simulation of flexible fibers composed of linked rigid bodies. J. Chem. Phys. 106 (7), 2949–2960.Google Scholar
Ryskin, G., Ryskin, G. & Rallison, J. M. 1980 The extensional viscosity of a dilute suspension of spherical particles at intermediate microscale Reynolds numbers. J. Fluid Mech. 99 (03), 513–529.Google Scholar
Salac, D. & Miksis, M. J. 2012 Reynolds number effects on lipid vesicles. J. Fluid Mech. 711, 122–146.Google Scholar
Shoemake, K. 1985 Animating rotation with quaternion curves. In ACM SIGGRAPH Computer Graphics, vol. 19, pp. 245–254. ACM.Google Scholar
Singh, A. & Nott, P. R. 2003 Experimental measurements of the normal stresses in sheared Stokesian suspensions. J. Fluid Mech. 490, 293–320.Google Scholar
Stickel, J. J. & Powell, R. L. 2005 Fluid mechanics and rheology of dense suspensions. Annu. Rev. Fluid Mech. 37, 129–149.Google Scholar
Stover, C. A., Koch, D. L. & Cohen, C. 1992 Observations of fibre orientation in simple shear flow of semi-dilute suspensions. J. Fluid Mech. 238, 277–296.Google Scholar
Subramanian, G., Koch, D. L., Zhang, J. & Yang, C. 2011 The influence of the inertially dominated outer region on the rheology of a dilute dispersion of low-Reynolds-number drops or rigid particles. J. Fluid Mech. 674, 307–358.Google Scholar
Tabilo-Munizaga, G. & Barbosa-Cánovas, G. V. 2005 Rheology for the food industry. J. Food Engng 67 (1), 147–156.CrossRefGoogle Scholar
Trulsson, M., Andreotti, B. & Claudin, P. 2012 Transition from the viscous to inertial regime in dense suspensions. Phys. Rev. Lett. 109 (11), 118305.Google Scholar
Verberg, R. & Koch, D. L. 2006 Rheology of particle suspensions with low to moderate fluid inertia at finite particle inertia. Phys. Fluids 18 (8), 083303.Google Scholar
Wilson, H. J. & Davis, R. H. 2000 The viscosity of a dilute suspension of rough spheres. J. Fluid Mech. 421, 339–367.Google Scholar
Wu, J. & Aidun, C. K. 2010 A method for direct simulation of flexible fiber suspensions using lattice Boltzmann equation with external boundary force. Intl J. Multiphase Flow 36 (3), 202–209.Google Scholar
Yeo, K. & Maxey, M. R. 2013 Dynamics and rheology of concentrated, finite-Reynolds-number suspensions in a homogeneous shear flow. Phys. Fluids 25 (5), 053303.Google Scholar
Zarraga, I. E., Hill, D. A. & Leighton, D. T. Jr 2000 The characterization of the total stress of concentrated suspensions of noncolloidal spheres in Newtonian fluids. J. Rheol. 44 (2), 185–220.Google Scholar
Zettner, C. M. & Yoda, M. 2001 Moderate-aspect-ratio elliptical cylinders in simple shear with inertia. J. Fluid Mech. 442, 241–266.Google Scholar