Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-26T23:35:32.680Z Has data issue: false hasContentIssue false
  • English
  • Français

Influence of active control on STG-based generation of streamwise vortices in near-wall turbulence

Published online by Cambridge University Press:  29 August 2012

B.-Q. Deng  and
C.-X. Xu
B.-Q. Deng
Affiliation:
Department of Engineering Mechanics, Tsinghua University, Beijing 100084, PR China
C.-X. Xu*
Affiliation:
Department of Engineering Mechanics, Tsinghua University, Beijing 100084, PR China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Near-wall streamwise vortices are closely related to the generation of high skin friction in wall-bounded turbulent flows. A common feature of controlled, friction-reduced turbulent flows is weakened near-wall streamwise vortices. In the present study, the streak transient growth (STG) mechanism for generating near-wall streamwise vortices by Schoppa & Hussain (J. Fluid Mech., vol. 453, 2002, pp. 57–108) is employed, and the opposition control proposed by Choi, Moin & Kim (J. Fluid Mech., vol. 262, 1994, pp. 75–110) is imposed during the transient growth process of perturbations to determine how active control affects the generation of quasi-streamwise vortices. 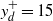In the transient growth stage, when the detection plane is located near the wall (), the control can suppress the production of streamwise vorticity by weakening the near-wall vertical velocity; when the detection plane moves away from the wall (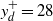), the control has the opposite effect. In the vortex generation stage, the control cannot change the dominance of the stretching effect. Controls imposed at different stages reveal the importance of the STG stage in vortex generation. Strengthened out-of-phase control and lessened in-phase control are proposed as an extension of the original opposition-control scheme. Application in a fully developed turbulent channel flow shows that strengthened 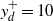control can yield an even higher drag reduction rate than the original control. Moreover, lessened 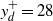control can also achieve drag reduction and turbulence suppression.

JFM classification

Flow Control: Drag reduction Turbulent Flows: Turbulence control Turbulent Flows: Turbulent boundary layers
Type
Papers
Information
Journal of Fluid Mechanics , Volume 710 , 10 November 2012 , pp. 234 - 259
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. del Álamo, J. C. & Jiménez, J. 2006 Linear energy amplification in turbulent channels. J. Fluid Mech. 559, 205–213.CrossRefGoogle Scholar
2. Bernard, P. S., Thomas, J. M. & Handler, R. A. 1993 Vortex dynamics and the production of Reynolds stress. J. Fluid Mech. 253, 385–419.CrossRefGoogle Scholar
3. Brooke, J. W. & Hanratty, T. J. 1993 Origin of turbulence-producing eddies in a channel flow. Phys. Fluids A 5, 1011–1022.CrossRefGoogle Scholar
4. Choi, H., Moin, P. & Kim, J. 1994 Active turbulence control for drag reduction in wall-bounded flows. J. Fluid Mech. 262, 75–110.CrossRefGoogle Scholar
5. Chung, Y. M. & Talha, T. 2011 Effectiveness of active flow control for turbulent skin friction drag reduction. Phys. Fluids 23, 025102.CrossRefGoogle Scholar
6. Collis, S. S., Joslin, R. D., Seifert, A. & Theofilis, V. 2004 Issues in active flow control: theory, control, simulation, and experiment. Prog. Aerosp. Sci. 40, 237–289.CrossRefGoogle Scholar
7. Cui, G., Zhou, H., Zhang, Z. & Shao, L. 2004 A new dynamic subgrid eddy viscosity model with application to turbulent channel flow. Phys. Fluids 16, 2835–2842.CrossRefGoogle Scholar
8. Endo, T., Kasagi, N. & Suzuki, Y. 2000 Feedback control of wall turbulence with wall deformation. Intl J. Heat Fluid Flow 21, 568–575.CrossRefGoogle Scholar
9. Hamilton, J. M., Kim, J. & Waleffe, F. 1995 Regeneration mechanisms of near-wall turbulence structures. J. Fluid Mech. 287, 317–348.CrossRefGoogle Scholar
10. Hammond, E. P., Bewley, T. R. & Moin, P. 1998 Observed mechanisms for turbulence attenuation and enhancement in opposition-controlled wall-bounded flows. Phys. Fluids 10, 2421–2423.CrossRefGoogle Scholar
11. Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.CrossRefGoogle Scholar
12. Jiménez, J. & Moin, P. 1991 The minimal flow unit in near-wall turbulence. J. Fluid Mech. 225, 213–240.CrossRefGoogle Scholar
13. Jiménez, J. & Pinelli, A. 1999 The autonomous cycle of near-wall turbulence. J. Fluid Mech. 389, 335–359.CrossRefGoogle Scholar
14. Jiménez, J. & Simens, M. P. 2001 Low-dimensional dynamics of a turbulent wall flow. J. Fluid Mech. 435, 81–91.CrossRefGoogle Scholar
15. Jovanovic, M. R. & Bamieh, B. 2005 Componentwise energy amplification in channel flows. J. Fluid Mech. 534, 145–183.CrossRefGoogle Scholar
16. Kasagi, N., Suzuki, Y. & Fukagata, K. 2009 Microelectromechanical systems-based feedback control of turbulence for skin friction reduction. Annu. Rev. Fluid Mech. 41, 231–251.CrossRefGoogle Scholar
17. Kim, J. 2003 Control of turbulent boundary layers. Phys. Fluids 15, 1093–1104.CrossRefGoogle Scholar
18. Kim, J. 2011 Physics and control of wall turbulence for drag reduction. Phil. Trans. R. Soc. A 369, 1396–1411.CrossRefGoogle ScholarPubMed
19. Kim, J. & Bewley, T. R. 2007 A linear systems approach to flow control. Annu. Rev. Fluid Mech. 39, 383–417.CrossRefGoogle Scholar
20. Kim, J. & Moin, P. 1986 Flow structures responsible for the bursting process. Bull. Am. Phys. Soc. 31, 1716.Google Scholar
21. Kim, J., Moin, P. & Moser, R. D. 1987 Turbulence statistics in fully developed channel flow at low Reynolds number. J. Fluid Mech. 177, 133–166.CrossRefGoogle Scholar
22. Koumoutsakos, P. 1997 Active control of vortex–wall interactions. Phys. Fluids 9, 3808–3816.CrossRefGoogle Scholar
23. Kravchenko, A. G., Choi, H. & Moin, P. 1993 On the relation of near-wall streamwise vortices to wall skin friction in turbulent boundary layers. Phys. Fluids A 5, 3307–3309.CrossRefGoogle Scholar
24. Lee, C., Kim, J. & Choi, H. 1998 Suboptimal control of turbulent channel flow for drag reduction. J. Fluid Mech. 358, 245–258.CrossRefGoogle Scholar
25. Nagata, M. 1990 Three-dimensional finite-amplitude solutions in plane Couette flow: bifurcation from infinity. J. Fluid Mech. 217, 519–527.CrossRefGoogle Scholar
26. Panton, R. L. 2001 Overview of the self-sustaining mechanisms of wall turbulence. Prog. Aerosp. Sci. 37, 341–383.CrossRefGoogle Scholar
27. Park, J., Hwang, Y. & Cossu, C. 2011 On the stability of large-scale streaks in turbulent Couette and Poiseuille flows. C. R. Mécanique 339, 1–5.CrossRefGoogle Scholar
28. Pujals, G., Garcia-Villalba, M., Cossu, C. & Depardon, S. 2009 A note on optimal transient growth in turbulent channel flows. Phys. Fluids 21, 015109.CrossRefGoogle Scholar
29. Robinson, S. K. 1991 Coherent motions in the turbulent boundary layer. Annu. Rev. Fluid Mech. 23, 601–639.CrossRefGoogle Scholar
30. Schoppa, W. & Hussain, F. 2002 Coherent structure generation in near-wall turbulence. J. Fluid Mech. 453, 57–108.CrossRefGoogle Scholar
31. Smith, C. R. & Walker, J. D. A. 1995 Turbulent wall-layer vortices. In Fluid Vortices (ed. Green, S. ). pp. 235–290. Kluwer.CrossRefGoogle Scholar
32. Swearingen, J. D. & Blackwelder, R. F. 1987 The growth and breakdown of streamwise vortices in the presence of a wall. J. Fluid Mech. 182, 255–290.CrossRefGoogle Scholar
33. Waleffe, F. 1997 On a self-sustaining process in shear flow. Phys. Fluids 9, 883–900.CrossRefGoogle Scholar
34. Waleffe, F. 1998 Three-dimensional coherent states in plane shear flows. Phys. Rev. Lett. 81, 4140–4143.CrossRefGoogle Scholar
35. Waleffe, F. 2001 Exact coherent structures in channel flow. J. Fluid Mech. 435, 93–102.CrossRefGoogle Scholar
36. Xu, C., Zhang, Z., Toonder, J. M. J. D. & Nieuwstadt, F. T. M. 1996 Origin of high kurtosis levels in the viscous sublayer: direct numerical simulation and experiment. Phys. Fluids 8, 1938–1944.CrossRefGoogle Scholar
37. Zhou, J., Adrian, R. J., Balachandar, S. & Kendall, T. M. 1999 Mechanisms for generating coherent packets of hairpin vortices in channel flow. J. Fluid Mech. 387, 353–396.CrossRefGoogle Scholar