Hostname: page-component-745bb68f8f-hvd4g Total loading time: 0 Render date: 2025-01-08T11:05:03.800Z Has data issue: false hasContentIssue false
  • English
  • Français

Inertial wave dynamics in a rotating liquid metal

Published online by Cambridge University Press:  25 July 2014

Tobias Vogt ,
Dirk Räbiger  and
Sven Eckert
Tobias Vogt*
Affiliation:
Institute of Fluid Dynamics, Helmholtz-Zentrum Dresden-Rossendorf (HZDR), PO Box 510119, 01314 Dresden, Germany
Dirk Räbiger
Affiliation:
Institute of Fluid Dynamics, Helmholtz-Zentrum Dresden-Rossendorf (HZDR), PO Box 510119, 01314 Dresden, Germany
Sven Eckert
Affiliation:
Institute of Fluid Dynamics, Helmholtz-Zentrum Dresden-Rossendorf (HZDR), PO Box 510119, 01314 Dresden, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The dynamics of free and forced inertial waves inside cylinders of different aspect ratios (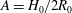$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}A=H_0/2R_0$) were investigated experimentally in this study. The liquid metal GaInSn was chosen as the fluid in order to enable a contactless stimulation of the flow by means of alternating electromagnetic fields. A rotating magnetic field generates the rotating motion of the liquid, whereas periodic modulations of the field strength and short pulses excite specific wave modes. Ultrasound Doppler velocimetry was used to record the flow structure and to identify inertial waves in the set-up. Our experiments demonstrate selective excitation of different inertial wave modes by deliberate variation of the magnetic field parameters. Furthermore, it was found that turbulent perturbations in the boundary layers of the swirling flow are able to induce an inertial wave mode that survives over a long time. Experiments at the fundamental resonance have shown that multiple harmonic wave modes appeared simultaneously. The measured inertial wave frequencies were compared to the predictions of the linear inviscid theory.

JFM classification

MHD and Electrohydrodynamics: MHD and Electrohydrodynamics Geophysical and Geological Flows: Waves in rotating fluids
Type
Papers
Information
Journal of Fluid Mechanics , Volume 753 , 25 August 2014 , pp. 472 - 498
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aldridge, K. D. & Lumb, L. I. 1987 Inertial waves identified in the Earth’s fluid outer core. Nature 325, 421–423.Google Scholar
Baruteau, C. & Rieutord, M. 2013 Inertial waves in a differentially rotating spherical shell. J. Fluid Mech. 719, 47–81.Google Scholar
Bjerknes, V. & Solberg, H. 1929 Zellulare Trägheitswellen und Turbulenz. Avhandl. Norske Vid. Akad. Mat. Nat (7), 1–16.Google Scholar
Brito, D., Nataf, H. C., Cardin, P., Aubert, J. & Masson, J. P. 2001 Ultrasonic Doppler velocimetry in liquid gallium. Exp. Fluids 31 (6), 653–663.Google Scholar
Davidson, P. A. 1992 Swirling flow in an axisymmetric cavity of arbitrary profile, driven by a rotating magnetic field. J. Fluid Mech. 245, 669–699.Google Scholar
Davidson, P. A. & Hunt, J. C. R. 1987 Swirling recirculating flow an a liquid-metal column generated by a rotating magnetic field. J. Fluid Mech. 185, 67–106.Google Scholar
Eckert, S. & Gerbeth, G. 2002 Velocity measurements in liquid sodium by means of ultrasound Doppler velocimetry. Exp. Fluids 32 (5), 542–546.Google Scholar
Eckert, S., Nikrityuk, P., Räbiger, D., Eckert, K. & Gerbeth, G. 2007 Efficient melt stirring using pulse sequences of a rotating magnetic field: part I. Flow field in a liquid metal column. Metall. Mater. Trans. B 38 (6), 977–988.Google Scholar
Fu, L. L.1980 Observations and models of inertial waves in the deep ocean. PhD thesis, Massachusetts Institute of Technology and Woods Hole Oceanographic Institution.Google Scholar
Fultz, D. 1959 A note on overstability and the elastoid-inertia oscillations of Kelvin, Solberg, and Bjerknes. J. Atmos. Sci. 16, 199–208.Google Scholar
Gailitis, A., Lielausis, O., Platacis, E., Dement’ev, S., Cifersons, A., Gerbeth, G., Gundrum, T., Stefani, F., Christen, M. & Will, G. 2001 Magnetic field saturation in the Riga dynamo experiment. Phys. Rev. Lett. 86 (14), 3024–3027.Google Scholar
Gorbachev, L. P., Nikitin, N. V. & Ustinov, A. L. 1974 Magnetohydrodynamic rotation of electrically conducting liquid in a cylindrical vessel of finite dimensions. Magn. Gidrodin. (4), 32–42.Google Scholar
Görtler, H.1957 On forced oscillations in rotating fluids. In Proceedings of the 5th Midwestern Conference on Fluid Mechanics, pp. 1–10.Google Scholar
Grants, I. & Gerbeth, G. 2001 Stability of axially symmetric flow driven by a rotating magnetic field in a cylindrical cavity. J. Fluid Mech. 431, 407–426.Google Scholar
Grants, I. & Gerbeth, G. 2002 Linear three-dimensional instability of a magnetically driven rotating flow. J. Fluid Mech. 463, 229–239.Google Scholar
Grants, I., Zhang, C., Eckert, S. & Gerbeth, G. 2008 Experimental observation of swirl accumulation in a magnetically driven flow. J. Fluid Mech. 616, 135–152.CrossRefGoogle Scholar
Greenspan, H. P. 1968 The Theory of Rotating Fluids. Cambridge University Press.Google Scholar
Griffiths, W. D. & McCartney, D. G. 1996 The effect of electromagnetic stirring during solidification on the structure of Al–Si alloys. Mater. Sci. Engng A 216, 47–60.CrossRefGoogle Scholar
Hollerbach, R. & Kerswell, R. R. 1995 Oscillatory inertial shear layer in rotating and precessing flows. J. Fluid Mech. 298, 327–339.Google Scholar
Kelley, D. H., Triana, S. A., Zimmerman, D. S. & Lathrop, D. P. 2010 Selection of inertial modes in spherical Couette flow. Phys. Rev. E 81, 026311.Google Scholar
Kelley, D. H., Triana, S. A., Zimmerman, D. S., Tilgner, A. & Lathrop, D. P. 2007 Inertial waves driven by differential rotation in a planetary geometry. Geophys. Astrophys. Fluid Dyn. 101, 469–487.Google Scholar
Kelvin, Lord 1880 Vibrations of a columnar vortex. Phil. Mag. 10 (5), 155–168.Google Scholar
Kobine, J. J. 1995 Inertial wave dynamics in a rotating and precessing cylinder. J. Fluid Mech. 303, 233–252.Google Scholar
Manasseh, R. 1992 Breakdown regimes of inertial waves in a precessing cylinder. J. Fluid Mech. 243, 261–296.CrossRefGoogle Scholar
Messio, L., Morize, C., Raubaud, M. & Moisy, F. 2008 Experimental observation using particle image velocimetry of inertial waves in a rotating fluid. Exp. Fluids 44 (1), 519–528.Google Scholar
Monchaux, R., Berhanu, M., Bourgoin, M., Moulin, M., Odier, P., Pinton, J.-F., Volk, R., Fauve, S., Mordant, N., Pétrélis, F., Chiffaudel, A., Daviaud, F., Dubrulle, B., Gasquet, C., Marié, L. & Ravelet, F. 2007 Generation of a magnetic field by dynamo action in a turbulent flow of liquid sodium. Phys. Rev. Lett. 98, 044502.Google Scholar
Nataf, H.-C., Alboussière, T., Brito, D., Cardin, P., Gagnière, N., Jault, D., Masson, J.-P. & Schmitt, D. 2006 Experimental study of super-rotation in a magnetostrophic spherical Couette flow. Geophys. Astrophys. Fluid Dyn. 100, 281–298.Google Scholar
Nikrityuk, P. A., Ungarish, M., Eckert, K. & Grundmann, R. 2005 Spin-up of a liquid metal flow driven by a rotating magnetic field in a finite cylinder: a numerical and an analytical study. Phys. Fluids 17, 067101.CrossRefGoogle Scholar
Pal, J., Cramer, A., Gundrum, T. & Gerbeth, G. 2009 MULTIMAG, a multipurpose magnetic system for physical modelling in magnetohydrodynamics. Flow Meas. Instrum. 20 (6), 241–251.Google Scholar
Räbiger, D., Eckert, S. & Gerbeth, G. 2010 Measurements of an unsteady liquid metal flow during spin-up driven by a rotating magnetic field. Exp. Fluids 48 (2), 233–244.Google Scholar
Räbiger, D., Eckert, S., Gerbeth, G., Czarske, J. & Franke, S. 2012 Flow structures arising from melt stirring by means of modulated rotating magnetic fields. Magnetohydrodynamics 48 (1), 213–220.Google Scholar
Rayleigh, Lord 1917 On the dynamics of revolving fluids. Proc. R. Soc. Lond. A 93 (648), 148–154.Google Scholar
Sauret, A., Cébron, D., Le Bars, M. & Le Dizès, S. 2005 Fluid flows in a librating cylinder. Phys. Fluids 24, 026603.Google Scholar
Schmitt, D., Alboussire, T., Brito, D., Cardin, P., Gagniere, N., Jault, D. & Nataf, H.-C. 2008 Rotating spherical Couette flow in a dipolar magnetic field: experimental study of magneto-inertial waves. J. Fluid Mech. 604, 175–197.Google Scholar
Stefani, F., Gundrum, T., Gerbeth, G., Rüdiger, G., Schultz, M., Szklarski, J. & Hollerbach, R. 2006 Experimental evidence for magnetorotational instability in a Taylor–Couette flow under the influence of a helical magnetic field. Phys. Rev. Lett. 97 (18), 184502.Google Scholar
Stieglitz, R. & Müller, U. 2001 Experimental demonstration of a homogeneous two-scale dynamo. Phys. Fluids 13, 561–564.CrossRefGoogle Scholar
Stiller, J., Fraňa, K. & Cramer, A. 2006 Transitional and weakly turbulent flow in a rotating magnetic field. Phys. Fluids 18, 074105.Google Scholar
Tacke, K. H. & Schwerdtfeger, K. 1979 Rührgeschwindigkeit in Rundstrangguss bei Rührung mit elektromagnetischen Drehfeldern. Stahl Eisen. 99 (1), 7–12.Google Scholar
Takeda, Y. 1991 Development of an ultrasound velocity profile monitor. Nucl. Engng Des. 126 (2), 277–284.Google Scholar
Thompson, R. 1978 Observation of inertial waves in the stratosphere. Q. J. R. Meteorol. Soc. 104 (441), 691–698.Google Scholar
Vogt, T., Grants, I., Räbiger, D., Eckert, S. & Gerbeth, G. 2012 On the formation of Taylor–Görtler vortices in RMF-driven spin-up flows. Exp. Fluids 52 (1), 1–10.Google Scholar
Vogt, T., Grants, I., Eckert, S. & Gerbeth, G. 2013 Spin-up of a magnetically driven tornado-like vortex. J. Fluid Mech. 736, 641–662.Google Scholar
Zhang, C., Shatrov, V., Priede, J., Eckert, S. & Gerbeth, G. 2011 Intermittent behavior caused by surface oxidation in a liquid metal flow driven by a rotating magnetic field. Metall. Mater. Trans. B 42 (6), 1188–1200.Google Scholar