Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-27T08:27:59.290Z Has data issue: false hasContentIssue false
  • English
  • Français

Inertial focusing of finite-size particles in microchannels

Published online by Cambridge University Press:  14 February 2018

Evgeny S. Asmolov Open the ORCID record for Evgeny S. Asmolov [Opens in a new window] ,
Alexander L. Dubov ,
Tatiana V. Nizkaya ,
Jens Harting  and
Olga I. Vinogradova
Evgeny S. Asmolov*
Affiliation:
A.N. Frumkin Institute of Physical Chemistry and Electrochemistry, Russian Academy of Sciences, 31 Leninsky Prospect, 119071 Moscow, Russia Institute of Mechanics, M. V. Lomonosov Moscow State University, 119991 Moscow, Russia
Alexander L. Dubov
Affiliation:
A.N. Frumkin Institute of Physical Chemistry and Electrochemistry, Russian Academy of Sciences, 31 Leninsky Prospect, 119071 Moscow, Russia
Tatiana V. Nizkaya
Affiliation:
A.N. Frumkin Institute of Physical Chemistry and Electrochemistry, Russian Academy of Sciences, 31 Leninsky Prospect, 119071 Moscow, Russia
Jens Harting
Affiliation:
Helmholtz Institute Erlangen-Nürnberg for Renewable Energy, Forschungszentrum Jülich, Fürther Str. 248, 90429 Nürnberg, Germany Department of Applied Physics, Eindhoven University of Technology, PO Box 513, 5600MB Eindhoven, The Netherlands Faculty of Science and Technology, University of Twente, 7500 AE Enschede, The Netherlands
Olga I. Vinogradova*
Affiliation:
A.N. Frumkin Institute of Physical Chemistry and Electrochemistry, Russian Academy of Sciences, 31 Leninsky Prospect, 119071 Moscow, Russia Department of Physics, M. V. Lomonosov Moscow State University, 119991 Moscow, Russia DWI – Leibniz Institute for Interactive Materials, Forckenbeckstr. 50, 52056 Aachen, Germany
*
†Email addresses for correspondence: [email protected], [email protected]
†Email addresses for correspondence: [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

At finite Reynolds numbers, $Re$, particles migrate across laminar flow streamlines to their equilibrium positions in microchannels. This migration is attributed to a lift force, and the balance between this lift and gravity determines the location of particles in channels. Here we demonstrate that velocity of finite-size particles located near a channel wall differs significantly from that of an undisturbed flow, and that their equilibrium position depends on this, referred to as slip velocity, difference. We then present theoretical arguments, which allow us to generalize expressions for a lift force, originally suggested for some limiting cases and 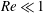$Re\ll 1$, to finite-size particles in a channel flow at 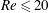$Re\leqslant 20$. Our theoretical model, validated by lattice Boltzmann simulations, provides considerable insight into inertial migration of finite-size particles in a microchannel and suggests some novel microfluidic approaches to separate them by size or density at a moderate 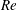$Re$.

JFM classification

Micro-/Nano-fluid dynamics: Microfluidics Multiphase and Particle-laden Flows: Particle/fluid flow Complex Fluids: Suspensions
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 840 , 10 April 2018 , pp. 613 - 630
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Asmolov, E. S. 1999 The inertial lift on a spherical particle in a plane Poiseuille flow at large channel Reynolds number. J. Fluid Mech. 381, 63–87.Google Scholar
Asmolov, E. S., Dubov, A. L., Nizkaya, T. V., Kuehne, A. J. C. & Vinogradova, O. I. 2015 Principles of transverse flow fractionation of microparticles in superhydrophobic channels. Lab on a Chip 15 (13), 2835–2841.Google Scholar
Benzi, R., Succi, S. & Vergassola, M. 1992 The lattice Boltzmann equation: theory and applications. Phys. Rep. 222, 145–197.Google Scholar
Bhagat, A. A. S., Kuntaegowdanahalli, S. S. & Papautsky, I. 2008 Continuous particle separation in spiral microchannels using dean flows and differential migration. Lab on a Chip 8 (11), 1906–1914.Google Scholar
Cherukat, P. & McLaughlin, J. B. 1994 The inertial lift on a rigid sphere in a linear shear flow field near a flat wall. J. Fluid Mech. 263, 1–18.Google Scholar
Choi, Y.-S., Seo, K.-W. & Lee, S.-J. 2011 Lateral and cross-lateral focusing of spherical particles in a square microchannel. Lab on a Chip 11 (3), 460–465.Google Scholar
Chun, B. & Ladd, A. J. C. 2006 Inertial migration of neutrally buoyant particles in a square duct: an investigation of multiple equilibrium positions. Phys. Fluids 18, 031704.Google Scholar
Cox, R. G. & Hsu, S. K. 1977 The lateral migration of solid particles in a laminar flow near a plane. Intl J. Multiphase Flow 3, 201–222.Google Scholar
Davis, A. M. J., Kezirian, M. T. & Brenner, H. 1994 On the Stokes–Einstein model of surface diffusion along solid surfaces: slip boundary conditions. J. Colloid Interface Sci. 165 (1), 129–140.CrossRefGoogle Scholar
Di Carlo, D., Edd, J. F., Humphry, K. J., Stone, H. A. & Toner, M. 2009 Particle segregation and dynamics in confined flows. Phys. Rev. Lett. 102 (9), 094503.Google Scholar
Di Carlo, D., Irimia, D., Tompkins, R. G. & Toner, M. 2007 Continuous inertial focusing, ordering, and separation of particles in microchannels. Proc. Natl Acad. Sci. USA 104 (48), 18892–18897.CrossRefGoogle ScholarPubMed
Dubov, A. L., Schmieschek, S., Asmolov, E. S., Harting, J. & Vinogradova, O. I. 2014 Lattice–Boltzmann simulations of the drag force on a sphere approaching a superhydrophobic striped plane. J. Chem. Phys. 140 (3), 034707.Google Scholar
Dutz, S., Hayden, M. E. & Häfeli, U. O. 2017 Fractionation of magnetic microspheres in a microfluidic spiral: interplay between magnetic and hydrodynamic forces. PLOS ONE 12 (1), e0169919.CrossRefGoogle Scholar
Feuillebois, F. 2004 Perturbation Problems at Low Reynolds Number, Lecture Notes-AMAS.Google Scholar
Feuillebois, F., Bazant, M. Z. & Vinogradova, O. I. 2010 Transverse flow in thin superhydrophobic channels. Phys. Rev. E 82, 055301(R).Google ScholarPubMed
Goldman, A. J., Cox, R. G. & Brenner, H. 1967 Slow viscous motion of a sphere parallel to a plane wall - II Couette flow. Chem. Engng Sci. 22, 653–660.CrossRefGoogle Scholar
Happel, J. & Brenner, H. 1965 Low Reynolds Number Hydrodynamics With Special Applications to Particulate Media. Prentice-Hall.Google Scholar
Harting, J., Frijters, S., Ramaioli, M., Robinson, M., Wolf, D. E. & Luding, S. 2014 Recent advances in the simulation of particle-laden flows. Eur. Phys. J. Spec. Topics 223, 2253–2267.Google Scholar
Ho, B. P. & Leal, L. G. 1974 Inertial migration of rigid spheres in two-dimensional unidirectional flows. J. Fluid Mech. 65, 365–400.Google Scholar
Hood, K., Kahkeshani, S., Di Carlo, D. & Roper, M. 2016 Direct measurement of particle inertial migration in rectangular microchannels. Lab on a Chip 16, 2840–2850.Google Scholar
Hood, K., Lee, S. & Roper, M. 2015 Inertial migration of a rigid sphere in three-dimensional Poiseuille flow. J. Fluid Mech. 765, 452–479.CrossRefGoogle Scholar
Janoschek, F.2013 Mesoscopic simulation of blood and general suspensions in flow. PhD thesis, Eindhoven University of Technology.Google Scholar
Janoschek, F., Toschi, F. & Harting, J. 2010 Simplified particulate model for coarse-grained hemodynamics simulations. Phys. Rev. E 82, 056710.Google Scholar
Kilimnik, A., Mao, W. & Alexeev, A. 2011 Inertial migration of deformable capsules in channel flow. Phys. Fluids 23 (12), 123302.Google Scholar
Krishnan, G. P. & Leighton, D. T. Jr. 1995 Inertial lift on a moving sphere in contact with a plane wall in a shear flow. Phys. Fluids 7 (11), 2538–2545.Google Scholar
Kunert, C., Harting, J. & Vinogradova, O. I. 2010 Random-roughness hydrodynamic boundary conditions. Phys. Rev. Lett. 105 (1), 016001.Google Scholar
Ladd, A. J. C. & Verberg, R. 2001 Lattice–Boltzmann simulations of particle-fluid suspensions. J. Stat. Phys. 104 (5), 1191.Google Scholar
Liu, C., Hu, G., Jiang, X. & Sun, J. 2015 Inertial focusing of spherical particles in rectangular microchannels over a wide range of Reynolds numbers. Lab on a Chip 15 (4), 1168–1177.CrossRefGoogle Scholar
Liu, C., Xue, C., Sun, J. & Hu, G. 2016 A generalized formula for inertial lift on a sphere in microchannels. Lab on a Chip 16 (5), 884–892.CrossRefGoogle ScholarPubMed
Loisel, V., Abbas, M., Masbernat, O. & Climent, E. 2015 Inertia-driven particle migration and mixing in a wall-bounded laminar suspension flow. Phys. Fluids 27 (12), 123304.Google Scholar
Martel, J. M. & Toner, M. 2014 Inertial focusing in microfluidics. Annu. Rev. Biomed. Engng 16, 371–396.Google Scholar
Matas, J.-P., Morris, J. F. & Guazzelli, E. 2004 Inertial migration of rigid spherical particles in Poiseuille flow. J. Fluid Mech. 515, 171–195.CrossRefGoogle Scholar
Matas, J.-P., Morris, J. F. & Guazzelli, E. 2009 Lateral force on a rigid sphere in large-inertia laminar pipe flow. J. Fluid Mech. 621, 59–67.Google Scholar
Miura, K., Itano, T. & Sugihara-Seki, M. 2014 Inertial migration of neutrally buoyant spheres in a pressure-driven flow through square channels. J. Fluid Mech. 749, 320–330.Google Scholar
Morita, Y., Itano, T. & Sugihara-Seki, M. 2017 Equilibrium radial positions of neutrally buoyant spherical particles over the circular cross-section in Poiseuille flow. J. Fluid Mech. 813, 750–767.Google Scholar
Neto, C., Evans, D., Bonaccurso, E., Butt, H. J. & Craig, V. J. 2005 Boundary slip in Newtonian liquids: a review of experimental studies. Rep. Prog. Phys. 68, 2859–2897.Google Scholar
Pasol, L., Sellier, A. & Feuillebois, F. 2006 A sphere in a second degree polynomial creeping flow parallel to a wall. Q. J. Mech. Appl. Maths 59 (4), 587–614.Google Scholar
Pimponi, D., Chinappi, M., Gualtieri, P. & Casciola, C. M. 2014 Mobility tensor of a sphere moving on a superhydrophobic wall: application to particle separation. Microfluid. Nanofluid. 16, 571–585.Google Scholar
Reschiglian, P., Melucci, D., Torsi, G. & Zattoni, A. 2000 Standardless method for quantitative particle-size distribution studies by gravitational field-flow fractionation. Application to silica particles. Chromatographia 51 (1–2), 87–94.CrossRefGoogle Scholar
Saffman, P. G. 1965 The lift on a small sphere in a slow shear flow. J. Fluid Mech. 22, 385–400.Google Scholar
Schmieschek, S., Belyaev, A. V., Harting, J. & Vinogradova, O. I. 2012 Tensorial slip of super-hydrophobic channels. Phys. Rev. E 85, 016324.Google Scholar
Schonberg, J. A. & Hinch, E. J. 1989 Inertial migration of a sphere in Poiseuille flow. J. Fluid Mech. 203, 517–524.Google Scholar
Segré, G. & Silberberg, A. 1962 Behaviour of macroscopic rigid spheres in Poiseuille flow. Part 2. Experimental results and interpretation. J. Fluid Mech. 14, 136–157.Google Scholar
Vasseur, P. & Cox, R. G. 1976 The lateral migration of a spherical particle in two-dimensional shear flows. J. Fluid Mech. 78, 385–413.Google Scholar
Vinogradova, O. I. 1996 Hydrodynamic interaction of curved bodies allowing slip on their surfaces. Langmuir 12, 5963–5968.Google Scholar
Vinogradova, O. I. 1999 Slippage of water over hydrophobic surfaces. Intl J. Miner. Process. 56, 31–60.Google Scholar
Vinogradova, O. I. & Belyaev, A. V. 2011 Wetting, roughness and flow boundary conditions. J. Phys.: Condens. Matter 23, 184104.Google Scholar
Wakiya, S., Darabaner, C. L. & Mason, S. G. 1967 Particle motions in sheared suspensions XXI: interactions of rigid spheres (theoretical). Rheol. Acta 6 (3), 264–273.Google Scholar
Yahiaoui, S. & Feuillebois, F. 2010 Lift on a sphere moving near a wall in a parabolic flow. J. Fluid Mech. 662, 447–474.Google Scholar
Zhang, J., Yan, S., Alici, G., Nguyen, N.-T., Di Carlo, D. & Li, W. 2014 Real-time control of inertial focusing in microfluidics using dielectrophoresis (dep). RSC Adv. 4 (107), 62076–62085.Google Scholar
Zhang, J., Yan, S., Yuan, D., Alici, G., Nguyen, N.-T., Warkiani, M. E. & Li, W. 2016 Fundamentals and applications of inertial microfluidics: a review. Lab on a Chip 16 (1), 10–34.Google Scholar