Article contents
Impact of inlet gas turbulence on the formation, development and breakup of interfacial waves in a two-phase mixing layer
Published online by Cambridge University Press: 29 June 2021
Abstract
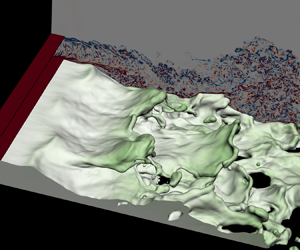
Understanding the development and breakup of interfacial waves in a two-phase mixing layer between the gas and liquid streams is paramount to atomization. Due to the velocity difference between the two streams, the shear on the interface triggers a longitudinal instability, which develops to interfacial waves that propagate downstream. As the interfacial waves grow spatially, transverse modulations arise, turning the interfacial waves from quasi-two-dimensional to fully three-dimensional. The inlet gas turbulence intensity has a strong impact on the interfacial instability. Therefore, parametric direct numerical simulations are performed in the present study to systematically investigate the effect of the inlet gas turbulence on the formation, development and breakup of the interfacial waves. The open-source multiphase flow solver, PARIS, is used for the simulations and the mass–momentum consistent volume-of-fluid method is used to capture the sharp gas–liquid interfaces. Two computational domain widths are considered and the wide domain will allow a detailed study of the transverse development of the interfacial waves. The dominant frequency and spatial growth rate of the longitudinal instability are found to increase with the inlet gas turbulence intensity. The dominant transverse wavenumber, determined by the Rayleigh–Taylor instability, scales with the longitudinal frequency, so it also increases with the inlet gas turbulence intensity. The holes formed in the liquid sheet are important to the disintegration of the interfacial waves. The hole formation is influenced by the inlet gas turbulence. As a result, the sheet breakup dynamics and the statistics of the droplets formed also change accordingly.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 13
- Cited by



