Article contents
The impact of imperfect heat transfer on the convective instability of a thermal boundary layer in a porous media
Published online by Cambridge University Press: 30 March 2016
Abstract
We consider convective instability in a deep porous medium cooled from above with a linearised thermal exchange at the upper surface, thus determining the impact of using a Robin boundary condition, in contrast to previous studies using a Dirichlet boundary condition. With the linearised surface exchange, the thermal flux out of the porous layer depends linearly on the temperature difference between the effective temperature of a heat sink at the upper boundary and the temperature at the surface of the porous layer. The rate of this exchange is characterised by a dimensionless Biot number,  $\mathit{Bi}$, determined by the effective thermal conductivity of exchange with the heat sink relative to the physical thermal conductivity of the porous layer. For a given temperature difference between the heat sink at the upper boundary and deep in the porous medium, we find that imperfectly cooled layers with finite Biot numbers are more stable to convective instabilities than perfectly cooled layers which have large, effectively infinite Biot numbers. Two regimes of behaviour were determined with contrasting stability behaviour and characteristic scales. When the Biot number is large the near-perfect heat transfer produces small corrections of order
$\mathit{Bi}$, determined by the effective thermal conductivity of exchange with the heat sink relative to the physical thermal conductivity of the porous layer. For a given temperature difference between the heat sink at the upper boundary and deep in the porous medium, we find that imperfectly cooled layers with finite Biot numbers are more stable to convective instabilities than perfectly cooled layers which have large, effectively infinite Biot numbers. Two regimes of behaviour were determined with contrasting stability behaviour and characteristic scales. When the Biot number is large the near-perfect heat transfer produces small corrections of order 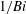 $1/\mathit{Bi}$ to the perfectly conducting behaviour found when the Biot number is infinite. In the insulating limit as the Biot number approaches zero, a different behaviour was found with significantly larger scales for the critical wavelength and depth of convection both scaling proportional to
$1/\mathit{Bi}$ to the perfectly conducting behaviour found when the Biot number is infinite. In the insulating limit as the Biot number approaches zero, a different behaviour was found with significantly larger scales for the critical wavelength and depth of convection both scaling proportional to 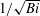 $1/\sqrt{\mathit{Bi}}$.
$1/\sqrt{\mathit{Bi}}$.
- Type
- Papers
- Information
- Copyright
- © 2016 Cambridge University Press
References
- 10
- Cited by



