2.1. Numerical solver

The numerical solutions presented in this paper are obtained by solving the incompressible Navier–Stokes equations,

(2.1)\begin{equation} \frac{\partial u_i}{\partial t}+ u_j \frac{\partial u_i}{\partial x_j} ={-}\frac{1}{\rho}\frac{\partial p}{\partial x_i} + \nu \frac{\partial^{2} u_i }{\partial x_j \partial x_j} , \end{equation}

under the divergence-free constraint, $\partial u_i / \partial x_i=0$, where $u_i$ and $p$ are the instantaneous velocity and pressure fields, $x_i$ and $t$ are the spatial coordinates and time and $\rho$ and $\nu$ are the fluid density and kinematic viscosity.

These equations are discretised in space and integrated in time using the high-order solver Nek5000, developed by Fischer, Lottes & Kerkemeier (Reference Fischer, Lottes and Kerkemeier2010), with adaptive mesh refinement (AMR) capabilities developed at KTH (Peplinski et al. Reference Peplinski, Offermans, Fischer and Schlatter2018; Offermans Reference Offermans2019; Offermans et al. Reference Offermans, Peplinski, Marin and Schlatter2020; Tanarro et al. Reference Tanarro, Mallor, Offermans, Peplinski, Vinuesa and Schlatter2020). Nek5000 is based on the spectral-element method (Patera Reference Patera1987), essentially a high-order finite-element method, which combines the flexibility of the finite-element formulation in meshing complex geometries with the numerical accuracy of spectral methods (Deville, Fischer & Mund Reference Deville, Fischer and Mund2002). Inside each element, the velocity field is represented by a polynomial of order $q$ (here $q=7$ in all cases) using Lagrange interpolants on the Gauss–Lobatto–Legendre (GLL) points ($N=q+1$ GLL points in each direction), while the pressure is represented on $q-1$ Gauss–Legendre points following the $P_N-P_{N-2}$ formulation (Maday, Patera & Rønquist Reference Maday, Patera and Rønquist1987). The nonlinear convective term is overintegrated on a grid with $3N/2$ Gauss–Legendre points in each direction, to avoid (or reduce) aliasing errors. Time stepping is performed by an implicit third-order backward-differentiation scheme for the viscous terms and an explicit third-order extrapolation for the nonlinear terms (Karniadakis, Israeli & Orszag Reference Karniadakis, Israeli and Orszag1991). A high-pass filter relaxation term (Schlatter, Stolz & Kleiser Reference Schlatter, Stolz and Kleiser2004) is added to the right-hand side of the Navier–Stokes equations. This term provides numerical stability and acts as a subgrid-scale dissipation. This specific set-up has been used and verified in several previous studies (Schlatter et al. Reference Schlatter, Li, Brethouwer, Johansson and Henningson2010; Eitel-Amor, Örlü & Schlatter Reference Eitel-Amor, Örlü and Schlatter2014), including wing simulations (Negi et al. Reference Negi, Vinuesa, Hanifi, Schlatter and Henningson2018; Vinuesa et al. Reference Vinuesa, Negi, Atzori, Hanifi, Henningson and Schlatter2018) and flow around obstacles (Lazpita et al. Reference Lazpita, Martinez-Sanchez, Corrochano, Hoyas, Le Clainche and Vinuesa2022; Atzori et al. Reference Atzori, Torres, Vidal, Le Clainche, Hoyas and Vinuesa2023).

The standard version of Nek5000 is based on hexahedral elements of conforming topology (i.e. no ‘hanging nodes’ are allowed). The AMR version adds the capability of handling non-conforming hexahedral elements with hanging nodes, and so adds an $h$-refinement capability where each element can be refined individually. Solution continuity at non-conforming interfaces is ensured by interpolating from the ‘coarse side’ onto the ‘fine side’. The AMR version includes some modifications to the pressure solver and preconditioner (Peplinski et al. Reference Peplinski, Offermans, Fischer and Schlatter2018), as well as the stiffness matrix and its direct summation operations (Offermans Reference Offermans2019; Offermans et al. Reference Offermans, Peplinski, Marin and Schlatter2020); however, the majority of the code is identical to the standard version. The AMR version of the code has gone through an extensive verification and validation process, including wing simulations (cf. Tanarro et al. Reference Tanarro, Mallor, Offermans, Peplinski, Vinuesa and Schlatter2020) and other flows (cf. Offermans et al. Reference Offermans, Peplinski, Marin and Schlatter2020).

2.2. Computational domain and set-up

There are a total of seven different configurations studied in this work. These include four periodic (infinite-span) wing sections and three finite-span wings. All cases are based on a symmetric NACA0012 profile. Both finite- and infinite-span wings have a non-tapered and non-swept planform with zero dihedral angle and no twist. This means that the airfoil chord and angle of attack are constant in the spanwise direction, and the line that connects the leading edge of the different spanwise sections of the wing is normal to both the free-stream direction and the lift direction (figure 2). The finite-span wings have an aspect ratio (equal to the full-span-to-chord ratio for rectangular planforms) of $b/c=2s/c=1.5$ and a rounded wing-tip geometry described by a semicircle centred at $(y',z')/c=(0,0.75)$ with a radius equal to the profile thickness at that $x'$ location (see figure 2).

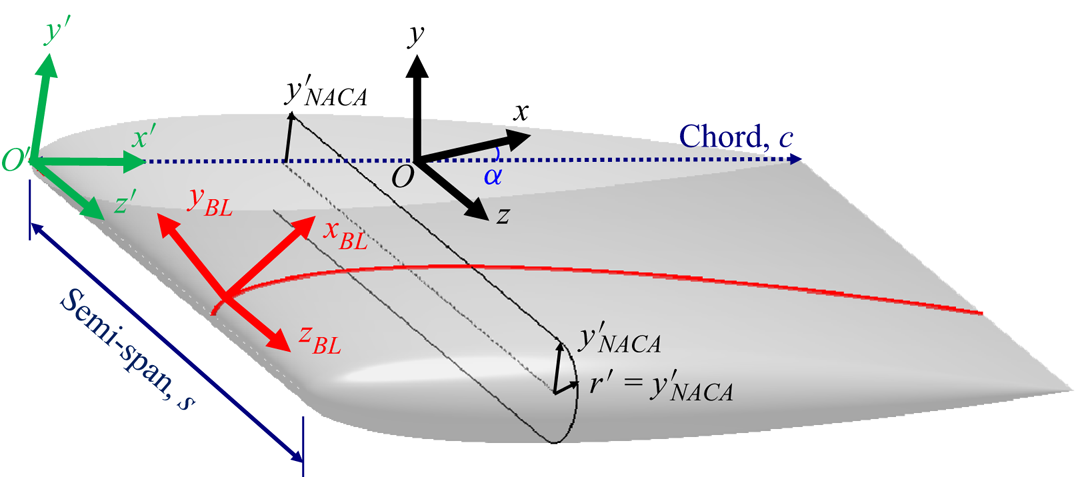
Figure 2. A schematic of the wing-surface definition and its placement in the computational domain with the global Cartesian coordinate system $(x,y,z)$ and its origin $O$ represented in black, the rotated wing coordinate system $(x',y',z')$ and its origin $O'$ represented in green, and the boundary layer coordinate system $(x_{BL},y_{BL},z_{BL})$ represented in red. Note that $(x_{BL},y_{BL},z_{BL})$ is shown at a random location on the wing for visual clarity, while its origin $O_{BL}$ is in fact on $O'$. The figure also shows the rounded (semicircle) wing-tip geometry and its definition. The wing has a chord length of $c$ and a semi-span of $s=b/2=0.75c$ (dark blue), with a geometric angle of attack of $\alpha$ (blue).

The wings are located such that their mid-chord, $(x',y',z')/c=(0.5,0,0)$, coincides with the origin of the global Cartesian coordinate system, $(x,y,z)_O=(0,0,0)$, and have a no-slip, no-penetration boundary condition. The computational domain has a rectangular cross-section in the $z$-normal plane that extends $20c$ upstream, $30c$ downstream and $20c$ in positive and negative $y$ directions. Different angles of attack are achieved by rotating the wing along the $z$ axis around its centre $(x',y')/c=(0.5,0)$, located at $(x,y)=(0,0)$, without changing the inflow boundary condition. This specific design is to allow for the use of the ‘outflow-normal’ boundary condition (cf. Deville et al. Reference Deville, Fischer and Mund2002) on $y$-normal boundaries, which allows for a non-zero $y$ component of velocity (inward at $y=20c$ and outward at $y=-20c$ in lift-generating configurations). The inflow boundary at $x=-20c$ has a Dirichlet boundary condition with $(u_1,u_2,u_3)=(U_\infty,0,0)=\boldsymbol {U}_\infty$, while the outlet boundary at $x=30c$ has the outflow boundary condition. In order to avoid backflow at the outlet there is a sponge region for all $x \geq 10c$ with a gradually increasing forcing term that acts to bring the velocity to its free-stream condition. The effect of the sponge region on the flow has been tested in a number of preliminary runs and deemed negligible for all quantities of interest here.

The finite-span wing domain extends $20c$ in the spanwise direction $z$ from the wing root (located at $z=0$) and has an ‘outflow-normal’ boundary condition at $z=20c$. A symmetry boundary condition is used at the $z=0$ plane (i.e. the wing root). While the symmetry condition forces the $z$ component of the velocity to be zero at the wing root (and thus, for instance, the corresponding Reynolds stress components), the impact of this boundary condition on turbulence quantities appears to be negligible for $z/c \geq 0.05$; therefore, simulation of full-span wings was not deemed necessary. The infinite-span (periodic) wings share the same domain design and boundary conditions in the $x$–$y$ plane but have a spanwise width of $L_z=0.6c$ with periodic boundary conditions in $z$. The wider $L_z$ in these simulations (compared with previous works, e.g. Hosseini et al. Reference Hosseini, Vinuesa, Schlatter, Hanifi and Henningson2016; Negi et al. Reference Negi, Vinuesa, Hanifi, Schlatter and Henningson2018; Vinuesa et al. Reference Vinuesa, Negi, Atzori, Hanifi, Henningson and Schlatter2018) is to allow for a more accurate simulation of the wake region (not discussed here).

The boundary layers are tripped on both the suction and pressure sides in all seven cases. The implemented trip is a randomised time-dependent wall-normal force proposed by Schlatter & Örlü (Reference Schlatter and Örlü2012) (also see Hosseini et al. Reference Hosseini, Vinuesa, Schlatter, Hanifi and Henningson2016) aimed at minimising the required development length and history effects from the trip. The finite-span wings are only tripped on the main section of the wing ($z<0.75c$), and not on the wing-tip region. On the suction side, the tripping is always located at $x'=0.1c$ regardless of the angle of attack, while on the pressure side it is at $x'=0.1c$ for the $0^\circ$, $2^\circ$ and $5^\circ$ angles of attack and at $x'=0.25c$ for $\alpha =10^\circ$. This change in the tripping location on the pressure side of the periodic wing at $\alpha =10^\circ$ was necessary since the acceleration parameter $K=(\nu /U_e^2)\,{\rm d}U_e/{\rm d}\kern0.07em x$ (where $U_e$ is the velocity at the boundary layer edge) far exceeded the rule-of-thumb value of $(2.5\unicode{x2013}3)\times 10^{-6}$ for relaminarisation (Spalart Reference Spalart1986; Yuan & Piomelli Reference Yuan and Piomelli2015) and the added energy in the tripping region was dissipated before generating turbulence. While this relaminarisation was only present in the periodic case, an early decision was made to match the location of the trip for finite- and infinite-span wings at the same geometric angle of attack $\alpha$. As will be seen in § 4, this was not an optimal choice; however, since it did not have an impact on the quantities studied here and due to the excessive cost of the simulations, this was not modified later.

2.3. Computational grids

The production grids used in this study are generated by iteratively adapting (i.e. refining) an initial grid using the solution-based spectral error indicator introduced by Mavriplis (Reference Mavriplis1990) for turbulent flows. From a mathematical point of view, this error indicator is an approximation of the interpolation error in the numerically obtained velocity field compared with its estimated exact counterpart (in an $L^2$-norm sense), computed by estimating the truncation and quadrature errors (Offermans et al. Reference Offermans, Peplinski, Marin and Schlatter2020; Tanarro et al. Reference Tanarro, Mallor, Offermans, Peplinski, Vinuesa and Schlatter2020). From a physical perspective, this error indicator estimates the sum of the small-scale and unresolved turbulent kinetic energy (Toosi & Larsson Reference Toosi and Larsson2017), and corresponds to the numerical, modelling (from the large-eddy simulation model) and projection errors (Toosi Reference Toosi2019).

The initial grid for the periodic cases is conformal and originally two-dimensional, which is then extruded (i.e. copied to an appropriate number of spanwise locations) to make sure that the spanwise homogeneity of the mesh is maintained. Different angles of attack share the same near-wing mesh (up to a few boundary layer thicknesses), which is rotated with the wing. The root of the finite-span wing shares a nearly identical mesh with the periodic counterpart.

The production grids are generated by iterative refinement of the initial grids, where at each iteration elements with the highest contribution to solution error, identified by the volume-weighted error indicator (cf. Lapenta Reference Lapenta2003; Park Reference Park2003; Toosi & Larsson Reference Toosi and Larsson2020), are selected for refinement. The convergence process is accelerated by some manual input from the user; for instance, by manually marking the wall elements for refinement. The initial grids are designed to reach their desired wall resolution after four refinements of the near-wall elements. The automatic refinement is continued for a few iterations (where in the first four the wall elements are manually marked for refinement), and the refinement regions are then manually extended for three more iterations (i.e. each element is refined if any of its neighbouring elements is refined). This last step helps to avoid repeating the refinement process further (a process which becomes expensive for these progressively finer grids) and leads to a smoother and more uniform mesh. The adaptation process is terminated after reaching the resolution criteria from literature (such as those used in Vinuesa et al. (Reference Vinuesa, Hosseini, Hanifi, Henningson and Schlatter2017b, Reference Vinuesa, Negi, Atzori, Hanifi, Henningson and Schlatter2018)), expressed in terms of the viscous length scale $\delta _\nu =\nu \sqrt {\rho /\tau _w}$ (where $\tau _w$ is the wall shear stress) for the boundary layer mesh, and the Kolmogorov length scale $\eta =(\nu ^3/\epsilon )^{1/4}$ (where $\epsilon$ is the local isotropic dissipation rate) for the wake region (cf. Pope Reference Pope2000).

Table 1 summarises some of the characteristics of the production grids used in this work. Note that the finite-span wings require significantly larger numbers of grid points compared with the periodic cases. This is partly to resolve the tip region and the larger span of these wings, and partly because of the decision to perform these simulations at slightly higher resolutions due to the potential insufficiency of the resolution criteria originally verified for canonical flows. For similar reasons, wings at higher angles of attack require higher numbers of grid points to accurately resolve all the important features of their more complex flow field with stronger vortices and secondary flows.

Table 1. Description of the production grids used in this study. The naming convention distinguishes the different set-ups by P-$\alpha$ for periodic wings at an angle of attack of $\alpha$ and RWT-$\alpha$ for finite-span wings at $\alpha ^\circ$ angle of attack. Here $N_{GLL}$ is the total number of GLL points (the number of independent grid points is around $0.67 N_{GLL}$). All values of $\Delta$ are based on the mean resolution computed as the element size divided by the polynomial order, whereas $\delta _1 y_{BL}$ is the distance from the wall of the first GLL point off the wall. Wall resolutions, including both $\Delta \ast _{BL}$ and $\Delta \ast _{tip}$, are reported along the wall coordinates $(x_{BL},y_{BL},z_{BL})$ (figure 2). The boundary layer resolutions $\Delta \ast _{BL}$ are normalised by the viscous length $\delta _\nu$ and reported at $(x',z')/c=(0.7,0.3)$ for the element on the wall. Tip resolutions $\Delta \ast _{tip}$ are normalised by the chord $c$ and reported at $x'/c=0.7$ and $z'=z'_{\rm max}$. Wake resolutions $\Delta \ast _{wake}$ are reported at $(x,z)/c=(2,0.3)$ at the location of minimum mean velocity.

Figure 3 shows the spectral elements of the RWT-10 grid, as a representative example of the grids used in this study, with instantaneous vortical structures of the flow visualised using the $\lambda _2$ vortex identification method (Jeong & Hussain Reference Jeong and Hussain1995) added for visual reference.

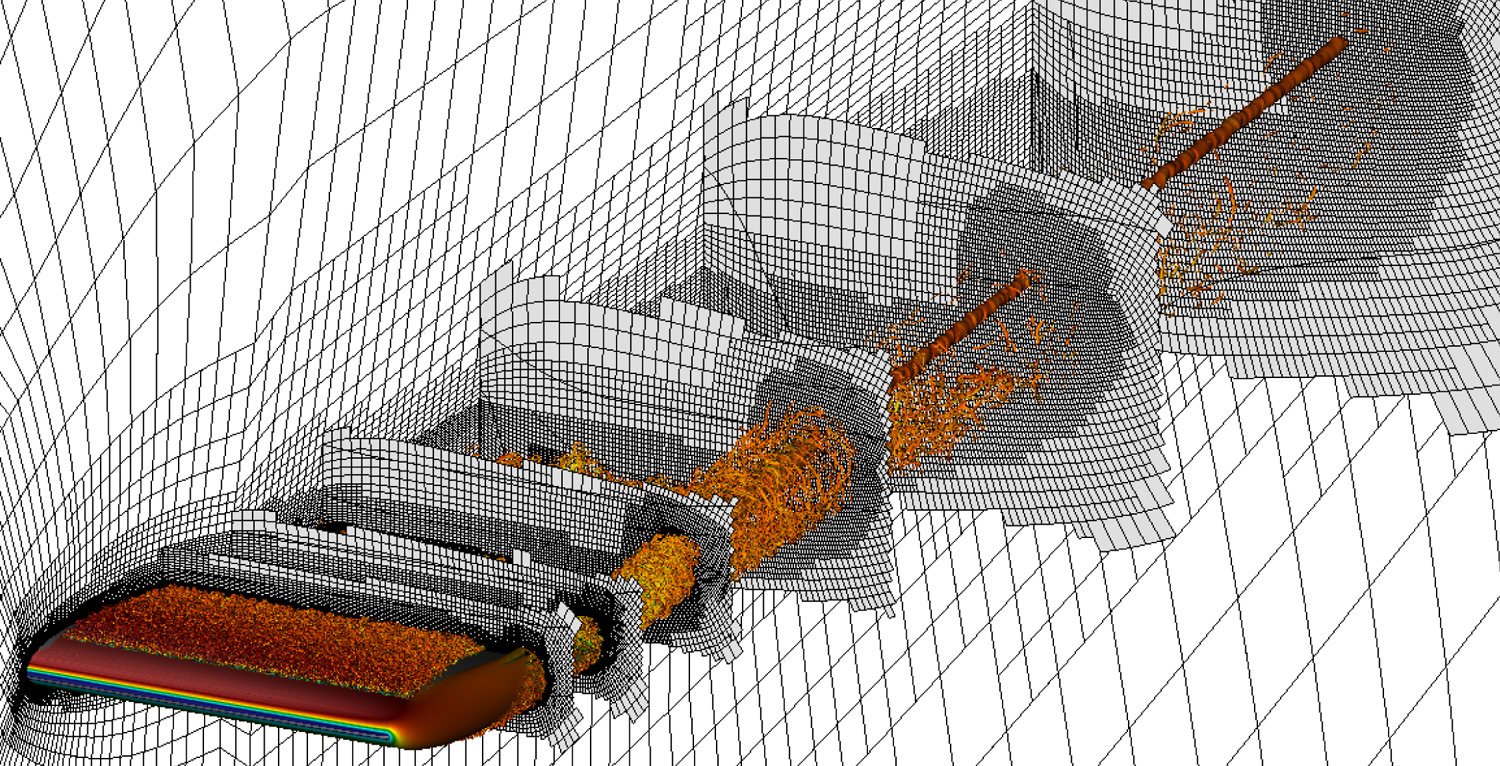
Figure 3. An example of the grids used in this study. Edges of the spectral elements of RWT-10 (with 4.23 million spectral elements; see table 1) are shown by black lines on a number of planar slices. The grid is generated using the $h$-adaptation capabilities of the AMR version of Nek5000. The figure also shows instantaneous vortical structures represented by isosurfaces of $\lambda _2 c^2/U_\infty ^2=-100$ coloured by streamwise velocity ranging from low (blue) to high (red) for visual reference.

2.4. Flow transients and statistical averaging

Flow transients are removed both during the grid-adaptation stage and after reaching the production grid. In total, a minimum of approximately $80c/U_\infty$ (equivalent to 80 convective time units, ${\mathcal {T}}_{conv}=c/U_\infty$) of integration time is discarded as transients. This comprises $50{\mathcal {T}}_{conv}$ on the coarse initial grid, roughly $25{\mathcal {T}}_{conv}$ on various adapted grids before reaching the production grid and an additional $4{\mathcal {T}}_{conv}$ on the production grid.

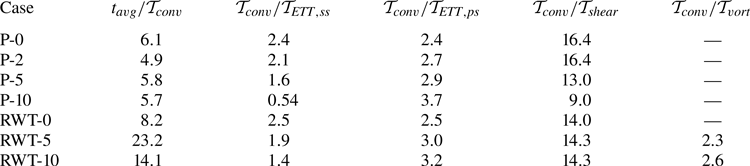
After the transient period, turbulence statistics are collected over a period of $5{\mathcal {T}}_{conv}$ or above in the periodic cases (P-0, P-2, P-5, P-10), around $8 {\mathcal {T}}_{conv}$ for RWT-0, around $14 {\mathcal {T}}_{conv}$ for RWT-10 and for a longer period of around $23 {\mathcal {T}}_{conv}$ for RWT-5. Table 2 summarises the averaging time $t_{\rm avg}$ used in each simulation in terms of the convective time unit. A conversion ratio is provided to relate ${\mathcal {T}}_{conv}$ to other time scales of the flow, including: the boundary layer eddy-turnover time ${\mathcal {T}}_{ETT}=\delta _{99}/u_\tau$, where $\delta _{99}$ is the $99\,\%$ boundary layer thickness and $u_\tau =\sqrt {\tau _w/\rho }$ is the friction velocity; the shear-layer (wake) time scale ${\mathcal {T}}_{shear}=\delta _{0.5, shear}/U_\infty$, where $\delta _{0.5, shear}$ is a measure of the wake thickness (see Pope Reference Pope2000); and the vortex-rotation time scale ${\mathcal {T}}_{vort}={\rm \pi} d_{vort}/u_{\theta,max}$, where $u_{\theta,{\rm max}}$ is the maximum azimuthal velocity around the vortex core and $d_{vort}$ is the vortex diameter measured as the distance between the two peaks in the azimuthal velocity around the core. Cases RWT-0 (which is symmetric around the $y=0$ plane and can be averaged in that direction) and RWT-5 have similar effective values of $t_{avg}/{\mathcal {T}}_{ETT,ss}$ (which is the most relevant time scale for the quantities studied here), while RWT-10 has a shorter integration time due to computational cost constraints.

Table 2. A summary of the averaging time $t_{avg}$ used in each case compared with different time scales in the flow. Here ${\mathcal {T}}_{conv}$ is the convective (flow-over) time scale, ${\mathcal {T}}_{ETT}$ is the boundary layer eddy turnover time reported at $(x',z')/c=(0.7,0.3)$, ${\mathcal {T}}_{shear}$ is the shear time scale in the wake reported at $(x,z)/c=(2.0,0.3)$ and ${\mathcal {T}}_{vort}$ is the time scale of vortex rotation reported at $x/c=2.0$. Subscripts ‘$ss$’ and ‘$ps$’ stand for the suction side and pressure side of the wing. Definition of time scales is given in the text.

The statistics are collected on the fly (see Appendix A for more details) at a sampling rate that is around an order of magnitude higher than the highest frequency of the flow, here dictated by the viscous time scale $\nu /u_\tau ^2$ (${\geq } 2 \times 10^{-3} {\mathcal {T}}_{conv}$ for cases studied here). The periodic cases are homogeneous in the spanwise direction and therefore an ensemble average in that direction is also performed when computing the statistics. While RWT-0, RWT-5 and RWT-10 are fully 3-D flows and exhibit a variation of solution statistics along the span, these variations are smooth over the turbulent boundary layer section of the wings. This allows for a spanwise filtering of the statistics using a (wide) Gaussian filter with a variable filter width that is adjusted based on flow physics and resembles an averaging process. This procedure is explained in more detail in Appendix A.

The temporally and spatially averaged (or filtered) fields are denoted by $\langle \cdot \rangle$; e.g. $\langle u_i \rangle$ and $\langle p \rangle$ for the mean velocity and mean pressure fields. The fluctuating part is then defined as the (point-wise) difference between the instantaneous and mean values; e.g. $u_i'=u_i-\langle u_i \rangle$ and $p'=p-\langle p \rangle$ for the fluctuating velocity and pressure fields.

In each case, the full statistical record is divided into four or more batches of equal size, which are used to estimate the uncertainty in solution statistics by computing the confidence intervals of each quantity of interest using the non-overlapping batch method (cf. Conway Reference Conway1963). Given the higher sensitivity of the Reynolds stresses, particularly for finite-span wings, their approximate error bars are included in the comparisons in § 4.3 and Appendix C. With the use of ensemble averaging along the span in periodic cases and the equivalent filtering in the wing-tip cases, the averaging times used in this work are deemed sufficient for the discussions here.

3.1. The instantaneous flow field

The flow around RWT-0, RWT-5 and RWT-10 is illustrated in figure 4 by the instantaneous vortical structures of the flow using the $\lambda _2$ visualisation method of Jeong & Hussain (Reference Jeong and Hussain1995). The figure visualises the turbulent boundary layers formed on the wings, the turbulent wake and the wing-tip vortex identified as a cylindrical structure (surrounded by turbulent structures) that separates from the wing somewhere close to the tip and remains coherent for a long distance downstream of the wing (the entire field of view in figure 4). Note that the wing-tip vortex is only present in lift-generating configurations and absent in RWT-0. As expected, RWT-10 has a stronger wing-tip vortex (leading to a larger vortex diameter for a fixed value of $\lambda _2$) which impacts a larger portion of the wing, and more strongly, compared with RWT-5. While not directly relevant to the discussions of § 4, it is interesting to note that the vortex core in RWT-10 has a higher streamwise velocity compared with RWT-5, which is associated in the literature with the lower core pressure of the stronger wing-tip vortex and thus flow entrainment into the core region (cf. Lee & Pereira Reference Lee and Pereira2010).

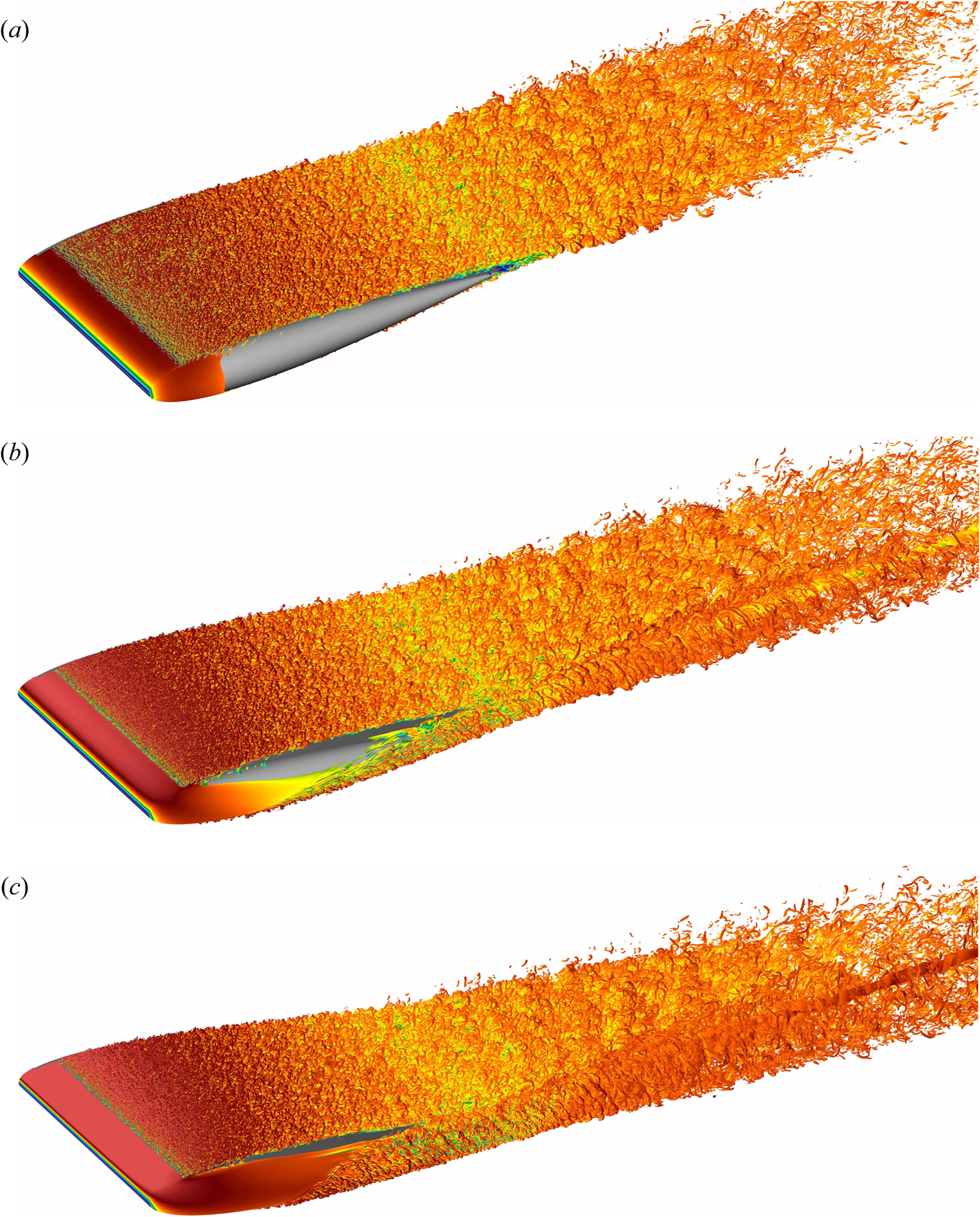
Figure 4. An overview of the flow around the finite-span wings: (a) RWT-0, (b) RWT-5 and (c) RWT-10. Plots show the instantaneous vortical structures, visualised by iso-surfaces of $\lambda _2 c^2/U_\infty ^2=-100$ coloured by $u_1/U_\infty$ from $-$0.3 (blue) to 1.3 (red). The wing surface is shown in light grey.

The location of tripping and its effectiveness is visually clear in figure 4 by the absence of turbulent structures upstream of the trip and the presence of hairpin vortices (which the trip introduces through wall-normal forcing) downstream of the tripping line. The relatively low friction Reynolds number of the flow ($200 \lesssim Re_\tau \lesssim 300$; see tables 3–5 in Appendix C) is apparent from the hairpin-dominated structure of the turbulent boundary layers (cf. Eitel-Amor et al. Reference Eitel-Amor, Örlü, Schlatter and Flores2015). Note that on both the suction and pressure sides the tripping line extends throughout the span of the wing from the root to the tip, but does not include the wing-tip region (the semicircle shown in figure 2). This results in a laminar flow around the wing tip and a laminar–turbulent interface at the spanwise end of the tripping line.

The figure shows a strong flow convection from the pressure side to the suction side (visualised by the convected turbulent structures from the pressure side), starting from the leading edge of the wing. This underscores the global impact of the finite span of the wing and the wing-tip vortices on the entire flow field. Case RWT-10 generates a higher lift and thus has stronger pressure gradients, leading to a stronger flow convection, stronger wing-tip vortices and stronger three-dimensionality in the flow field.

It is also important to note the appearance of a non-turbulent area on the suction side of RWT-5 and RWT-10 in a region close to the wing-tip vortex. This might initially suggest a relaminarisation of the turbulent boundary layer due to the increased pressure gradient, rotation and flow acceleration in the vicinity of the wing-tip vortex. However, upon further investigation this was proved not to be the case, since the turbulent region of the boundary layer was almost exactly following the streamlines of the flow released at the edge of the boundary layer near the spanwise end of the tripping line. In other words, the appearance of this laminar region should be primarily attributed to the non-zero spanwise velocity towards the root that convects the spanwise laminar–turbulent interface of the boundary layer in that direction and causes a laminar region to appear near the wing-tip region. Nevertheless, the present behaviour does not mean that extending the tripping line to include the tip region will necessarily lead to a fully turbulent suction side, since the strong flow acceleration, rotation and pressure gradients might still be sufficiently high to result in relaminarisation.

3.2. Mean-flow streamlines

The extent of three-dimensionality of the flow and its impact on the boundary layers are shown in figure 5 using the streamlines obtained from the mean velocity field $\langle u_i \rangle$. The large deflection angle of the streamlines can be easily observed by comparison with the approaching free-stream direction (chord-wise lines). As expected, this deflection increases for all cases closer to the tip. Note that the deflection from the free-stream direction starts at the beginning of the boundary layer development and spans across a large region of the wing (almost the entire semi-span for these low-aspect-ratio wings). An interesting observation is that the streamlines of RWT-0 (which does not have a wing-tip vortex) are still impacted by the finite span of the wing and deflected towards the root, albeit with a lower deflection angle compared with RWT-5 and RWT-10. This is the reason for the appearance of a small laminar region close to the trailing edge of RWT-0 in figure 4(a). The other interesting observation is the large spanwise extent of the impacted boundary layer streamlines on the pressure side of RWT-5 and RWT-10 which is comparable to that on their suction side. On both the suction and pressure sides, the deflection angle approaches zero at the root of the wing as a result of the symmetry.

Figure 5. Contour plots of the pressure coefficient $c_p$ on the wing surface for (a,d) RWT-0, (b,e) RWT-5 and (c, f) RWT-10 on the suction side (a–c) and pressure side (d–f) of the wings. Contour plots are overlaid with streamlines released at $x'/c=0.12$ at different $z'/c$ locations (0.1, 0.26, 0.43, 0.59, 0.75). The contour levels are from $-$1 (dark blue) to 1 (dark red) in increments of 0.1. The dotted black lines on the wing surface show the chord-wise direction (i.e. constant $z'$, equivalent to the approaching free-stream direction) at the same spanwise locations as the streamlines are released as a visual reference for their deflection. Arrows indicate the approximate direction of the free-stream velocity $\boldsymbol{U}_\infty$.

The more interesting observation from figure 5 is that the deflection angle of streamlines varies in the wall-normal direction across the boundary layer thickness $\delta _{99}$. The streamlines closer to the wall have a larger deflection angle compared with those farther from the wall, and this is true on both the suction and pressure sides and at all spanwise locations. This is a common feature observed in many 3-D boundary layers (cf. Johnston Reference Johnston1960; Perry & Joubert Reference Perry and Joubert1965; Pierce et al. Reference Pierce, McAllister and Tennant1983; Ölçmen & Simpson Reference Ölçmen and Simpson1995; Devenport & Lowe Reference Devenport and Lowe2022) which happens due to the lateral pressure gradient encountered by the boundary layer and the variable balance between the different terms of the momentum equation, such that the fluid closer to the wall (which has a lower momentum) responds faster to the pressure gradient (cf. Devenport & Lowe (Reference Devenport and Lowe2022) and references therein). The variable deflection angle of the streamlines in the streamwise, wall-normal and spanwise directions has a number of consequences on the boundary layers, which is discussed further in § 4.

The wing-tip vortices are visible in RWT-5 and RWT-10 in figure 5(b,c) as streamlines that are clustered together and formed into spirals on the suction side of the wings. Additional details about the flow in the vicinity of the tip can be found in Appendix B. The generated wing-tip vortex impacts the surrounding flow differently depending on the distance. This is primarily related to the velocity induced by the vortex (the Biot–Savart law), which is proportional to the inverse of the distance from the vortex core (refer to the caption of figure 1) and approximately in the azimuthal direction of a cylindrical coordinate system with the vortex core at its origin. Due to the large variations in both magnitude and direction of the induced velocity, the flow in the vicinity of the vortex core is highly non-homogeneous. The flow acceleration due to pressure gradient is also larger near the tip. At larger spanwise distances from the vortex – and farther downstream from the location of its initial formation – the induced velocity on the wing surface becomes a nearly unidirectional downwash with a variable magnitude (and, thus, a variable effective angle of attack along the span). We take advantage of this behaviour of finite-span wings to facilitate the analyses in § 4.

Figure 5 also shows the contour lines of the pressure coefficient $c_p=2(p-p_\infty )/\rho U_\infty ^2$ on the surface of the wings. Note that the wall-parallel component of pressure gradient (at the wall and across the boundary layer thickness) is orthogonal to the constant $c_p$ lines represented in the figure. An increasing trend in the pressure coefficient (and therefore pressure) can be observed approaching the tip region. This pressure gradient is the main cause for the observed streamline deflections in RWT-0. The boundary layer streamlines on the suction side of RWT-5 and RWT-10 are deflected towards the root as a result of these pressure gradients and the high momentum of the flow approaching the suction side from the pressure side. There is also a pressure gradient on the pressure sides of RWT-5 and RWT-10, albeit weaker, which causes the flow to accelerate towards the tip of the wing. On both sides, the pressure gradient decreases in magnitude and becomes more aligned with the streamlines closer to the wing root (characterised by contour level lines that are more aligned with the spanwise direction and farther apart from each other). This implies that the fluid particles following the streamlines are subjected to reduced accelerations due to pressure gradient. Consequently, the streamlines approximate straighter lines, leading to less variation in the deflection along a given streamline. This in turn mitigates some of the 3-D effects that result from the skewed velocity profile.

4.1. The impact of the effective angle of attack

Figure 6 plots the $99\,\%$ boundary layer thickness $\delta _{99}$ defined based on the diagnostic scaling (Vinuesa et al. Reference Vinuesa, Bobke, Örlü and Schlatter2016) and the Clauser pressure-gradient parameter $\beta _{x_{BL}}$ (Clauser Reference Clauser1954, Reference Clauser1956), at a spanwise location near the root. The Clauser parameter is computed along $x_{BL}$ (defined in figure 2) as

(4.1)\begin{equation} \beta_{x_{BL}} = \frac{\delta^*}{\vert \tau_{w} \vert} \frac{\partial P_e}{\partial x_{BL}} , \end{equation}

where $\delta ^*$ is the boundary layer displacement thickness, $\vert \tau _{w} \vert$ is the shear-stress magnitude at the wall and $P_e=\langle p(x_{BL},\delta _{99},z_{BL}) \rangle$ is the pressure at the edge of the boundary layer.

Figure 6. The $99\,\%$ boundary layer thickness $\delta _{99}$ (a,b) and the Clauser pressure-gradient parameter $\beta _{x_{BL}}$ (c,d) plotted at $z'/c=0.1$ (close to the root) on the suction side (a,c) and pressure side (b,d) of the wings of this study. The finite-span wings are plotted in solid lines, while periodic airfoils are shown by the dotted lines. Colours, from light to dark, correspond to angles of attack of $\alpha =0^\circ$, $2^\circ$, $5^\circ$ and $10^\circ$, respectively, for infinite-span wings. The three finite-span wings are shown with matched colours to the infinite-span ones at the same $\alpha$. The observed differences are mainly attributed to the impact of the reduced effective angle of attack $\alpha _{eff}$ (an inviscid effect caused by the induced downwash of the wing-tip vortex; see figure 1) on the boundary layers.

There is a large difference between the boundary layers formed on the finite-span wings compared with the periodic ones at the same angle of attack. Namely, the boundary layers on the suction sides are thinner and encounter lower adverse pressure gradients (lower $\beta _{x_{BL}}$ values), while they are thicker on the pressure side and encounter a larger Clauser parameter (interesting to note that $\beta _{x_{BL}}>0$ over the majority of the pressure side as well). Furthermore, the discrepancies are larger for higher angles of attack and nearly vanish for $\alpha =0^\circ$. These are all consequences of the lower effective angle of attack $\alpha _{eff}$ of the finite-span wings compared with their geometric angle of attack $\alpha$ (a well-known inviscid effect caused by the induced downwash of the wing-tip vortex, explained in figure 1). This is further demonstrated by figure 7 which shows the section-wise pressure component of the lift and drag coefficients of all cases defined as

(4.2)$$\begin{gather} c_{L,p}(z_0) = \frac{2}{\rho U_\infty^2 c} \oint {(\langle p(x_{BL},0,z_0) \rangle \boldsymbol{\cdot} \boldsymbol{e}_{n})\, {\rm d}\kern0.07em{x}_{BL}}, \end{gather}$$

(4.3)$$\begin{gather}c_{D,p}(z_0) = \frac{2}{\rho U_\infty^2 c} \oint {(\langle p(x_{BL},0,z_0) \rangle \boldsymbol{\cdot} \boldsymbol{e}_{t}) \,{\rm d}\kern0.07em{x}_{BL}}, \end{gather}$$

where $\boldsymbol {e}_{n}$ is a unit vector normal to the wall and opposite to $y_{BL}$, $\boldsymbol {e}_{t}$ is a unit vector tangential to the wall in the same direction as $x_{BL}$ and $\rho$ and $c$ are the fluid density and chord length. The integrals are taken on the wing surface ($y_{BL}=0$) over both the suction and pressure sides at a fixed spanwise location $z_{BL}=z_0$. Assuming a linear variation of the lift coefficient with angle of attack in infinite-span wings (which is approximately true for relatively low angles of attack; cf. Houghton et al. Reference Houghton, Carpenter, Collicott and Valentine2013; Federal Aviation Administration 2016) we could make the approximation that $3^\circ \leq \alpha _{eff} \leq 4.5^\circ$ for RWT-10 (with it being closer to $4.5^\circ$ at the root and around $3^\circ$ close to the tip) and $1^\circ \leq \alpha _{eff} \leq 2^\circ$ for RWT-5 (the lifting-line theory (cf. Houghton et al. Reference Houghton, Carpenter, Collicott and Valentine2013) leads to similar approximated values for $\alpha _{eff}$ over the majority of the span). This justifies the fact that in figure 6 both the boundary layer thickness and the Clauser parameter on the suction side of RWT-10 are very close to those of P-5, or why the RWT-5 curves (corresponding to $\alpha _{eff} \approx 2^\circ$ at the root) are closer to P-2.

Figure 7. The section-wise (a) pressure lift and (b) pressure drag coefficients along the span for all the geometries of this study. Line styles and colours are the same as those in figure 6. The additional thin solid lines in (a) are from inviscid simulations of finite-span wings for the same configurations as RWT-0, RWT-5 and RWT-10. The nearly identical variation of pressure lift coefficient (approximately proportional to $\alpha _{eff}$) emphasises the inviscid nature of the phenomenon. Refer to figure 1(b) and its caption for a schematic explanation of the induced drag which explains the increased drag coefficient of the finite-span wings.

It is important to emphasise that the observed similarity based on effective angles of attack is in fact a result of an approximate similarity of the pressure gradient and boundary layer histories. Therefore, our reliance on $\alpha _{eff}$ in the next sections is meant as an approximate equivalence of pressure gradients and history effects. In fact, there are additional (inviscid) effects caused by a positive (i.e. away from the wall) and variable wall-normal velocity along the span, especially near the tip at locations earlier in the development of the wing-tip vortex, that are not captured by the simplified use of $\alpha _{eff}$. These effects are discussed further in § 4.3.

Figure 7(a) also compares the turbulent finite-span wings, RWT-0, RWT-5 and RWT-10, with inviscid (incompressible Euler) simulations of the same set-ups (without the tripping) performed using the open-source solver SU2 (Economon et al. Reference Economon, Palacios, Copeland, Lukaczyk and Alonso2016). The nearly identical variation of the lift (and effective angle of attack) over a large portion of the span emphasises the inviscid nature of the phenomenon. The larger observed differences near the tip should be mainly attributed to the difference in vortex formation and the resulting change in both the vertical and spanwise location of the vortex in inviscid flows. These differences are discussed briefly in Appendix B. Additional viscous effects are present near the tip and are expected to contribute to the observed differences in that region; however, they are not discussed here.

4.2. Collateral flow and transformation into wall-shear coordinates

The skewed velocity profile and the variable deflection angle of the streamlines (figure 5) result in additional non-zero velocity gradient components, and, consequently, the activation of additional production terms in the transport equations of $\tilde {{\mathsf{R}}}_{13}$, $\tilde {{\mathsf{R}}}_{23}$ and $\tilde {{\mathsf{R}}}_{33}$ (for $\tilde {{\mathsf{R}}}_{ij}=\langle u'_i u'_j \rangle _{BL}$ denoting the Reynolds stress expressed in $(x_{BL},y_{BL},z_{BL})$ coordinates) with the largest impact on the production of $\tilde {{\mathsf{R}}}_{13}$.

The main goal of this section is to use the flow characteristics to find a coordinate system that simplifies the analysis.

Figure 8 plots the deflection angle $\gamma _{stream}$ defined as the angle between the wall-parallel part of the velocity vector and the wall-parallel direction $x_{BL}$ (with the wall-normal component of the velocity vector $\langle u_2 \rangle _{BL}$ excluded). Visually, this is the angle that the streamlines of figure 5 make with the chord-wise lines (free-stream direction). While $\gamma _{stream}$ is highly variable across the boundary layer thickness, it exhibits an important quality: it is approximately constant in the most active and important region of wall turbulence, $y_{BL}^+ \leq 30$. This region of collateral flow is a common feature of many 3-D boundary layers (cf. Johnston Reference Johnston1960; Perry & Joubert Reference Perry and Joubert1965; Pierce et al. Reference Pierce, McAllister and Tennant1983; Ölçmen & Simpson Reference Ölçmen and Simpson1995; Devenport & Lowe Reference Devenport and Lowe2022). In the context of our discussion, this means that the most active part of the near-wall region can potentially be considered nearly two-dimensional in a rotated coordinate system that is aligned with the direction of the streamlines in the $y_{BL}^+ \leq 30$ region. The region above $30\delta _\nu$ still experiences a variable shear, but since both the Reynolds stresses and mean velocity gradients are significantly smaller in this region, the contribution from the new production terms (which are the product of the Reynolds stresses and the mean velocity gradient) will remain low for a large portion of the boundary layer.

Figure 8. The deflection angle (in degrees) at $x'/c=0.7$ for multiple spanwise locations from $z'/c=0.1$ to 0.6 (with 0.02 increments) on the suction side (a–c) and the pressure side (d–f) of (a,d) RWT-0, (b,e) RWT-5 and (c, f) RWT-10. Darker colours are sampled closer to the tip. Circles show the edge of the boundary layer, $\delta _{99}$. Negative (positive) $\gamma _{stream}$ values correspond to deflection towards the root (tip). Note the relatively constant value of $\gamma _{stream}$ for $y_{BL}^+ \leq 30$.

In the rest of this paper the boundary layers are studied in a coordinate system $(x_\tau,y_\tau,z_\tau )$, which is defined by rotating $(x_{BL},y_{BL},z_{BL})$ (figure 2) around its wall-normal axis $y_{BL}$ in such a way that $x_\tau$ becomes aligned with the direction of the wall-shear stress (the streamlines). Note that the orientation of this coordinate system changes across both the span and the chord of the wing (see the streamlines of figure 5). This does not affect the analysis in the rest of the paper which is performed in local coordinates.

The rotated Reynolds stress components exhibit a structure that is very close to that of a two-dimensional boundary layer, where ${\mathsf{R}}_{13} \approx {\mathsf{R}}_{23} \approx 0$ (for ${\mathsf{R}}_{ij}=\langle u'_i u'_j \rangle _\tau$ denoting the Reynolds stress expressed in $(x_\tau,y_\tau,z_\tau )$ coordinates). This simplification is possible for these boundary layers mostly because of the relatively small change in the deflection angle across the boundary layer thickness (less than $10\,^\circ$; see figure 8) and is not necessarily possible for all 3-D boundary layers. The spanwise component of the mean velocity is by definition close to zero for $y_\tau ^+\leq 30$ in this coordinate system and only increases closer to the edge of the boundary layer. This results in a Reynolds stress production term close to that of a statistically two-dimensional boundary layer for similar conditions. The obtained simplification by rotation into the $(x_\tau,y_\tau,z_\tau )$ coordinate system cannot remove the less local effects. Such effects are discussed in the next section.

4.3. Additional departures from infinite-span wings

Two other notable effects are associated with the finite span of the wing and the 3-D flow field:

1. (i) Flow acceleration from the pressure side towards the suction side, during the formation of the wing-tip vortices, results in a wall-normal velocity that is different along the chord and the span of the wing. This variation is such that the locations closer to the tip and earlier in the development of the wing-tip vortex (i.e. closer to the leading edge) experience higher positive wall-normal velocities. The wall-normal velocity impacts the development of the boundary layers, such that increased values of this quantity lead to a faster growth rate. As a result, there is a slight increase in $\delta _{99}$ on the suction side for locations closer to the tip, and similarly thinner boundary layers closer to the tip on the pressure side. This is primarily an inviscid effect.

2. (ii) Variation of the deflection angle with normal distance from the wall (a result of variable momentum across $y_\tau$, mainly due to viscous effects) means that streamlines crossing a wall-normal line have converged from different spanwise locations on the wing. Since the effective angle of attack, and thus the streamwise pressure gradients, are different at different spanwise locations (mostly an inviscid effect), fluid particles at different wall-normal locations have experienced different histories. This effect is larger closer to the tip region, where both the variation in the deflection angle (see figure 8) and the variation in the pressure gradient (approximately characterised by the effective angle of attack; see figure 7) are larger.

This section investigates and characterises these effects by comparing the boundary layers formed on the finite-span wings with their equivalent from the infinite-span wings. Equivalence is quantified here as closeness in terms of the local values of $Re_\tau$ and $\beta _{x_\tau }$, as well as similarity of their history. Appendix C explains in more detail the procedure used to match each of these quantities. The importance of this procedure is discussed briefly at the end of this section.

Figure 9 depicts the variation of the normal streamwise Reynolds stress, ${\mathsf{R}}_{11}=\langle u'_1 u'_1 \rangle _\tau$, in RWT-5 as a function of the streamwise and spanwise locations. Additional Reynolds stress components, additional locations and other angles of attack are included in figures 12–14 in Appendix C. Only a subset of those profiles that are representative of the overall trends and behaviours is shown here. We focus solely on the suction side of the wings, both here and in Appendix C. This choice is motivated by the higher Reynolds number and more interesting behaviour exhibited on the suction side. Information concerning the pressure side of the wings, as well as the suction side, including all turbulence statistics, is accessible in the simulation database (refer to the data availability statement).

Figure 9. Variation of the inner-scaled Reynolds stress ${\mathsf{R}}_{11}^+$ with (a,b) streamwise distance from the leading edge and (c,d) spanwise distance from the root. The evolution along streamlines released at (a) $(x'_{start},z'_{start})/c=(0.12,0.1)$ and (b) $(x'_{start},z'_{start})/c=(0.12,0.72)$, and the intersection of four streamlines released at $x'_{start}/c=0.12$ at spanwise locations $z'_{start}/c=0.1$, $0.31$, $0.51$, $0.72$, with planes at (c) $x'/c=0.6$ and (d) $x'/c=0.9$. Profiles are shifted vertically by $2$ units with increasing $x'$ or $z'$ for visual clarity. The profiles are from RWT-5 (solid lines) and P-2 (dashed lines), and are a subset of those plotted in figure 13. All streamlines are released in the near-wall (approximately collateral) region. See text and Appendix C for more details. Shaded regions correspond to 80 % confidence intervals of the inner-scaled Reynolds stress profiles (due to finite time averaging), computed from the non-overlapping batch method (cf. Conway Reference Conway1963). Case P-2 has extremely narrow confidence intervals which are not plotted.

The initial pattern identified in figure 9(a,b) reveals an overall increase in ${\mathsf{R}}_{11}^+={\mathsf{R}}_{11}/u_\tau ^2$ in the direction of development of the streamlines (i.e. increasing $x'$) and the emergence of a more active outer region. This is observed in both finite-span and infinite-span profiles and exhibits considerable similarity across both types. This phenomenon is attributed to the adverse pressure gradient effects, a topic extensively studied in the literature (cf. Spalart & Watmuff Reference Spalart and Watmuff1993; Perry et al. Reference Perry, Marusic and Jones2002; Aubertine & Eaton Reference Aubertine and Eaton2005; Monty et al. Reference Monty, Harun and Marusic2011; Harun et al. Reference Harun, Monty, Mathis and Marusic2013; Bobke et al. Reference Bobke, Vinuesa, Örlü and Schlatter2017; Bross et al. Reference Bross, Fuchs and Kahler2019; Devenport & Lowe Reference Devenport and Lowe2022; Pozuelo et al. Reference Pozuelo, Li, Schlatter and Vinuesa2022). Despite its significance, this phenomenon is not the primary focus of this study. Instead, our attention is devoted exclusively to exploring the differences between the finite- and infinite-span boundary layers.

Figure 9(b) demonstrates a reduction in the near-wall peak of ${\mathsf{R}}_{11}^+$, which appears to magnify downstream. This discrepancy mainly arises due to the milder adverse pressure gradient experienced by the boundary layer closer to the tip. This is a consequence of the lower effective angle of attack in that region.

Another trend evident in figure 9(b) is the increased Reynolds stress level in the outer region of the boundary layer, particularly near its edge. This effect becomes more pronounced in spanwise locations closer to the tip (compare figure 9a,b), and it displays a growing spanwise influence as one moves downstream (compare figure 9c,d). This phenomenon can be attributed to the increased wall-normal velocity and its wall-normal gradient closer to the wing's tip. This is illustrated in figure 10(a) for two streamwise locations. The increased wall-normal velocity results in increased boundary layer growth (enhanced $\partial \langle u_1 \rangle _\tau / \partial x_\tau$ due to the continuity equation) and an increased boundary layer thickness, manifesting as a non-zero Reynolds stress at greater distances from the wall. It is worth noting that the spanwise variation in wall-normal velocity diminishes as one moves downstream. Such observations suggest that the widening spanwise influence of this effect originates from the upstream state of the boundary layer, its flow development towards the root and propagation of non-zero fluctuations in the spanwise direction (due to mixing and transport).

Figure 10. The variation of wall-normal velocity along the span at two streamwise planes (a) and the variation of the outer-scaled ${\mathsf{R}}_{11}$ in the streamwise direction (b). All solid lines correspond to RWT-5. Darker colours in (a) denote locations closer to the tip at the intersection of the same streamlines used in figure 9. Profiles shown in (b) are the same as plotted in figure 9(b), with dotted lines corresponding to matched $x'$ locations from P-2, and dashed lines corresponding to matched $Re_\tau$ and $\beta _{x_\tau }$ (simple dashed lines) and matched boundary layer thickness $\delta _{99}$ (dashed lines with triangles), both from P-2. Profiles are shifted vertically by $0.01$ units in (a) and $5\times 10^{-3}$ units in (b) for visual clarity. Shaded regions in (b) correspond to 80 % confidence intervals in the outer-scaled Reynolds stress profiles (due to finite time averaging), computed from the non-overlapping batch method. Panel (a) has narrow confidence intervals which are not plotted.

At these low Reynolds numbers, the development length available for the boundary layers, proportional to $c/\delta _{99}$, is quite limited. This results in a transient response from the boundary layers to the change in their thickness, primarily characterised as a shift in the location of the outer structures away from the wall, which propagates throughout the boundary layer thickness as it develops. This hypothesis is examined in figure 10(b), where the profiles from figure 9(b) are shown in outer units. These profiles are contrasted in the figure with their infinite-span counterparts at matched $(Re_\tau, \beta _{x_\tau })$ and matched $\delta _{99}$. A shift in the location of the outer structures away from the wall would manifest itself as a coincident Reynolds stress profile near the boundary layer edge, which is indeed the observed behaviour in figure 10(b).

The wall-normal extent of agreement around the boundary layer edge decreases downstream. This phenomenon may be attributed to the development of the boundary layers and their gradual response to the changes induced by the increased boundary layer thickness. Further implications of this behaviour are discussed later.

The spanwise variation of ${\mathsf{R}}_{11}^+$ is illustrated in figure 9(c,d). A trend of diminishing magnitude in the near-wall peak of the Reynolds stress is evident, particularly when approaching the tip. This phenomenon can be attributed to a few underlying mechanisms.

First, the effective angle of attack, which varies in the spanwise direction, diminishes closer to the tip. As a result, milder adverse pressure gradients occur near the tip (characterised by lower $\beta _{x_\tau }$ values). This leads to a lower increase in the inner-scaled Reynolds stress profile and the turbulent kinetic energy due to adverse pressure gradients (in their development in the streamwise direction). Consequently, the overall inner-scaled Reynolds stress levels decrease, resulting in a diminished near-wall peak. In RWT-10 (see figure 14), there is a larger variation in $\alpha _{eff}$ and $\beta _{x_\tau }$ across the span compared with RWT-5, which can partially explain the steeper decline of the near-wall peak in RWT-10.

Closer to the tip, the increased deflection angle near the wall results in laminar flow entrainment into the turbulent boundary layers at their outmost spanwise extent. This entrainment is more pronounced in the collateral region $y_{BL}^+\leq 30$ with the highest deflection angles. Consequently, diminished near-wall turbulence activity can be expected close to the spanwise edge of the turbulent boundary layers. Such reductions in turbulence can influence the near-wall peak of ${\mathsf{R}}_{11}^+$ outside areas directly subjected to flow entrainment. This could be another reason for the observed decline of the near-wall peak for locations plotted in figure 9. Further investigations are necessary to evaluate the significance of this mechanism in comparison with others.

The other mechanism that contributes to attenuation of the near-wall peak of ${\mathsf{R}}_{11}^+$ is linked to the discussed increase in growth rate of the boundary layers near the tip. This, on the one hand, reduces the production term near the wall through decreased velocity gradients in the wall-normal direction, and, on the other, leads to additional transport away from the wall. Both of these mechanisms contribute towards reducing the peak in ${\mathsf{R}}_{11}^+$ profiles.

Another element to consider is the spanwise pressure gradient present within the boundary layers, quantified by $\beta _{z_\tau }$ in tables 3–5. To take advantage of the findings of Lozano-Durán et al. (Reference Lozano-Durán, Giometto, Park and Moin2020), an equivalent expression for their parameter $\varPi$ can be established by considering the relation $\partial \langle p \rangle / \partial x=\tau _w/\delta$ in fully developed channel flows (where $\delta$ is the channel half-height). This leads to a definition of $\varPi$ in turbulent boundary layers as

(4.4)\begin{equation} \varPi \approx \frac{\delta_{99}}{\tau_w} \frac{\partial P_e}{\partial z_\tau} = \frac{\delta_{99}}{\delta^*}\beta_{z_\tau} . \end{equation}

This parameter was found to be always below $0.015 Re_\tau$ at all locations reported here and in figures 12–14. This is well below the range of the approximate value of $\varPi > 0.03 Re_\tau$ suggested by Lozano-Durán et al. (Reference Lozano-Durán, Giometto, Park and Moin2020) as the regime in which the effect of spanwise pressure gradients becomes significant. As a result, it is unlikely for this mechanism to have a significant impact on the attenuation of turbulence and the near-wall peak of the Reynolds stress profile. It should be noted that the recommended criterion was derived for higher $Re_\tau$, in the absence of adverse pressure gradients, and for an initially fully developed channel flow. Whether a violation of these conditions leads to significant departures from the proposed criterion is not entirely clear and merits further investigation.

It is important to acknowledge certain observed departures in figures 12–14 from the trends described above. Firstly, RWT-0 exhibits a profile almost identical to P-0 near the root at $x'/c=0.6$ (figure 12a), yet later evolves to display lower levels of fluctuations for $y_{BL}^+ \geq 30$ at downstream locations as early as $x'/c=0.7$, despite having the same boundary layer thickness. While this is most likely an artefact caused by differences in the trip or different response of the boundary layers to the trip (e.g. a history effect), further investigations are needed to ascertain whether this might be a consequence of additional unidentified mechanisms. Another noticeable departure is found in the case of RWT-10, particularly at $x'/c=0.8$ and $0.9$ (figure 14k,l,o, p), which do not display a decrease in the near-wall peak. Possible explanations for this behaviour could include the larger variation of $\beta _{x_\tau }$ at those locations, the reduced deflection angle at $x'/c=0.8$ and $0.9$ (see figure 5c) and the stronger 3-D effects in RWT-10.

Before concluding this section, we should emphasise that the reference profiles chosen for the comparisons in this section were specifically selected to eliminate as many causes of difference between the finite- and infinite-span profiles as possible. This ensures that the observed differences primarily arise from a few key mechanisms. However, this selection also results in smaller differences between the compared profiles, which may not be fully representative of the real discrepancy. This point is partially illustrated in figure 10(b), which shows a larger difference in ${\mathsf{R}}_{11}$ profiles when only the streamwise locations are matched. It is worth noting that, at the spanwise location selected for this figure, RWT-5 exhibits a reduced effective angle of attack of approximately $1^\circ$. Given that a decreased effective angle of attack leads to thinner boundary layers and a higher outer-scaled near-wall peak in ${\mathsf{R}}_{11}$, the actual disparity between the finite- and infinite-span wings is likely greater than what is depicted in the figure.