Hostname: page-component-78c5997874-lj6df Total loading time: 0 Render date: 2024-11-17T13:20:04.505Z Has data issue: false hasContentIssue false
  • English
  • Français

Ice formation within a thin film flowing over a flat plate

Published online by Cambridge University Press:  22 March 2017

Madeleine Rose Moore Open the ORCID record for Madeleine Rose Moore [Opens in a new window] ,
M. S. Mughal  and
D. T. Papageorgiou
Madeleine Rose Moore*
Affiliation:
Department of Mathematics, Imperial College London, South Kensington Campus, London SW7 2AZ, UK Mathematical Institute, University of Oxford, Radcliffe Observatory Quarter, Woodstock Road, Oxford OX2 6GG, UK
M. S. Mughal
Affiliation:
Department of Mathematics, Imperial College London, South Kensington Campus, London SW7 2AZ, UK
D. T. Papageorgiou
Affiliation:
Department of Mathematics, Imperial College London, South Kensington Campus, London SW7 2AZ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We present a model for ice formation in a thin, viscous liquid film driven by a Blasius boundary layer after heating is switched off along part of the flat plate. The flow is assumed to initially be in the Nelson et al. (J. Fluid Mech., vol. 284, 1995, pp. 159–169) steady-state configuration with a constant flux of liquid supplied at the tip of the plate, so that the film thickness grows like 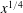$x^{1/4}$ in distance along the plate. Plate cooling is applied downstream of a point, 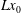$Lx_{0}$, an 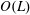$O(L)$-distance from the tip of the plate, where 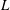$L$ is much larger than the film thickness. The cooling is assumed to be slow enough that the flow is quasi-steady. We present a thorough asymptotic derivation of the governing equations from the incompressible Navier–Stokes equations in each fluid and the corresponding Stefan problem for ice growth. The problem breaks down into two temporal regimes corresponding to the relative size of the temperature difference across the ice, which are analysed in detail asymptotically and numerically. In each regime, two distinct spatial regions arise, an outer region of the length scale of the plate, and an inner region close to $x_{0}$ in which the film and air are driven over the growing ice layer. Moreover, in the early time regime, there is an additional intermediate region in which the air–water interface propagates a slope discontinuity downstream due to the sudden onset of the ice at the switch-off point. For each regime, we present ice profiles and growth rates, and show that for large times, the film is predicted to rupture in the outer region when the slope discontinuity becomes sufficiently enhanced.

JFM classification

Aerodynamics: Aerodynamics Boundary Layers: Boundary Layers Phase change: Icing
Type
Papers
Information
Journal of Fluid Mechanics , Volume 817 , 25 April 2017 , pp. 455 - 489
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Article last updated 07 March 2023

References

Biddle, J. W., Holten, V., Sengers, J. V. & Anisimov, M. A. 2013 Thermal conductivity of supercooled water. Phys. Rev. E 87 (4), 042302.Google Scholar
Dehaoui, A., Issenmann, B. & Caupin, F. 2015 Viscosity of deeply supercooled water and its coupling to molecular diffusion. Proc. Natl Acad. Sci. USA 112 (39), 12020–12025.Google Scholar
Elliott, J. W. & Smith, F. T. 2017 Ice formation on a smooth or rough cold surface due to the impact of a supercooled water droplet. J. Engng Maths 102 (1), 35–64.10.1007/s10665-015-9784-zGoogle Scholar
Gent, R. W., Dart, N. P. & Cansdale, J. T. 2000 Aircraft icing. Phil. Trans. R. Soc. Lond. A 358 (1776), 2873–2911.Google Scholar
Hansman, R. J., Yamaguchi, K., Berkowitz, B. & Potapczuk, M. 1991 Modeling of surface roughness effects on glaze ice accretion. J. Thermophys. Heat Transfer 5 (1), 54–60.10.2514/3.226Google Scholar
Hare, D. E. & Sorensen, C. M. 1987 The density of supercooled water. ii. Bulk samples cooled to the homogeneous nucleation limit. J. Chem. Phys. 87 (8), 4840–4845.Google Scholar
Higuera, F. J. 1991 Viscous-inviscid interaction due to the freezing of a liquid flowing on a flat plate. Phys. Fluids A 3 (12), 2875–2886.Google Scholar
Holten, V., Bertrand, C. E., Anisimov, M. A. & Sengers, J. V. 2012 Thermodynamics of supercooled water. J. Chem. Phys. 136 (9), 094507.10.1063/1.3690497Google Scholar
Hrubý, J., Vinš, V., Mareš, R., Hykl, J. & Kalová, J. 2014 Surface tension of supercooled water: no inflection point down to - 25 °C. J. Phys. Chem. Lett. 5 (3), 425–428.Google Scholar
Jung, S., Tiwari, M. K., Doan, N. V. & Poulikakos, D. 2012 Mechanism of supercooled droplet freezing on surfaces. Nat. Commun. 3, 615.Google Scholar
Lighthill, M. J. 1950 Contributions to the theory of heat transfer through a laminar boundary layer. Proc. R. Soc. Lond. A 202, 359–377.Google Scholar
Lynch, F. T. & Khodadoust, A. 2001 Effects of ice accretions on aircraft aerodynamics. Prog. Aerosp. Sci. 37 (8), 669–767.Google Scholar
Messinger, B. L. 1953 Equilibrium temperature of an unheated icing surface as a function of air speed. J. Aero. Sci. 20, 29–42.Google Scholar
Messiter, A. F. 1970 Boundary-layer interaction theory. Trans. ASME J. Appl. Maths 18, 241–257.Google Scholar
Mitchell, S. L. & Myers, T. G. 2008 Approximate solution methods for one-dimensional solidification from an incoming fluid. Appl. Maths Comput. 202, 311–326.Google Scholar
Mitchell, S. L. & Myers, T. G. 2012 Application of heat balance integral methods to one-dimensional phase change problems. Intl. J. Differ. Equ. 2012, 187902.Google Scholar
Myers, T. G. 2001 Extension to the Messinger model for aircraft icing. AIAA J. 39 (2), 211–218.Google Scholar
Myers, T. G. & Charpin, J. P. F. 2004 A mathematical model for atmospheric ice accretion and water flow on a cold surface. Intl J. Heat Mass Transfer 47 (25), 5483–5500.Google Scholar
Myers, T. G., Charpin, J. P. F. & Chapman, S. J. 2002a The flow and solidification of a thin fluid film on an arbitrary three-dimensional surface. Phys. Fluids 14 (8), 2788–2803.Google Scholar
Myers, T. G., Charpin, J. P. F. & Thompson, C. P. 2002b Slowly accreting ice due to supercooled water impacting on a cold surface. Phys. Fluids 14 (1), 240–256.Google Scholar
Myers, T. G. & Hammond, D. W. 1999 Ice and water film growth from incoming supercooled droplets. Intl J. Heat Mass Transfer 42 (12), 2233–2242.Google Scholar
Neiland, V. Y. 1969 Towards a theory of separation of the laminar boundary layer in a supersonic stream. Izv. Akad. Nauk SSSR Mekh. Zhidk. Gaza 4, 33–35.Google Scholar
Nelson, J. J., Alving, A. E. & Joseph, D. D. 1995 Boundary layer flow of air over water on a flat plate. J. Fluid Mech. 284, 159–169.Google Scholar
Olsen, W. & Walker, E.1987 Experimental evidence for modifying the current physical model for ice accretion on aircraft surfaces. NASA TM 87184.Google Scholar
Otta, S. P. & Rothmayer, A. P. 2007 A simple boundary-layer water film model for aircraft icing. In 45th AIAA Aerospace Sciences Meeting. AIAA.Google Scholar
Otta, S. P. & Rothmayer, A. P. 2009 Instability of stagnation line icing. Comput. Fluids 38, 273–283.Google Scholar
Pelekasis, N. A. & Tsamopoulos, J. A. 2001 Linear stability of a gas boundary layer flowing past a thin liquid film over a flat plate. J. Fluid Mech. 436, 321–352.Google Scholar
Quero, M., Hammond, D. W., Purvis, R. & Smith, F. T. 2006 Analysis of super-cooled water droplet impact on a thin water layer and ice growth. In 44th AIAA Aerospace Sciences Meeting. AIAA.Google Scholar
Rothmayer, A. P. 2003a On the creation of ice surface roughness by interfacial instabilities. In 41st AIAA Aerospace Sciences Meeting. AIAA.Google Scholar
Rothmayer, A. P. 2003b Scaling laws for water and ice layers on airfoils. In 41st AIAA Aerospace Sciences Meeting. AIAA.Google Scholar
Rothmayer, A. P. 2006 Stagnation point icing. In 44th AIAA Aerospace Sciences Meeting. AIAA.Google Scholar
Rothmayer, A. P. & Tsao, J. C. 2000 Water film runback on an airfoil surface. In 38th AIAA Aerospace Sciences Meeting. AIAA.Google Scholar
Shapiro, E. & Timoshin, S. 2006 Linear stability of ice growth under a gravity-driven water film. Phys. Fluids 18 (7), 074106.Google Scholar
Shapiro, E. & Timoshin, S. 2007 On ice-induced instability in free-surface flows. J. Fluid Mech. 577, 25–52.Google Scholar
Smith, F. T. 1973 Laminar flow over a small hump on a flat plate. J. Fluid Mech. 57 (04), 803–824.Google Scholar
Smyrnaios, D. N., Pelekasis, N. A. & Tsamopoulos, J. A. 2000 Boundary layer flow of air past solid surfaces in the presence of rainfall. J. Fluid Mech. 425, 79–110.Google Scholar
Stewartson, K. & Williams, P. G. 1969 Self-induced separation. Proc. R. Soc. Lond. A 312, 181–206.Google Scholar
Sweby, P. K. 1984 High resolution schemes using flux limiters for hyperbolic conservation laws. SIAM J. Numer. Anal. 21 (5), 995–1011.Google Scholar
Thomas, S. K., Cassoni, R. P. & MacArthur, C. D. 1996 Aircraft anti-icing and de-icing techniques and modeling. J. Aircraft 33 (5), 841–854.Google Scholar
Timoshin, S. N. 1997 Instabilities in a high-Reynolds-number boundary layer on a film-coated surface. J. Fluid Mech. 353 (163), 163–195.Google Scholar
Tsao, J. C. & Rothmayer, A. P. 2002 Application of triple-deck theory to the prediction of glaze ice roughness formation on an airfoil leading edge. Comput. Fluids 31 (8), 977–1014.Google Scholar
Tsao, J.-C., Rothmayer, A. P. & Ruban, A. I. 1997 Stability of air flow past thin liquid films on airfoils. Comput. Fluids 26 (5), 427–452.10.1016/S0045-7930(97)00005-4Google Scholar
Ueno, K. & Farzaneh, M. 2011 Linear stability analysis of ice growth under supercooled water film driven by a laminar airflow. Phys. Fluids 23 (4), 042103.Google Scholar
Vargas, M. 2007 Current experimental basis for modeling ice accretions on swept wings. J. Aircraft 44 (1), 274–290.Google Scholar