Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-26T16:43:30.375Z Has data issue: false hasContentIssue false
  • English
  • Français

Hydrodynamics of swimming in stingrays: numerical simulations and the role of the leading-edge vortex

Published online by Cambridge University Press:  05 January 2016

R. G. Bottom II ,
I. Borazjani ,
E. L. Blevins  and
G. V. Lauder
R. G. Bottom II
Affiliation:
Department of Mechanical and Aerospace Engineering, University at Buffalo, State University of New York, Buffalo, NY 14260, USA
I. Borazjani*
Affiliation:
Department of Mechanical and Aerospace Engineering, University at Buffalo, State University of New York, Buffalo, NY 14260, USA
E. L. Blevins
Affiliation:
Department of Organismic and Evolutionary Biology, Harvard University, Cambridge, MA 02138, USA
G. V. Lauder
Affiliation:
Department of Organismic and Evolutionary Biology, Harvard University, Cambridge, MA 02138, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Stingrays, in contrast with many other aquatic animals, have flattened disk-shaped bodies with expanded pectoral ‘wings’, which are used for locomotion in water. To discover the key features of stingray locomotion, large-eddy simulations of a self-propelled stingray, modelled closely after the freshwater stingray, Potamotrygon orbignyi, are performed. The stingray’s body motion was prescribed based on three-dimensional experimental measurement of wing and body kinematics in live stingrays at two different swimming speeds of 1.5 and 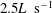$2.5L~\text{s}^{-1}$ (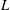$L$ is the disk length of the stingray). The swimming speeds predicted by the self-propelled simulations were within 12 % of the nominal swimming speeds in the experiments. It was found that the fast-swimming stingray (Reynolds number 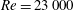$Re=23\,000$ and Strouhal number 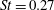$St=0.27$) is approximately 12 % more efficient than the slow-swimming one (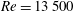$Re=13\,500$, $St=0.34$). This is related to the wake of the fast- and slow-swimming stingrays, which was visualized along with the pressure on the stingray’s body. A horseshoe vortex was discovered to be present at the anterior margin of the stingray, creating a low-pressure region that enhances thrust for both fast and slow swimming speeds. Furthermore, it was found that a leading-edge vortex (LEV) on the pectoral disk of swimming stingrays generates a low-pressure region in the fast-swimming stingray, whereas the low- and high-pressure regions in the slow-swimming one are in the back half of the wing and not close to any vortical structures. The undulatory motion creates thrust by accelerating the adjacent fluid (the added-mass mechanism), which is maximized in the back of the wing because of higher undulations and velocities in the back. However, the thrust enhancement by the LEV occurs in the front portion of the wing. By computing the forces on the front half and the back half of the wing, it was found that the contribution of the back half of the wing to thrust in a slow-swimming stingray is several-fold higher than in the fast-swimming one. This indicates that the LEV enhances thrust in fast-swimming stingrays and improves the efficiency of swimming.

JFM classification

Biological Fluid Dynamics: Biological Fluid Dynamics Biological Fluid Dynamics: Propulsion Biological Fluid Dynamics: Swimming/flying
Type
Papers
Information
Journal of Fluid Mechanics , Volume 788 , 10 February 2016 , pp. 407 - 443
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alben, S., Witt, C., Baker, T. V., Anderson, E. & Lauder, G. V. 2012 Dynamics of freely swimming flexible foils. Phys. Fluids 24 (5), 051901.CrossRefGoogle Scholar
Anderson, J. M., Streitlien, K. & Barrett, D. S. 1998 Oscillating foils of high propulsive efficiency. J. Fluid Mech. 360, 41–72.Google Scholar
Aono, H., Liang, F. & Liu, H. 2008 Near- and far-field aerodynamics in insect hovering flight: an integrated computational study. J. Expl Biol. 211 (2), 239–257.CrossRefGoogle ScholarPubMed
Balay, S., Buschelman, K., Gropp, W. D., Kaushik, D., Knepley, M. G., McInnes, L. C., Smith, B. F. & Zhang, H.2004 PETSc webpage, 2001; http://www.mcs.anl.gov/petsc.Google Scholar
Barrett, D. S., Triantafyllou, M. S. & Yue, D. K. P. 1999 Drag reduction in fish-like locomotion. J. Fluid Mech. 392, 183–212.CrossRefGoogle Scholar
Beaudan, P. & Moin, P.1994 Numerical experiments on the flow past a circular cylinder at sub-critical Reynolds number. Tech. Rep. ADA289937. DTIC Document.Google Scholar
Beddhu, M., Taylor, L. K. & Whitfield, D. L. 1996 Strong conservative form of the incompressible Navier–Stokes equations in a rotating frame with a solution procedure. J. Comput. Phys. 128 (2), 427–437.CrossRefGoogle Scholar
Beem, H. R., Rival, D. E. & Triantafyllou, M. S. 2012 On the stabilization of leading-edge vortices with spanwise flow. Exp. Fluids 52 (2), 511–517.CrossRefGoogle Scholar
Birch, J. M. & Dickinson, M. H. 2001 Spanwise flow and the attachment of the leading-edge vortex on insect wings. Nature 412 (6848), 729–733.Google Scholar
Birch, J. M. & Dickinson, M. H. 2003 The influence of wing–wake interactions on the production of aerodynamic forces in flapping flight. J. Expl Biol. 206 (13), 2257–2272.CrossRefGoogle ScholarPubMed
Birch, J. M., Dickson, W. B. & Dickinson, M. H. 2004 Force production and flow structure of the leading edge vortex on flapping wings at high and low Reynolds numbers. J. Expl Biol. 207 (7), 1063–1072.CrossRefGoogle Scholar
Blevins, E. L. & Lauder, G. V. 2012 Rajiform locomotion: three-dimensional kinematics of the pectoral fin surface during swimming in the freshwater stingray Potamotrygon orbignyi . J. Expl Biol. 215 (18), 3231–3241.Google Scholar
Bomphrey, R. J., Taylor, G. K. & Thomas, A. L. R. 2010 Smoke visualization of free-flying bumblebees indicates independent leading-edge vortices on each wing pair. In Animal Locomotion (ed. Taylor, G. K., Triantafyllou, M. S. & Tropea, C.), pp. 249–259. Springer.Google Scholar
Borazjani, I. 2013a Fluid–structure interaction, immersed boundary-finite element method simulations of bio-prosthetic heart valves. Comput. Meth. Appl. Mech. Engng 257, 103–116.CrossRefGoogle Scholar
Borazjani, I. 2013b The functional role of caudal and anal/dorsal fins during the C-start of a bluegill sunfish. J. Expl Biol. 216 (9), 1658–1669.Google ScholarPubMed
Borazjani, I. 2015 Simulations of unsteady aquatic locomotion: from unsteadiness in straight-line swimming to fast-starts. Integr. Compar. Biol. 55 (4), 740–752.CrossRefGoogle ScholarPubMed
Borazjani, I. & Daghooghi, M. 2013 The fish tail motion forms an attached leading edge vortex. Proc. R. Soc. Lond. B 280 (1756), 2012–2071.Google ScholarPubMed
Borazjani, I., Ge, L., Le, T. & Sotiropoulos, F. 2013 A parallel overset–curvilinear–immersed boundary framework for simulating complex 3D incompressible flows. Comput. Fluids 77, 76–96.CrossRefGoogle ScholarPubMed
Borazjani, I., Ge, L. & Sotiropoulos, F. 2008 Curvilinear immersed boundary method for simulating fluid structure interaction with complex 3D rigid bodies. J. Comput. Phys. 227 (16), 7587–7620.Google Scholar
Borazjani, I. & Sotiropoulos, F. 2008 Numerical investigation of the hydrodynamics of carangiform swimming in the transitional and inertial flow regimes. J. Expl Biol. 211 (10), 1541–1558.Google Scholar
Borazjani, I. & Sotiropoulos, F. 2009 Numerical investigation of the hydrodynamics of anguilliform swimming in the transitional and inertial flow regimes. J. Expl Biol. 212 (4), 576–592.CrossRefGoogle ScholarPubMed
Borazjani, I. & Sotiropoulos, F. 2010 On the role of form and kinematics on the hydrodynamics of self-propelled body/caudal fin swimming. J. Expl Biol. 213 (1), 89–107.CrossRefGoogle ScholarPubMed
Borazjani, I., Sotiropoulos, F., Tytell, E. D. & Lauder, G. V. 2012 Hydrodynamics of the bluegill sunfish C-start escape response: three-dimensional simulations and comparison with experimental data. J. Expl Biol. 215 (4), 671–684.CrossRefGoogle ScholarPubMed
Breder, C. M. 1926 The locomotion of fishes. Zoologica 4, 159–256.Google Scholar
Breuer, M. 1998 Large eddy simulation of the subcritical flow past a circular cylinder: numerical and modeling aspects. Intl J. Numer. Meth. Fluids 28 (9), 1281–1302.3.0.CO;2-#>CrossRefGoogle Scholar
Chang, Y.-H., Ting, S.-C., Su, J.-Y., Soong, C.-Y. & Yang, J.-T. 2013 Ventral-clap modes of hovering passerines. Phys. Rev. E 87 (2), 022707.CrossRefGoogle ScholarPubMed
Cheng, B., Roll, J., Liu, Y., Troolin, D. R. & Deng, X. 2014 Three-dimensional vortex wake structure of flapping wings in hovering flight. J. R. Soc. Interface 11 (91), 20130984.Google Scholar
Chopra, M. G. 1976 Large amplitude lunate-tail theory of fish locomotion. J. Fluid Mech. 74, 161.CrossRefGoogle Scholar
Clark, R. P. & Smits, A. J. 2006 Thrust production and wake structure of a batoid-inspired oscillating fin. J. Fluid Mech. 562 (1), 415–429.CrossRefGoogle ScholarPubMed
Daghooghi, M. & Borazjani, I. 2015 The hydrodynamic advantages of synchronized swimming in a rectangular pattern. Bioinspir. Biomim. 10 (5), 056018.CrossRefGoogle Scholar
Dewey, P. A., Carriou, A. & Smits, A. J. 2012 On the relationship between efficiency and wake structure of a batoid-inspired oscillating fin. J. Fluid Mech. 691 (1), 245–266.Google Scholar
Dickinson, M. H. & Gotz, K. G. 1993 Unsteady aerodynamic performance of model wings at low Reynolds numbers. J. Expl Biol. 174 (1), 45–64.Google Scholar
Dong, H., Bozkurttas, M., Mittal, R., Madden, P. & Lauder, G. V. 2010 Computational modelling and analysis of the hydrodynamics of a highly deformable fish pectoral fin. J. Fluid Mech. 645, 345–373.CrossRefGoogle Scholar
Dong, H., Mittal, R. & Najjar, F. M. 2006 Wake topology and hydrodynamic performance of low-aspect-ratio flapping foils. J. Fluid Mech. 566, 309–344.Google Scholar
Du, G. & Sun, M. 2010 Effects of wing deformation on aerodynamic forces in hovering hoverflies. J. Expl Biol. 213 (13), 2273–2283.Google Scholar
Dumitrescu, H. & Cardos, V. 2012 Inboard stall delay due to rotation. J. Aircraft 49 (1), 101–107.CrossRefGoogle Scholar
Earnshaw, P. B. I. 1962 An Experimental Investigation of the Structure of a Leading-Edge Vortex. HM Stationery Office.Google Scholar
Eberle, A. L., Reinhall, P. G. & Daniel, T. L. 2014 Fluid–structure interaction in compliant insect wings. Bioinspir. Biomim. 9 (2), 025005.Google Scholar
Ellington, C. P., Van Den Berg, C., Willmott, A. P. & Thomas, A. L. R. 1996 Leading-edge vortices in insect flight. Nature 384, 626–630.CrossRefGoogle Scholar
Fish, F. E., Haj-Hariri, H., Smits, A. J., Bart-Smith, H. & Iwasaki, T. 2012 Biomimetic swimmer inspired by the manta ray. In Biomimetics: Nature-Based Innovation (ed. Bar-Cohen, Y.), chapter 17, pp. 495–523. CRC.Google Scholar
Fish, F. E. & Lauder, G. V. 2006 Passive and active flow control by swimming fishes and mammals. Annu. Rev. Fluid Mech. 38 (1), 193–224.Google Scholar
Gad-el Hak, M. & Blackwelder, R. F. 1985 The discrete vortices from a delta wing. AIAA J. 23 (6), 961–962.Google Scholar
Ge, L. & Sotiropoulos, F. 2007 A numerical method for solving the 3D unsteady incompressible Navier–Stokes equations in curvilinear domains with complex immersed boundaries. J. Comput. Phys. 225 (2), 1782–1809.CrossRefGoogle ScholarPubMed
Germano, M., Piomelli, U., Moin, P. & Cabot, W. H. 1991 A dynamic subgrid-scale eddy viscosity model. Phys. Fluids A 3 (7), 1760–1765.Google Scholar
Gilmanov, A. & Sotiropoulos, F. 2005 A hybrid Cartesian/immersed boundary method for simulating flows with 3D, geometrically complex, moving bodies. J. Comput. Phys. 207 (2), 457–492.Google Scholar
Gray, J. 1933 Studies in animal locomotion. I. The movement of fish with special reference to the eel. J. Expl Biol. 10 (1), 88–104.CrossRefGoogle Scholar
Harbig, R. R., Sheridan, J. & Thompson, M. C. 2013 Reynolds number and aspect ratio effects on the leading-edge vortex for rotating insect wing planforms. J. Fluid Mech. 717, 166–192.Google Scholar
Jordan, S. A. 1999 A large-eddy simulation methodology in generalized curvilinear coordinates. J. Comput. Phys. 148 (2), 322–340.Google Scholar
Kang, S., Borazjani, I., Colby, J. A. & Sotiropoulos, F. 2012 Numerical simulation of 3D flow past a real-life marine hydrokinetic turbine. Adv. Water Resour. 39, 33–43.Google Scholar
Kern, S. & Koumoutsakos, P. 2006 Simulations of optimized anguilliform swimming. J. Expl Biol. 209 (24), 4841–4857.Google Scholar
Khosronejad, A., Kang, S., Borazjani, I. & Sotiropoulos, F. 2011 Curvilinear immersed boundary method for simulating coupled flow and bed morphodynamic interactions due to sediment transport phenomena. Adv. Water Resour. 34 (7), 829–843.CrossRefGoogle Scholar
Kim, D. & Choi, H. 2006 Immersed boundary method for flow around an arbitrarily moving body. J. Comput. Phys. 212 (2), 662.Google Scholar
Kim, D. & Gharib, M. 2010 Experimental study of three-dimensional vortex structures in translating and rotating plates. Exp. Fluids 49 (1), 329–339.Google Scholar
Koochesfahani, M. M. 1989 Vortical patterns in the wake of an oscillating airfoil. AIAA J. 27 (9), 1200–1205.CrossRefGoogle Scholar
Kravchenko, A. G. & Moin, P. 2000 Numerical studies of flow over a circular cylinder at $Re_{D}=3900$ . Phys. Fluids 12 (2), 403–417.Google Scholar
Kuethe, A. M. & Schetzer, J. D. 1950 Foundation of Aerodynamics. John Wiley & Sons.Google Scholar
Lee, H. M. & Wu, Y. 2013 An experimental study of stall delay on the blade of a horizontal-axis wind turbine using tomographic particle image velocimetry. J. Wind Engng Ind. Aerodyn. 123, 56–68.Google Scholar
Lentink, D. & Dickinson, M. H. 2009a Biofluiddynamic scaling of flapping, spinning and translating fins and wings. J. Expl Biol. 212 (16), 2691–2704.Google Scholar
Lentink, D. & Dickinson, M. H. 2009b Rotational accelerations stabilize leading edge vortices on revolving fly wings. J. Expl Biol. 212 (16), 2705–2719.Google Scholar
Lentink, D., Dickson, W. B., Van Leeuwen, J. L. & Dickinson, M. H. 2009 Leading-edge vortices elevate lift of autorotating plant seeds. Science 324 (5933), 1438–1440.Google Scholar
Lewin, G. C. & Haj-Hariri, H. 2003 Modelling thrust generation of a two-dimensional heaving airfoil in a viscous flow. J. Fluid Mech. 492, 339–362.CrossRefGoogle Scholar
Lighthill, M. J. 1960 Note on swimming of slender fish. J. Fluid Mech. 9 (2), 305.Google Scholar
Lighthill, M. J. 1969 Hydromechanics of aquatic animal propulsion. Annu Rev. Fluid Mech. 1 (1), 413–446.Google Scholar
Lighthill, M. J. 1971 Large-amplitude elongated-body theory of fish locomotion. Proc. R. Soc. Lond. B 179 (1055), 125.Google Scholar
Liu, H. 2005 Simulation-based biological fluid dynamics in animal locomotion. Appl. Mech. Rev. 58 (1–6), 269.CrossRefGoogle Scholar
Liu, H. & Kawachi, K. 1999 A numerical study of undulatory swimming. J. Comput. Phys. 155 (2), 223.CrossRefGoogle Scholar
Maxworthy, T. 1981 The fluid dynamics of insect flight. Annu. Rev. Fluid Mech. 13 (1), 329–350.Google Scholar
Mittal, R., Dong, H., Bozkurttas, M., Lauder, G. V. & Madden, P. 2006 Locomotion with flexible propulsors: II. Computational modeling of pectoral fin swimming in sunfish. Bioinspir. Biomim. 1 (4), S35.CrossRefGoogle ScholarPubMed
Mittal, R. & Iaccarino, G. 2005 Immersed boundary methods. Annu. Rev. Fluid Mech. 37, 239–261.Google Scholar
Mittal, R. & Moin, P. 1997 Suitability of upwind-biased finite difference schemes for large-eddy simulation of turbulent flows. AIAA J. 35 (8), 1415–1417.Google Scholar
Moored, K. W., Dewey, P. A., Smits, A. J. & Haj-Hariri, H. 2012 Hydrodynamic wake resonance as an underlying principle of efficient unsteady propulsion. J. Fluid Mech. 708, 329.Google Scholar
Mountcastle, A. M. & Combes, S. A. 2013 Wing flexibility enhances load-lifting capacity in bumblebees. Proc. R. Soc. Lond. B 280 (1759), 20130531.Google Scholar
Muijres, F. T., Johansson, L. C., Barfield, R., Wolf, M., Spedding, G. R. & Hedenström, A. 2008 Leading-edge vortex improves lift in slow-flying bats. Science 319 (5867), 1250–1253.Google Scholar
Muijres, F. T., Johansson, L. C., Winter, Y. & Hedenström, A. 2014 Leading edge vortices in lesser long-nosed bats occurring at slow but not fast flight speeds. Bioinspir. Biomim. 9 (2), 025006.Google Scholar
Muller, U. K., Smit, J., Stamhuis, E. J. & Videler, J. J. 2001 How the body contributes to the wake in undulatory fish swimming: flow fields of a swimming eel (Anguilla anguilla). J. Expl Biol. 204 (16), 2751–2762.CrossRefGoogle Scholar
Muller, U. K., Stamhuis, E. J. & Videler, J. J. 2000 Hydrodynamics of unsteady fish swimming and the effects of body size: comparing the flow fields of fish larvae and adults. J. Expl Biol. 203 (2), 193–206.Google Scholar
Nakata, T. & Liu, H. 2012 A fluid–structure interaction model of insect flight with flexible wings. J. Comput. Phys. 231 (4), 1822–1847.Google Scholar
Nauen, J. C. & Lauder, G. V. 2001 Locomotion in scombrid fishes: visualization of flow around the caudal peduncle and finlets of the chub mackerel Scomber japonicus . J. Expl Biol. 204 (13), 2251–2263.CrossRefGoogle ScholarPubMed
Newman, J. N. & Wu, T. Y. 1975 Hydromechanical aspects of fish swimming. In Swimming and Flying in Nature, pp. 615–634. Springer.Google Scholar
Norberg, C.1987. Effects of Reynolds number and a low-intensity freestream turbulence on the flow around a circular cylinder. Technolological Publications, Chalmers University, Goteborg, Sweden, vol. 87, no. 2.Google Scholar
Ong, L. & Wallace, J. 1996 The velocity field of the turbulent very near wake of a circular cylinder. Exp. Fluids 20 (6), 441–453.Google Scholar
Ozen, C. A. & Rockwell, D. 2012 Three-dimensional vortex structure on a rotating wing. J. Fluid Mech. 707, 541–550.Google Scholar
Pederzani, J. & Haj-Hariri, H. 2006 A numerical method for the analysis of flexible bodies in unsteady viscous flows. Intl J. Numer. Meth. Engng 68 (10), 1096–1112.Google Scholar
Peskin, C. S. 1972 Flow patterns around heart valves: a numerical method. J. Comput. Phys. 10, 252–271.Google Scholar
Peskin, C. S. 1977 Numerical analysis of blood flow in the heart. J. Comput. Phys. 25, 220.Google Scholar
Peskin, C. S. & McQueen, D. M. 1989 A three-dimensional computational method for blood flow in the heart. 1. Immersed elastic fibers in a viscous incompressible fluid. J. Comput. Phys. 81 (2), 372–405.Google Scholar
Pitt Ford, C. W. & Babinsky, H. 2013 Lift and the leading-edge vortex. J. Fluid Mech. 720, 280–313.Google Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.Google Scholar
Quinn, D. B., Lauder, G. V. & Smits, A. J. 2014 Scaling the propulsive performance of heaving flexible panels. J. Fluid Mech. 738, 250–267.Google Scholar
Rosenberger, L. J. 2001 Pectoral fin locomotion in batoid fishes: undulation versus oscillation. J. Expl Biol. 204 (2), 379–394.Google Scholar
Sane, S. P. 2003 The aerodynamics of insect flight. J. Expl Biol. 206 (23), 4191–4208.Google Scholar
Shen, L., Zhang, X., Yue, D. K. P. & Triantafyllou, M. S. 2003 Turbulent flow over a flexible wall undergoing a streamwise travelling wave motion. J. Fluid Mech. 484, 197–221.Google Scholar
Sicot, C., Devinant, P., Loyer, S. & Hureau, J. 2008 Rotational and turbulence effects on a wind turbine blade. Investigation of the stall mechanisms. J. Wind Engng Ind. Aerodyn. 96 (8), 1320–1331.CrossRefGoogle Scholar
Smagorinsky, J. 1963 General circulation experiments with the primitive equations: I. The basic experiment. Mon. Weath. Rev. 91 (3), 99–164.Google Scholar
Taneda, S. & Tomonari, Y. 1974 An experiment on the flow around a waving plate. J. Phys. Soc. Japan 36 (6), 1683–1689.Google Scholar
Tangler, J. L. 2004 Insight into wind turbine stall and post-stall aerodynamics. Wind Energy 7 (3), 247–260.Google Scholar
Thielicke, W. & Stamhuis, E. J. 2015 The influence of wing morphology on the three-dimensional flow patterns of a flapping wing at bird scale. J. Fluid Mech. 768, 240–260.Google Scholar
Triantafyllou, M. S., Techet, A. H. & Hover, F. S. 2004 Review of experimental work in biomimetic foils. IEEE J. Ocean. Engng 29 (3), 585–594.Google Scholar
Tytell, E. D. & Lauder, G. V. 2004 The hydrodynamics of eel swimming I: wake structure. J. Expl Biol. 207 (11), 1825–1841.Google Scholar
Usherwood, J. R. & Ellington, C. P. 2002 The aerodynamics of revolving wings I: model hawkmoth wings. J. Expl Biol. 205 (11), 1547–1564.CrossRefGoogle ScholarPubMed
Van Den Berg, C. & Ellington, C. P. 1997 The three-dimensional leading-edge vortex of a hovering model hawkmoth. Phil. Trans. R. Soc. Lond. B 352 (1351), 329–340.Google Scholar
Videler, J. J. & Hess, F. 1984 Fast continuous swimming of two pelagic predators, saithe (Pollachius virens) and mackerel (Scomber scombrus): a kinematic analysis. J. Expl Biol. 109 (1), 209–228.Google Scholar
Videler, J. J., Stamhuis, E. J. & Povel, G. D. E. 2004 Leading-edge vortex lifts swifts. Science 306 (5703), 1960–1962.Google Scholar
Wang, Z. J. 2000 Two dimensional mechanism for insect hovering. Phys. Rev. Lett. 85 (10), 2216.Google Scholar
Wang, Z. J. 2005 Dissecting insect flight. Annu. Rev. Fluid Mech. 37, 183–210.Google Scholar
Webb, P. W. 1984 Form and function in fish swimming. Sci. Am. 251 (1), 72–82.Google Scholar
Weis-Fogh, T. 1973 Quick estimates of flight fitness in hovering animals, including novel mechanisms for lift production. J. Expl Biol. 59 (1), 169–230.Google Scholar
Wojcik, C. J. & Buchholz, J. H. J. 2014 Vorticity transport in the leading-edge vortex on a rotating blade. J. Fluid Mech. 743, 249–261.Google Scholar
Wolfgang, M. J., Anderson, J. M., Grosenbaugh, M. A., Yue, D. K. & Triantafyllou, M. S. 1999 Near-body flow dynamics in swimming fish. J. Expl Biol. 202 (17), 2303–2327.Google Scholar
Wu, T. Y. 2011 Fish swimming and bird/insect flight. Annu. Rev. Fluid Mech. 43, 25–58.Google Scholar
Wu, T. Y.-T. 1960 Swimming of a waving plate. J. Fluid Mech. 10 (3), 321.Google Scholar
Wu, T. Y.-T. 1971 Hydromechanics of swimming propulsion. Part 1. Swimming of a two-dimensional flexible plate at variable forward speeds in an inviscid fluid. J. Fluid Mech. 46 (2), 337.Google Scholar
Zhao, L., Huang, Q., Deng, X. & Sane, S. P. 2010 Aerodynamic effects of flexibility in flapping wings. J. R. Soc. Interface 7 (44), 485–497.Google Scholar
Zhu, Q., Wolfgang, M. J., Yue, D. K. P. & Triantafyllou, M. S. 2002 Three-dimensional flow structures and vorticity control in fish-like swimming. J. Fluid Mech. 468, 1–28.Google Scholar

Bottom et al. supplementary movie

Stingray swimming at high speed (high Re)

Download Bottom et al. supplementary movie(Video)
Video 8.4 MB

Bottom et al. supplementary movie

Stingray swimming at low speed (low Re)

Download Bottom et al. supplementary movie(Video)
Video 3.7 MB