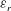1 Introduction
Fully developed Navier–Stokes turbulence at high Reynolds numbers is characterized by a large range of length scales, varying from the geometrical lengths of the flow over the so-called integral length scale
![]() $L$
, at which large velocity fluctuations occur on average, down to the Kolmogorov or dissipation scale
$L$
, at which large velocity fluctuations occur on average, down to the Kolmogorov or dissipation scale
![]() $\unicode[STIX]{x1D702}$
, at which kinetic energy is dissipated. While flow properties at the geometrical and integral length scales are influenced by boundary conditions, it is believed that fluctuations at the small scales are approximately isotropic in a statistical sense. In order to study these fluctuations, one focuses on an idealized flow, the so-called homogeneous isotropic turbulence, where all mean velocities are zero. This case may then be treated by methods of statistical physics, conditioned on strictly satisfying the Navier–Stokes equations. Statistics can be described by two-point equations for statistical moments of velocity fluctuations, which contain the mean dissipation
$\unicode[STIX]{x1D702}$
, at which kinetic energy is dissipated. While flow properties at the geometrical and integral length scales are influenced by boundary conditions, it is believed that fluctuations at the small scales are approximately isotropic in a statistical sense. In order to study these fluctuations, one focuses on an idealized flow, the so-called homogeneous isotropic turbulence, where all mean velocities are zero. This case may then be treated by methods of statistical physics, conditioned on strictly satisfying the Navier–Stokes equations. Statistics can be described by two-point equations for statistical moments of velocity fluctuations, which contain the mean dissipation
![]() $\langle \unicode[STIX]{x1D700}\rangle$
at the second order. The understanding is that there is a steady cascade of kinetic energy from the large scales to the small scales, with
$\langle \unicode[STIX]{x1D700}\rangle$
at the second order. The understanding is that there is a steady cascade of kinetic energy from the large scales to the small scales, with
![]() $\langle \unicode[STIX]{x1D700}\rangle$
being equal to the rate of energy transfer from the large scales to the small scales. Hence,
$\langle \unicode[STIX]{x1D700}\rangle$
being equal to the rate of energy transfer from the large scales to the small scales. Hence,
![]() $\langle \unicode[STIX]{x1D700}\rangle$
may be determined at the large scales, essentially at the integral scale
$\langle \unicode[STIX]{x1D700}\rangle$
may be determined at the large scales, essentially at the integral scale
![]() $L$
, and therefore is an external parameter imposed on the two-point equations describing small-scale turbulence.
$L$
, and therefore is an external parameter imposed on the two-point equations describing small-scale turbulence.
The basis of this theory was laid by two papers of Kolmogorov in 1941. In Kolmogorov (Reference Kolmogorov1941b
) he described velocity fluctuations separated by a distance
![]() $r$
as two-point velocity differences or velocity increments, the statistical moments of which are known as structure functions. He then introduced the dissipation scale
$r$
as two-point velocity differences or velocity increments, the statistical moments of which are known as structure functions. He then introduced the dissipation scale
![]() $\unicode[STIX]{x1D702}$
, which essentially subdivides the range of small-scale turbulence into two subranges, a dissipative subrange for small
$\unicode[STIX]{x1D702}$
, which essentially subdivides the range of small-scale turbulence into two subranges, a dissipative subrange for small
![]() $r$
, where both the viscosity
$r$
, where both the viscosity
![]() $\unicode[STIX]{x1D708}$
and the dissipation
$\unicode[STIX]{x1D708}$
and the dissipation
![]() $\langle \unicode[STIX]{x1D700}\rangle$
determine the solution of the second-order structure function equation, and an inertial range for large
$\langle \unicode[STIX]{x1D700}\rangle$
determine the solution of the second-order structure function equation, and an inertial range for large
![]() $r$
, where only
$r$
, where only
![]() $\langle \unicode[STIX]{x1D700}\rangle$
remains as a scaling parameter. In Kolmogorov (Reference Kolmogorov1941a
) he presented, based on the ‘known equation of von Kármán for the isotropic turbulence in the sense of Taylor’, an exact ordinary differential equation for the second-order structure function, in which the distance
$\langle \unicode[STIX]{x1D700}\rangle$
remains as a scaling parameter. In Kolmogorov (Reference Kolmogorov1941a
) he presented, based on the ‘known equation of von Kármán for the isotropic turbulence in the sense of Taylor’, an exact ordinary differential equation for the second-order structure function, in which the distance
![]() $r$
between the two points is the independent variable. Kolmogorov (Reference Kolmogorov1941a
) presented two separate asymptotic solutions, one for the viscous range, defined by the asymptotic limit
$r$
between the two points is the independent variable. Kolmogorov (Reference Kolmogorov1941a
) presented two separate asymptotic solutions, one for the viscous range, defined by the asymptotic limit
![]() $r\rightarrow 0$
, where the viscous term and the source term balance, and another one for the inertial range known as the
$r\rightarrow 0$
, where the viscous term and the source term balance, and another one for the inertial range known as the
![]() $4/5$
law, where the transport term balances the dissipation term. The inertial range is defined as the range of large
$4/5$
law, where the transport term balances the dissipation term. The inertial range is defined as the range of large
![]() $r$
where the
$r$
where the
![]() $4/5$
law is valid. The two papers Kolmogorov (Reference Kolmogorov1941a
,Reference Kolmogorov
b
) are known as K41 in the literature. These two results are of high importance, because they are both exact (under the assumptions of (local) isotropy, (local) homogeneity and very large Reynolds numbers) and were derived from the governing Navier–Stokes equations, i.e. they are solutions of the second-order structure function equations. One of the main aims of the present paper is to analyse the fourth-order structure function equations in this spirit with emphasis on their source terms. The notion is that solutions for the higher-order structure functions must be contained in the resulting system of equations.
$4/5$
law is valid. The two papers Kolmogorov (Reference Kolmogorov1941a
,Reference Kolmogorov
b
) are known as K41 in the literature. These two results are of high importance, because they are both exact (under the assumptions of (local) isotropy, (local) homogeneity and very large Reynolds numbers) and were derived from the governing Navier–Stokes equations, i.e. they are solutions of the second-order structure function equations. One of the main aims of the present paper is to analyse the fourth-order structure function equations in this spirit with emphasis on their source terms. The notion is that solutions for the higher-order structure functions must be contained in the resulting system of equations.
Kolmogorov (Reference Kolmogorov1941b
) had postulated that
![]() $\unicode[STIX]{x1D708}$
and
$\unicode[STIX]{x1D708}$
and
![]() $\langle \unicode[STIX]{x1D700}\rangle$
are the only scaling parameters for the entire distribution function of two-point velocity differences. Because only two quantities with different physical units are needed to non-dimensionalize the structure function equations, this was viewed as a claim for universality. However, Landau has argued that universality would be violated by variations of the dissipation at the large scales (cf. Landau & Lifshitz Reference Landau and Lifshitz1959; Frisch Reference Frisch1995). To address Landau’s criticism, Obukhov (Reference Obukhov1962) suggested the replacement of
$\langle \unicode[STIX]{x1D700}\rangle$
are the only scaling parameters for the entire distribution function of two-point velocity differences. Because only two quantities with different physical units are needed to non-dimensionalize the structure function equations, this was viewed as a claim for universality. However, Landau has argued that universality would be violated by variations of the dissipation at the large scales (cf. Landau & Lifshitz Reference Landau and Lifshitz1959; Frisch Reference Frisch1995). To address Landau’s criticism, Obukhov (Reference Obukhov1962) suggested the replacement of
![]() $\langle \unicode[STIX]{x1D700}\rangle$
by a local average
$\langle \unicode[STIX]{x1D700}\rangle$
by a local average
![]() $\unicode[STIX]{x1D700}_{r}$
over a volume of size
$\unicode[STIX]{x1D700}_{r}$
over a volume of size
![]() $r$
, where
$r$
, where
![]() $\unicode[STIX]{x1D700}_{r}$
is a fluctuating quantity, and assumed that the dissipation was log-normally distributed. Kolmogorov (Reference Kolmogorov1962) then quantified the variance of
$\unicode[STIX]{x1D700}_{r}$
is a fluctuating quantity, and assumed that the dissipation was log-normally distributed. Kolmogorov (Reference Kolmogorov1962) then quantified the variance of
![]() $\unicode[STIX]{x1D700}_{r}$
and used this concept to predict the inertial-range scaling exponents
$\unicode[STIX]{x1D700}_{r}$
and used this concept to predict the inertial-range scaling exponents
![]() $\unicode[STIX]{x1D701}_{n,0}$
defined by
$\unicode[STIX]{x1D701}_{n,0}$
defined by
where
![]() $\boldsymbol{u}$
is the velocity field and
$\boldsymbol{u}$
is the velocity field and
![]() $S_{n,0}$
is the longitudinal velocity structure function of order
$S_{n,0}$
is the longitudinal velocity structure function of order
![]() $n$
. While in K41 the inertial-range scaling exponents had been
$n$
. While in K41 the inertial-range scaling exponents had been
![]() $\unicode[STIX]{x1D701}_{n,0}=n/3$
, Kolmogorov (Reference Kolmogorov1962) predicted deviations from the K41 scaling, referred to as anomalous scaling in the literature, which are described by a single parameter, the intermittency coefficient
$\unicode[STIX]{x1D701}_{n,0}=n/3$
, Kolmogorov (Reference Kolmogorov1962) predicted deviations from the K41 scaling, referred to as anomalous scaling in the literature, which are described by a single parameter, the intermittency coefficient
![]() $\unicode[STIX]{x1D707}$
.
$\unicode[STIX]{x1D707}$
.
The two papers Kolmogorov (Reference Kolmogorov1962) and Obukhov (Reference Obukhov1962) are known as K62 in the literature, or as the refined similarity hypothesis (RSH). While K41 is based on the Navier–Stokes equations, the RSH remains a phenomenological model with no apparent connection to the Navier–Stokes equations. However, since dissipation fluctuations must be contained in the Navier–Stokes equations, the parameters describing them should be hidden somewhere in the equations for the higher-order structure functions or in additional equations related to them. More specifically, the moments of the dissipation distribution function should appear in averaged two-point equations derived from the Navier–Stokes equations. We will show in this paper that moments of specific forms of dissipation do indeed appear in additional equations to be derived. Hereafter, we will refer to these moments as dissipation parameters. Below, we find that the approach of using
![]() $\unicode[STIX]{x1D700}_{r}$
is consistent with the two-point theory of small-scale turbulence in the sense that
$\unicode[STIX]{x1D700}_{r}$
is consistent with the two-point theory of small-scale turbulence in the sense that
![]() $\unicode[STIX]{x1D700}_{r}$
appears in the system of the newly derived equations. However, the relation of the ansatz
$\unicode[STIX]{x1D700}_{r}$
appears in the system of the newly derived equations. However, the relation of the ansatz
![]() $\langle \unicode[STIX]{x1D700}_{r}^{n/3}\rangle$
for the
$\langle \unicode[STIX]{x1D700}_{r}^{n/3}\rangle$
for the
![]() $n$
th-order structure function to the Navier–Stokes equations is still missing.
$n$
th-order structure function to the Navier–Stokes equations is still missing.
There have been various attempts to modify the RSH by proposing other functions than the log-normal distribution function. Some examples are the multifractal model by Meneveau & Sreenivasan (Reference Meneveau and Sreenivasan1991), or the She & Lévêque (Reference She and Lévêque1994) model, which later was shown to be equivalent to assuming a log-Poisson distribution for the dissipation, cf. Dubrulle (Reference Dubrulle1994) and She & Waymire (Reference She and Waymire1995). A discussion of these models is given by Frisch (Reference Frisch1995). A survey of the work existing in 1997 on the RSH, anomalous scaling exponents, velocity derivative statistics and intermittency models was given by Sreenivasan & Antonia (Reference Sreenivasan and Antonia1997).
The basis of our analysis lies in the exact equations for structure functions of all orders which Hill (Reference Hill2001) and Yakhot (Reference Yakhot2001) derived from the Navier–Stokes equations. Other than in the trace of the second-order equation, the source terms in the higher-order equations depend on
![]() $r$
and contain correlations between pressure gradients, velocity gradients squared and velocity differences. The source terms containing pressure gradients will be called pressure source terms and those containing velocity gradients will be called dissipation source terms in the following.
$r$
and contain correlations between pressure gradients, velocity gradients squared and velocity differences. The source terms containing pressure gradients will be called pressure source terms and those containing velocity gradients will be called dissipation source terms in the following.
The papers of Hill (Reference Hill2001) and Yakhot (Reference Yakhot2001) differ substantially (cf. http://arxiv.org/abs/physics/0102055). Hill (Reference Hill2001) derived the structure function equations using no approximations, then simplified them for the anisotropic locally homogeneous and then to the (locally) homogeneous isotropic case. For the latter he invented a matrix algorithm to generate expressions for the isotropic forms for the transport terms and the viscous terms for all orders
![]() $n$
from 2 to 7. By using isotropic tensor relations, he identified the non-zero and zero components of the tensors and derived kinematic relationships between the non-zero components. Yakhot (Reference Yakhot2001) started his derivation from a generating function and allowed for arbitrary dimensions. Assuming locally homogeneous and isotropic turbulence, the structure functions can be generated by successive differentiation. For the three-dimensional case, he considered the equations of uneven order (which contain the even-order structure function in the transport terms) and neglected the dissipation source terms therein. For the pressure source terms, he derived a model using the probability density function of the transverse velocity increments. The purpose of Yakhot’s paper was to derive a formula for the transverse inertial-range scaling exponents of even order. More recently, Falkovich, Fouxon & Oz (Reference Falkovich, Fouxon and Oz2010) started from a general flux equation from which they derived an analogue to Kolmogorov’s
$n$
from 2 to 7. By using isotropic tensor relations, he identified the non-zero and zero components of the tensors and derived kinematic relationships between the non-zero components. Yakhot (Reference Yakhot2001) started his derivation from a generating function and allowed for arbitrary dimensions. Assuming locally homogeneous and isotropic turbulence, the structure functions can be generated by successive differentiation. For the three-dimensional case, he considered the equations of uneven order (which contain the even-order structure function in the transport terms) and neglected the dissipation source terms therein. For the pressure source terms, he derived a model using the probability density function of the transverse velocity increments. The purpose of Yakhot’s paper was to derive a formula for the transverse inertial-range scaling exponents of even order. More recently, Falkovich, Fouxon & Oz (Reference Falkovich, Fouxon and Oz2010) started from a general flux equation from which they derived an analogue to Kolmogorov’s
![]() $4/5$
law for compressible turbulence, as well as a new fifth-order relation for incompressible turbulence. L’vov & Procaccia (Reference L’vov and Procaccia1996b
) considered multipoint correlation functions for which they formulated fusion rules and used correlations between the one-point dissipation rates and velocity differences to relate the scaling exponents of structure functions and dissipation fluctuations. The multipoint approach by L’vov & Procaccia (Reference L’vov and Procaccia1996b
) can be viewed as a generalization of the classical two-point statistics, as the latter are contained in the former by fusion of the coordinates. Fusion rule predictions were examined by Fairhall et al. (Reference Fairhall, Dhruva, L’vov, Procaccia and Sreenivasan1997) and Benzi, Biferale & Toschi (Reference Benzi, Biferale and Toschi1998) and found to be in good agreement with measurements. In principle, it is possible to derive transport equations for the general multipoint quantities and their source terms using the procedure of Hill (Reference Hill2001). However, these equations are somewhat cumbersome to derive and to examine. For that reason, here we rather focus on the classical two-point structure functions characterized by only one separation distance and not two or more, thus simplifying the analysis.
$4/5$
law for compressible turbulence, as well as a new fifth-order relation for incompressible turbulence. L’vov & Procaccia (Reference L’vov and Procaccia1996b
) considered multipoint correlation functions for which they formulated fusion rules and used correlations between the one-point dissipation rates and velocity differences to relate the scaling exponents of structure functions and dissipation fluctuations. The multipoint approach by L’vov & Procaccia (Reference L’vov and Procaccia1996b
) can be viewed as a generalization of the classical two-point statistics, as the latter are contained in the former by fusion of the coordinates. Fusion rule predictions were examined by Fairhall et al. (Reference Fairhall, Dhruva, L’vov, Procaccia and Sreenivasan1997) and Benzi, Biferale & Toschi (Reference Benzi, Biferale and Toschi1998) and found to be in good agreement with measurements. In principle, it is possible to derive transport equations for the general multipoint quantities and their source terms using the procedure of Hill (Reference Hill2001). However, these equations are somewhat cumbersome to derive and to examine. For that reason, here we rather focus on the classical two-point structure functions characterized by only one separation distance and not two or more, thus simplifying the analysis.
In the literature, source terms of higher-order structure function equations have been analysed by direct numerical simulations (DNS) and, to the extent that this was possible, by hot-wire measurements. Hill & Boratav (Reference Hill and Boratav2001) analysed the third-order structure function equations based on DNS and experiments. From their analysis, it appears that only the pressure source terms determine the solutions at the third order. Kurien & Sreenivasan (Reference Kurien and Sreenivasan2001) discussed the Yakhot (Reference Yakhot2001) paper and the models presented therein in detail. They then used high-Reynolds-number experimental data from the atmospheric boundary layer to compute the pressure terms from Yakhot’s model and balance the terms of the transverse and mixed fourth-order structure function equations in the inertial range. Gotoh & Nakano (Reference Gotoh and Nakano2003) have examined on the basis of DNS data the balances between the even-order structure functions and the pressure source terms in the uneven-order equations up to eighth order and proposed a model for the pressure source terms. Yakhot (Reference Yakhot2003) has modified this model to obtain the same formula as in Yakhot (Reference Yakhot2001), but now for longitudinal inertial-range scaling exponents. Based on a model for the probability density function for longitudinal velocity increments, Yakhot (Reference Yakhot2006) discussed the closure of the structure function equations in terms of the dissipation anomaly (cf. Polyakov Reference Polyakov1995; Yakhot & Sreenivasan Reference Yakhot and Sreenivasan2005). Yakhot (Reference Yakhot2006) also introduced order-dependent dissipation scales
![]() $\unicode[STIX]{x1D702}_{n}$
. Using DNS data, Nakano, Gotoh & Fukayama (Reference Nakano, Gotoh and Fukayama2003) normalized the dissipation source terms in the longitudinal equations up to eighth order by the next-order structure function. They found that for all even-order equations, the normalized dissipation source terms are of order unity. This suggests that the dissipation source terms rather than the pressure source terms are dominant in the even-order equations.
$\unicode[STIX]{x1D702}_{n}$
. Using DNS data, Nakano, Gotoh & Fukayama (Reference Nakano, Gotoh and Fukayama2003) normalized the dissipation source terms in the longitudinal equations up to eighth order by the next-order structure function. They found that for all even-order equations, the normalized dissipation source terms are of order unity. This suggests that the dissipation source terms rather than the pressure source terms are dominant in the even-order equations.
Here, we will focus on the trace of the fourth-order structure function equations rather than the component equations, because it simplifies the treatment of the equations. Additionally, there is an analogy to the trace of the second-order structure function equations, which contains the mean dissipation
![]() $\langle \unicode[STIX]{x1D700}\rangle$
. We may therefore expect to find the second moment
$\langle \unicode[STIX]{x1D700}\rangle$
. We may therefore expect to find the second moment
![]() $\langle \unicode[STIX]{x1D700}^{2}\rangle$
in the fourth-order equations. In this context, it is also worth mentioning that the trace is of particular interest because it is invariant, i.e. independent of the coordinate system, as are
$\langle \unicode[STIX]{x1D700}^{2}\rangle$
in the fourth-order equations. In this context, it is also worth mentioning that the trace is of particular interest because it is invariant, i.e. independent of the coordinate system, as are
![]() $\langle \unicode[STIX]{x1D700}\rangle$
and Siggia (Reference Siggia1981)’s invariants. This is especially important if one is interested in scaling parameters such as
$\langle \unicode[STIX]{x1D700}\rangle$
and Siggia (Reference Siggia1981)’s invariants. This is especially important if one is interested in scaling parameters such as
![]() $\langle \unicode[STIX]{x1D700}^{m}\rangle$
. For instance, Hill (Reference Hill2002) showed that the mean dissipation
$\langle \unicode[STIX]{x1D700}^{m}\rangle$
. For instance, Hill (Reference Hill2002) showed that the mean dissipation
![]() $\langle \unicode[STIX]{x1D700}\rangle$
is found in the trace of the second-order structure function equations (those quantities being invariants), while the equations for the components of the second-order structure function contain the pseudo-dissipation tensor
$\langle \unicode[STIX]{x1D700}\rangle$
is found in the trace of the second-order structure function equations (those quantities being invariants), while the equations for the components of the second-order structure function contain the pseudo-dissipation tensor
![]() $\langle \unicode[STIX]{x1D626}_{ij}\rangle$
. Kolmogorov’s K41 equation relating a component of the third-order structure function to the invariant
$\langle \unicode[STIX]{x1D626}_{ij}\rangle$
. Kolmogorov’s K41 equation relating a component of the third-order structure function to the invariant
![]() $\langle \unicode[STIX]{x1D700}\rangle$
is obtained because of the incompressibility relation (cf. (3.22)–(3.23) in Hill (Reference Hill2002)). Analogous incompressibility relations do not exist at higher order, such that one should not expect equations relating individual components of higher-order structure functions to an invariant quantity analogous to
$\langle \unicode[STIX]{x1D700}\rangle$
is obtained because of the incompressibility relation (cf. (3.22)–(3.23) in Hill (Reference Hill2002)). Analogous incompressibility relations do not exist at higher order, such that one should not expect equations relating individual components of higher-order structure functions to an invariant quantity analogous to
![]() $\langle \unicode[STIX]{x1D700}\rangle$
.
$\langle \unicode[STIX]{x1D700}\rangle$
.
The paper is organized as follows. In § 2, we will present the equation for the trace of the fourth-order structure functions and the equation for the dissipation source term therein. We will find in the source term equations several new dissipation parameters. In § 3, we will relate these dissipation parameters to each other and to the second moment
![]() $\langle \unicode[STIX]{x1D700}^{2}\rangle$
of the distribution function of the dissipation
$\langle \unicode[STIX]{x1D700}^{2}\rangle$
of the distribution function of the dissipation
![]() $\unicode[STIX]{x1D700}$
. We will also show how they are related to the fourth-order moments of the velocity gradient distribution function and to the solutions of the fourth-order structure functions in the viscous range. In § 4, we will investigate the effect of dissipation parameters on the inertial-range scaling exponents by integrating the structure function equations in the inertial range. Using order-of-magnitude estimates for the different source terms, we thereby determine the fifth-order scaling exponents. We will also look at the connection between dissipation fluctuations traditionally represented by the volume-averaged dissipation
$\unicode[STIX]{x1D700}$
. We will also show how they are related to the fourth-order moments of the velocity gradient distribution function and to the solutions of the fourth-order structure functions in the viscous range. In § 4, we will investigate the effect of dissipation parameters on the inertial-range scaling exponents by integrating the structure function equations in the inertial range. Using order-of-magnitude estimates for the different source terms, we thereby determine the fifth-order scaling exponents. We will also look at the connection between dissipation fluctuations traditionally represented by the volume-averaged dissipation
![]() $\unicode[STIX]{x1D700}_{r}$
and the dissipation parameters identified in the dissipative source term equations. Conclusions are briefly presented in § 5.
$\unicode[STIX]{x1D700}_{r}$
and the dissipation parameters identified in the dissipative source term equations. Conclusions are briefly presented in § 5.
2 Equations for the trace of structure functions and dissipation source terms at the fourth-order level
With the procedure used by Hill (Reference Hill2001), we have derived equations for successive source terms appearing in the equations for structure functions up to the sixth order under the assumptions of homogeneity and isotropy. The idea is that the source terms determine the solution of the structure function equations, i.e. the structure functions themselves. In the following, we present the equations used in the next sections. The entire set of successive equations (up to the sixth order) is documented in the archive material available from http://arxiv.org/abs/1504.07490. While it is possible to carry out the same analysis at higher orders, both the number of equations and the number of terms to be analysed increase very quickly; for that reason we consider only the fourth order here. We will look at the fourth-order equations in the viscous range in § 3, while the inertial-range assumptions are used in § 4. We have also performed DNS in a cubic box for Taylor-based Reynolds numbers up to 529 and for some parts of the analysis up to
![]() $Re_{\unicode[STIX]{x1D706}}=754$
. This is briefly described in appendix A.
$Re_{\unicode[STIX]{x1D706}}=754$
. This is briefly described in appendix A.
The two points at which velocity fluctuations are sampled are denoted as
![]() $\boldsymbol{x}=(x_{1},x_{2},x_{3})$
and
$\boldsymbol{x}=(x_{1},x_{2},x_{3})$
and
![]() $\boldsymbol{x}^{\prime }=(x_{1}^{\prime },x_{2}^{\prime },x_{3}^{\prime })$
, with
$\boldsymbol{x}^{\prime }=(x_{1}^{\prime },x_{2}^{\prime },x_{3}^{\prime })$
, with
![]() $x_{1}$
pointing in the longitudinal direction and
$x_{1}$
pointing in the longitudinal direction and
![]() $x_{2}$
and
$x_{2}$
and
![]() $x_{3}$
in the transverse direction. Structure functions are defined as
$x_{3}$
in the transverse direction. Structure functions are defined as
![]() $S_{p,q}=\langle (\unicode[STIX]{x0394}u_{1})^{p}(\unicode[STIX]{x0394}u_{2})^{q}\rangle$
, with
$S_{p,q}=\langle (\unicode[STIX]{x0394}u_{1})^{p}(\unicode[STIX]{x0394}u_{2})^{q}\rangle$
, with
![]() $\unicode[STIX]{x0394}u_{1}=u_{1}-u_{1}^{\prime }$
being the longitudinal and
$\unicode[STIX]{x0394}u_{1}=u_{1}-u_{1}^{\prime }$
being the longitudinal and
![]() $\unicode[STIX]{x0394}u_{2}=u_{2}-u_{2}^{\prime }$
the transverse velocity difference, with
$\unicode[STIX]{x0394}u_{2}=u_{2}-u_{2}^{\prime }$
the transverse velocity difference, with
![]() $u_{1}$
and
$u_{1}$
and
![]() $u_{2}$
taken at
$u_{2}$
taken at
![]() $x_{1}$
and
$x_{1}$
and
![]() $u_{1}^{\prime }$
and
$u_{1}^{\prime }$
and
![]() $u_{2}^{\prime }$
at
$u_{2}^{\prime }$
at
![]() $x_{1}^{\prime }$
. For the
$x_{1}^{\prime }$
. For the
![]() $n$
th-order moment,
$n$
th-order moment,
![]() $n=p+q$
in these equations. Hence, at the fourth order,
$n=p+q$
in these equations. Hence, at the fourth order,
![]() $S_{4,0}$
is the longitudinal,
$S_{4,0}$
is the longitudinal,
![]() $S_{2,2}$
is the mixed and
$S_{2,2}$
is the mixed and
![]() $S_{0,4}$
is the transverse structure function. In the following, Einstein’s summation convention is used, which implies summation over indices appearing twice. For instance,
$S_{0,4}$
is the transverse structure function. In the following, Einstein’s summation convention is used, which implies summation over indices appearing twice. For instance,
![]() $\langle \unicode[STIX]{x1D700}_{ij}^{2}\rangle =\langle \unicode[STIX]{x1D700}_{ij}\unicode[STIX]{x1D700}_{ij}\rangle$
, where the sum is over
$\langle \unicode[STIX]{x1D700}_{ij}^{2}\rangle =\langle \unicode[STIX]{x1D700}_{ij}\unicode[STIX]{x1D700}_{ij}\rangle$
, where the sum is over
![]() $i$
and
$i$
and
![]() $j$
. The equations for
$j$
. The equations for
![]() $S_{4,0}$
,
$S_{4,0}$
,
![]() $S_{2,2}$
and
$S_{2,2}$
and
![]() $S_{0,4}$
are given in appendix B. In this paper, we examine the trace of the structure function tensor
$S_{0,4}$
are given in appendix B. In this paper, we examine the trace of the structure function tensor
and the same analysis can be carried over to the individual equations. As
cf. Hill (Reference Hill2001), the trace amounts to the sum
Consequently, the transport equation for
![]() $\mathit{S}_{[4]}$
is derived by adding the individual structure function equations (B 1)–(B 3) as given in appendix B multiplied by the corresponding prefactor. This results in
$\mathit{S}_{[4]}$
is derived by adding the individual structure function equations (B 1)–(B 3) as given in appendix B multiplied by the corresponding prefactor. This results in
Here, the second term on the left-hand side is the transport term containing the fifth-order longitudinal, mixed and transverse structure functions
![]() $S_{5,0}$
,
$S_{5,0}$
,
![]() $S_{3,2}$
and
$S_{3,2}$
and
![]() $S_{1,4}$
respectively. The first term on the right-hand side of (2.4) is the viscous term containing the fourth-order structure functions
$S_{1,4}$
respectively. The first term on the right-hand side of (2.4) is the viscous term containing the fourth-order structure functions
![]() $S_{4,0}$
,
$S_{4,0}$
,
![]() $S_{2,2}$
and
$S_{2,2}$
and
![]() $S_{0,4}$
. The remaining two terms in the equation are the pressure source term and the dissipative source term in that order. Equation (2.4) is closely related to equation (4.3) of Falkovich et al. (Reference Falkovich, Fouxon and Oz2010) (also a fourth-order equation), which they derived from the kinetic energy equation. From this, they found a new relation for the pressure–velocity correlation in the inertial range, similar to Kolmogorov’s
$S_{0,4}$
. The remaining two terms in the equation are the pressure source term and the dissipative source term in that order. Equation (2.4) is closely related to equation (4.3) of Falkovich et al. (Reference Falkovich, Fouxon and Oz2010) (also a fourth-order equation), which they derived from the kinetic energy equation. From this, they found a new relation for the pressure–velocity correlation in the inertial range, similar to Kolmogorov’s
![]() $4/5$
law stemming from the second order.
$4/5$
law stemming from the second order.
In explicit notation, the transport term is written as
 $$\begin{eqnarray}\displaystyle \frac{\unicode[STIX]{x2202}}{\unicode[STIX]{x2202}r_{n}}\langle \unicode[STIX]{x0394}u_{n}(\unicode[STIX]{x0394}u_{i})^{2}(\unicode[STIX]{x0394}u_{j})^{2}\rangle & = & \displaystyle \frac{\unicode[STIX]{x2202}S_{5,0}}{\unicode[STIX]{x2202}r}+4\frac{\unicode[STIX]{x2202}S_{3,2}}{\unicode[STIX]{x2202}r}+\frac{8}{3}\frac{\unicode[STIX]{x2202}S_{1,4}}{\unicode[STIX]{x2202}r}+\frac{2}{r}S_{5,0}+\frac{8}{r}S_{3,2}-\frac{16}{3r}S_{1,4}\nonumber\\ \displaystyle & = & \displaystyle \frac{\unicode[STIX]{x2202}\mathit{S}_{[5]}}{\unicode[STIX]{x2202}r}+\frac{2}{r}\mathit{S}_{[5]},\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \frac{\unicode[STIX]{x2202}}{\unicode[STIX]{x2202}r_{n}}\langle \unicode[STIX]{x0394}u_{n}(\unicode[STIX]{x0394}u_{i})^{2}(\unicode[STIX]{x0394}u_{j})^{2}\rangle & = & \displaystyle \frac{\unicode[STIX]{x2202}S_{5,0}}{\unicode[STIX]{x2202}r}+4\frac{\unicode[STIX]{x2202}S_{3,2}}{\unicode[STIX]{x2202}r}+\frac{8}{3}\frac{\unicode[STIX]{x2202}S_{1,4}}{\unicode[STIX]{x2202}r}+\frac{2}{r}S_{5,0}+\frac{8}{r}S_{3,2}-\frac{16}{3r}S_{1,4}\nonumber\\ \displaystyle & = & \displaystyle \frac{\unicode[STIX]{x2202}\mathit{S}_{[5]}}{\unicode[STIX]{x2202}r}+\frac{2}{r}\mathit{S}_{[5]},\end{eqnarray}$$
where
![]() $\mathit{S}_{[5]}$
is the trace of the general fifth-order structure function tensor,
$\mathit{S}_{[5]}$
is the trace of the general fifth-order structure function tensor,
The pressure source term is given by
 $$\begin{eqnarray}\displaystyle \langle T_{[4]}\rangle & = & \displaystyle 4\langle (\unicode[STIX]{x0394}u_{i})^{2}\unicode[STIX]{x0394}u_{j}\unicode[STIX]{x0394}P_{j}\rangle \nonumber\\ \displaystyle & = & \displaystyle 4\langle (\unicode[STIX]{x0394}u_{1})^{3}\unicode[STIX]{x0394}P_{1}\rangle +8\langle (\unicode[STIX]{x0394}u_{2})^{2}\unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}P_{1}+(\unicode[STIX]{x0394}u_{1})^{2}\unicode[STIX]{x0394}u_{2}\unicode[STIX]{x0394}P_{2}\rangle \nonumber\\ \displaystyle & & \displaystyle +\,{\textstyle \frac{32}{3}}\langle (\unicode[STIX]{x0394}u_{2})^{3}\unicode[STIX]{x0394}P_{2}\rangle ,\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \langle T_{[4]}\rangle & = & \displaystyle 4\langle (\unicode[STIX]{x0394}u_{i})^{2}\unicode[STIX]{x0394}u_{j}\unicode[STIX]{x0394}P_{j}\rangle \nonumber\\ \displaystyle & = & \displaystyle 4\langle (\unicode[STIX]{x0394}u_{1})^{3}\unicode[STIX]{x0394}P_{1}\rangle +8\langle (\unicode[STIX]{x0394}u_{2})^{2}\unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}P_{1}+(\unicode[STIX]{x0394}u_{1})^{2}\unicode[STIX]{x0394}u_{2}\unicode[STIX]{x0394}P_{2}\rangle \nonumber\\ \displaystyle & & \displaystyle +\,{\textstyle \frac{32}{3}}\langle (\unicode[STIX]{x0394}u_{2})^{3}\unicode[STIX]{x0394}P_{2}\rangle ,\end{eqnarray}$$
the dissipative source term by
 $$\begin{eqnarray}\displaystyle \langle E_{[4]}\rangle & = & \displaystyle \langle 2(\unicode[STIX]{x0394}u_{i})^{2}(\unicode[STIX]{x1D700}_{jj}+\unicode[STIX]{x1D700}_{jj}^{\prime })+4\unicode[STIX]{x0394}u_{i}\unicode[STIX]{x0394}u_{j}(\unicode[STIX]{x1D700}_{ij}+\unicode[STIX]{x1D700}_{ij}^{\prime })\rangle \nonumber\\ \displaystyle & = & \displaystyle 6\langle (\unicode[STIX]{x0394}u_{1})^{2}(\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })\rangle +4\langle (\unicode[STIX]{x0394}u_{2})^{2}(\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })+(\unicode[STIX]{x0394}u_{1})^{2}(\unicode[STIX]{x1D700}_{22}+\unicode[STIX]{x1D700}_{22}^{\prime })\rangle \nonumber\\ \displaystyle & & \displaystyle +\,16\langle \unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}u_{2}(\unicode[STIX]{x1D700}_{12}+\unicode[STIX]{x1D700}_{12}^{\prime })+(\unicode[STIX]{x0394}u_{2})^{2}(\unicode[STIX]{x1D700}_{22}+\unicode[STIX]{x1D700}_{22}^{\prime })\rangle\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \langle E_{[4]}\rangle & = & \displaystyle \langle 2(\unicode[STIX]{x0394}u_{i})^{2}(\unicode[STIX]{x1D700}_{jj}+\unicode[STIX]{x1D700}_{jj}^{\prime })+4\unicode[STIX]{x0394}u_{i}\unicode[STIX]{x0394}u_{j}(\unicode[STIX]{x1D700}_{ij}+\unicode[STIX]{x1D700}_{ij}^{\prime })\rangle \nonumber\\ \displaystyle & = & \displaystyle 6\langle (\unicode[STIX]{x0394}u_{1})^{2}(\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })\rangle +4\langle (\unicode[STIX]{x0394}u_{2})^{2}(\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })+(\unicode[STIX]{x0394}u_{1})^{2}(\unicode[STIX]{x1D700}_{22}+\unicode[STIX]{x1D700}_{22}^{\prime })\rangle \nonumber\\ \displaystyle & & \displaystyle +\,16\langle \unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}u_{2}(\unicode[STIX]{x1D700}_{12}+\unicode[STIX]{x1D700}_{12}^{\prime })+(\unicode[STIX]{x0394}u_{2})^{2}(\unicode[STIX]{x1D700}_{22}+\unicode[STIX]{x1D700}_{22}^{\prime })\rangle\end{eqnarray}$$
and the viscous term by
 $$\begin{eqnarray}\displaystyle 2\unicode[STIX]{x1D708}\frac{\unicode[STIX]{x2202}^{2}\mathit{S}_{[4]}}{\unicode[STIX]{x2202}r_{n}^{2}} & = & \displaystyle 2\unicode[STIX]{x1D708}\left[\frac{\unicode[STIX]{x2202}^{2}S_{4,0}}{\unicode[STIX]{x2202}r^{2}}+\frac{2}{r}\frac{\unicode[STIX]{x2202}S_{4,0}}{\unicode[STIX]{x2202}r}+4\frac{\unicode[STIX]{x2202}^{2}S_{2,2}}{\unicode[STIX]{x2202}r^{2}}+\frac{8}{r}\frac{\unicode[STIX]{x2202}S_{2,2}}{\unicode[STIX]{x2202}r}+\frac{8}{3}\frac{\unicode[STIX]{x2202}^{2}S_{0,4}}{\unicode[STIX]{x2202}r^{2}}+\frac{16}{3r}\frac{\unicode[STIX]{x2202}S_{0,4}}{\unicode[STIX]{x2202}r}\right]\nonumber\\ \displaystyle & = & \displaystyle 2\unicode[STIX]{x1D708}\left[\frac{\unicode[STIX]{x2202}^{2}\mathit{S}_{[4]}}{\unicode[STIX]{x2202}r^{2}}+\frac{2}{r}\frac{\unicode[STIX]{x2202}\mathit{S}_{[4]}}{\unicode[STIX]{x2202}r}\right].\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle 2\unicode[STIX]{x1D708}\frac{\unicode[STIX]{x2202}^{2}\mathit{S}_{[4]}}{\unicode[STIX]{x2202}r_{n}^{2}} & = & \displaystyle 2\unicode[STIX]{x1D708}\left[\frac{\unicode[STIX]{x2202}^{2}S_{4,0}}{\unicode[STIX]{x2202}r^{2}}+\frac{2}{r}\frac{\unicode[STIX]{x2202}S_{4,0}}{\unicode[STIX]{x2202}r}+4\frac{\unicode[STIX]{x2202}^{2}S_{2,2}}{\unicode[STIX]{x2202}r^{2}}+\frac{8}{r}\frac{\unicode[STIX]{x2202}S_{2,2}}{\unicode[STIX]{x2202}r}+\frac{8}{3}\frac{\unicode[STIX]{x2202}^{2}S_{0,4}}{\unicode[STIX]{x2202}r^{2}}+\frac{16}{3r}\frac{\unicode[STIX]{x2202}S_{0,4}}{\unicode[STIX]{x2202}r}\right]\nonumber\\ \displaystyle & = & \displaystyle 2\unicode[STIX]{x1D708}\left[\frac{\unicode[STIX]{x2202}^{2}\mathit{S}_{[4]}}{\unicode[STIX]{x2202}r^{2}}+\frac{2}{r}\frac{\unicode[STIX]{x2202}\mathit{S}_{[4]}}{\unicode[STIX]{x2202}r}\right].\end{eqnarray}$$
In (2.4)–(2.9), the definitions
have been used.
The balance of the different terms in (2.4) is shown in figure 1(a) for the case
![]() $R0$
(
$R0$
(
![]() $\mathit{Re}_{\unicode[STIX]{x1D706}}=88$
) and in figure 1(b) for the case
$\mathit{Re}_{\unicode[STIX]{x1D706}}=88$
) and in figure 1(b) for the case
![]() $R5$
(
$R5$
(
![]() $\mathit{Re}_{\unicode[STIX]{x1D706}}=529$
) of our DNS. We will need this balance to estimate which terms we may neglect when we integrate (2.4) in the viscous range in § 3 and in the inertial range in § 4 below. The terms have been normalized with
$\mathit{Re}_{\unicode[STIX]{x1D706}}=529$
) of our DNS. We will need this balance to estimate which terms we may neglect when we integrate (2.4) in the viscous range in § 3 and in the inertial range in § 4 below. The terms have been normalized with
![]() $(\unicode[STIX]{x1D708}^{2}\langle \unicode[STIX]{x1D700}^{2}\rangle ^{3})^{1/4}$
and the separation distance
$(\unicode[STIX]{x1D708}^{2}\langle \unicode[STIX]{x1D700}^{2}\rangle ^{3})^{1/4}$
and the separation distance
![]() $r$
with the Kolmogorov scale
$r$
with the Kolmogorov scale
![]() $\unicode[STIX]{x1D702}$
. It is seen that in the viscous range for values of
$\unicode[STIX]{x1D702}$
. It is seen that in the viscous range for values of
![]() $r/\unicode[STIX]{x1D702}$
up to approximately 5, the transport term and the pressure source term are an order of magnitude smaller than the viscous terms and the dissipation source term for the six data sets
$r/\unicode[STIX]{x1D702}$
up to approximately 5, the transport term and the pressure source term are an order of magnitude smaller than the viscous terms and the dissipation source term for the six data sets
![]() $R0$
–
$R0$
–
![]() $R5$
(
$R5$
(
![]() $R1$
–
$R1$
–
![]() $R4$
are not shown here). These terms therefore balance to leading order, which will lead to exact relations in the viscous range, as will be shown in § 3. As expected, the inertial range increases with increasing Reynolds number: beginning at
$R4$
are not shown here). These terms therefore balance to leading order, which will lead to exact relations in the viscous range, as will be shown in § 3. As expected, the inertial range increases with increasing Reynolds number: beginning at
![]() $r/\unicode[STIX]{x1D702}=30$
, for the case
$r/\unicode[STIX]{x1D702}=30$
, for the case
![]() $R0$
(
$R0$
(
![]() $Re_{\unicode[STIX]{x1D706}}=88$
) only a very small inertial range is identifiable, whereas for
$Re_{\unicode[STIX]{x1D706}}=88$
) only a very small inertial range is identifiable, whereas for
![]() $R5$
(
$R5$
(
![]() $Re_{\unicode[STIX]{x1D706}}=529$
) it extends to
$Re_{\unicode[STIX]{x1D706}}=529$
) it extends to
![]() $r/\unicode[STIX]{x1D702}\approx 300$
. In the inertial range and for all data sets, the pressure source term is smaller by a factor of four on average and the viscous term is much smaller. This order-of-magnitude estimate will be used in § 4.
$r/\unicode[STIX]{x1D702}\approx 300$
. In the inertial range and for all data sets, the pressure source term is smaller by a factor of four on average and the viscous term is much smaller. This order-of-magnitude estimate will be used in § 4.

Figure 1. Balance of the different terms in (2.4) for the cases
![]() $R0$
(
$R0$
(
![]() $\mathit{Re}_{\unicode[STIX]{x1D706}}=88$
) (a) and
$\mathit{Re}_{\unicode[STIX]{x1D706}}=88$
) (a) and
![]() $R5$
(
$R5$
(
![]() $\mathit{Re}_{\unicode[STIX]{x1D706}}=529$
) (b): ○, transport term; ▫, dissipative source term; ▿, pressure source term; ▵, viscous term.
$\mathit{Re}_{\unicode[STIX]{x1D706}}=529$
) (b): ○, transport term; ▫, dissipative source term; ▿, pressure source term; ▵, viscous term.
The dissipation term
![]() $\langle E_{[4]}\rangle$
defined in (2.8) is a correlation between squared velocity increments
$\langle E_{[4]}\rangle$
defined in (2.8) is a correlation between squared velocity increments
![]() $\unicode[STIX]{x0394}u_{1}$
and
$\unicode[STIX]{x0394}u_{1}$
and
![]() $\unicode[STIX]{x0394}u_{2}$
and the instantaneous dissipations defined by (2.11) at
$\unicode[STIX]{x0394}u_{2}$
and the instantaneous dissipations defined by (2.11) at
![]() $x_{1}$
and likewise at
$x_{1}$
and likewise at
![]() $x_{1}^{\prime }$
. Since it balances the transport term in the inertial range and the viscous term in the viscous range,
$x_{1}^{\prime }$
. Since it balances the transport term in the inertial range and the viscous term in the viscous range,
![]() $\langle E_{[4]}\rangle$
is of particular interest and will be further examined in the following. Furthermore, one might expect the dissipative source term to contain dissipative fluctuations. The equation for the dissipative source term,
$\langle E_{[4]}\rangle$
is of particular interest and will be further examined in the following. Furthermore, one might expect the dissipative source term to contain dissipative fluctuations. The equation for the dissipative source term,
reads with the equations for
![]() $\langle E_{4,0}\rangle$
,
$\langle E_{4,0}\rangle$
,
![]() $\langle E_{2,2}\rangle$
and
$\langle E_{2,2}\rangle$
and
![]() $\langle E_{0,4}\rangle$
, cf. (B 10), (B 12) and (B 14) given in appendix B,
$\langle E_{0,4}\rangle$
, cf. (B 10), (B 12) and (B 14) given in appendix B,
 $$\begin{eqnarray}\displaystyle \frac{\langle \unicode[STIX]{x2202}E_{[4]}\rangle }{\unicode[STIX]{x2202}t}+\frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{n}E_{[4]}\rangle }{\unicode[STIX]{x2202}r_{n}} & = & \displaystyle \unicode[STIX]{x1D708}\frac{\unicode[STIX]{x2202}^{2}}{\unicode[STIX]{x2202}r_{n}^{2}}\langle E_{[4]}\rangle \nonumber\\ \displaystyle & & \displaystyle -\,\underbrace{(F_{[4]}+Q_{[4]}+P_{[4]}+T_{[4]}+D_{[4]}+\unicode[STIX]{x1D700}_{[4]}^{2})}_{\sum \mathop{P}_{[4]}^{E}},\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \frac{\langle \unicode[STIX]{x2202}E_{[4]}\rangle }{\unicode[STIX]{x2202}t}+\frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{n}E_{[4]}\rangle }{\unicode[STIX]{x2202}r_{n}} & = & \displaystyle \unicode[STIX]{x1D708}\frac{\unicode[STIX]{x2202}^{2}}{\unicode[STIX]{x2202}r_{n}^{2}}\langle E_{[4]}\rangle \nonumber\\ \displaystyle & & \displaystyle -\,\underbrace{(F_{[4]}+Q_{[4]}+P_{[4]}+T_{[4]}+D_{[4]}+\unicode[STIX]{x1D700}_{[4]}^{2})}_{\sum \mathop{P}_{[4]}^{E}},\end{eqnarray}$$
where
![]() $\sum P_{[4]}^{E}$
is the trace of the sum of source terms in the dissipative source term equation, where the transport term is defined as
$\sum P_{[4]}^{E}$
is the trace of the sum of source terms in the dissipative source term equation, where the transport term is defined as
 $$\begin{eqnarray}\displaystyle \frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{n}E_{[4]}\rangle }{\unicode[STIX]{x2202}r_{n}} & = & \displaystyle \frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{1}E_{4,0}\rangle }{\unicode[STIX]{x2202}r}+4\frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{1}E_{2,2}\rangle }{\unicode[STIX]{x2202}r}+\frac{8}{3}\frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{1}E_{0,4}\rangle }{\unicode[STIX]{x2202}r}\nonumber\\ \displaystyle & & \displaystyle +\,\frac{2}{r}\langle \unicode[STIX]{x0394}u_{1}E_{4,0}\rangle +\frac{8}{r}\langle \unicode[STIX]{x0394}u_{1}E_{2,2}\rangle +\frac{16}{3r}\langle \unicode[STIX]{x0394}u_{1}E_{0,4}\rangle \nonumber\\ \displaystyle & = & \displaystyle \frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{1}E_{[4]}\rangle }{\unicode[STIX]{x2202}r}+\frac{2}{r}\langle \unicode[STIX]{x0394}u_{1}E_{[4]}\rangle ,\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{n}E_{[4]}\rangle }{\unicode[STIX]{x2202}r_{n}} & = & \displaystyle \frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{1}E_{4,0}\rangle }{\unicode[STIX]{x2202}r}+4\frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{1}E_{2,2}\rangle }{\unicode[STIX]{x2202}r}+\frac{8}{3}\frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{1}E_{0,4}\rangle }{\unicode[STIX]{x2202}r}\nonumber\\ \displaystyle & & \displaystyle +\,\frac{2}{r}\langle \unicode[STIX]{x0394}u_{1}E_{4,0}\rangle +\frac{8}{r}\langle \unicode[STIX]{x0394}u_{1}E_{2,2}\rangle +\frac{16}{3r}\langle \unicode[STIX]{x0394}u_{1}E_{0,4}\rangle \nonumber\\ \displaystyle & = & \displaystyle \frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{1}E_{[4]}\rangle }{\unicode[STIX]{x2202}r}+\frac{2}{r}\langle \unicode[STIX]{x0394}u_{1}E_{[4]}\rangle ,\end{eqnarray}$$
with
the viscous term
the
![]() $F$
-term
$F$
-term
the
![]() $Q$
-term
$Q$
-term
the
![]() $P$
-term
$P$
-term
the
![]() $T$
-term
$T$
-term
the
![]() $D$
-term
$D$
-term
 $$\begin{eqnarray}\displaystyle D_{[4]} & = & \displaystyle 4\unicode[STIX]{x1D708}\left\langle \left(\frac{\unicode[STIX]{x2202}\left(\unicode[STIX]{x0394}u_{i}\right)^{2}}{\unicode[STIX]{x2202}x_{n}}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{jj}+\unicode[STIX]{x1D700}_{jj}^{\prime }}{\unicode[STIX]{x2202}x_{n}}+\frac{\unicode[STIX]{x2202}\left(\unicode[STIX]{x0394}u_{i}\right)^{2}}{\unicode[STIX]{x2202}x_{n}^{\prime }}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{jj}^{\prime }+\unicode[STIX]{x1D700}_{jj}^{\prime }}{\unicode[STIX]{x2202}x_{n}^{\prime }}\right)\right.\nonumber\\ \displaystyle & & \displaystyle \left.+\,2\left(\frac{\unicode[STIX]{x2202}\unicode[STIX]{x0394}u_{i}\unicode[STIX]{x0394}u_{j}}{\unicode[STIX]{x2202}x_{n}}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{ij}+\unicode[STIX]{x1D700}_{ij}^{\prime }}{\unicode[STIX]{x2202}x_{n}}+\frac{\unicode[STIX]{x2202}\unicode[STIX]{x0394}u_{i}\unicode[STIX]{x0394}u_{j}}{\unicode[STIX]{x2202}x_{n}^{\prime }}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{ij}^{\prime }+\unicode[STIX]{x1D700}_{ij}^{\prime }}{\unicode[STIX]{x2202}x_{n}^{\prime }}\right)\right\rangle\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle D_{[4]} & = & \displaystyle 4\unicode[STIX]{x1D708}\left\langle \left(\frac{\unicode[STIX]{x2202}\left(\unicode[STIX]{x0394}u_{i}\right)^{2}}{\unicode[STIX]{x2202}x_{n}}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{jj}+\unicode[STIX]{x1D700}_{jj}^{\prime }}{\unicode[STIX]{x2202}x_{n}}+\frac{\unicode[STIX]{x2202}\left(\unicode[STIX]{x0394}u_{i}\right)^{2}}{\unicode[STIX]{x2202}x_{n}^{\prime }}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{jj}^{\prime }+\unicode[STIX]{x1D700}_{jj}^{\prime }}{\unicode[STIX]{x2202}x_{n}^{\prime }}\right)\right.\nonumber\\ \displaystyle & & \displaystyle \left.+\,2\left(\frac{\unicode[STIX]{x2202}\unicode[STIX]{x0394}u_{i}\unicode[STIX]{x0394}u_{j}}{\unicode[STIX]{x2202}x_{n}}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{ij}+\unicode[STIX]{x1D700}_{ij}^{\prime }}{\unicode[STIX]{x2202}x_{n}}+\frac{\unicode[STIX]{x2202}\unicode[STIX]{x0394}u_{i}\unicode[STIX]{x0394}u_{j}}{\unicode[STIX]{x2202}x_{n}^{\prime }}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{ij}^{\prime }+\unicode[STIX]{x1D700}_{ij}^{\prime }}{\unicode[STIX]{x2202}x_{n}^{\prime }}\right)\right\rangle\end{eqnarray}$$
and the
![]() $\unicode[STIX]{x1D700}^{2}$
-term
$\unicode[STIX]{x1D700}^{2}$
-term
In our notation,
![]() $\unicode[STIX]{x1D700}_{ii}=\unicode[STIX]{x1D700}$
is twice the pseudo-dissipation. For better readability, the definitions
$\unicode[STIX]{x1D700}_{ii}=\unicode[STIX]{x1D700}$
is twice the pseudo-dissipation. For better readability, the definitions
and
have been used in (2.17)–(2.20).
For the stationary case, the transport term on the left-hand side in (2.13) balances the viscous term and the sum of source terms
![]() $\sum P_{[4]}^{E}$
. The source terms are defined in (2.17) to (2.22) and are shown in figure 2 for the cases
$\sum P_{[4]}^{E}$
. The source terms are defined in (2.17) to (2.22) and are shown in figure 2 for the cases
![]() $R1$
(
$R1$
(
![]() $Re_{\unicode[STIX]{x1D706}}=119$
) and
$Re_{\unicode[STIX]{x1D706}}=119$
) and
![]() $R4$
(
$R4$
(
![]() $Re_{\unicode[STIX]{x1D706}}=331$
) together with the transport term and the viscous term. Negative terms are denoted with a minus sign, meaning that we have changed their sign in order to be able to show them in a log–log plot. As seen in figure 2, the largest ones are the
$Re_{\unicode[STIX]{x1D706}}=331$
) together with the transport term and the viscous term. Negative terms are denoted with a minus sign, meaning that we have changed their sign in order to be able to show them in a log–log plot. As seen in figure 2, the largest ones are the
![]() $F$
-term and the
$F$
-term and the
![]() $Q$
-term, which nearly balance each other. The
$Q$
-term, which nearly balance each other. The
![]() $F$
-term is a correlation between the velocity increments squared and the triple product of velocity gradients
$F$
-term is a correlation between the velocity increments squared and the triple product of velocity gradients
![]() $(A_{ij}+A_{ij}^{\prime })$
. The
$(A_{ij}+A_{ij}^{\prime })$
. The
![]() $Q$
-term is a correlation between the velocity increments and
$Q$
-term is a correlation between the velocity increments and
![]() $(\unicode[STIX]{x1D712}_{ij}+\unicode[STIX]{x1D712}_{ij}^{\prime })$
. The term
$(\unicode[STIX]{x1D712}_{ij}+\unicode[STIX]{x1D712}_{ij}^{\prime })$
. The term
![]() $\unicode[STIX]{x1D712}_{ij}$
describes the dissipation of velocity gradients squared, while
$\unicode[STIX]{x1D712}_{ij}$
describes the dissipation of velocity gradients squared, while
![]() $A_{ij}$
describes their production by stretching. The next two source terms, called the
$A_{ij}$
describes their production by stretching. The next two source terms, called the
![]() $T$
-term and the
$T$
-term and the
![]() $P$
-term, containing pressure derivatives, are relatively small and will not be discussed here in detail. Finally, there are the
$P$
-term, containing pressure derivatives, are relatively small and will not be discussed here in detail. Finally, there are the
![]() $D$
-term and the
$D$
-term and the
![]() $\unicode[STIX]{x1D700}^{2}$
-term, which balance each other in the inertial range, but strongly diverge from each other in the viscous range. In the following, the focus will be on the
$\unicode[STIX]{x1D700}^{2}$
-term, which balance each other in the inertial range, but strongly diverge from each other in the viscous range. In the following, the focus will be on the
![]() $\unicode[STIX]{x1D700}^{2}$
-term, which is the sum of the second-order dissipation parameters.
$\unicode[STIX]{x1D700}^{2}$
-term, which is the sum of the second-order dissipation parameters.

Figure 2. Balance of the different terms in (2.13) for the cases
![]() $R1$
(
$R1$
(
![]() $Re_{\unicode[STIX]{x1D706}}=119$
) (a) and
$Re_{\unicode[STIX]{x1D706}}=119$
) (a) and
![]() $R4$
(
$R4$
(
![]() $Re_{\unicode[STIX]{x1D706}}=331$
) (b): grey ○, transport term; grey ▫,
$Re_{\unicode[STIX]{x1D706}}=331$
) (b): grey ○, transport term; grey ▫,
![]() $F$
-term; grey ▿,
$F$
-term; grey ▿,
![]() $P$
-term; grey ▵,
$P$
-term; grey ▵,
![]() $T$
-term; ○,
$T$
-term; ○,
![]() $Q$
-term; ▫,
$Q$
-term; ▫,
![]() $D$
-term; ▿,
$D$
-term; ▿,
![]() $\unicode[STIX]{x1D700}^{2}$
-term; ▵, viscous term.
$\unicode[STIX]{x1D700}^{2}$
-term; ▵, viscous term.
The
![]() $\unicode[STIX]{x1D700}^{2}$
-term is defined as
$\unicode[STIX]{x1D700}^{2}$
-term is defined as
![]() $2\langle (\unicode[STIX]{x1D700}_{ii}+\unicode[STIX]{x1D700}_{ii}^{\prime })^{2}\rangle +4\langle (\unicode[STIX]{x1D700}_{ij}+\unicode[STIX]{x1D700}_{ij}^{\prime })^{2}\rangle$
and is a two-point quantity depending on
$2\langle (\unicode[STIX]{x1D700}_{ii}+\unicode[STIX]{x1D700}_{ii}^{\prime })^{2}\rangle +4\langle (\unicode[STIX]{x1D700}_{ij}+\unicode[STIX]{x1D700}_{ij}^{\prime })^{2}\rangle$
and is a two-point quantity depending on
![]() $r$
. Noticeably, Hill (Reference Hill2002) showed that the sum of the two-point dissipation
$r$
. Noticeably, Hill (Reference Hill2002) showed that the sum of the two-point dissipation
![]() $\langle \unicode[STIX]{x1D700}+\unicode[STIX]{x1D700}^{\prime }\rangle$
also appears in the trace of the second-order structure function equations, which then reduces to
$\langle \unicode[STIX]{x1D700}+\unicode[STIX]{x1D700}^{\prime }\rangle$
also appears in the trace of the second-order structure function equations, which then reduces to
![]() $2\langle \unicode[STIX]{x1D700}\rangle$
independent of
$2\langle \unicode[STIX]{x1D700}\rangle$
independent of
![]() $r$
for homogeneous flows.
$r$
for homogeneous flows.
Since
![]() $\unicode[STIX]{x1D700}_{ij}$
becomes equal to
$\unicode[STIX]{x1D700}_{ij}$
becomes equal to
![]() $\unicode[STIX]{x1D700}_{ij}^{\prime }$
at
$\unicode[STIX]{x1D700}_{ij}^{\prime }$
at
![]() $r\rightarrow 0$
,
$r\rightarrow 0$
,
![]() $\unicode[STIX]{x1D700}_{[4]}^{2}$
approaches the value
$\unicode[STIX]{x1D700}_{[4]}^{2}$
approaches the value
![]() $8\langle \unicode[STIX]{x1D700}_{ii}^{2}\rangle +16\langle \unicode[STIX]{x1D700}_{ij}^{2}\rangle$
and balances the viscous term there, as seen in figure 2. The quantity
$8\langle \unicode[STIX]{x1D700}_{ii}^{2}\rangle +16\langle \unicode[STIX]{x1D700}_{ij}^{2}\rangle$
and balances the viscous term there, as seen in figure 2. The quantity
![]() $\langle \unicode[STIX]{x1D700}_{ii}^{2}\rangle =\langle \unicode[STIX]{x1D700}^{2}\rangle$
is the second-order moment of the dissipation distribution of
$\langle \unicode[STIX]{x1D700}_{ii}^{2}\rangle =\langle \unicode[STIX]{x1D700}^{2}\rangle$
is the second-order moment of the dissipation distribution of
![]() $\unicode[STIX]{x1D700}$
. It is a sum of dissipation parameters that appear in the set of successive equations presented in the archive. It is worth noting that the
$\unicode[STIX]{x1D700}$
. It is a sum of dissipation parameters that appear in the set of successive equations presented in the archive. It is worth noting that the
![]() $\unicode[STIX]{x1D700}^{2}$
-term in figure 2 starts as a constant for small values of
$\unicode[STIX]{x1D700}^{2}$
-term in figure 2 starts as a constant for small values of
![]() $r$
, then decreases slightly and becomes constant again at the large scales. In the latter range,
$r$
, then decreases slightly and becomes constant again at the large scales. In the latter range,
![]() $2\langle (\unicode[STIX]{x1D700}_{ii}+\unicode[STIX]{x1D700}_{ii}^{\prime })^{2}\rangle +4\langle (\unicode[STIX]{x1D700}_{ij}+\unicode[STIX]{x1D700}_{ij}^{\prime })^{2}\rangle$
approaches the asymptotic limit
$2\langle (\unicode[STIX]{x1D700}_{ii}+\unicode[STIX]{x1D700}_{ii}^{\prime })^{2}\rangle +4\langle (\unicode[STIX]{x1D700}_{ij}+\unicode[STIX]{x1D700}_{ij}^{\prime })^{2}\rangle$
approaches the asymptotic limit
![]() $4\langle \unicode[STIX]{x1D700}_{ii}^{2}\rangle +8\langle \unicode[STIX]{x1D700}_{ij}^{2}\rangle$
; i.e. it differs from the value for
$4\langle \unicode[STIX]{x1D700}_{ii}^{2}\rangle +8\langle \unicode[STIX]{x1D700}_{ij}^{2}\rangle$
; i.e. it differs from the value for
![]() $r\rightarrow 0$
only by a constant factor of
$r\rightarrow 0$
only by a constant factor of
![]() $2$
. That is, the
$2$
. That is, the
![]() $\unicode[STIX]{x1D700}^{2}$
-term links the remaining terms of (2.13) at the very large and very small scales, similarly to
$\unicode[STIX]{x1D700}^{2}$
-term links the remaining terms of (2.13) at the very large and very small scales, similarly to
![]() $\langle \unicode[STIX]{x1D700}\rangle$
at the second order. In the following, we are mostly interested in the influence of the
$\langle \unicode[STIX]{x1D700}\rangle$
at the second order. In the following, we are mostly interested in the influence of the
![]() $\unicode[STIX]{x1D700}^{2}$
-term, since this term is closest to the classical picture of dissipative fluctuations in turbulent flows in the spirit of Kolmogorov.
$\unicode[STIX]{x1D700}^{2}$
-term, since this term is closest to the classical picture of dissipative fluctuations in turbulent flows in the spirit of Kolmogorov.
3 Relations between the trace of fourth-order structure functions
 $\mathit{S}_{[4]}$
and
$\mathit{S}_{[4]}$
and
 $\langle \unicode[STIX]{x1D700}_{[4]}^{2}\rangle$
in the viscous range
$\langle \unicode[STIX]{x1D700}_{[4]}^{2}\rangle$
in the viscous range
First, we examine the equations outlined above in the viscous range, before we look at the inertial range in the next section. We find that for
![]() $r\rightarrow 0$
,
$r\rightarrow 0$
,
![]() $\mathit{S}_{[4]}$
is exactly determined by
$\mathit{S}_{[4]}$
is exactly determined by
![]() $\unicode[STIX]{x1D700}_{[4]}^{2}$
,
$\unicode[STIX]{x1D700}_{[4]}^{2}$
,
![]() $\unicode[STIX]{x1D708}$
and
$\unicode[STIX]{x1D708}$
and
![]() $r$
.
$r$
.
In the viscous range, the transport term of the fourth-order structure function (2.4) as well as the pressure source terms are proportional to
![]() $r^{4}$
for
$r^{4}$
for
![]() $r\rightarrow 0$
, while the viscous and the dissipative source terms are proportional to
$r\rightarrow 0$
, while the viscous and the dissipative source terms are proportional to
![]() $r^{2}$
. Therefore, to leading order the transport and pressure source terms may be neglected in the viscous range and (2.4) simplifies to
$r^{2}$
. Therefore, to leading order the transport and pressure source terms may be neglected in the viscous range and (2.4) simplifies to
Similarly, from the equation for
![]() $\langle E_{[4]}\rangle$
, the viscous and
$\langle E_{[4]}\rangle$
, the viscous and
![]() $\unicode[STIX]{x1D700}^{2}$
-term balance,
$\unicode[STIX]{x1D700}^{2}$
-term balance,
where
![]() $\unicode[STIX]{x1D700}_{[4]}^{2}$
is constant in the viscous range. Solving (3.2) and inserting the result into (3.1) then gives
$\unicode[STIX]{x1D700}_{[4]}^{2}$
is constant in the viscous range. Solving (3.2) and inserting the result into (3.1) then gives
Since
![]() $\mathit{S}_{[4]}$
is the sum of
$\mathit{S}_{[4]}$
is the sum of
![]() $S_{4,0}$
,
$S_{4,0}$
,
![]() $S_{2,2}$
and
$S_{2,2}$
and
![]() $S_{0,4}$
, all of which are also proportional to
$S_{0,4}$
, all of which are also proportional to
![]() $r^{4}$
for
$r^{4}$
for
![]() $r\rightarrow 0$
, the individual structure functions are also determined by
$r\rightarrow 0$
, the individual structure functions are also determined by
![]() $\unicode[STIX]{x1D700}_{[4]}^{2}$
in the viscous range. In particular, by expanding the structure functions as
$\unicode[STIX]{x1D700}_{[4]}^{2}$
in the viscous range. In particular, by expanding the structure functions as
one can relate
![]() $\unicode[STIX]{x1D700}_{[4]}^{2}/\unicode[STIX]{x1D708}^{2}$
to the sum of
$\unicode[STIX]{x1D700}_{[4]}^{2}/\unicode[STIX]{x1D708}^{2}$
to the sum of
![]() $F_{1}$
,
$F_{1}$
,
![]() $F_{2}$
and
$F_{2}$
and
![]() $F_{3}$
. Siggia (Reference Siggia1981) has identified the four invariants of the fourth-order velocity gradient tensor as
$F_{3}$
. Siggia (Reference Siggia1981) has identified the four invariants of the fourth-order velocity gradient tensor as
where
![]() $\mathit{s}_{ij}=(\unicode[STIX]{x2202}u_{i}/\unicode[STIX]{x2202}x_{j}+\unicode[STIX]{x2202}u_{j}/\unicode[STIX]{x2202}x_{i})/2$
is the rate of strain tensor and the vorticity
$\mathit{s}_{ij}=(\unicode[STIX]{x2202}u_{i}/\unicode[STIX]{x2202}x_{j}+\unicode[STIX]{x2202}u_{j}/\unicode[STIX]{x2202}x_{i})/2$
is the rate of strain tensor and the vorticity
![]() $\unicode[STIX]{x1D714}_{i}=\unicode[STIX]{x1D716}_{ijk}(\unicode[STIX]{x2202}u_{k}/\unicode[STIX]{x2202}x_{j})$
, where
$\unicode[STIX]{x1D714}_{i}=\unicode[STIX]{x1D716}_{ijk}(\unicode[STIX]{x2202}u_{k}/\unicode[STIX]{x2202}x_{j})$
, where
![]() $\unicode[STIX]{x1D716}_{ijk}$
is the Levi-Civita symbol. Siggia then derived
$\unicode[STIX]{x1D716}_{ijk}$
is the Levi-Civita symbol. Siggia then derived
resulting in
Since
cf. (C 20) in appendix C, the trace
![]() $\unicode[STIX]{x1D700}_{[4]}^{2}$
is related in the viscous range to the dissipation parameters
$\unicode[STIX]{x1D700}_{[4]}^{2}$
is related in the viscous range to the dissipation parameters
A similar analysis can be carried out for higher even orders in the viscous range. However, there are more intermediate equations linking the higher-order moments of the dissipation to the higher-order dissipative source term and ultimately to the structure function; cf. the archive for the sixth-order equations. For instance, one then finds for the sixth order
![]() $\mathit{S}_{[6]}\sim r^{6}\unicode[STIX]{x1D700}_{[6]}^{3}/\unicode[STIX]{x1D708}^{3}$
in the viscous range, with two intermediate equations.
$\mathit{S}_{[6]}\sim r^{6}\unicode[STIX]{x1D700}_{[6]}^{3}/\unicode[STIX]{x1D708}^{3}$
in the viscous range, with two intermediate equations.
4 Relation between dissipation fluctuations and inertial-range scaling exponents
In the inertial range, Kolmogorov (Reference Kolmogorov1962) included dissipation fluctuations by using a locally averaged dissipation
![]() $\unicode[STIX]{x1D700}_{r}$
as the structure function scaling parameter instead of only the mean value
$\unicode[STIX]{x1D700}_{r}$
as the structure function scaling parameter instead of only the mean value
![]() $\langle \unicode[STIX]{x1D700}\rangle$
. One would therefore think that
$\langle \unicode[STIX]{x1D700}\rangle$
. One would therefore think that
![]() $\unicode[STIX]{x1D700}_{r}$
must appear in the system of equations. For that reason, we examine in this section a connection between the
$\unicode[STIX]{x1D700}_{r}$
must appear in the system of equations. For that reason, we examine in this section a connection between the
![]() $\unicode[STIX]{x1D700}^{2}$
-term and the second moment of
$\unicode[STIX]{x1D700}^{2}$
-term and the second moment of
![]() $\unicode[STIX]{x1D700}_{r}$
. Therefore, in order to analyse the effect of the dissipation parameter
$\unicode[STIX]{x1D700}_{r}$
. Therefore, in order to analyse the effect of the dissipation parameter
![]() $\unicode[STIX]{x1D700}_{[4]}^{2}$
on the inertial-range scaling exponent
$\unicode[STIX]{x1D700}_{[4]}^{2}$
on the inertial-range scaling exponent
![]() $\unicode[STIX]{x1D701}_{[5]}$
, we must consider the dissipative source term equation. In a first step, we will integrate (2.4) in the inertial range in order to calculate the fifth-order scaling exponent
$\unicode[STIX]{x1D701}_{[5]}$
, we must consider the dissipative source term equation. In a first step, we will integrate (2.4) in the inertial range in order to calculate the fifth-order scaling exponent
![]() $\unicode[STIX]{x1D701}_{[5]}$
implicitly defined by assuming a power law
$\unicode[STIX]{x1D701}_{[5]}$
implicitly defined by assuming a power law
![]() $\mathit{S}_{[5]}\sim r^{\unicode[STIX]{x1D701}_{[5]}}$
. The idea is that because there are equations for the structure functions as well as equations for their source terms, the scaling exponent has to be contained in the system of equations.
$\mathit{S}_{[5]}\sim r^{\unicode[STIX]{x1D701}_{[5]}}$
. The idea is that because there are equations for the structure functions as well as equations for their source terms, the scaling exponent has to be contained in the system of equations.
For some time, there has been an expectation that the connection between the Navier–Stokes equations and the RSH would be discovered one day or, as Kaneda & Morishita (Reference Kaneda, Morishita, Davidson, Kaneda and Sreenivasan2013) proffered, ‘the link between these models and the Navier–Stokes dynamics governing the fluid motion appears still to be missing’. This would require that a correlation exists between
![]() $\unicode[STIX]{x1D700}_{r}$
and the terms representing dissipation fluctuations in the Navier–Stokes equations. In the two-point equations derived systematically from the Navier–Stokes equations in the archive material, from which we derived (2.13) shown above, we have identified the terms describing dissipation fluctuations as
$\unicode[STIX]{x1D700}_{r}$
and the terms representing dissipation fluctuations in the Navier–Stokes equations. In the two-point equations derived systematically from the Navier–Stokes equations in the archive material, from which we derived (2.13) shown above, we have identified the terms describing dissipation fluctuations as
![]() $\unicode[STIX]{x1D700}^{2}$
-terms. They contain moments of the sum of components of the dissipation at two points, for instance the moments
$\unicode[STIX]{x1D700}^{2}$
-terms. They contain moments of the sum of components of the dissipation at two points, for instance the moments
![]() $\langle (\unicode[STIX]{x1D700}+\unicode[STIX]{x1D700}^{\prime })^{m}\rangle$
. An integral expression such as
$\langle (\unicode[STIX]{x1D700}+\unicode[STIX]{x1D700}^{\prime })^{m}\rangle$
. An integral expression such as
where
![]() $V\sim O(r^{3})$
is a volume of dimension
$V\sim O(r^{3})$
is a volume of dimension
![]() $r^{3}$
, or the corresponding one-dimensional expression
$r^{3}$
, or the corresponding one-dimensional expression
where the integral is over any length
![]() $r$
, does not appear directly in these equations.
$r$
, does not appear directly in these equations.
Since
![]() $\unicode[STIX]{x1D700}_{r}$
exhibits a power-law scaling in the inertial range, dimensional analysis suggests that this could be related to the power-law scaling of the structure functions, as Kolmogorov (Reference Kolmogorov1962) had predicted. Stolovitzky, Kailasnath & Sreenivasan (Reference Stolovitzky, Kailasnath and Sreenivasan1992) have performed a test of the relation between
$\unicode[STIX]{x1D700}_{r}$
exhibits a power-law scaling in the inertial range, dimensional analysis suggests that this could be related to the power-law scaling of the structure functions, as Kolmogorov (Reference Kolmogorov1962) had predicted. Stolovitzky, Kailasnath & Sreenivasan (Reference Stolovitzky, Kailasnath and Sreenivasan1992) have performed a test of the relation between
![]() $\unicode[STIX]{x0394}u(r)$
and
$\unicode[STIX]{x0394}u(r)$
and
![]() $(r\unicode[STIX]{x1D700}_{r})^{1/3}$
by investigating the statistical properties of
$(r\unicode[STIX]{x1D700}_{r})^{1/3}$
by investigating the statistical properties of
![]() $\unicode[STIX]{x0394}u(r)/(r\unicode[STIX]{x1D700}_{r})^{1/3}$
. They found that the distribution function of
$\unicode[STIX]{x0394}u(r)/(r\unicode[STIX]{x1D700}_{r})^{1/3}$
. They found that the distribution function of
![]() $\unicode[STIX]{x0394}u(r)/(r\unicode[STIX]{x1D700}_{r})^{1/3}$
is independent of
$\unicode[STIX]{x0394}u(r)/(r\unicode[STIX]{x1D700}_{r})^{1/3}$
is independent of
![]() $r$
and
$r$
and
![]() $\unicode[STIX]{x1D700}_{r}$
in the inertial range and that it is approximately universal. Additional work addressing tests of the RSH is referenced by Sreenivasan & Antonia (Reference Sreenivasan and Antonia1997).
$\unicode[STIX]{x1D700}_{r}$
in the inertial range and that it is approximately universal. Additional work addressing tests of the RSH is referenced by Sreenivasan & Antonia (Reference Sreenivasan and Antonia1997).
On the other hand, properties such as inertial-range scaling exponents should, as a matter of principle, result from the two-point equations. If a power-law scaling can be assumed for the source terms in the structure function equations, the scaling exponents would follow from equations like (4.7) below, to which both the pressure source term and the dissipation source term contribute. For even-order scaling exponents resulting from the odd-order structure function equations, Yakhot (Reference Yakhot2003) has argued that the dissipation source terms can be neglected and has proposed an algebraic closure relating the pressure source terms of arbitrary order to the structure functions. A similar closure could conceivably be developed for the odd-order scaling exponents in the even-order structure function equations. In these equations, the closure would be between the dissipation source terms and structure functions, while the pressure source terms can be neglected. The two-point equations show that dissipation fluctuations are represented by the dissipation parameters appearing in the successive source term equations and not by the source terms in the structure function equations. If one wanted to reconcile the RSH with the two-point equations, one would have to develop a closure between the moments of
![]() $\unicode[STIX]{x1D700}_{r}$
and the dominating source terms in the structure function equations.
$\unicode[STIX]{x1D700}_{r}$
and the dominating source terms in the structure function equations.
It can be shown that the second moment of
![]() $\unicode[STIX]{x1D700}_{r}$
is related to the dissipation correlation
$\unicode[STIX]{x1D700}_{r}$
is related to the dissipation correlation
![]() $\langle \unicode[STIX]{x1D700}\unicode[STIX]{x1D700}^{\prime }\rangle$
under the assumption of homogeneity by
$\langle \unicode[STIX]{x1D700}\unicode[STIX]{x1D700}^{\prime }\rangle$
under the assumption of homogeneity by
cf. Nelkin (Reference Nelkin1994), and similar relations can be found for the higher moments of
![]() $\unicode[STIX]{x1D700}_{r}$
. We only discuss the second moment, because we examine here the fourth-order structure function equations. In particular, we only look at the trace (2.13), but our findings carry over to the individual equations for longitudinal, mixed and transverse dissipative source terms as well. If one assumes a power law for
$\unicode[STIX]{x1D700}_{r}$
. We only discuss the second moment, because we examine here the fourth-order structure function equations. In particular, we only look at the trace (2.13), but our findings carry over to the individual equations for longitudinal, mixed and transverse dissipative source terms as well. If one assumes a power law for
![]() $\langle \unicode[STIX]{x1D700}_{r}^{2}\rangle \sim r^{\unicode[STIX]{x1D6FE}}$
, where the scaling exponent and the prefactor are independent of
$\langle \unicode[STIX]{x1D700}_{r}^{2}\rangle \sim r^{\unicode[STIX]{x1D6FE}}$
, where the scaling exponent and the prefactor are independent of
![]() $r$
, as one is apt to do and which is at the very core of the RSH and similar theories, one also finds by virtue of (4.3) that
$r$
, as one is apt to do and which is at the very core of the RSH and similar theories, one also finds by virtue of (4.3) that
![]() $\langle \unicode[STIX]{x1D700}\unicode[STIX]{x1D700}^{\prime }\rangle \sim r^{\unicode[STIX]{x1D6FE}}$
and hence has the same
$\langle \unicode[STIX]{x1D700}\unicode[STIX]{x1D700}^{\prime }\rangle \sim r^{\unicode[STIX]{x1D6FE}}$
and hence has the same
![]() $r$
-scaling. The first term of the
$r$
-scaling. The first term of the
![]() $\unicode[STIX]{x1D700}^{2}$
-term in (2.22) can be written as the sum of constants and correlations; i.e.
$\unicode[STIX]{x1D700}^{2}$
-term in (2.22) can be written as the sum of constants and correlations; i.e.
In other words, the
![]() $r$
-dependence of
$r$
-dependence of
![]() $\unicode[STIX]{x1D700}_{r}$
is manifested in the
$\unicode[STIX]{x1D700}_{r}$
is manifested in the
![]() $\unicode[STIX]{x1D700}^{2}$
-terms in the dissipative source terms by virtue of (4.3) and (4.4).
$\unicode[STIX]{x1D700}^{2}$
-terms in the dissipative source terms by virtue of (4.3) and (4.4).
We begin with the integration of (2.4) to link the structure function exponent to the source terms. We will neglect the diffusive and unsteady terms in (2.4) and (2.13) and perform an order-of-magnitude estimate of the source terms. If we assume in (2.4) a power-law scaling for the source terms of the form
which is consistent with figure 1, the trace of the fifth-order structure functions
![]() $\mathit{S}_{[5]}$
can be determined from (2.4) by integration. Of course, (4.5) and (4.6) are approximations. However, without these assumptions, a power law of the form
$\mathit{S}_{[5]}$
can be determined from (2.4) by integration. Of course, (4.5) and (4.6) are approximations. However, without these assumptions, a power law of the form
![]() $\mathit{S}_{[5]}=C_{[5]}r^{\unicode[STIX]{x1D701}_{[5]}}$
is not compatible with (2.4). In other words, only if the source terms follow a power law in the inertial range, do the structure functions as well. The range of integration will be divided into two parts. The first part ranges from
$\mathit{S}_{[5]}=C_{[5]}r^{\unicode[STIX]{x1D701}_{[5]}}$
is not compatible with (2.4). In other words, only if the source terms follow a power law in the inertial range, do the structure functions as well. The range of integration will be divided into two parts. The first part ranges from
![]() $r=0$
to
$r=0$
to
![]() $r^{\ast }\approx 30\unicode[STIX]{x1D702}$
, where the power law in the inertial range starts to be valid. The second part ranges from there on to the value
$r^{\ast }\approx 30\unicode[STIX]{x1D702}$
, where the power law in the inertial range starts to be valid. The second part ranges from there on to the value
![]() $r$
of interest in the inertial range. The integration then yields
$r$
of interest in the inertial range. The integration then yields
 $$\begin{eqnarray}\displaystyle -\!\mathit{S}_{[5]} & = & \displaystyle \frac{1}{r^{2}}\int _{0}^{r^{\ast }}r^{2}(\langle T_{[4]}\rangle +\langle E_{[4]}\rangle )\,\text{d}r-\frac{1}{r^{2}}\left\{\frac{A_{[4]}^{T}r^{3+\unicode[STIX]{x1D709}_{[4]}^{T}}}{3+\unicode[STIX]{x1D709}_{[4]}^{T}}+\frac{A_{[4]}^{E}r^{3+\unicode[STIX]{x1D709}_{[4]}^{E}}}{3+\unicode[STIX]{x1D709}_{[4]}^{E}}\right\}_{r=r^{\ast }}\nonumber\\ \displaystyle & & \displaystyle +\,\frac{A_{[4]}^{T}r^{1+\unicode[STIX]{x1D709}_{[4]}^{T}}}{3+\unicode[STIX]{x1D709}_{[4]}^{T}}+\frac{A_{[4]}^{E}r^{1+\unicode[STIX]{x1D709}_{[4]}^{E}}}{3+\unicode[STIX]{x1D709}_{[4]}^{E}}.\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle -\!\mathit{S}_{[5]} & = & \displaystyle \frac{1}{r^{2}}\int _{0}^{r^{\ast }}r^{2}(\langle T_{[4]}\rangle +\langle E_{[4]}\rangle )\,\text{d}r-\frac{1}{r^{2}}\left\{\frac{A_{[4]}^{T}r^{3+\unicode[STIX]{x1D709}_{[4]}^{T}}}{3+\unicode[STIX]{x1D709}_{[4]}^{T}}+\frac{A_{[4]}^{E}r^{3+\unicode[STIX]{x1D709}_{[4]}^{E}}}{3+\unicode[STIX]{x1D709}_{[4]}^{E}}\right\}_{r=r^{\ast }}\nonumber\\ \displaystyle & & \displaystyle +\,\frac{A_{[4]}^{T}r^{1+\unicode[STIX]{x1D709}_{[4]}^{T}}}{3+\unicode[STIX]{x1D709}_{[4]}^{T}}+\frac{A_{[4]}^{E}r^{1+\unicode[STIX]{x1D709}_{[4]}^{E}}}{3+\unicode[STIX]{x1D709}_{[4]}^{E}}.\end{eqnarray}$$
Since the diffusion terms have been neglected from the very beginning, the first integral is incomplete. The purpose here is to show that this and the second term on the right-hand side of (4.7) can be neglected for large
![]() $r\gg r^{\ast }$
because of the term
$r\gg r^{\ast }$
because of the term
![]() $r^{-2}$
in front of them. Then, only the last two terms on the right-hand side of (4.7) remain, and we find the trace of the structure functions to be given as a weighted sum of the two power laws (4.5) and (4.6). More specifically, there are several possible contributions to the inertial range that have been neglected: the integral from 0 to
$r^{-2}$
in front of them. Then, only the last two terms on the right-hand side of (4.7) remain, and we find the trace of the structure functions to be given as a weighted sum of the two power laws (4.5) and (4.6). More specifically, there are several possible contributions to the inertial range that have been neglected: the integral from 0 to
![]() $r$
over the viscous term might not be negligible in the inertial range at
$r$
over the viscous term might not be negligible in the inertial range at
![]() $r$
; the integral of the dissipative and pressure source terms over 0 to
$r$
; the integral of the dissipative and pressure source terms over 0 to
![]() $r^{\ast }$
might not be negligible in the inertial range at some
$r^{\ast }$
might not be negligible in the inertial range at some
![]() $r$
; the value of the transport term at
$r$
; the value of the transport term at
![]() $r=r^{\ast }$
might not be small compared with its inertial-range value. Additionally, because
$r=r^{\ast }$
might not be small compared with its inertial-range value. Additionally, because
![]() $\unicode[STIX]{x1D702}$
is not the correct dissipative length scale for the fourth-order equations,
$\unicode[STIX]{x1D702}$
is not the correct dissipative length scale for the fourth-order equations,
![]() $r^{\ast }/\unicode[STIX]{x1D702}$
is dependent on the Reynolds number, and this dependence is required to be small. While the importance of some of these contributions may already be estimated from the balance figure 1, it is more advantageous to look at the integrated balance, in the spirit of (4.7) and the present section. For that reason, the numerical integration of (2.4) over
$r^{\ast }/\unicode[STIX]{x1D702}$
is dependent on the Reynolds number, and this dependence is required to be small. While the importance of some of these contributions may already be estimated from the balance figure 1, it is more advantageous to look at the integrated balance, in the spirit of (4.7) and the present section. For that reason, the numerical integration of (2.4) over
![]() $r$
is presented in figure 3. The integrated balances for the data sets
$r$
is presented in figure 3. The integrated balances for the data sets
![]() $R0$
(
$R0$
(
![]() $Re_{\unicode[STIX]{x1D706}}=88$
) and
$Re_{\unicode[STIX]{x1D706}}=88$
) and
![]() $R5$
(
$R5$
(
![]() $Re_{\unicode[STIX]{x1D706}}=529$
) normalized by
$Re_{\unicode[STIX]{x1D706}}=529$
) normalized by
![]() $\langle \unicode[STIX]{x1D700}^{2}\rangle$
and
$\langle \unicode[STIX]{x1D700}^{2}\rangle$
and
![]() $\unicode[STIX]{x1D708}$
are plotted over
$\unicode[STIX]{x1D708}$
are plotted over
![]() $r/\unicode[STIX]{x1D702}$
. Indeed, the integrated viscous terms are negligible compared with all other integrated terms in the inertial range after
$r/\unicode[STIX]{x1D702}$
. Indeed, the integrated viscous terms are negligible compared with all other integrated terms in the inertial range after
![]() $r/\unicode[STIX]{x1D702}=30$
. Moreover, the integrated dissipative source term, pressure source term and transport term are much smaller in the viscous range than in the inertial range. This holds for all data sets and not only the cases
$r/\unicode[STIX]{x1D702}=30$
. Moreover, the integrated dissipative source term, pressure source term and transport term are much smaller in the viscous range than in the inertial range. This holds for all data sets and not only the cases
![]() $R0$
and
$R0$
and
![]() $R5$
shown in figure 3(a). Last, as shown in figure 3(b), both the integrated dissipative source terms and the integrated viscous terms cross over to the inertial range at approximately the same value of
$R5$
shown in figure 3(a). Last, as shown in figure 3(b), both the integrated dissipative source terms and the integrated viscous terms cross over to the inertial range at approximately the same value of
![]() $r/\unicode[STIX]{x1D702}$
for the data sets analysed here, i.e. the true viscous cutoff length scale depends only weakly on the Reynolds number. Therefore, the simplifications with regard to the integration carried out in (4.7) as described above seem to be justifiable.
$r/\unicode[STIX]{x1D702}$
for the data sets analysed here, i.e. the true viscous cutoff length scale depends only weakly on the Reynolds number. Therefore, the simplifications with regard to the integration carried out in (4.7) as described above seem to be justifiable.

Figure 3. (a) Terms of (2.4) numerically integrated over
![]() $r$
for cases
$r$
for cases
![]() $R0$
(grey) and
$R0$
(grey) and
![]() $R5$
(black), where ○ is the transport term, ▫ is the dissipative source term, ▿ is the pressure source term and ▵ is the viscous term. The integrated dissipative source term ▫ and integrated viscous terms
$R5$
(black), where ○ is the transport term, ▫ is the dissipative source term, ▿ is the pressure source term and ▵ is the viscous term. The integrated dissipative source term ▫ and integrated viscous terms
![]() $\triangle$
for all cases
$\triangle$
for all cases
![]() $R0$
–
$R0$
–
![]() $R5$
(from light grey to black) are shown in (b).
$R5$
(from light grey to black) are shown in (b).
The sum of two power laws with constant prefactor and exponent does not give a power law with constant prefactor and exponent. Only if
![]() $\unicode[STIX]{x1D709}_{[4]}^{T}=\unicode[STIX]{x1D709}_{[4]}^{E}$
or if one of the two terms is much smaller than the other one will the scaling of
$\unicode[STIX]{x1D709}_{[4]}^{T}=\unicode[STIX]{x1D709}_{[4]}^{E}$
or if one of the two terms is much smaller than the other one will the scaling of
![]() $\mathit{S}_{[5]}$
result in an (approximate) power law. We will explore the second possibility by approximating the scaling exponent
$\mathit{S}_{[5]}$
result in an (approximate) power law. We will explore the second possibility by approximating the scaling exponent
![]() $\unicode[STIX]{x1D701}_{[5]}$
by neglecting the pressure source term (cf. figure 1, where the pressure source term is smaller than the dissipative source terms by a factor of four, and also figure 3), resulting in
$\unicode[STIX]{x1D701}_{[5]}$
by neglecting the pressure source term (cf. figure 1, where the pressure source term is smaller than the dissipative source terms by a factor of four, and also figure 3), resulting in
This relationship between
![]() $\unicode[STIX]{x1D701}_{[5]}$
and
$\unicode[STIX]{x1D701}_{[5]}$
and
![]() $\unicode[STIX]{x1D709}_{[4]}^{E}$
is consistent with fusion rules, cf. L’vov & Procaccia (Reference L’vov and Procaccia1996a
) and Benzi et al. (Reference Benzi, Biferale and Toschi1998), where the same relation for the fifth-order structure function is provided for the case when one of the two separation distances is in the dissipative and the other in the inertial range. Here, (4.8) follows directly from neglecting the pressure source term. We have calculated the scaling exponent
$\unicode[STIX]{x1D709}_{[4]}^{E}$
is consistent with fusion rules, cf. L’vov & Procaccia (Reference L’vov and Procaccia1996a
) and Benzi et al. (Reference Benzi, Biferale and Toschi1998), where the same relation for the fifth-order structure function is provided for the case when one of the two separation distances is in the dissipative and the other in the inertial range. Here, (4.8) follows directly from neglecting the pressure source term. We have calculated the scaling exponent
![]() $\unicode[STIX]{x1D709}_{[4]}^{E}$
of
$\unicode[STIX]{x1D709}_{[4]}^{E}$
of
![]() $\langle E_{[4]}\rangle$
already introduced in (4.6) for all of our DNS cases by using
$\langle E_{[4]}\rangle$
already introduced in (4.6) for all of our DNS cases by using
As seen from figure 4,
![]() $\unicode[STIX]{x1D709}_{[4]}^{E}=0.56$
in the inertial range for our data sets
$\unicode[STIX]{x1D709}_{[4]}^{E}=0.56$
in the inertial range for our data sets
![]() $R1$
–
$R1$
–
![]() $R6$
. The trace of the fifth-order structure functions
$R6$
. The trace of the fifth-order structure functions
![]() $\mathit{S}_{[5]}$
is shown in figure 5 in compensated form; i.e. divided by
$\mathit{S}_{[5]}$
is shown in figure 5 in compensated form; i.e. divided by
![]() $(r/\unicode[STIX]{x1D702})^{1.56}$
and normalized by
$(r/\unicode[STIX]{x1D702})^{1.56}$
and normalized by
![]() $\unicode[STIX]{x1D708}$
and
$\unicode[STIX]{x1D708}$
and
![]() $\langle \unicode[STIX]{x1D700}^{2}\rangle$
. Since
$\langle \unicode[STIX]{x1D700}^{2}\rangle$
. Since
![]() $\langle \unicode[STIX]{x1D700}^{2}\rangle$
represents the dissipation parameters at the fourth-order level,
$\langle \unicode[STIX]{x1D700}^{2}\rangle$
represents the dissipation parameters at the fourth-order level,
![]() $\langle \unicode[STIX]{x1D700}^{2}\rangle$
provides indeed a better scaling than
$\langle \unicode[STIX]{x1D700}^{2}\rangle$
provides indeed a better scaling than
![]() $\langle \unicode[STIX]{x1D700}\rangle$
for quantities at that level. We plot the scaling exponents
$\langle \unicode[STIX]{x1D700}\rangle$
for quantities at that level. We plot the scaling exponents
![]() $\unicode[STIX]{x1D701}_{[5]}$
in figure 5, as computed by
$\unicode[STIX]{x1D701}_{[5]}$
in figure 5, as computed by
where again implicitly a power law for
![]() $\mathit{S}_{[5]}$
with constant prefactors and exponents is assumed. The dashed black horizontal line indicates the value
$\mathit{S}_{[5]}$
with constant prefactors and exponents is assumed. The dashed black horizontal line indicates the value
![]() $\unicode[STIX]{x1D701}_{[5]}=1.56$
.
$\unicode[STIX]{x1D701}_{[5]}=1.56$
.

Figure 4. Scaling exponent
![]() $\unicode[STIX]{x1D709}_{[4]}^{E}$
for the cases
$\unicode[STIX]{x1D709}_{[4]}^{E}$
for the cases
![]() $R1$
–
$R1$
–
![]() $R6$
with
$R6$
with
![]() $\mathit{Re}_{\unicode[STIX]{x1D706}}$
ranging from 119 to 754 (higher Reynolds numbers indicated by darker shading). The dashed black horizontal line indicates
$\mathit{Re}_{\unicode[STIX]{x1D706}}$
ranging from 119 to 754 (higher Reynolds numbers indicated by darker shading). The dashed black horizontal line indicates
![]() $\unicode[STIX]{x1D709}_{[4]}^{E}=0.56$
.
$\unicode[STIX]{x1D709}_{[4]}^{E}=0.56$
.

Figure 5. Compensated structure function
![]() $\mathit{S}_{[5]}$
in the inertial range for the cases
$\mathit{S}_{[5]}$
in the inertial range for the cases
![]() $R0$
–
$R0$
–
![]() $R6$
with
$R6$
with
![]() $Re_{\unicode[STIX]{x1D706}}$
ranging from 88 to 754 (a) and scaling exponent
$Re_{\unicode[STIX]{x1D706}}$
ranging from 88 to 754 (a) and scaling exponent
![]() $\unicode[STIX]{x1D701}_{[5]}$
(b). Higher Reynolds numbers are indicated by darker shading. The horizontal dashed black line in (b) indicates
$\unicode[STIX]{x1D701}_{[5]}$
(b). Higher Reynolds numbers are indicated by darker shading. The horizontal dashed black line in (b) indicates
![]() $\unicode[STIX]{x1D701}_{[5]}=1.56$
.
$\unicode[STIX]{x1D701}_{[5]}=1.56$
.
In the next step, a relation between
![]() $\unicode[STIX]{x1D709}_{[4]}^{E}$
and the
$\unicode[STIX]{x1D709}_{[4]}^{E}$
and the
![]() $\unicode[STIX]{x1D700}^{2}$
-term is needed. Therefore, we need to look at the transport equation of the dissipative source term
$\unicode[STIX]{x1D700}^{2}$
-term is needed. Therefore, we need to look at the transport equation of the dissipative source term
![]() $\langle E_{[4]}\rangle$
in more detail. However, under the assumption of stationarity, there is no term containing
$\langle E_{[4]}\rangle$
in more detail. However, under the assumption of stationarity, there is no term containing
![]() $\langle E_{[4]}\rangle$
in its transport equation when the inertial-range assumptions are invoked, as both the unsteady and the viscous terms are neglected. That is, information is lost by averaging, and, hence, additional assumptions and closures are needed. The situation is similar to the second-order structure function equations, where one finds in the inertial range a solution for the third-order structure functions (i.e. the
$\langle E_{[4]}\rangle$
in its transport equation when the inertial-range assumptions are invoked, as both the unsteady and the viscous terms are neglected. That is, information is lost by averaging, and, hence, additional assumptions and closures are needed. The situation is similar to the second-order structure function equations, where one finds in the inertial range a solution for the third-order structure functions (i.e. the
![]() $4/5$
law), but all connections to the second order are lost. If one is interested in the solution of the second order in the inertial range, one has to make additional assumptions such as Kolmogorov’s constant-skewness assumption.
$4/5$
law), but all connections to the second order are lost. If one is interested in the solution of the second order in the inertial range, one has to make additional assumptions such as Kolmogorov’s constant-skewness assumption.
That is, we would now need a relation between
![]() $\langle \unicode[STIX]{x0394}u_{1}E_{[4]}\rangle$
and
$\langle \unicode[STIX]{x0394}u_{1}E_{[4]}\rangle$
and
![]() $\langle E_{[4]}\rangle$
, e.g. by employing a gradient flux ansatz. In the following, we rather use a slightly different approach. In analogy to K41 and K62 theory, one might postulate that fluctuations of the dissipation represent the relevant scaling parameter in the inertial range. Since the transport equation for
$\langle E_{[4]}\rangle$
, e.g. by employing a gradient flux ansatz. In the following, we rather use a slightly different approach. In analogy to K41 and K62 theory, one might postulate that fluctuations of the dissipation represent the relevant scaling parameter in the inertial range. Since the transport equation for
![]() $\langle E_{[4]}\rangle$
contains the
$\langle E_{[4]}\rangle$
contains the
![]() $\unicode[STIX]{x1D700}^{2}$
-term, which represents dissipation fluctuations, it seems reasonable to scale the dissipative source term with
$\unicode[STIX]{x1D700}^{2}$
-term, which represents dissipation fluctuations, it seems reasonable to scale the dissipative source term with
![]() $\unicode[STIX]{x1D700}_{[4]}^{2}$
and
$\unicode[STIX]{x1D700}_{[4]}^{2}$
and
![]() $r$
. It should be mentioned that the
$r$
. It should be mentioned that the
![]() $r$
-dependence of the
$r$
-dependence of the
![]() $\unicode[STIX]{x1D700}^{2}$
-term is cancelled out by the
$\unicode[STIX]{x1D700}^{2}$
-term is cancelled out by the
![]() $D$
-term in the sum of the source terms. However, neither of the other terms combined with only
$D$
-term in the sum of the source terms. However, neither of the other terms combined with only
![]() $r$
is sufficient to provide a scaling of the dissipative source term without an additional parameter like
$r$
is sufficient to provide a scaling of the dissipative source term without an additional parameter like
![]() $\unicode[STIX]{x1D6FF}_{[4]}^{E}$
, and the same holds for the cancellations of
$\unicode[STIX]{x1D6FF}_{[4]}^{E}$
, and the same holds for the cancellations of
![]() $D_{[4]}+\unicode[STIX]{x1D700}_{[4]}^{2}$
and
$D_{[4]}+\unicode[STIX]{x1D700}_{[4]}^{2}$
and
![]() $F_{[4]}+Q_{[4]}$
. This indicates that some combination of the source terms in (3.1) is needed to scale the dissipative source term and ultimately
$F_{[4]}+Q_{[4]}$
. This indicates that some combination of the source terms in (3.1) is needed to scale the dissipative source term and ultimately
![]() $\mathit{S}_{[5]}$
. Consequently, not only the dissipation fluctuations characterized by the
$\mathit{S}_{[5]}$
. Consequently, not only the dissipation fluctuations characterized by the
![]() $\unicode[STIX]{x1D700}^{2}$
-term are relevant, but also the other source terms in (3.1). However, the necessary combination of source terms cannot be determined by scaling arguments alone, because there are only two dimensions (m) and (s) and more than two scaling quantities. Furthermore, all terms are needed if the prefactor is also of interest.
$\unicode[STIX]{x1D700}^{2}$
-term are relevant, but also the other source terms in (3.1). However, the necessary combination of source terms cannot be determined by scaling arguments alone, because there are only two dimensions (m) and (s) and more than two scaling quantities. Furthermore, all terms are needed if the prefactor is also of interest.
In the following, we proceed to look only at the dissipation fluctuations, because they remain a quantity of high interest. We generalize the scaling of
![]() $\langle E_{[4]}\rangle$
with
$\langle E_{[4]}\rangle$
with
![]() $\unicode[STIX]{x1D700}_{[4]}^{2}$
and
$\unicode[STIX]{x1D700}_{[4]}^{2}$
and
![]() $r$
by adding a prefactor
$r$
by adding a prefactor
![]() $(r/r^{\ast })^{\unicode[STIX]{x1D6FF}_{[4]}^{E}}$
, i.e. we use the ansatz
$(r/r^{\ast })^{\unicode[STIX]{x1D6FF}_{[4]}^{E}}$
, i.e. we use the ansatz
Here,
![]() $r^{\ast \unicode[STIX]{x1D6FF}_{[4]}^{E}}$
is contained in
$r^{\ast \unicode[STIX]{x1D6FF}_{[4]}^{E}}$
is contained in
![]() $c_{[4]}^{E}$
. Of course, this is an ad hoc ansatz and only one of many possibilities. We do not want to claim that this is the best or only way to close the equations; rather, we use it for its simplicity. We use this closure for analytical purposes only, i.e. do not want to make predictions regarding higher orders, other data sets or flows. Because there are other source terms in (2.13) that contribute to the balance, one cannot expect that
$c_{[4]}^{E}$
. Of course, this is an ad hoc ansatz and only one of many possibilities. We do not want to claim that this is the best or only way to close the equations; rather, we use it for its simplicity. We use this closure for analytical purposes only, i.e. do not want to make predictions regarding higher orders, other data sets or flows. Because there are other source terms in (2.13) that contribute to the balance, one cannot expect that
![]() $\unicode[STIX]{x1D6FF}_{[4]}^{E}$
vanishes. Indeed, from our DNS, we find that
$\unicode[STIX]{x1D6FF}_{[4]}^{E}$
vanishes. Indeed, from our DNS, we find that
![]() $\unicode[STIX]{x1D6FF}_{[4]}^{E}=-0.09$
. The numerical values of
$\unicode[STIX]{x1D6FF}_{[4]}^{E}=-0.09$
. The numerical values of
![]() $\unicode[STIX]{x1D6FF}_{[4]}^{E}$
for
$\unicode[STIX]{x1D6FF}_{[4]}^{E}$
for
![]() $R1$
–
$R1$
–
![]() $R6$
are shown in figure 6(a), where
$R6$
are shown in figure 6(a), where
and the dashed horizontal black line corresponds to
![]() $\unicode[STIX]{x1D6FF}_{[4]}^{E}=-0.09$
. This value is empirically determined and not claimed to be universal or to carry over to other flows. Moreover, its exact value is not important in the present context. We will now insert (4.11) into (4.7) and integrate. That is, we now examine the scaling of the dissipative source term
$\unicode[STIX]{x1D6FF}_{[4]}^{E}=-0.09$
. This value is empirically determined and not claimed to be universal or to carry over to other flows. Moreover, its exact value is not important in the present context. We will now insert (4.11) into (4.7) and integrate. That is, we now examine the scaling of the dissipative source term
![]() $\langle E_{[4]}\rangle$
compared with the scaling of the
$\langle E_{[4]}\rangle$
compared with the scaling of the
![]() $\unicode[STIX]{x1D700}^{2}$
-term,
$\unicode[STIX]{x1D700}^{2}$
-term,
![]() $\unicode[STIX]{x1D700}_{[4]}^{2}$
, which contains
$\unicode[STIX]{x1D700}_{[4]}^{2}$
, which contains
![]() $\langle \unicode[STIX]{x1D700}_{r}^{2}\rangle$
. Using the ansatz (4.11), we find that this ratio should scale as
$\langle \unicode[STIX]{x1D700}_{r}^{2}\rangle$
. Using the ansatz (4.11), we find that this ratio should scale as
![]() $r^{2/3+\unicode[STIX]{x1D6FF}_{[4]}^{E}}$
in the inertial range, under the assumption that we may approximate the terms by power laws in the inertial range. The ratio
$r^{2/3+\unicode[STIX]{x1D6FF}_{[4]}^{E}}$
in the inertial range, under the assumption that we may approximate the terms by power laws in the inertial range. The ratio
![]() $\langle E_{[4]}\rangle /(\unicode[STIX]{x1D700}_{[4]}^{2}r^{2/3+\unicode[STIX]{x1D6FF}_{[4]}^{E}})$
is shown in figure 6(b) for the cases
$\langle E_{[4]}\rangle /(\unicode[STIX]{x1D700}_{[4]}^{2}r^{2/3+\unicode[STIX]{x1D6FF}_{[4]}^{E}})$
is shown in figure 6(b) for the cases
![]() $R1$
–
$R1$
–
![]() $R6$
. We find a scaling range in the inertial range. In short, we have now replaced the dissipative source terms with
$R6$
. We find a scaling range in the inertial range. In short, we have now replaced the dissipative source terms with
![]() $\unicode[STIX]{x1D700}_{[4]}^{2}r^{2/3+\unicode[STIX]{x1D6FF}_{[4]}^{E}}$
, which has the same
$\unicode[STIX]{x1D700}_{[4]}^{2}r^{2/3+\unicode[STIX]{x1D6FF}_{[4]}^{E}}$
, which has the same
![]() $r$
-scaling in the inertial range. If the
$r$
-scaling in the inertial range. If the
![]() $\unicode[STIX]{x1D700}^{2}$
-term has a power-law scaling in the inertial range, we can now integrate to obtain for the structure function trace
$\unicode[STIX]{x1D700}^{2}$
-term has a power-law scaling in the inertial range, we can now integrate to obtain for the structure function trace
This should be compared with the RSH, for which
That is, from the system of equations we would rather have a contribution from
![]() $\langle \unicode[STIX]{x1D700}_{r}^{2}\rangle$
, which is contained in
$\langle \unicode[STIX]{x1D700}_{r}^{2}\rangle$
, which is contained in
![]() $\unicode[STIX]{x1D700}_{[4]}^{2}$
, instead of
$\unicode[STIX]{x1D700}_{[4]}^{2}$
, instead of
![]() $\langle \unicode[STIX]{x1D700}_{r}^{5/3}\rangle$
. As the RSH gives satisfactory results when compared with experimental data, the differences have to be contained in the parameter
$\langle \unicode[STIX]{x1D700}_{r}^{5/3}\rangle$
. As the RSH gives satisfactory results when compared with experimental data, the differences have to be contained in the parameter
![]() $\unicode[STIX]{x1D6FF}_{[4]}^{E}$
, which contains empirically the influence of the other source terms in (3.1) on the dissipative source term
$\unicode[STIX]{x1D6FF}_{[4]}^{E}$
, which contains empirically the influence of the other source terms in (3.1) on the dissipative source term
![]() $\langle E_{[4]}\rangle$
. It should be noted that (4.14) implies
$\langle E_{[4]}\rangle$
. It should be noted that (4.14) implies
![]() $\langle E_{[4]}\rangle \sim r^{2/3}\langle \unicode[STIX]{x1D700}_{r}^{5/3}\rangle$
, if a power law for
$\langle E_{[4]}\rangle \sim r^{2/3}\langle \unicode[STIX]{x1D700}_{r}^{5/3}\rangle$
, if a power law for
![]() $\unicode[STIX]{x1D700}_{r}$
can be assumed. However, there is no term containing
$\unicode[STIX]{x1D700}_{r}$
can be assumed. However, there is no term containing
![]() $\langle \unicode[STIX]{x1D700}_{r}^{5/3}\rangle$
in either the fourth- or the fifth-order equations.
$\langle \unicode[STIX]{x1D700}_{r}^{5/3}\rangle$
in either the fourth- or the fifth-order equations.

Figure 6. The term
![]() $\unicode[STIX]{x1D6FF}_{[4]}^{E}$
as evaluated by (4.12) for
$\unicode[STIX]{x1D6FF}_{[4]}^{E}$
as evaluated by (4.12) for
![]() $R1$
–
$R1$
–
![]() $R6$
(a). Plot of the ratio
$R6$
(a). Plot of the ratio
![]() $\langle E_{[4]}\rangle /(\unicode[STIX]{x1D700}_{[4]}^{2}r^{2/3-\unicode[STIX]{x1D6FF}_{[4]}^{E}})$
with
$\langle E_{[4]}\rangle /(\unicode[STIX]{x1D700}_{[4]}^{2}r^{2/3-\unicode[STIX]{x1D6FF}_{[4]}^{E}})$
with
![]() $\unicode[STIX]{x1D6FF}_{[4]}^{E}=-0.09$
(b). Higher Reynolds numbers are indicated by darker shading.
$\unicode[STIX]{x1D6FF}_{[4]}^{E}=-0.09$
(b). Higher Reynolds numbers are indicated by darker shading.
We may conclude that the RSH assumption of using
![]() $\unicode[STIX]{x1D700}_{r}$
instead of the K41 ansatz using
$\unicode[STIX]{x1D700}_{r}$
instead of the K41 ansatz using
![]() $\langle \unicode[STIX]{x1D700}\rangle$
is compatible with the results of our approach here, in the sense that
$\langle \unicode[STIX]{x1D700}\rangle$
is compatible with the results of our approach here, in the sense that
![]() $\unicode[STIX]{x1D700}_{r}$
appears in the
$\unicode[STIX]{x1D700}_{r}$
appears in the
![]() $\unicode[STIX]{x1D700}^{2}$
-term. However, we find in the system of equations a contribution to the fifth-order structure functions by
$\unicode[STIX]{x1D700}^{2}$
-term. However, we find in the system of equations a contribution to the fifth-order structure functions by
![]() $\langle \unicode[STIX]{x1D700}_{r}^{2}\rangle$
; i.e. the connection to
$\langle \unicode[STIX]{x1D700}_{r}^{2}\rangle$
; i.e. the connection to
![]() $\langle \unicode[STIX]{x1D700}_{r}^{n/3}\rangle$
is still missing. We expect similar results at higher orders, which should then be related to higher moments of
$\langle \unicode[STIX]{x1D700}_{r}^{n/3}\rangle$
is still missing. We expect similar results at higher orders, which should then be related to higher moments of
![]() $\unicode[STIX]{x1D700}_{r}$
. However, the pressure source terms also might be important at higher orders, which one would then need to close as well. Furthermore, we find that the
$\unicode[STIX]{x1D700}_{r}$
. However, the pressure source terms also might be important at higher orders, which one would then need to close as well. Furthermore, we find that the
![]() $r$
-scaling of the
$r$
-scaling of the
![]() $\unicode[STIX]{x1D700}^{2}$
-term is cancelled out by the
$\unicode[STIX]{x1D700}^{2}$
-term is cancelled out by the
![]() $D$
-term in the full system of equations. We are left with the fact that while we have found all higher moments of
$D$
-term in the full system of equations. We are left with the fact that while we have found all higher moments of
![]() $\unicode[STIX]{x1D700}_{r}$
in the dissipative source term equations and further equations derived therefrom, we cannot say why
$\unicode[STIX]{x1D700}_{r}$
in the dissipative source term equations and further equations derived therefrom, we cannot say why
![]() $n$
th-order structure functions should be determined by
$n$
th-order structure functions should be determined by
![]() $\langle \unicode[STIX]{x1D700}_{r}^{n/3}\rangle$
, i.e. by the RSH or other similar theories. While the numerical values obtained in either way agree fairly well (at least for the fourth order we examined here), such a connection cannot be obtained from the Navier–Stokes equations without additional empirical closures.
$\langle \unicode[STIX]{x1D700}_{r}^{n/3}\rangle$
, i.e. by the RSH or other similar theories. While the numerical values obtained in either way agree fairly well (at least for the fourth order we examined here), such a connection cannot be obtained from the Navier–Stokes equations without additional empirical closures.
The analysis can be carried to higher orders. It can easily be shown that the third successive equation for dissipation source terms in the trace of the eighth-order structure function equations contains a term
![]() $\langle (\unicode[STIX]{x1D700}_{ii}+\unicode[STIX]{x1D700}_{ii}^{\prime })(\unicode[STIX]{x1D700}_{jj}+\unicode[STIX]{x1D700}_{jj}^{\prime })(\unicode[STIX]{x1D700}_{kk}+\unicode[STIX]{x1D700}_{kk}^{\prime })(\unicode[STIX]{x1D700}_{ll}+\unicode[STIX]{x1D700}_{ll}^{\prime })\rangle$
, which generates a dissipation parameter
$\langle (\unicode[STIX]{x1D700}_{ii}+\unicode[STIX]{x1D700}_{ii}^{\prime })(\unicode[STIX]{x1D700}_{jj}+\unicode[STIX]{x1D700}_{jj}^{\prime })(\unicode[STIX]{x1D700}_{kk}+\unicode[STIX]{x1D700}_{kk}^{\prime })(\unicode[STIX]{x1D700}_{ll}+\unicode[STIX]{x1D700}_{ll}^{\prime })\rangle$
, which generates a dissipation parameter
![]() $\langle \unicode[STIX]{x1D700}^{4}\rangle$
. As one continues further, one will find all moments of the dissipation distribution function in the system of averaged equations. On the basis of the equations at the sixth-order structure function level, for instance, one could perform similar integrations to those for the fourth-order level. For instance, in the archive material, we have derived an equation for the dissipation source term
$\langle \unicode[STIX]{x1D700}^{4}\rangle$
. As one continues further, one will find all moments of the dissipation distribution function in the system of averaged equations. On the basis of the equations at the sixth-order structure function level, for instance, one could perform similar integrations to those for the fourth-order level. For instance, in the archive material, we have derived an equation for the dissipation source term
![]() $\langle E_{6,0}\rangle =15\langle (\unicode[STIX]{x0394}u_{1})^{4}(\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })\rangle$
which appears in the sixth-order longitudinal structure function equation. In the equation for
$\langle E_{6,0}\rangle =15\langle (\unicode[STIX]{x0394}u_{1})^{4}(\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })\rangle$
which appears in the sixth-order longitudinal structure function equation. In the equation for
![]() $\langle E_{6,0}\rangle$
, a source term
$\langle E_{6,0}\rangle$
, a source term
![]() $\langle F_{6,0}\rangle =15\langle (\unicode[STIX]{x0394}u_{1})^{2}(\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })^{2}\rangle$
appears. In the equation for
$\langle F_{6,0}\rangle =15\langle (\unicode[STIX]{x0394}u_{1})^{2}(\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })^{2}\rangle$
appears. In the equation for
![]() $\langle F_{6,0}\rangle$
, the term
$\langle F_{6,0}\rangle$
, the term
![]() $15\langle (\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })^{3}\rangle$
appears, which generates the new dissipation parameter
$15\langle (\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })^{3}\rangle$
appears, which generates the new dissipation parameter
![]() $\langle \unicode[STIX]{x1D700}_{11}^{3}\rangle$
, and combined with the mixed and transverse equations, one finds an
$\langle \unicode[STIX]{x1D700}_{11}^{3}\rangle$
, and combined with the mixed and transverse equations, one finds an
![]() $\unicode[STIX]{x1D700}_{[6]}^{3}$
-term which contains
$\unicode[STIX]{x1D700}_{[6]}^{3}$
-term which contains
![]() $\langle (\unicode[STIX]{x1D700}+\unicode[STIX]{x1D700}^{\prime })^{3}\rangle$
, i.e. one would expect to find a contribution by
$\langle (\unicode[STIX]{x1D700}+\unicode[STIX]{x1D700}^{\prime })^{3}\rangle$
, i.e. one would expect to find a contribution by
![]() $\langle \unicode[STIX]{x1D700}_{r}^{3}\rangle$
in the sixth-order equations. In order to calculate seventh-order inertial-range scaling coefficients from the sixth-order structure function equations and their dissipation source terms, the trace of the structure function equations and two successive source term equations would have to be integrated. An ansatz similar to (4.11) would provide at leading order the exponent
$\langle \unicode[STIX]{x1D700}_{r}^{3}\rangle$
in the sixth-order equations. In order to calculate seventh-order inertial-range scaling coefficients from the sixth-order structure function equations and their dissipation source terms, the trace of the structure function equations and two successive source term equations would have to be integrated. An ansatz similar to (4.11) would provide at leading order the exponent
![]() $7/3$
. The deviation from this K41 scaling exponent would then involve more empirical terms. Similar arguments will hold for all other uneven-order scaling exponents. Because there are no dissipation parameters in the uneven-order source term equations, even-order scaling exponents cannot be determined in this way.
$7/3$
. The deviation from this K41 scaling exponent would then involve more empirical terms. Similar arguments will hold for all other uneven-order scaling exponents. Because there are no dissipation parameters in the uneven-order source term equations, even-order scaling exponents cannot be determined in this way.
5 Conclusion
In addition to
![]() $\unicode[STIX]{x1D708}$
and
$\unicode[STIX]{x1D708}$
and
![]() $\langle \unicode[STIX]{x1D700}\rangle$
, all higher-order moments of the dissipation distribution function appear as dissipation parameters in the extended system of two-point equations for small-scale turbulence. The effect of the higher-order dissipation parameters on the solutions of these equations is demonstrated for the trace of the fourth-order structure function equations, which are invariant, independent of the coordinate system. The procedure can also be carried over to the individual structure function equations at higher even orders; for example, in the sixth-order equations, the third-order dissipation parameters
$\langle \unicode[STIX]{x1D700}\rangle$
, all higher-order moments of the dissipation distribution function appear as dissipation parameters in the extended system of two-point equations for small-scale turbulence. The effect of the higher-order dissipation parameters on the solutions of these equations is demonstrated for the trace of the fourth-order structure function equations, which are invariant, independent of the coordinate system. The procedure can also be carried over to the individual structure function equations at higher even orders; for example, in the sixth-order equations, the third-order dissipation parameters
![]() $\langle (\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })^{3}\rangle$
etc. are found. The analysis uses exact equations, but because of the inherent closure problem needs to apply empirical closure assumptions between some of the terms. The conclusions are twofold. (1) The higher-order dissipation parameters determine the solutions of the even-order structure functions in the viscous range. (2) The trace of the dissipation parameters in the system of equations contains the moments of
$\langle (\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })^{3}\rangle$
etc. are found. The analysis uses exact equations, but because of the inherent closure problem needs to apply empirical closure assumptions between some of the terms. The conclusions are twofold. (1) The higher-order dissipation parameters determine the solutions of the even-order structure functions in the viscous range. (2) The trace of the dissipation parameters in the system of equations contains the moments of
![]() $\unicode[STIX]{x1D700}_{r}$
. However, the relation of the specific assumption
$\unicode[STIX]{x1D700}_{r}$
. However, the relation of the specific assumption
![]() $\langle \unicode[STIX]{x1D700}_{r}^{n/3}\rangle$
for the
$\langle \unicode[STIX]{x1D700}_{r}^{n/3}\rangle$
for the
![]() $n$
th-order structure functions in the inertial range to the dissipation parameters derived from the Navier–Stokes equations is missing.
$n$
th-order structure functions in the inertial range to the dissipation parameters derived from the Navier–Stokes equations is missing.
Acknowledgements
This work was supported by the Deutsche Forschungsgemeinschaft through the grant Pe 241/38-2. The authors gratefully acknowledge the computing time granted by the JARA-HPC-Vergabegremium provided on the JARA-HPC Partition part of the supercomputer JUQUEEN at the Forschungszentrum Jülich.
Appendix A. Direct numerical simulation (DNS) in a cubic box for Taylor-based Reynolds numbers up to 529
We solve the three-dimensional Navier–Stokes equations for incompressible fluids in rotational form,
with the continuity equation
where
![]() $\unicode[STIX]{x1D74E}=\unicode[STIX]{x1D735}\times \boldsymbol{u}$
is the vorticity,
$\unicode[STIX]{x1D74E}=\unicode[STIX]{x1D735}\times \boldsymbol{u}$
is the vorticity,
![]() $p$
is the ratio of pressure and density, and
$p$
is the ratio of pressure and density, and
![]() $\unicode[STIX]{x1D708}$
is the kinematic viscosity;
$\unicode[STIX]{x1D708}$
is the kinematic viscosity;
![]() $\boldsymbol{f}$
is a forcing term which acts on large scales only.
$\boldsymbol{f}$
is a forcing term which acts on large scales only.
Equation (A 1) is solved by a pseudo-spectral approach in Fourier space, cf. Rogallo (Reference Rogallo1981) and Canuto et al. (Reference Canuto, Hussaini, Quarteroni and Zang1988). Temporal integration is carried out by an explicit second-order Adams–Bashforth method, cf. Butcher (Reference Butcher2004). The integration domain is a triply periodic cube with length
![]() $2\unicode[STIX]{x03C0}$
. An external stochastic forcing, cf. Eswaran & Pope (Reference Eswaran and Pope1988), is applied to the velocity field to maintain a statistically steady state. The simulations have been carried out with an in-house hybrid MPI/OpenMP parallelized simulation code which efficiently utilizes the BlueGene/Q architecture.
$2\unicode[STIX]{x03C0}$
. An external stochastic forcing, cf. Eswaran & Pope (Reference Eswaran and Pope1988), is applied to the velocity field to maintain a statistically steady state. The simulations have been carried out with an in-house hybrid MPI/OpenMP parallelized simulation code which efficiently utilizes the BlueGene/Q architecture.
Characteristic parameters of the DNS are listed in table 1. Here,
![]() $N$
denotes the number of grid points in one direction,
$N$
denotes the number of grid points in one direction,
![]() $Re_{\unicode[STIX]{x1D706}}$
the Taylor-based Reynolds number,
$Re_{\unicode[STIX]{x1D706}}$
the Taylor-based Reynolds number,
![]() $\langle k\rangle$
the mean turbulent kinetic energy,
$\langle k\rangle$
the mean turbulent kinetic energy,
![]() $\langle \unicode[STIX]{x1D700}\rangle$
the mean energy dissipation rate,
$\langle \unicode[STIX]{x1D700}\rangle$
the mean energy dissipation rate,
![]() $\unicode[STIX]{x1D70F}=\langle k\rangle /\langle \unicode[STIX]{x1D700}\rangle$
the integral time scale,
$\unicode[STIX]{x1D70F}=\langle k\rangle /\langle \unicode[STIX]{x1D700}\rangle$
the integral time scale,
![]() $\unicode[STIX]{x1D708}$
the kinematic viscosity,
$\unicode[STIX]{x1D708}$
the kinematic viscosity,
![]() $\unicode[STIX]{x1D702}=(\unicode[STIX]{x1D708}^{3}/\langle \unicode[STIX]{x1D700}\rangle )^{1/4}$
the Kolmogorov length scale and
$\unicode[STIX]{x1D702}=(\unicode[STIX]{x1D708}^{3}/\langle \unicode[STIX]{x1D700}\rangle )^{1/4}$
the Kolmogorov length scale and
![]() $\unicode[STIX]{x1D70F}$
the integral time scale, whereas
$\unicode[STIX]{x1D70F}$
the integral time scale, whereas
![]() $t_{avg}$
is the average time up to which the simulations have been performed;
$t_{avg}$
is the average time up to which the simulations have been performed;
![]() $M$
is the number of independent data sets over which averages have been taken and
$M$
is the number of independent data sets over which averages have been taken and
![]() $\unicode[STIX]{x0394}t$
is the time step. Following Ishihara et al. (Reference Ishihara, Kaneda, Yokokawa and Uno2007), a resolution
$\unicode[STIX]{x0394}t$
is the time step. Following Ishihara et al. (Reference Ishihara, Kaneda, Yokokawa and Uno2007), a resolution
![]() $k_{max}\unicode[STIX]{x1D702}>1.7$
is maintained in order to accurately compute higher-order statistics.
$k_{max}\unicode[STIX]{x1D702}>1.7$
is maintained in order to accurately compute higher-order statistics.
Table 1. Characteristic parameters of the DNS.

Appendix B. Longitudinal, transverse and mixed fourth-order structure function and dissipative source term equations
Here, the longitudinal, mixed and transverse fourth-order structure function equations are briefly presented. A detailed derivation can be found in Hill (Reference Hill2001). In their homogeneous isotropic form, the structure function equations read as follows.
Longitudinal:
 $$\begin{eqnarray}\displaystyle & & \displaystyle \frac{\unicode[STIX]{x2202}S_{4,0}}{\unicode[STIX]{x2202}t}+\frac{\unicode[STIX]{x2202}S_{5,0}}{\unicode[STIX]{x2202}r}+\frac{2}{r}S_{5,0}-\frac{8}{r}S_{3,2}\nonumber\\ \displaystyle & & \displaystyle \quad =-\langle T_{4,0}\rangle -\langle E_{4,0}\rangle +2\unicode[STIX]{x1D708}\left[\frac{\unicode[STIX]{x2202}^{2}S_{4,0}}{\unicode[STIX]{x2202}r^{2}}+\frac{2}{r}\frac{\unicode[STIX]{x2202}S_{4,0}}{\unicode[STIX]{x2202}r}-\frac{8}{r^{2}}S_{4,0}+\frac{24}{r^{2}}S_{2,2}\right].\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \frac{\unicode[STIX]{x2202}S_{4,0}}{\unicode[STIX]{x2202}t}+\frac{\unicode[STIX]{x2202}S_{5,0}}{\unicode[STIX]{x2202}r}+\frac{2}{r}S_{5,0}-\frac{8}{r}S_{3,2}\nonumber\\ \displaystyle & & \displaystyle \quad =-\langle T_{4,0}\rangle -\langle E_{4,0}\rangle +2\unicode[STIX]{x1D708}\left[\frac{\unicode[STIX]{x2202}^{2}S_{4,0}}{\unicode[STIX]{x2202}r^{2}}+\frac{2}{r}\frac{\unicode[STIX]{x2202}S_{4,0}}{\unicode[STIX]{x2202}r}-\frac{8}{r^{2}}S_{4,0}+\frac{24}{r^{2}}S_{2,2}\right].\end{eqnarray}$$
Mixed:
 $$\begin{eqnarray}\displaystyle & & \displaystyle \frac{\unicode[STIX]{x2202}S_{2,2}}{\unicode[STIX]{x2202}t}+\frac{\unicode[STIX]{x2202}S_{3,2}}{\unicode[STIX]{x2202}r}+\frac{4}{r}S_{3,2}-\frac{8}{3r}S_{1,4}\nonumber\\ \displaystyle & & \displaystyle \quad =-\langle T_{2,2}\rangle -\langle E_{2,2}\rangle +2\unicode[STIX]{x1D708}\left[\frac{2}{r^{2}}S_{4,0}+\frac{\unicode[STIX]{x2202}^{2}S_{2,2}}{\unicode[STIX]{x2202}r^{2}}+\frac{2}{r}\frac{\unicode[STIX]{x2202}S_{2,2}}{\unicode[STIX]{x2202}r}-\frac{14}{r^{2}}S_{2,2}+\frac{8}{3r^{2}}S_{0,4}\right].\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \frac{\unicode[STIX]{x2202}S_{2,2}}{\unicode[STIX]{x2202}t}+\frac{\unicode[STIX]{x2202}S_{3,2}}{\unicode[STIX]{x2202}r}+\frac{4}{r}S_{3,2}-\frac{8}{3r}S_{1,4}\nonumber\\ \displaystyle & & \displaystyle \quad =-\langle T_{2,2}\rangle -\langle E_{2,2}\rangle +2\unicode[STIX]{x1D708}\left[\frac{2}{r^{2}}S_{4,0}+\frac{\unicode[STIX]{x2202}^{2}S_{2,2}}{\unicode[STIX]{x2202}r^{2}}+\frac{2}{r}\frac{\unicode[STIX]{x2202}S_{2,2}}{\unicode[STIX]{x2202}r}-\frac{14}{r^{2}}S_{2,2}+\frac{8}{3r^{2}}S_{0,4}\right].\end{eqnarray}$$
Transverse:
The coefficients in the viscous terms differ from those given by Hill (Reference Hill2001). Our derivation may be found in the archive material. In agreement with the present formulae, http://arxiv.org/abs/physics/0102055 (derivation for Hill (Reference Hill2001)) now contains corrected viscous-term formulae, matrix algorithm and table of formulae. The pressure source terms
![]() $\langle T_{4,0}\rangle$
,
$\langle T_{4,0}\rangle$
,
![]() $\langle T_{2,2}\rangle$
and
$\langle T_{2,2}\rangle$
and
![]() $\langle T_{0,4}\rangle$
are defined as
$\langle T_{0,4}\rangle$
are defined as
where
![]() $\unicode[STIX]{x0394}P_{i}=(\unicode[STIX]{x2202}p/\unicode[STIX]{x2202}x_{i}-\unicode[STIX]{x2202}p^{\prime }/\unicode[STIX]{x2202}x_{i}^{\prime })$
is the difference of pressure gradients at the two points. The dissipation source terms
$\unicode[STIX]{x0394}P_{i}=(\unicode[STIX]{x2202}p/\unicode[STIX]{x2202}x_{i}-\unicode[STIX]{x2202}p^{\prime }/\unicode[STIX]{x2202}x_{i}^{\prime })$
is the difference of pressure gradients at the two points. The dissipation source terms
![]() $\langle E_{4,0}\rangle$
,
$\langle E_{4,0}\rangle$
,
![]() $\langle E_{2,2}\rangle$
and
$\langle E_{2,2}\rangle$
and
![]() $\langle E_{0,4}\rangle$
are defined as
$\langle E_{0,4}\rangle$
are defined as
respectively, with
![]() $\unicode[STIX]{x1D700}_{ij}=2\unicode[STIX]{x1D708}(\unicode[STIX]{x2202}u_{i}/\unicode[STIX]{x2202}x_{k})(\unicode[STIX]{x2202}u_{j}/\unicode[STIX]{x2202}x_{k})$
.
$\unicode[STIX]{x1D700}_{ij}=2\unicode[STIX]{x1D708}(\unicode[STIX]{x2202}u_{i}/\unicode[STIX]{x2202}x_{k})(\unicode[STIX]{x2202}u_{j}/\unicode[STIX]{x2202}x_{k})$
.
Noticeably enough, we have three equations for three unknown structure functions in the viscous range as well as under the inertial-range assumptions. This implies that we can then integrate the equations starting with (B 3), inserting the solution into (B 2), integrating this equation, and then finally solve (B 1), if the source terms are known. This characteristic is found for all even orders, but not for odd orders. Indeed, one can obtain Kolmogorov’s
![]() $4/5$
and
$4/5$
and
![]() $4/15$
laws by integrating the second-order equations in the same manner, cf. Hill (Reference Hill1997).
$4/15$
laws by integrating the second-order equations in the same manner, cf. Hill (Reference Hill1997).
The individual equations for the fourth-order dissipative source terms are given in the archive material. The transport equation for
![]() $\langle E_{4,0}\rangle$
reads
$\langle E_{4,0}\rangle$
reads
 $$\begin{eqnarray}\displaystyle & & \displaystyle \frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{1}E_{4,0}\rangle }{\unicode[STIX]{x2202}r}+\frac{2}{r}\langle \unicode[STIX]{x0394}u_{1}E_{4,0}\rangle -\frac{8}{r}\langle \unicode[STIX]{x0394}u_{2}E_{3,1}\rangle \nonumber\\ \displaystyle & & \displaystyle \quad =2\unicode[STIX]{x1D708}\left[\frac{\unicode[STIX]{x2202}^{2}\langle E_{4,0}\rangle }{\unicode[STIX]{x2202}r^{2}}+\frac{2}{r}\frac{\unicode[STIX]{x2202}\langle E_{4,0}\rangle }{\unicode[STIX]{x2202}r}-\frac{8}{r^{2}}\langle E_{4,0}\rangle +\frac{24}{r^{2}}\langle E_{2,2}\rangle \right]-\sum \mathop{P}_{4,0}^{E},\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{1}E_{4,0}\rangle }{\unicode[STIX]{x2202}r}+\frac{2}{r}\langle \unicode[STIX]{x0394}u_{1}E_{4,0}\rangle -\frac{8}{r}\langle \unicode[STIX]{x0394}u_{2}E_{3,1}\rangle \nonumber\\ \displaystyle & & \displaystyle \quad =2\unicode[STIX]{x1D708}\left[\frac{\unicode[STIX]{x2202}^{2}\langle E_{4,0}\rangle }{\unicode[STIX]{x2202}r^{2}}+\frac{2}{r}\frac{\unicode[STIX]{x2202}\langle E_{4,0}\rangle }{\unicode[STIX]{x2202}r}-\frac{8}{r^{2}}\langle E_{4,0}\rangle +\frac{24}{r^{2}}\langle E_{2,2}\rangle \right]-\sum \mathop{P}_{4,0}^{E},\end{eqnarray}$$
where the sum of source terms is
 $$\begin{eqnarray}\displaystyle \sum \mathop{P}_{4,0}^{E} & = & \displaystyle 24\unicode[STIX]{x1D708}\langle (\unicode[STIX]{x0394}u_{1})^{2}(A_{11}+A_{11}^{\prime })\rangle +12\unicode[STIX]{x1D708}\langle (\unicode[STIX]{x0394}u_{1})^{2}(\unicode[STIX]{x1D712}_{11}+\unicode[STIX]{x1D712}_{11}^{\prime })\rangle \nonumber\\ \displaystyle & & \displaystyle +\,12\langle \unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}P_{1}(\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })\rangle +24\unicode[STIX]{x1D708}\langle \unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}u_{1}(P_{11}+P_{11}^{\prime })\rangle \nonumber\\ \displaystyle & & \displaystyle +\,12\unicode[STIX]{x1D708}\left\langle \left(\frac{\unicode[STIX]{x2202}(\unicode[STIX]{x0394}u_{1})^{2}}{\unicode[STIX]{x2202}x_{n}}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{11}}{\unicode[STIX]{x2202}x_{n}}+\frac{\unicode[STIX]{x2202}(\unicode[STIX]{x0394}u_{1})^{2}}{\unicode[STIX]{x2202}x_{n}^{\prime }}\frac{\unicode[STIX]{x1D700}_{11}^{\prime }}{\unicode[STIX]{x2202}x_{n}^{\prime }}\right)\right\rangle +6\langle (\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })^{2}\rangle .\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \sum \mathop{P}_{4,0}^{E} & = & \displaystyle 24\unicode[STIX]{x1D708}\langle (\unicode[STIX]{x0394}u_{1})^{2}(A_{11}+A_{11}^{\prime })\rangle +12\unicode[STIX]{x1D708}\langle (\unicode[STIX]{x0394}u_{1})^{2}(\unicode[STIX]{x1D712}_{11}+\unicode[STIX]{x1D712}_{11}^{\prime })\rangle \nonumber\\ \displaystyle & & \displaystyle +\,12\langle \unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}P_{1}(\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })\rangle +24\unicode[STIX]{x1D708}\langle \unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}u_{1}(P_{11}+P_{11}^{\prime })\rangle \nonumber\\ \displaystyle & & \displaystyle +\,12\unicode[STIX]{x1D708}\left\langle \left(\frac{\unicode[STIX]{x2202}(\unicode[STIX]{x0394}u_{1})^{2}}{\unicode[STIX]{x2202}x_{n}}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{11}}{\unicode[STIX]{x2202}x_{n}}+\frac{\unicode[STIX]{x2202}(\unicode[STIX]{x0394}u_{1})^{2}}{\unicode[STIX]{x2202}x_{n}^{\prime }}\frac{\unicode[STIX]{x1D700}_{11}^{\prime }}{\unicode[STIX]{x2202}x_{n}^{\prime }}\right)\right\rangle +6\langle (\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })^{2}\rangle .\end{eqnarray}$$
For the mixed dissipative source term
![]() $\langle E_{2,2}\rangle$
one obtains the transport equation
$\langle E_{2,2}\rangle$
one obtains the transport equation
 $$\begin{eqnarray}\displaystyle & & \displaystyle \frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{1}E_{2,2}\rangle }{\unicode[STIX]{x2202}r}+\frac{2}{r}\langle \unicode[STIX]{x0394}u_{1}E_{2,2}\rangle +\frac{2}{r}\langle \unicode[STIX]{x0394}u_{2}E_{3,1}\rangle -\frac{8}{3r}\langle \unicode[STIX]{x0394}u_{2}E_{1,3}\rangle \nonumber\\ \displaystyle & & \displaystyle \quad =2\unicode[STIX]{x1D708}\left[\frac{\unicode[STIX]{x2202}^{2}\langle E_{2,2}\rangle }{\unicode[STIX]{x2202}r^{2}}+\frac{2}{r}\frac{\unicode[STIX]{x2202}\langle E_{2,2}\rangle }{\unicode[STIX]{x2202}r}-\frac{14}{r^{2}}\langle E_{2,2}\rangle +\frac{2}{r^{2}}\langle E_{4,0}\rangle +\frac{8}{3r^{2}}\langle E_{0,4}\rangle \right]-\sum \mathop{P}_{2,2}^{E},\qquad\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{1}E_{2,2}\rangle }{\unicode[STIX]{x2202}r}+\frac{2}{r}\langle \unicode[STIX]{x0394}u_{1}E_{2,2}\rangle +\frac{2}{r}\langle \unicode[STIX]{x0394}u_{2}E_{3,1}\rangle -\frac{8}{3r}\langle \unicode[STIX]{x0394}u_{2}E_{1,3}\rangle \nonumber\\ \displaystyle & & \displaystyle \quad =2\unicode[STIX]{x1D708}\left[\frac{\unicode[STIX]{x2202}^{2}\langle E_{2,2}\rangle }{\unicode[STIX]{x2202}r^{2}}+\frac{2}{r}\frac{\unicode[STIX]{x2202}\langle E_{2,2}\rangle }{\unicode[STIX]{x2202}r}-\frac{14}{r^{2}}\langle E_{2,2}\rangle +\frac{2}{r^{2}}\langle E_{4,0}\rangle +\frac{8}{3r^{2}}\langle E_{0,4}\rangle \right]-\sum \mathop{P}_{2,2}^{E},\qquad\end{eqnarray}$$
where the sum of source terms is
 $$\begin{eqnarray}\displaystyle \sum \mathop{P}_{2,2}^{E} & = & \displaystyle 2\unicode[STIX]{x1D708}\langle 2(\unicode[STIX]{x0394}u_{1})^{2}(A_{22}+A_{22}^{\prime })+4\unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}u_{2}(A_{12}+A_{12}^{\prime }+A_{21}+A_{21}^{\prime })\nonumber\\ \displaystyle & & \displaystyle +\,2(\unicode[STIX]{x0394}u_{2})^{2}(A_{11}+A_{11}^{\prime })\rangle +2\unicode[STIX]{x1D708}\langle (\unicode[STIX]{x0394}u_{1})^{2}(\unicode[STIX]{x1D712}_{22}+\unicode[STIX]{x1D712}_{22}^{\prime })+2\unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}u_{2}(\unicode[STIX]{x1D712}_{12}+\unicode[STIX]{x1D712}_{12}^{\prime })\nonumber\\ \displaystyle & & \displaystyle +\,(\unicode[STIX]{x0394}u_{2})^{2}(\unicode[STIX]{x1D712}_{11}+\unicode[STIX]{x1D712}_{11}^{\prime })\rangle +2\langle \unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}P_{1}(\unicode[STIX]{x1D700}_{22}+\unicode[STIX]{x1D700}_{22}^{\prime })\nonumber\\ \displaystyle & & \displaystyle +\,2(\unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}P_{2}+\unicode[STIX]{x0394}u_{2}\unicode[STIX]{x0394}P_{1})(\unicode[STIX]{x1D700}_{12}+\unicode[STIX]{x1D700}_{12}^{\prime })+\unicode[STIX]{x0394}u_{2}\unicode[STIX]{x0394}P_{2}(\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })\rangle \nonumber\\ \displaystyle & & \displaystyle +\,2\unicode[STIX]{x1D708}\langle 2(\unicode[STIX]{x0394}u_{1})^{2}(P_{22}+P_{22}^{\prime })+4\unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}u_{2}(P_{12}+P_{12}^{\prime }+P_{21}+P_{21}^{\prime })\nonumber\\ \displaystyle & & \displaystyle +\,2(\unicode[STIX]{x0394}u_{2})^{2}(P_{11}+P_{11}^{\prime })\rangle +2\unicode[STIX]{x1D708}\left\langle \left(\frac{\unicode[STIX]{x2202}(\unicode[STIX]{x0394}u_{1})^{2}}{\unicode[STIX]{x2202}x_{n}}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{22}}{\unicode[STIX]{x2202}x_{n}}+4\frac{\unicode[STIX]{x2202}\unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}u_{2}}{\unicode[STIX]{x2202}x_{n}}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{12}}{\unicode[STIX]{x2202}x_{n}}\right.\right.\nonumber\\ \displaystyle & & \displaystyle +\left.\left.\frac{\unicode[STIX]{x2202}(\unicode[STIX]{x0394}u_{2})^{2}}{\unicode[STIX]{x2202}x_{n}}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{11}}{\unicode[STIX]{x2202}x_{n}}+\frac{\unicode[STIX]{x2202}(\unicode[STIX]{x0394}u_{1})^{2}}{\unicode[STIX]{x2202}x_{n}^{\prime }}\frac{\unicode[STIX]{x1D700}_{22}^{\prime }}{\unicode[STIX]{x2202}x_{n}^{\prime }}+\frac{\unicode[STIX]{x2202}\unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}u_{2}}{\unicode[STIX]{x2202}x_{n}^{\prime }}\frac{\unicode[STIX]{x1D700}_{12}^{\prime }}{\unicode[STIX]{x2202}x_{n}^{\prime }}+\frac{\unicode[STIX]{x2202}(\unicode[STIX]{x0394}u_{2})^{2}}{\unicode[STIX]{x2202}x_{n}^{\prime }}\frac{\unicode[STIX]{x1D700}_{11}^{\prime }}{\unicode[STIX]{x2202}x_{n}^{\prime }}\right)\right\rangle \nonumber\\ \displaystyle & & \displaystyle +\,2\langle (\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })(\unicode[STIX]{x1D700}_{22}+\unicode[STIX]{x1D700}_{22}^{\prime })+(\unicode[STIX]{x1D700}_{12}+\unicode[STIX]{x1D700}_{12}^{\prime })(\unicode[STIX]{x1D700}_{12}+\unicode[STIX]{x1D700}_{12}^{\prime })\rangle .\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \sum \mathop{P}_{2,2}^{E} & = & \displaystyle 2\unicode[STIX]{x1D708}\langle 2(\unicode[STIX]{x0394}u_{1})^{2}(A_{22}+A_{22}^{\prime })+4\unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}u_{2}(A_{12}+A_{12}^{\prime }+A_{21}+A_{21}^{\prime })\nonumber\\ \displaystyle & & \displaystyle +\,2(\unicode[STIX]{x0394}u_{2})^{2}(A_{11}+A_{11}^{\prime })\rangle +2\unicode[STIX]{x1D708}\langle (\unicode[STIX]{x0394}u_{1})^{2}(\unicode[STIX]{x1D712}_{22}+\unicode[STIX]{x1D712}_{22}^{\prime })+2\unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}u_{2}(\unicode[STIX]{x1D712}_{12}+\unicode[STIX]{x1D712}_{12}^{\prime })\nonumber\\ \displaystyle & & \displaystyle +\,(\unicode[STIX]{x0394}u_{2})^{2}(\unicode[STIX]{x1D712}_{11}+\unicode[STIX]{x1D712}_{11}^{\prime })\rangle +2\langle \unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}P_{1}(\unicode[STIX]{x1D700}_{22}+\unicode[STIX]{x1D700}_{22}^{\prime })\nonumber\\ \displaystyle & & \displaystyle +\,2(\unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}P_{2}+\unicode[STIX]{x0394}u_{2}\unicode[STIX]{x0394}P_{1})(\unicode[STIX]{x1D700}_{12}+\unicode[STIX]{x1D700}_{12}^{\prime })+\unicode[STIX]{x0394}u_{2}\unicode[STIX]{x0394}P_{2}(\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })\rangle \nonumber\\ \displaystyle & & \displaystyle +\,2\unicode[STIX]{x1D708}\langle 2(\unicode[STIX]{x0394}u_{1})^{2}(P_{22}+P_{22}^{\prime })+4\unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}u_{2}(P_{12}+P_{12}^{\prime }+P_{21}+P_{21}^{\prime })\nonumber\\ \displaystyle & & \displaystyle +\,2(\unicode[STIX]{x0394}u_{2})^{2}(P_{11}+P_{11}^{\prime })\rangle +2\unicode[STIX]{x1D708}\left\langle \left(\frac{\unicode[STIX]{x2202}(\unicode[STIX]{x0394}u_{1})^{2}}{\unicode[STIX]{x2202}x_{n}}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{22}}{\unicode[STIX]{x2202}x_{n}}+4\frac{\unicode[STIX]{x2202}\unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}u_{2}}{\unicode[STIX]{x2202}x_{n}}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{12}}{\unicode[STIX]{x2202}x_{n}}\right.\right.\nonumber\\ \displaystyle & & \displaystyle +\left.\left.\frac{\unicode[STIX]{x2202}(\unicode[STIX]{x0394}u_{2})^{2}}{\unicode[STIX]{x2202}x_{n}}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{11}}{\unicode[STIX]{x2202}x_{n}}+\frac{\unicode[STIX]{x2202}(\unicode[STIX]{x0394}u_{1})^{2}}{\unicode[STIX]{x2202}x_{n}^{\prime }}\frac{\unicode[STIX]{x1D700}_{22}^{\prime }}{\unicode[STIX]{x2202}x_{n}^{\prime }}+\frac{\unicode[STIX]{x2202}\unicode[STIX]{x0394}u_{1}\unicode[STIX]{x0394}u_{2}}{\unicode[STIX]{x2202}x_{n}^{\prime }}\frac{\unicode[STIX]{x1D700}_{12}^{\prime }}{\unicode[STIX]{x2202}x_{n}^{\prime }}+\frac{\unicode[STIX]{x2202}(\unicode[STIX]{x0394}u_{2})^{2}}{\unicode[STIX]{x2202}x_{n}^{\prime }}\frac{\unicode[STIX]{x1D700}_{11}^{\prime }}{\unicode[STIX]{x2202}x_{n}^{\prime }}\right)\right\rangle \nonumber\\ \displaystyle & & \displaystyle +\,2\langle (\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })(\unicode[STIX]{x1D700}_{22}+\unicode[STIX]{x1D700}_{22}^{\prime })+(\unicode[STIX]{x1D700}_{12}+\unicode[STIX]{x1D700}_{12}^{\prime })(\unicode[STIX]{x1D700}_{12}+\unicode[STIX]{x1D700}_{12}^{\prime })\rangle .\end{eqnarray}$$
The transport equation for the transverse dissipation source term
![]() $\langle E_{0,4}\rangle$
is
$\langle E_{0,4}\rangle$
is
 $$\begin{eqnarray}\displaystyle & & \displaystyle \frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{1}E_{0,4}\rangle }{\unicode[STIX]{x2202}r}+\frac{2}{r}\langle \unicode[STIX]{x0394}u_{1}E_{0,4}\rangle +\frac{4}{r}\langle \unicode[STIX]{x0394}u_{2}E_{1,3}\rangle \nonumber\\ \displaystyle & & \displaystyle \quad =2\unicode[STIX]{x1D708}\left[\frac{\unicode[STIX]{x2202}^{2}\langle E_{0,4}\rangle }{\unicode[STIX]{x2202}r^{2}}+\frac{2}{r}\frac{\unicode[STIX]{x2202}\langle E_{0,4}\rangle }{\unicode[STIX]{x2202}r}-\frac{4}{r}\langle E_{0,4}\rangle +\frac{12}{r^{2}}\langle E_{2,2}\rangle \right]-\sum \mathop{P}_{0.4}^{E},\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \frac{\unicode[STIX]{x2202}\langle \unicode[STIX]{x0394}u_{1}E_{0,4}\rangle }{\unicode[STIX]{x2202}r}+\frac{2}{r}\langle \unicode[STIX]{x0394}u_{1}E_{0,4}\rangle +\frac{4}{r}\langle \unicode[STIX]{x0394}u_{2}E_{1,3}\rangle \nonumber\\ \displaystyle & & \displaystyle \quad =2\unicode[STIX]{x1D708}\left[\frac{\unicode[STIX]{x2202}^{2}\langle E_{0,4}\rangle }{\unicode[STIX]{x2202}r^{2}}+\frac{2}{r}\frac{\unicode[STIX]{x2202}\langle E_{0,4}\rangle }{\unicode[STIX]{x2202}r}-\frac{4}{r}\langle E_{0,4}\rangle +\frac{12}{r^{2}}\langle E_{2,2}\rangle \right]-\sum \mathop{P}_{0.4}^{E},\end{eqnarray}$$
where the sum of the source term is
 $$\begin{eqnarray}\displaystyle \sum \mathop{P}_{0,4}^{E} & = & \displaystyle 24\unicode[STIX]{x1D708}\langle (\unicode[STIX]{x0394}u_{2})^{2}(A_{2,2}+A_{2,2}^{\prime })\rangle +12\unicode[STIX]{x1D708}\langle (\unicode[STIX]{x0394}u_{2})^{2}(\unicode[STIX]{x1D712}_{22}+\unicode[STIX]{x1D712}_{22}^{\prime })\rangle \nonumber\\ \displaystyle & & \displaystyle +\,12\langle \unicode[STIX]{x0394}u_{2}\unicode[STIX]{x0394}P_{2}(\unicode[STIX]{x1D700}_{22}+\unicode[STIX]{x1D700}_{22}^{\prime })\rangle +24\unicode[STIX]{x1D708}\langle \unicode[STIX]{x0394}u_{2}^{2}(P_{22}+P_{22}^{\prime })\rangle \nonumber\\ \displaystyle & & \displaystyle +\,12\unicode[STIX]{x1D708}\left\langle \frac{\unicode[STIX]{x2202}\unicode[STIX]{x0394}u_{2}^{2}}{\unicode[STIX]{x2202}x_{n}}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{22}}{\unicode[STIX]{x2202}x_{n}}+\frac{\unicode[STIX]{x2202}\unicode[STIX]{x0394}u_{2}^{2}}{\unicode[STIX]{x2202}x_{n}^{\prime }}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{22}^{\prime }}{\unicode[STIX]{x2202}x_{n}^{\prime }}\right\rangle +6\langle (\unicode[STIX]{x1D700}_{22}+\unicode[STIX]{x1D700}_{22}^{\prime })^{2}\rangle .\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \sum \mathop{P}_{0,4}^{E} & = & \displaystyle 24\unicode[STIX]{x1D708}\langle (\unicode[STIX]{x0394}u_{2})^{2}(A_{2,2}+A_{2,2}^{\prime })\rangle +12\unicode[STIX]{x1D708}\langle (\unicode[STIX]{x0394}u_{2})^{2}(\unicode[STIX]{x1D712}_{22}+\unicode[STIX]{x1D712}_{22}^{\prime })\rangle \nonumber\\ \displaystyle & & \displaystyle +\,12\langle \unicode[STIX]{x0394}u_{2}\unicode[STIX]{x0394}P_{2}(\unicode[STIX]{x1D700}_{22}+\unicode[STIX]{x1D700}_{22}^{\prime })\rangle +24\unicode[STIX]{x1D708}\langle \unicode[STIX]{x0394}u_{2}^{2}(P_{22}+P_{22}^{\prime })\rangle \nonumber\\ \displaystyle & & \displaystyle +\,12\unicode[STIX]{x1D708}\left\langle \frac{\unicode[STIX]{x2202}\unicode[STIX]{x0394}u_{2}^{2}}{\unicode[STIX]{x2202}x_{n}}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{22}}{\unicode[STIX]{x2202}x_{n}}+\frac{\unicode[STIX]{x2202}\unicode[STIX]{x0394}u_{2}^{2}}{\unicode[STIX]{x2202}x_{n}^{\prime }}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D700}_{22}^{\prime }}{\unicode[STIX]{x2202}x_{n}^{\prime }}\right\rangle +6\langle (\unicode[STIX]{x1D700}_{22}+\unicode[STIX]{x1D700}_{22}^{\prime })^{2}\rangle .\end{eqnarray}$$
That is, we find the second-order dissipation parameters
![]() $\langle (\unicode[STIX]{x1D700}_{ij}+\unicode[STIX]{x1D700}_{ij}^{\prime })(\unicode[STIX]{x1D700}_{kl}+\unicode[STIX]{x1D700}_{kl}^{\prime })\rangle$
in the fourth-order dissipative source term equations.
$\langle (\unicode[STIX]{x1D700}_{ij}+\unicode[STIX]{x1D700}_{ij}^{\prime })(\unicode[STIX]{x1D700}_{kl}+\unicode[STIX]{x1D700}_{kl}^{\prime })\rangle$
in the fourth-order dissipative source term equations.
Appendix C. Relations between fourth-order structure functions and second-order dissipation parameters in the viscous range
In this section we will briefly discuss the individual fourth-order structure functions in the viscous range.
In the viscous range, we need to consider only the balance between the viscous terms and the dissipation source terms in the fourth-order longitudinal, mixed and transverse structure functions, cf. figure 1:
In the limit
![]() $r\rightarrow 0$
, the viscous terms balance to leading order. In the dissipative source term equations, the viscous terms balance the
$r\rightarrow 0$
, the viscous terms balance to leading order. In the dissipative source term equations, the viscous terms balance the
![]() $\unicode[STIX]{x1D700}^{2}$
-terms, resulting in
$\unicode[STIX]{x1D700}^{2}$
-terms, resulting in
 $$\begin{eqnarray}\displaystyle & & \displaystyle 2\unicode[STIX]{x1D708}\left[\frac{2}{r^{2}}\langle E_{4,0}\rangle +\frac{\unicode[STIX]{x2202}^{2}\langle E_{2,2}\rangle }{\unicode[STIX]{x2202}r^{2}}+\frac{2}{r}\frac{\unicode[STIX]{x2202}\langle E_{2,2}\rangle }{\unicode[STIX]{x2202}r}-\frac{14}{r^{2}}\langle E_{2,2}\rangle +\frac{8}{3r^{2}}\langle E_{0,4}\rangle \right]\nonumber\\ \displaystyle & & \displaystyle \quad =2\langle (\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })(\unicode[STIX]{x1D700}_{22}+\unicode[STIX]{x1D700}_{22}^{\prime })\rangle +4\langle (\unicode[STIX]{x1D700}_{12}+\unicode[STIX]{x1D700}_{12}^{\prime })^{2}\rangle ,\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle 2\unicode[STIX]{x1D708}\left[\frac{2}{r^{2}}\langle E_{4,0}\rangle +\frac{\unicode[STIX]{x2202}^{2}\langle E_{2,2}\rangle }{\unicode[STIX]{x2202}r^{2}}+\frac{2}{r}\frac{\unicode[STIX]{x2202}\langle E_{2,2}\rangle }{\unicode[STIX]{x2202}r}-\frac{14}{r^{2}}\langle E_{2,2}\rangle +\frac{8}{3r^{2}}\langle E_{0,4}\rangle \right]\nonumber\\ \displaystyle & & \displaystyle \quad =2\langle (\unicode[STIX]{x1D700}_{11}+\unicode[STIX]{x1D700}_{11}^{\prime })(\unicode[STIX]{x1D700}_{22}+\unicode[STIX]{x1D700}_{22}^{\prime })\rangle +4\langle (\unicode[STIX]{x1D700}_{12}+\unicode[STIX]{x1D700}_{12}^{\prime })^{2}\rangle ,\end{eqnarray}$$
Expanding the structure functions as in (3.4)–(3.6) and the dissipation source terms as
 $$\begin{eqnarray}\displaystyle E_{2,2}=\unicode[STIX]{x1D6FD}_{2,2}^{0}r^{2}+\cdots & = & \displaystyle \left(2\left\langle \left(\frac{\unicode[STIX]{x2202}u_{1}}{\unicode[STIX]{x2202}x_{1}}\right)^{2}\unicode[STIX]{x1D700}_{11}\right\rangle \right.\nonumber\\ \displaystyle & & \displaystyle +\left.8\langle \left(\frac{\unicode[STIX]{x2202}u_{1}}{\unicode[STIX]{x2202}x_{1}}\right)\left(\frac{\unicode[STIX]{x2202}u_{2}}{\unicode[STIX]{x2202}x_{1}}\right)\unicode[STIX]{x1D700}_{12}\rangle +2\left\langle \left(\frac{\unicode[STIX]{x2202}u_{1}}{\unicode[STIX]{x2202}x_{1}}\right)^{2}\unicode[STIX]{x1D700}_{22}\right\rangle \right)r^{2}\ldots ,\quad\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle E_{2,2}=\unicode[STIX]{x1D6FD}_{2,2}^{0}r^{2}+\cdots & = & \displaystyle \left(2\left\langle \left(\frac{\unicode[STIX]{x2202}u_{1}}{\unicode[STIX]{x2202}x_{1}}\right)^{2}\unicode[STIX]{x1D700}_{11}\right\rangle \right.\nonumber\\ \displaystyle & & \displaystyle +\left.8\langle \left(\frac{\unicode[STIX]{x2202}u_{1}}{\unicode[STIX]{x2202}x_{1}}\right)\left(\frac{\unicode[STIX]{x2202}u_{2}}{\unicode[STIX]{x2202}x_{1}}\right)\unicode[STIX]{x1D700}_{12}\rangle +2\left\langle \left(\frac{\unicode[STIX]{x2202}u_{1}}{\unicode[STIX]{x2202}x_{1}}\right)^{2}\unicode[STIX]{x1D700}_{22}\right\rangle \right)r^{2}\ldots ,\quad\end{eqnarray}$$
we obtain to leading order the relations for
![]() $F_{1}$
,
$F_{1}$
,
![]() $F_{2}$
and
$F_{2}$
and
![]() $F_{3}$
:
$F_{3}$
:
Gauss elimination leads to a singular system and thereby to the compatibility condition
By inserting (C 10)–(C 12) into the equations for the fourth-order dissipation source terms, we obtain to leading order the relations
Gauss elimination leads again to a singular system and a compatibility condition relating the dissipation parameters,
Since, due to isotropy,
![]() $\langle \unicode[STIX]{x1D700}_{11}^{2}\rangle =\langle \unicode[STIX]{x1D700}_{22}^{2}\rangle$
and (C 17) may be written as
$\langle \unicode[STIX]{x1D700}_{11}^{2}\rangle =\langle \unicode[STIX]{x1D700}_{22}^{2}\rangle$
and (C 17) may be written as
by using (C 10) and (C 11) in (C 12), one obtains a relation between
![]() $F_{1}$
,
$F_{1}$
,
![]() $F_{2}$
,
$F_{2}$
,
![]() $F_{3}$
and
$F_{3}$
and
![]() $\langle \unicode[STIX]{x1D700}_{11}^{2}\rangle$
as
$\langle \unicode[STIX]{x1D700}_{11}^{2}\rangle$
as
Table 2. Ratios of invariants of the fourth-order velocity derivative tensor.

Using (3.8), all second-order dissipation parameters may be expressed in terms of Siggia’s invariants,
As noted by Siggia (Reference Siggia1981), the ratios
![]() $\mathit{I}_{2}/\mathit{I}_{1}$
,
$\mathit{I}_{2}/\mathit{I}_{1}$
,
![]() $\mathit{I}_{3}/\mathit{I}_{1}$
and
$\mathit{I}_{3}/\mathit{I}_{1}$
and
![]() $\mathit{I}_{4}/\mathit{I}_{1}$
should be Reynolds-number-independent for large Reynolds numbers. This is shown to be approximately true for the Reynolds numbers of our DNS calculations as shown in table 2, where the ratios are also compared with the values obtained by Ishihara et al. (Reference Ishihara, Kaneda, Yokokawa and Uno2007) calculated at
$\mathit{I}_{4}/\mathit{I}_{1}$
should be Reynolds-number-independent for large Reynolds numbers. This is shown to be approximately true for the Reynolds numbers of our DNS calculations as shown in table 2, where the ratios are also compared with the values obtained by Ishihara et al. (Reference Ishihara, Kaneda, Yokokawa and Uno2007) calculated at
![]() $Re_{\unicode[STIX]{x1D706}}=680$
. Taking the numbers of Ishihara et al. (Reference Ishihara, Kaneda, Yokokawa and Uno2007) for these ratios, we obtain with
$Re_{\unicode[STIX]{x1D706}}=680$
. Taking the numbers of Ishihara et al. (Reference Ishihara, Kaneda, Yokokawa and Uno2007) for these ratios, we obtain with
![]() $\mathit{I}_{1}=\langle \unicode[STIX]{x1D700}^{2}\rangle /(4\unicode[STIX]{x1D708}^{2})$
$\mathit{I}_{1}=\langle \unicode[STIX]{x1D700}^{2}\rangle /(4\unicode[STIX]{x1D708}^{2})$
All second-order dissipation parameters should therefore scale with the Reynolds number in the same way as
![]() $\langle \unicode[STIX]{x1D700}^{2}\rangle$
.
$\langle \unicode[STIX]{x1D700}^{2}\rangle$
.