Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Xu, Zhigang
Wang, Longlong
Wang, Tianyou
and
Che, Zhizhao
2021.
Crown rupture during droplet impact on a dry smooth surface at increased pressure.
Physics of Fluids,
Vol. 33,
Issue. 12,
Zhang, Haixiang
Gao, Ye
Zhang, Xiwen
Yi, Xian
Du, Yanxia
He, Feng
Jin, Zheyan
and
Hao, Pengfei
2021.
Characteristics of secondary droplets produced by the impact of drops onto a smooth surface.
Advances in Aerodynamics,
Vol. 3,
Issue. 1,
Bourouiba, Lydia
2021.
Fluid Dynamics of Respiratory Infectious Diseases.
Annual Review of Biomedical Engineering,
Vol. 23,
Issue. 1,
p.
547.
Constante-Amores, C.R.
Chergui, J.
Shin, S.
Juric, D.
Castrejón-Pita, J.R.
and
Castrejón-Pita, A.A.
2022.
Role of surfactant-induced Marangoni stresses in retracting liquid sheets.
Journal of Fluid Mechanics,
Vol. 949,
Issue. ,
Zhou, Chenglin
Zou, Jianfeng
and
Zhang, Yang
2022.
Effect of Streamwise Perturbation Frequency on Formation Mechanism of Ligament and Droplet in Liquid Circular Jet.
Aerospace,
Vol. 9,
Issue. 4,
p.
191.
Zhang, Haixiang
Zhang, Xiwen
Yi, Xian
Du, Yanxia
He, Feng
Niu, Fenglei
and
Hao, Pengfei
2022.
How surface roughness promotes or suppresses drop splash.
Physics of Fluids,
Vol. 34,
Issue. 2,
Wang, Y.
and
Bourouiba, L.
2022.
Mass, momentum and energy partitioning in unsteady fragmentation.
Journal of Fluid Mechanics,
Vol. 935,
Issue. ,
Hernandez-Rueda, Javier
Liu, Bo
Hemminga, Diko J.
Mostafa, Yahia
Meijer, Randy A.
Kurilovich, Dmitry
Basko, Mikhail
Gelderblom, Hanneke
Sheil, John
and
Versolato, Oscar O.
2022.
Early-time hydrodynamic response of a tin droplet driven by laser-produced plasma.
Physical Review Research,
Vol. 4,
Issue. 1,
Liu, Bo
Hernandez-Rueda, Javier
Gelderblom, Hanneke
and
Versolato, Oscar O.
2022.
Speed of fragments ejected by an expanding liquid tin sheet.
Physical Review Fluids,
Vol. 7,
Issue. 8,
Jackiw, Isaac M.
and
Ashgriz, Nasser
2022.
Prediction of the droplet size distribution in aerodynamic droplet breakup.
Journal of Fluid Mechanics,
Vol. 940,
Issue. ,
Wang, Y.
and
Bourouiba, L.
2023.
Non-Galilean Taylor–Culick law governs sheet dynamics in unsteady fragmentation.
Journal of Fluid Mechanics,
Vol. 969,
Issue. ,
Jaberi, Amin
Debenest, Gérald
and
Plouraboué, Franck
2023.
Marangoni-driven spreading and receding of a volatile droplet on a liquid layer.
Physical Review Fluids,
Vol. 8,
Issue. 7,
Lathia, Rutvik
Dey Modak, Chandantaru
and
Sen, Prosenjit
2023.
Suppression of droplet pinch-off by early onset of interfacial instability.
Journal of Colloid and Interface Science,
Vol. 646,
Issue. ,
p.
606.
Kant, Pallav
Pairetti, Cesar
Saade, Youssef
Popinet, Stéphane
Zaleski, Stéphane
and
Lohse, Detlef
2023.
Bag-mediated film atomization in a cough machine.
Physical Review Fluids,
Vol. 8,
Issue. 7,
Liu, Bo
Meijer, Randy A.
Li, Wei
Hernandez-Rueda, Javier
Gelderblom, Hanneke
and
Versolato, Oscar O.
2023.
Mass Partitioning in Fragmenting Tin Sheets.
Physical Review Applied,
Vol. 20,
Issue. 1,
Goswami, Anjan
and
Hardalupas, Yannis
2023.
Simultaneous impact of droplet pairs on solid surfaces.
Journal of Fluid Mechanics,
Vol. 961,
Issue. ,
Mohd, Javed
Yadav, Amar
and
Das, Debopam
2024.
Classifications and scaling of generic shaped, steady and unsteady water bells formed by a jet impinging onto a vial.
Journal of Fluid Mechanics,
Vol. 999,
Issue. ,
Wang, Geng
Lei, Timan
Yang, Junyu
Fei, Linlin
Chen, Jin
and
Luo, Kai H.
2024.
Lattice Boltzmann modelling and study of droplet equatorial streaming in an electric field.
Journal of Fluid Mechanics,
Vol. 988,
Issue. ,
Tang, K.
Adcock, T.A.A.
and
Mostert, W.
2024.
Fragmentation of colliding liquid rims.
Journal of Fluid Mechanics,
Vol. 987,
Issue. ,
Bauer, Tobias
and
Gilet, Tristan
2024.
Rim dynamics and droplet ejections upon drop impact on star-shaped poles.
Physical Review Fluids,
Vol. 9,
Issue. 8,
 $\text {Bond number} = 1 \text { criterion}$, Wang et al. (Phys. Rev. Lett., vol. 120, 2018, 204503)) and scales as
$\text {Bond number} = 1 \text { criterion}$, Wang et al. (Phys. Rev. Lett., vol. 120, 2018, 204503)) and scales as  $N_c \sim We^{3/4}$ with Weber number,
$N_c \sim We^{3/4}$ with Weber number,  $We$. The number of ligaments scales as
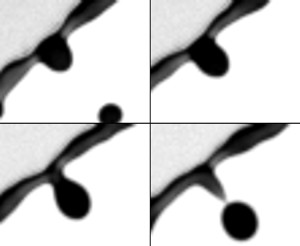
$We$. The number of ligaments scales as  $N_{\ell } \sim We^{3/8}$. The growth of a ligament is governed by the competition between the constraint imposed by the geometry of the local rim–ligament junction; the local force balance including the fictitious force from the continuously decelerating rim; and the global rim mass conservation constraint. The temporal evolution of the average width of ligaments is predicted. Key to understanding the ligament population, a minimum distance between two corrugations is required to enable their actual transition into ligaments. By predicting this minimal distance, we derive the evolution of the number of ligaments. We show that droplets are shed, one at a time, following a chaotic dripping end-pinching regime independent of
$N_{\ell } \sim We^{3/8}$. The growth of a ligament is governed by the competition between the constraint imposed by the geometry of the local rim–ligament junction; the local force balance including the fictitious force from the continuously decelerating rim; and the global rim mass conservation constraint. The temporal evolution of the average width of ligaments is predicted. Key to understanding the ligament population, a minimum distance between two corrugations is required to enable their actual transition into ligaments. By predicting this minimal distance, we derive the evolution of the number of ligaments. We show that droplets are shed, one at a time, following a chaotic dripping end-pinching regime independent of  $We$. Finally, the number of droplets shed per unit of time decreases over time and scales as
$We$. Finally, the number of droplets shed per unit of time decreases over time and scales as  $S_d \sim We^{3/4}$; while the volume shed per unit of time increases over time and is independent of
$S_d \sim We^{3/4}$; while the volume shed per unit of time increases over time and is independent of  $We$. Theoretical predictions are validated without fitting parameters.
$We$. Theoretical predictions are validated without fitting parameters.