Article contents
Global modes of variable-viscosity two-phase swirling flows and their triadic resonance
Published online by Cambridge University Press: 16 January 2023
Abstract
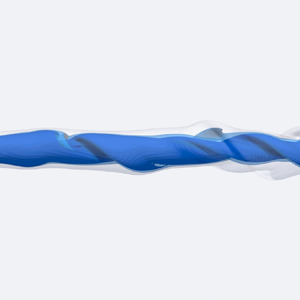
The linear and nonlinear dynamics of two-phase swirling flows produced by the Grabowski–Berger profile under the influence of a viscosity stratification are investigated. We perform axisymmetric nonlinear simulations and fully three-dimensional linear global stability analysis of the flow for several swirl numbers  $S$ and viscosity ratios
$S$ and viscosity ratios  $\tilde {\mu }$. They are accompanied by nonlinear three-dimensional simulations and subsequent modal analysis using the bispectral mode decomposition, recently introduced by Schmidt (Nonlinear Dyn., vol. 102, issue 4, 2020, pp. 2479–2501). We find a pronounced destabilising effect of the viscosity stratification on both the onset of axisymmetric vortex breakdown and helical instability that is linked to the required shear stress continuity across the interface. Consequently, destabilisation is shifted to lower
$\tilde {\mu }$. They are accompanied by nonlinear three-dimensional simulations and subsequent modal analysis using the bispectral mode decomposition, recently introduced by Schmidt (Nonlinear Dyn., vol. 102, issue 4, 2020, pp. 2479–2501). We find a pronounced destabilising effect of the viscosity stratification on both the onset of axisymmetric vortex breakdown and helical instability that is linked to the required shear stress continuity across the interface. Consequently, destabilisation is shifted to lower  $S$ as compared with an equivalent flow with uniform viscosity. Further, the stability analysis reveals the simultaneous destabilisation of two global modes with wavenumbers
$S$ as compared with an equivalent flow with uniform viscosity. Further, the stability analysis reveals the simultaneous destabilisation of two global modes with wavenumbers  $m=1$ and
$m=1$ and  $m=2$ that have harmonic frequencies. The analysis of the nonlinear flow reveals a strong triadic resonance between these modes that governs the nonlinear dynamics and leads to a rapid departure from the linear dynamics. At larger swirl, the bifurcation of additional modes initiates an interaction cascade by means of triadic resonance which is elucidated by the bispectral analysis. It leads to the emergence of a variety of additional modes in the nonlinear flow. This study contributes to an improved understanding of the influence of viscosity stratification on the onset of vortex breakdown and the destabilisation of global modes. Further, it provides a clear picture of the dynamics of swirling flows with a codimension-two point and related triadic interaction of two global modes at harmonic frequencies and wavenumbers.
$m=2$ that have harmonic frequencies. The analysis of the nonlinear flow reveals a strong triadic resonance between these modes that governs the nonlinear dynamics and leads to a rapid departure from the linear dynamics. At larger swirl, the bifurcation of additional modes initiates an interaction cascade by means of triadic resonance which is elucidated by the bispectral analysis. It leads to the emergence of a variety of additional modes in the nonlinear flow. This study contributes to an improved understanding of the influence of viscosity stratification on the onset of vortex breakdown and the destabilisation of global modes. Further, it provides a clear picture of the dynamics of swirling flows with a codimension-two point and related triadic interaction of two global modes at harmonic frequencies and wavenumbers.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
References
REFERENCES
- 8
- Cited by



