Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-17T09:18:11.308Z Has data issue: false hasContentIssue false
  • English
  • Français

Forced synchronization and asynchronous quenching of periodic oscillations in a thermoacoustic system

Published online by Cambridge University Press:  01 February 2019

Sirshendu Mondal Open the ORCID record for Sirshendu Mondal [Opens in a new window] ,
Samadhan A. Pawar Open the ORCID record for Samadhan A. Pawar [Opens in a new window]  and
R. I. Sujith
Sirshendu Mondal
Affiliation:
Department of Aerospace Engineering, Indian Institute of Technology Madras, Chennai 600036, India
Samadhan A. Pawar
Affiliation:
Department of Aerospace Engineering, Indian Institute of Technology Madras, Chennai 600036, India
R. I. Sujith
Affiliation:
Department of Aerospace Engineering, Indian Institute of Technology Madras, Chennai 600036, India
Get access Rights & Permissions [Opens in a new window]

Abstract

We perform an experimental and theoretical study to investigate the interaction between an external harmonic excitation and a self-excited oscillatory mode ($f_{n0}$) of a prototypical thermoacoustic system, a horizontal Rijke tube. Such an interaction can lead to forced synchronization through the routes of phase locking or suppression. We characterize the transition in the synchronization behaviour of the forcing and the response signals of the acoustic pressure while the forcing parameters, i.e. 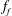amplitude ($A_{f}$) and frequency ($f_{f}$) of forcing are independently varied. Further, suppression is categorized into synchronous quenching and asynchronous quenching depending upon the value of frequency detuning (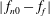$|\,f_{n0}-f_{f}|$). When the applied forcing frequency is close to the natural frequency of the system, the suppression in the amplitude of the self-excited oscillation is known as synchronous quenching. However, this suppression is associated with resonant amplification of the forcing signal, leading to an overall increase in the response amplitude of oscillations. On the other hand, an almost 80 % reduction in the root mean square value of the response oscillation is observed when the system is forced for a sufficiently large value of the frequency detuning (only for 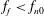$f_{f}<f_{n0}$). Such a reduction in amplitude occurs due to asynchronous quenching where resonant amplification of the forcing signal does not occur, as the frequency detuning is significantly high. Further, the results from a reduced-order model developed for a horizontal Rijke tube show a qualitative agreement with the dynamics observed in experiments. The relative phase between the acoustic pressure (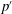$p^{\prime }$) and the heat release rate (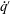$\dot{q}^{\prime }$) oscillations in the model explains the occurrence of maximum reduction in the pressure amplitude due to asynchronous quenching. Such a reduction occurs when the positive coupling between 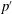$p^{\prime }$ and 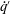$\dot{q}^{\prime }$ is disrupted and their interaction results in overall acoustic damping, although both of them oscillate at the forcing frequency. Our study on the phenomenon of asynchronous quenching thus presents new possibilities to suppress self-sustained oscillations in fluid systems in general.

JFM classification

Flow Control: Instability control Acoustics: Noise control Instability: Nonlinear instability
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 864 , 10 April 2019 , pp. 73 - 96
Check for updates
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

†

Present address: Department of Mechanical Engineering, National Institute of Technology, Durgapur 713209, India. Email address for correspondence: [email protected]

References

Abel, M., Ahnert, K. & Bergweiler, S. 2009 Synchronization of sound sources. Phys. Rev. Lett. 103 (11), 114301.Google Scholar
Anishchenko, V. S., Vadivasova, T. E., Postnov, D. E. & Safonova, M. A. 1992 Synchronization of chaos. Intl J. Bifurcation Chaos 2 (03), 633–644.Google Scholar
Balanov, A., Janson, N., Postnov, D. & Sosnovtseva, O. 2009 Synchronization. In Springer Series in Synergetics. Springer.Google Scholar
Balasubramanian, K. & Sujith, R. I. 2008 Thermoacoustic instability in a Rijke tube: non-normality and nonlinearity. Phys. Fluids 20 (4), 044103.Google Scholar
Balusamy, S., Li, L. K. B., Han, Z. & Hochgreb, S. 2017 Extracting flame describing functions in the presence of self-excited thermoacoustic oscillations. Proc. Combust. Inst. 36 (3), 3851–3861.Google Scholar
Balusamy, S., Li, L. K. B., Han, Z., Juniper, M. P. & Hochgreb, S. 2015 Nonlinear dynamics of a self-excited thermoacoustic system subjected to acoustic forcing. Proc. Combust. Inst. 35 (3), 3229–3236.Google Scholar
Battogtokh, D., Aihara, K. & Tyson, J. J. 2006 Synchronization of eukaryotic cells by periodic forcing. Phys. Rev. Lett. 96 (14), 148102.Google Scholar
Bellows, B. D., Hreiz, A. & Lieuwen, T. 2008 Nonlinear interactions between forced and self-excited acoustic oscillations in premixed combustor. J. Propul. Power 24 (3), 628–630.Google Scholar
Bellows, B. D., Neumeier, Y. & Lieuwen, T. 2006 Forced response of a swirling, premixed flame to flow disturbances. J. Propul. Power 22 (5), 1075–1084.Google Scholar
Blažek, V. 1968 A semiconductor laser as a classical van der Pol oscillator controlled by an external signal. Czech. J. Phys. B 18 (5), 644–646.Google Scholar
Boccaletti, S., Allaria, E., Meucci, R. & Arecchi, F. T. 2002 Experimental characterization of the transition to phase synchronization of chaotic CO2 laser systems. Phys. Rev. Lett. 89 (19), 194101.Google Scholar
Crocco, L. & Cheng, S.-I.1956 Theory of combustion instability in liquid propellant rocket motors. Tech. Rep. Princeton University NJ.Google Scholar
Dines, P. J.1984 Active control of flame noise. PhD thesis, University of Cambridge.Google Scholar
Dowling, A. P. & Morgans, A. S. 2005 Feedback control of combustion oscillations. Annu. Rev. Fluid Mech. 37, 151–182.Google Scholar
Emerson, B., O’Connor, J., Juniper, M. & Lieuwen, T. 2012 Density ratio effects on reacting bluff-body flow field characteristics. J. Fluid Mech. 706, 219–250.Google Scholar
Etikyala, S. & Sujith, R. I. 2017 Change of criticality in a prototypical thermoacoustic system. Chaos: Interdiscip. J. Nonlinear Sci. 27 (2), 023106.Google Scholar
Gopalakrishnan, E. A. & Sujith, R. I. 2014 Influence of system parameters on the hysteresis characteristics of a horizontal Rijke tube. Intl J. Spray Combust. Dyn. 6 (3), 293–316.Google Scholar
Gopalakrishnan, E. A. & Sujith, R. I. 2015 Effect of external noise on the hysteresis characteristics of a thermoacoustic system. J. Fluid Mech. 776, 334–353.Google Scholar
Greenblatt, D. & Wygnanski, I. J. 2000 The control of flow separation by periodic excitation. Prog. Aerosp. Sci. 36 (7), 487–545.Google Scholar
Guan, Y., Gupta, V., Kashinath, K. & Li, L. K. B. 2018 Open-loop control of periodic thermoacoustic oscillations: experiments and low-order modelling in a synchronization framework. In Proceedings of the Combustion Institute, Elsevier.Google Scholar
Hallberg, M. P. & Strykowski, P. J. 2008 Open-loop control of fully nonlinear self-excited oscillations. Phys. Fluids 20 (4), 041703.Google Scholar
Han, Z., Balusamy, S. & Hochgreb, S. 2015 Spatial analysis on forced heat release response of turbulent stratified flames. J. Engng Gas Turbines Power 137 (6), 061504.Google Scholar
Han, Z. & Hochgreb, S. 2015 The response of stratified swirling flames to acoustic forcing: experiments and comparison to model. Proc. Combust. Inst. 35 (3), 3309–3315.Google Scholar
Heckl, M. A. 1988 Active control of the noise from a Rijke tube. J. Sound Vib. 124 (1), 117–133.Google Scholar
Heckl, M. A. 1990 Non-linear acoustic effects in the Rijke tube. Acta Acust. United Ac. 72 (1), 63–71.Google Scholar
Huerre, P. & Monkewitz, P. A. 1985 Absolute and convective instabilities in free shear layers. J. Fluid Mech. 159, 151–168.Google Scholar
Huerre, P. & Monkewitz, P. A. 1990 Local and global instabilities in spatially developing flows. Annu. Rev. Fluid Mech. 22 (1), 473–537.Google Scholar
Hyodo, H. & Biwa, T. 2018 Phase-locking and suppression states observed in forced synchronization of thermoacoustic oscillator. J. Phys. Soc. Japan 87 (3), 034402.Google Scholar
Juniper, M. P. 2011 Triggering in the horizontal Rijke tube: non-normality, transient growth and bypass transition. J. Fluid Mech. 667, 272–308.Google Scholar
Juniper, M. P. & Sujith, R. I. 2018 Sensitivity and nonlinearity of thermoacoustic oscillations. Annu. Rev. Fluid Mech. 50, 661–689.Google Scholar
Kashinath, K., Li, L. K. B. & Juniper, M. P. 2018 Forced synchronization of periodic and aperiodic thermoacoustic oscillations: lock-in, bifurcations and open-loop control. J. Fluid Mech. 838, 690–714.Google Scholar
Keen, B. E. & Fletcher, W. H. W. 1969 Suppression and enhancement of an ion-sound instability by nonlinear resonance effects in a plasma. Phys. Rev. Lett. 23 (14), 760.Google Scholar
Kim, K. T. & Hochgreb, S. 2011 The nonlinear heat release response of stratified lean-premixed flames to acoustic velocity oscillations. Combust. Flame 158 (12), 2482–2499.Google Scholar
Kim, K. T. & Hochgreb, S. 2012 Measurements of triggering and transient growth in a model lean-premixed gas turbine combustor. Combust. Flame 159 (3), 1215–1227.Google Scholar
King, L. V. 1914 On the convection of heat from small cylinders in a stream of fluid: determination of the convection constants of small platinum wires with applications to hot-wire anemometry. Phil. Trans. R. Soc. Lond. A 214, 373–432.Google Scholar
Kiss, I. Z. & Hudson, J. L. 2001 Phase synchronization and suppression of chaos through intermittency in forcing of an electrochemical oscillator. Phys. Rev. E 64 (4), 046215.Google Scholar
Lang, W., Poinsot, T., Bourienne, F., Candel, S. & Esposito, E.1987a Suppression of combustion instabilities by active control. AIAA Paper 87-1876.Google Scholar
Lang, W., Poinsot, T. & Candel, S. 1987b Active control of combustion instability. Combust. Flame 70 (3), 281–289.Google Scholar
Li, L. K. B. & Juniper, M. P. 2013a Lock-in and quasiperiodicity in a forced hydrodynamically self-excited jet. J. Fluid Mech. 726, 624–655.Google Scholar
Li, L. K. B. & Juniper, M. P. 2013b Lock-in and quasiperiodicity in hydrodynamically self-excited flames: experiments and modelling. Proc. Combust. Inst. 34 (1), 947–954.Google Scholar
Li, L. K. B. & Juniper, M. P. 2013c Phase trapping and slipping in a forced hydrodynamically self-excited jet. J. Fluid Mech. 735, R5.Google Scholar
Lieuwen, T. C. & Yang, V. 2005 Combustion Instabilities in Gas Turbine Engines: Operational Experience, Fundamental Mechanisms, and Modeling. AIAA.Google Scholar
Lores, M. E. & Zinn, B. T. 1973 Nonlinear longitudinal combustion instability in rocket motors. Combust. Sci. Technol. 7 (6), 245–256.Google Scholar
Lubarsky, E., Shcherbik, D., Zinn, B., McManus, K., Fric, T. & Srinivasan, S. 2003 Active control of combustion oscillations by non-coherent fuel flow modulation. In 9th AIAA/CEAS Aeroacoustics Conference and Exhibit, p. 3180. ARC-AIAA.Google Scholar
Magri, L. & Juniper, M. P. 2013 Sensitivity analysis of a time-delayed thermo-acoustic system via an adjoint-based approach. J. Fluid Mech. 719, 183–202.Google Scholar
Mariappan, S.2012 Theoretical and experimental investigation of the non-normal nature of thermoacoustic interactions. PhD thesis, Indian Institute of Technology Madras.Google Scholar
Matveev, K. I.2003 Thermoacoustic instabilities in the Rijke tube: experiments and modeling. PhD thesis, California Institute of Technology.Google Scholar
McManus, K. R., Vandsburger, U. & Bowman, C. T. 1990 Combustor performance enhancement through direct shear layer excitation. Combust. Flame 82 (1), 75–92.Google Scholar
Minorsky, N. 1967 Comments ‘On asynchronous quenching’. IEEE Trans. Autom. Control 12 (2), 225–227.Google Scholar
Monkewitz, P. A. 1988 The absolute and convective nature of instability in two-dimensional wakes at low Reynolds numbers. Phys. Fluids 31 (5), 999–1006.Google Scholar
Najm, H. & Ghoniem, A. 1991 Numerical simulation of the convective instability in a dump combustor. AIAA J. 29 (6), 911–919.Google Scholar
Nicoud, F. & Wieczorek, K. 2009 About the zero Mach number assumption in the calculation of thermoacoustic instabilities. Intl J. Spray Combust. Dyn. 1 (1), 67–111.Google Scholar
Nomura, T., Sato, S., Doi, S., Segundo, J. P. & Stiber, M. D. 1993 A Bonhoeffer–van der Pol oscillator model of locked and non-locked behaviors of living pacemaker neurons. Biol. Cybernet. 69 (5–6), 429–437.Google Scholar
Odajima, K., Nishida, Y. & Hatta, Y. 1974 Synchronous quenching of drift-wave instability. Phys. Fluids 17 (8), 1631–1633.Google Scholar
Ohe, K. & Takeda, S. 1974 Asynchronous quenching and resonance excitation of ionization waves in positive columns. Contrib. Plasma Phys. 14 (2), 55–65.Google Scholar
Ohsawa, T. 1980 Synchronous quenching due to nonlinear mode coupling in beam-plasma system. J. Phys. Soc. Japan 49 (6), 2340–2348.Google Scholar
Oyediran, A., Darling, D. & Radhakrishnan, K. 1995 Review of combustion-acoustics instabilities. In 31st Joint Propulsion Conference and Exhibit, p. 2469. ARC-AIAA.Google Scholar
Pikovsky, A., Rosenblum, M. & Kurths, J. 2003 Synchronization: A Universal Concept in Nonlinear Sciences, vol. 12. Cambridge University Press.Google Scholar
van der Pol, B. 1920 A theory of the amplitude of free and forced triode vibrations. Radio Rev. 1 (1920), 701–710.Google Scholar
Provansal, M., Mathis, C. & Boyer, L. 1987 Bénard–von Kármán instability: transient and forced regimes. J. Fluid Mech. 182, 1–22.Google Scholar
Rayleigh, Lord 1878 The explanation of certain acoustical phenomena. Roy. Inst. Proc. 8, 536–542.Google Scholar
Staubli, T. 1987 Entrainment of self-sustained flow oscillations: phaselooking or asynchronous quenching? J. Appl. Mech. 54, 707.Google Scholar
Sterling, J. D. & Zukoski, E. E. 1991 Nonlinear dynamics of laboratory combustor pressure oscillations. Combust. Sci. Technol. 77 (4–6), 225–238.Google Scholar
Sujith, R. I., Juniper, M. P. & Schmid, P. J. 2016 Non-normality and nonlinearity in thermoacoustic instabilities. Intl J. Spray Combust. Dyn. 8 (2), 119–146.Google Scholar
Thévenin, J., Romanelli, M., Vallet, M., Brunel, M. & Erneux, T. 2011 Resonance assisted synchronization of coupled oscillators: frequency locking without phase locking. Phys. Rev. Lett. 107 (10), 104101.Google Scholar
Thomas, N., Mondal, S., Pawar, S. A. & Sujith, R. I. 2018 Effect of time-delay and dissipative coupling on amplitude death in coupled thermoacoustic oscillators. Chaos: Interdiscip. J. Nonlinear Sci. 28 (3), 033119.Google Scholar
Van Der Pol, B. & Van Der Mark, J. 1928 Lxxii. The heartbeat considered as a relaxation oscillation, and an electrical model of the heart. Lond. Edinb. Dubl. Phil. Mag. J. Sci. 6 (38), 763–775.Google Scholar
Yoshida, T., Yazaki, T., Ueda, Y. & Biwa, T. 2013 Forced synchronization of periodic oscillations in a gas column: where is the power source? J. Phys. Soc. Japan 82 (10), 103001.Google Scholar
Zhao, D. & Reyhanoglu, M. 2014 Feedback control of acoustic disturbance transient growth in triggering thermoacoustic instability. J. Sound Vib. 333 (16), 3639–3656.Google Scholar