Hostname: page-component-586b7cd67f-r5fsc Total loading time: 0 Render date: 2024-11-26T07:37:03.143Z Has data issue: false hasContentIssue false
  • English
  • Français

Flow-induced vibrations of a rotating cylinder

Published online by Cambridge University Press:  06 February 2014

Rémi Bourguet  and
David Lo Jacono
Rémi Bourguet*
Affiliation:
Institut de Mécanique des Fluides de Toulouse, CNRS, UPS and Université de Toulouse, 31400 Toulouse, France
David Lo Jacono
Affiliation:
Institut de Mécanique des Fluides de Toulouse, CNRS, UPS and Université de Toulouse, 31400 Toulouse, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

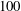The flow-induced vibrations of a circular cylinder, free to oscillate in the cross-flow direction and subjected to a forced rotation about its axis, are analysed by means of two- and three-dimensional numerical simulations. The impact of the symmetry breaking caused by the forced rotation on the vortex-induced vibration (VIV) mechanisms is investigated for a Reynolds number equal to $100$, based on the cylinder diameter and inflow velocity. The cylinder is found to oscillate freely up to a rotation rate (ratio between the cylinder surface and inflow velocities) close to 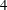$4$. Under forced rotation, the vibration amplitude exhibits a bell-shaped evolution as a function of the reduced velocity (inverse of the oscillator natural frequency) and reaches 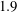$1.9$ diameters, i.e. three times the maximum amplitude in the non-rotating case. The free vibrations of the rotating cylinder occur under a condition of wake–body synchronization similar to the lock-in condition driving non-rotating cylinder VIV. The largest vibration amplitudes are associated with a novel asymmetric wake pattern composed of a triplet of vortices and a single vortex shed per cycle, the ${\rm T} + {\rm S}$ pattern. In the low-frequency vibration regime, the flow exhibits another new topology, the U pattern, characterized by a transverse undulation of the spanwise vorticity layers without vortex detachment; consequently, free oscillations of the rotating cylinder may also develop in the absence of vortex shedding. The symmetry breaking due to the rotation is shown to directly impact the selection of the higher harmonics appearing in the fluid force spectra. The rotation also influences the mechanism of phasing between the force and the structural response.

JFM classification

Wakes/Jets: Vortex streets Wakes/Jets: Wakes
Type
Papers
Information
Journal of Fluid Mechanics , Volume 740 , 10 February 2014 , pp. 342 - 380
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Badr, H. M., Coutanceau, M., Dennis, S. C. R. & Ménard, C. 1990 Unsteady flow past a rotating circular cylinder at Reynolds numbers $10^{3}$ and $10^{4}$. J. Fluid Mech. 220, 459–484.CrossRefGoogle Scholar
Bearman, P. W. 1984 Vortex shedding from oscillating bluff bodies. Annu. Rev. Fluid Mech. 16, 195–222.Google Scholar
Bearman, P. W. 2011 Circular cylinder wakes and vortex-induced vibrations. J. Fluids Struct. 27, 648–658.Google Scholar
Bearman, P. W., Gartshore, I. S., Maull, D. J. & Parkinson, G. V. 1987 Experiments on flow-induced vibration of a square-section cylinder. J. Fluids Struct. 1, 19–34.Google Scholar
Berger, E. 1967 Suppression of vortex shedding and turbulence behind oscillating cylinders. Phys. Fluids 10, S191.Google Scholar
Bishop, R. E. D. & Hassan, A. Y. 1964 The lift and drag forces on a circular cylinder oscillating in a flowing fluid. Proc. R. Soc. A 277, 51–75.Google Scholar
Blackburn, H. M. & Henderson, R. D. 1999 A study of two-dimensional flow past an oscillating cylinder. J. Fluid Mech. 385, 255–286.CrossRefGoogle Scholar
Blevins, R. D. 1990 Flow-Induced Vibration. Van Nostrand Reinhold.Google Scholar
Bourguet, R., Karniadakis, G. E. & Triantafyllou, M. S. 2011a Lock-in of the vortex-induced vibrations of a long tensioned beam in shear flow. J. Fluids Struct. 27, 838–847.Google Scholar
Bourguet, R., Karniadakis, G. E. & Triantafyllou, M. S. 2011b Vortex-induced vibrations of a long flexible cylinder in shear flow. J. Fluid Mech. 677, 342–382.Google Scholar
Braza, M., Chassaing, P. & Ha Minh, H. 1986 Numerical study and physical analysis of the pressure and velocity fields in the near wake of a circular cylinder. J. Fluid Mech. 165, 79–130.Google Scholar
Brika, D. & Laneville, A. 1993 Vortex-induced vibrations of a long flexible circular cylinder. J. Fluid Mech. 250, 481–508.Google Scholar
Carberry, J., Sheridan, J. & Rockwell, D. 2001 Forces and wake modes of an oscillating cylinder. J. Fluids Struct. 15, 523–532.Google Scholar
Carberry, J., Sheridan, J. & Rockwell, D. 2005 Controlled oscillations of a cylinder: forces and wake modes. J. Fluid Mech. 538, 31–69.Google Scholar
Chew, Y. T., Cheng, M. & Luo, S. C. 1995 A numerical study of flow past a rotating circular cylinder using a hybrid vortex scheme. J. Fluid Mech. 299, 35–71.Google Scholar
Corless, R. M. & Parkinson, G. V. 1988 A model of the combined effects of vortex-induced oscillation and galloping. J. Fluids Struct. 2, 203–220.CrossRefGoogle Scholar
Coutanceau, M. & Ménard, C. 1985 Influence of rotation on the near-wake development behind an impulsively started circular cylinder. J. Fluid Mech. 158, 399–446.Google Scholar
Dahl, J. M., Hover, F. S., Triantafyllou, M. S., Dong, S. & Karniadakis, G. E. 2007 Resonant vibrations of bluff bodies cause multivortex shedding and high frequency forces. Phys. Rev. Lett. 99, 144503.CrossRefGoogle ScholarPubMed
Dahl, J. M., Hover, F. S., Triantafyllou, M. S. & Oakley, O. H. 2010 Dual resonance in vortex-induced vibrations at subcritical and supercritical Reynolds numbers. J. Fluid Mech. 643, 395–424.Google Scholar
El Akoury, R., Braza, M., Perrin, R., Harran, G. & Hoarau, Y. 2008 The three-dimensional transition in the flow around a rotating cylinder. J. Fluid Mech. 607, 1–11.CrossRefGoogle Scholar
Evangelinos, C. & Karniadakis, G. E. 1999 Dynamics and flow structures in the turbulent wake of rigid and flexible cylinders subject to vortex-induced vibrations. J. Fluid Mech. 400, 91–124.Google Scholar
Govardhan, R. & Williamson, C. H. K. 2000 Modes of vortex formation and frequency response of a freely vibrating cylinder. J. Fluid Mech. 420, 85–130.Google Scholar
Hover, F. S., Techet, A. H. & Triantafyllou, M. S. 1998 Forces on oscillating uniform and tapered cylinders in crossflow. J. Fluid Mech. 363, 97–114.CrossRefGoogle Scholar
Jauvtis, N. & Williamson, C. H. K. 2004 The effect of two degrees of freedom on vortex-induced vibration at low mass and damping. J. Fluid Mech. 509, 23–62.Google Scholar
Jeon, D. & Gharib, M. 2001 On circular cylinders undergoing two-degree-of-freedom forced motions. J. Fluids Struct. 15, 533–541.Google Scholar
Kang, S., Choi, H. & Lee, S. 1999 Laminar flow past a rotating circular cylinder. Phys. Fluids 11, 3312–3321.Google Scholar
Karniadakis, G. E. & Sherwin, S. 1999 Spectral/hp Element Methods for CFD. 1st edn. Oxford University Press.Google Scholar
Khalak, A. & Williamson, C. H. K. 1999 Motions, forces and mode transitions in vortex-induced vibrations at low mass-damping. J. Fluids Struct. 13, 813–851.Google Scholar
Kim, J., Kim, D. & Choi, H. 2001 An immersed-boundary finite-volume method for simulations of flow in complex geometries. J. Comput. Phys. 171, 132–150.Google Scholar
Klamo, J. T., Leonard, A. & Roshko, A. 2006 The effects of damping on the amplitude and frequency response of a freely vibrating cylinder in cross-flow. J. Fluids Struct. 22, 845–856.Google Scholar
Leontini, J. S., Stewart, B. E., Thompson, M. C. & Hourigan, K. 2006 Wake state and energy transitions of an oscillating cylinder at low Reynolds number. Phys. Fluids 18, 067101.Google Scholar
Leontini, J. S., Thompson, M. C. & Hourigan, K. 2007 Three-dimensional transition in the wake of a transversely oscillating cylinder. J. Fluid Mech. 577, 79–104.Google Scholar
Lucor, D. & Triantafyllou, M. S. 2008 Parametric study of a two degree-of-freedom cylinder subject to vortex-induced vibrations. J. Fluids Struct. 24, 1284–1293.CrossRefGoogle Scholar
Meena, J., Sidarth, G. S., Khan, M. H. & Mittal, S. 2011 Three-dimensional instabilities in flow past a spinning and translating cylinder. In IUTAM Symposium on Bluff Body Flows, IIT-Kanpur, India (ed. Mittal, S. & Biswas, G.), pp. 75–78.Google Scholar
Mittal, S. 2004 A finite element study of incompressible flows past oscillating cylinders and aerofoils. J. Appl. Mech. 71, 89–95.Google Scholar
Mittal, S. & Kumar, B. 2003 Flow past a rotating cylinder. J. Fluid Mech. 476, 303–334.CrossRefGoogle Scholar
Mittal, S. & Tezduyar, T. E. 1992 A finite element study of incompressible flows past oscillating cylinders and aerofoils. Intl J. Numer. Meth. Fluids 15, 1073–1118.Google Scholar
Modarres-Sadeghi, Y., Mukundan, H., Dahl, J. M., Hover, F. S. & Triantafyllou, M. S. 2010 The effect of higher harmonic forces on fatigue life of marine risers. J. Sound Vib. 329, 43–55.Google Scholar
Modi, V. J. 1997 Moving surface boundary-layer control: a review. J. Fluids Struct. 11, 627–663.CrossRefGoogle Scholar
Naudascher, E. & Rockwell, D. 1994 Flow-Induced Vibrations: an Engineering Guide. Dover.Google Scholar
Navrose,  & Mittal, S. 2013 Free vibrations of a cylinder: 3-D computations at $Re=1000$. J. Fluids Struct. 41, 109–118.CrossRefGoogle Scholar
Nemes, A., Zhao, J., Lo Jacono, D. & Sheridan, J. 2012 The interaction between flow-induced vibration mechanisms of a square cylinder with varying angles of attack. J. Fluid Mech. 710, 102–130.CrossRefGoogle Scholar
Newman, D. J. & Karniadakis, G. E. 1997 A direct numerical simulation study of flow past a freely vibrating cable. J. Fluid Mech. 344, 95–136.Google Scholar
Païdoussis, M. P., Price, S. J. & de Langre, E. 2010 Fluid–Structure Interactions: Cross-Flow-Induced Instabilities. Cambridge University Press.CrossRefGoogle Scholar
Pralits, J. O., Brandt, L. & Giannetti, F. 2010 Instability and sensitivity of the flow around a rotating circular cylinder. J. Fluid Mech. 650, 513–536.Google Scholar
Pralits, J. O., Giannetti, F. & Brandt, L. 2013 Three-dimensional instability of the flow around a rotating circular cylinder. J. Fluid Mech. 730, 5–18.Google Scholar
Prandtl, L. 1926 Application of the ‘Magnus effect’ to the wind propulsion of ships. NACA Tech. Mem. 367.Google Scholar
Prasanth, T. K. & Mittal, S. 2008 Vortex-induced vibrations of a circular cylinder at low Reynolds numbers. J. Fluid Mech. 594, 463–491.CrossRefGoogle Scholar
Raghavan, K. & Bernitsas, M. M. 2011 Experimental investigation of Reynolds number effect on vortex-induced vibration of rigid circular cylinder on elastic supports. Ocean Engng 38, 719–731.Google Scholar
Rao, A., Leontini, J. S., Thompson, M. C. & Hourigan, K. 2013a Three-dimensionality in the wake of a rapidly rotating cylinder in uniform flow. J. Fluid Mech. 730, 379–391.Google Scholar
Rao, A., Leontini, J. S., Thompson, M. C. & Hourigan, K. 2013b Three-dimensionality in the wake of a rotating cylinder in a uniform flow. J. Fluid Mech. 717, 1–29.Google Scholar
Sarpkaya, T. 1979 Vortex-induced oscillations: a selective review. Trans. ASME: J. Appl. Mech. 46, 241–258.Google Scholar
Sarpkaya, T. 2004 A critical review of the intrinsic nature of vortex-induced vibrations. J. Fluids Struct. 19, 389–447.CrossRefGoogle Scholar
Shen, L., Chan, E.-S. & Lin, P. 2009 Calculation of hydrodynamic forces acting on a submerged moving object using immersed boundary method. Comput. Fluids 38, 691–702.CrossRefGoogle Scholar
Shiels, D., Leonard, A. & Roshko, A. 2001 Flow-induced vibration of a circular cylinder at limiting structural parameters. J. Fluids Struct. 15, 3–21.Google Scholar
Singh, S. P. & Mittal, S. 2005 Vortex-induced oscillations at low Reynolds numbers: hysteresis and vortex-shedding modes. J. Fluids Struct. 20, 1085–1104.Google Scholar
Stansby, P. K. & Rainey, R. C. T. 2001 On the orbital response of a rotating cylinder in a current. J. Fluid Mech. 439, 87–108.Google Scholar
Stansby, P. K. & Slaouti, A. 1993 Simulation of vortex shedding including blockage by the random-vortex and other methods. Intl J. Numer. Meth. Fluids 17, 1003–1013.Google Scholar
Stojković, D., Breuer, M. & Durst, F. 2002 Effect of high rotation rates on the laminar flow around a circular cylinder. Phys. Fluids 14, 3160–3178.Google Scholar
Stojković, D., Schön, P., Breuer, M. & Durst, F. 2003 On the new vortex shedding mode past a rotating circular cylinder. Phys. Fluids 15, 1257–1260.Google Scholar
Vandiver, J. K., Jaiswal, V. & Jhingran, V. 2009 Insights on vortex-induced, travelling waves on long risers. J. Fluids Struct. 25, 641–653.Google Scholar
Vikestad, K., Vandiver, J. K. & Larsen, C. M. 2000 Added mass and oscillation frequency for a circular cylinder subjected to vortex-induced vibrations and external disturbance. J. Fluids Struct. 14, 1071–1088.Google Scholar
Wang, X. Q., So, R. M. C. & Chan, K. T. 2003 A nonlinear fluid force model for vortex-induced vibration of an elastic cylinder. J. Sound Vib. 260, 287–305.Google Scholar
Williamson, C. H. K. 1988 The existence of two stages in the transition to three-dimensionality of a cylinder wake. Phys. Fluids 31, 3165–3168.Google Scholar
Williamson, C. H. K. & Govardhan, R. 2004 Vortex-induced vibrations. Annu. Rev. Fluid Mech. 36, 413–455.Google Scholar
Williamson, C. H. K. & Roshko, A. 1988 Vortex formation in the wake of an oscillating cylinder. J. Fluids Struct. 2, 355–381.Google Scholar
Wu, X., Ge, F. & Hong, Y. 2012 A review of recent studies on vortex-induced vibrations of long slender cylinders. J. Fluids Struct. 28, 292–308.Google Scholar
Yogeswaran, V. & Mittal, S. 2011 Vortex-induced and galloping response of a rotating circular cylinder. In IUTAM Symposium on Bluff Body Flows, IIT-Kanpur, India (ed. Mittal, S. & Biswas, G.), pp. 153–156.Google Scholar
Zhao, M., Cheng, L. & Zhou, T. 2013 Numerical simulation of vortex-induced vibration of a square cylinder at a low Reynolds number. Phys. Fluids 25, 023603.Google Scholar
Zhou, C. Y., So, R. M. C. & Lam, K. 1999 Vortex-induced vibrations of an elastic circular cylinder. J. Fluids Struct. 13, 165–189.CrossRefGoogle Scholar