Hostname: page-component-78c5997874-4rdpn Total loading time: 0 Render date: 2024-11-17T13:17:01.589Z Has data issue: false hasContentIssue false
  • English
  • Français

Flow regimes for a square cross-section cylinder in oscillatory flow

Published online by Cambridge University Press:  17 January 2017

Feifei Tong ,
Liang Cheng ,
Chengwang Xiong ,
Scott Draper ,
Hongwei An  and
Xiaofan Lou
Feifei Tong*
Affiliation:
School of Civil, Environmental and Mining Engineering, The University of Western Australia, 35 Stirling Highway, Perth, WA 6009, Australia
Liang Cheng
Affiliation:
School of Civil, Environmental and Mining Engineering, The University of Western Australia, 35 Stirling Highway, Perth, WA 6009, Australia State Key Laboratory of Coastal and Offshore Engineering, Dalian University of Technology, Dalian, 116024, China
Chengwang Xiong
Affiliation:
School of Civil, Environmental and Mining Engineering, The University of Western Australia, 35 Stirling Highway, Perth, WA 6009, Australia
Scott Draper
Affiliation:
School of Civil, Environmental and Mining Engineering, The University of Western Australia, 35 Stirling Highway, Perth, WA 6009, Australia Centre of Offshore Foundation System, The University of Western Australia, 35 Stirling Highway, Perth, WA 6009, Australia
Hongwei An
Affiliation:
School of Civil, Environmental and Mining Engineering, The University of Western Australia, 35 Stirling Highway, Perth, WA 6009, Australia
Xiaofan Lou
Affiliation:
School of Civil, Environmental and Mining Engineering, The University of Western Australia, 35 Stirling Highway, Perth, WA 6009, Australia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Two-dimensional direct numerical simulation and Floquet stability analysis have been performed at moderate Keulegan–Carpenter number ($KC$) and low Reynolds number (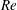$Re$) for a square cross-section cylinder with its face normal to the oscillatory flow. Based on the numerical simulations a map of flow regimes is formed and compared to the map of flow around an oscillating circular cylinder by Tatsuno & Bearman (J. Fluid Mech., vol. 211, 1990, pp. 157–182). Two new flow regimes have been observed, namely A$^{\prime }$ and F$^{\prime }$. 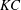The regime A$^{\prime }$ found at low $KC$ is characterised by the transverse convection of fluid particles perpendicular to the motion; and the regime F$^{\prime }$ found at high $KC$ shows a quasi-periodic feature with a well-defined secondary period, which is larger than the oscillation period. The Floquet analysis demonstrates that when the two-dimensional flow breaks the reflection symmetry about the axis of oscillation, the quasi-periodic instability and the synchronous instability with the imposed oscillation occur alternately for the square cylinder along the curve of marginal stability. This alternate pattern in instabilities leads to four distinct flow regimes. When compared to the vortex shedding in otherwise unidirectional flow, the two quasi-periodic flow regimes are observed when the oscillation frequency is close to the Strouhal frequency (or to half of it). Both the flow regimes and marginal stability curve shift in the $(Re,KC)$-space compared to the oscillatory flow around a circular cylinder and this shift appears to be consistent with the change in vortex formation time associated with the lower Strouhal frequency of the square cylinder.

JFM classification

Instability: Instability Low-Reynolds-number flows: Low-Reynolds-number flows Vortex Flows: Vortex Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 813 , 25 February 2017 , pp. 85 - 109
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

An, H., Cheng, L. & Zhao, M. 2011 Direct numerical simulation of oscillatory flow around a circular cylinder at low Keulegan–Carpenter number. J. Fluid Mech. 666, 77–103.Google Scholar
Barkley, D. & Henderson, R. D. 1996 Three-dimensional Floquet stability analysis of the wake of a circular cylinder. J. Fluid Mech. 322, 215–241.Google Scholar
Barrero-Gil, A. 2011 Hydrodynamic in-line force coefficients of oscillating bluff cylinders (circular and square) at low Reynolds numbers. J. Vib. Acoust. 133 (5), 051012.Google Scholar
Bearman, P. W., Downie, M. J., Graham, J. M. R. & Obasaju, E. D. 1985 Forces on cylinders in viscous oscillatory flow at low Keulegan–Carpenter numbers. J. Fluid Mech. 154, 337–356.Google Scholar
Bearman, P. W., Graham, J. M. R., Obasaju, E. D. & Drossopoulos, G. M. 1984 The influence of corner radius on the forces experienced by cylindrical bluff bodies in oscillatory flow. Appl. Ocean Res. 6 (2), 83–89.Google Scholar
Cantwell, C. D., Moxey, D., Comerford, A., Bolis, A., Rocco, G., Mengaldo, G., De Grazia, D., Yakovlev, S., Lombard, J.-E., Ekelschot, D. et al. 2015 Nektar++: An open-source spectral/hp element framework. Comput. Phys. Commun. 192, 205–219.Google Scholar
Chern, M.-J., Lu, Y.-J., Chang, S.-C. & Cheng, I.-C. 2007 Interaction of oscillatory flows with a square cylinder. J. Mech. 23 (04), 445–450.CrossRefGoogle Scholar
Deng, J. & Caulfield, C. P. 2016 Dependence on aspect ratio of symmetry breaking for oscillating foils: implications for flapping flight. J. Fluid Mech. 787, 16–49.Google Scholar
Elston, J. R., Blackburn, H. M. & Sheridan, J. 2006 The primary and secondary instabilities of flow generated by an oscillating circular cylinder. J. Fluid Mech. 550, 359–389.Google Scholar
Elston, J. R., Sheridan, J. & Blackburn, H. M. 2004 Two-dimensional Floquet stability analysis of the flow produced by an oscillating circular cylinder in quiescent fluid. Eur. J. Mech. (B/Fluids) 23 (1), 99–106.Google Scholar
Graham, J. M. R. 1980 The forces on sharp-edged cylinders in oscillatory flow at low Keulegan–Carpenter numbers. J. Fluid Mech. 97 (02), 331–346.Google Scholar
Honji, H. 1981 Streaked flow around an oscillating circular cylinder. J. Fluid Mech. 107, 509–520.Google Scholar
Justesen, P. 1991 A numerical study of oscillating flow around a circular cylinder. J. Fluid Mech. 222, 157–196.CrossRefGoogle Scholar
Karniadakis, G. E., Israeli, M. & Orszag, S. A. 1991 High-order splitting methods for the incompressible Navier–Stokes equations. J. Comput. Phys. 97 (2), 414–443.Google Scholar
Keulegan, G. H. & Carpenter, L. H. 1958 Forces on cylinders and plates in an oscillating fluid. J. Res. Natl Bur. Stand. 60 (5), 423–440.Google Scholar
Koehler, C., Beran, P., Vanella, M. & Balaras, E. 2015 Flows produced by the combined oscillatory rotation and translation of a circular cylinder in a quiescent fluid. J. Fluid Mech. 764, 148–170.CrossRefGoogle Scholar
Nehari, D., Armenio, V. & Ballio, F. 2004 Three-dimensional analysis of the unidirectional oscillatory flow around a circular cylinder at low Keulegan–Carpenter and 𝛽 numbers. J. Fluid Mech. 520, 157–186.Google Scholar
Okajima, A. 1982 Strouhal numbers of rectangular cylinders. J. Fluid Mech. 123, 379–398.Google Scholar
Sarpkaya, T.1976 Vortex shedding and resistance in harmonic flow about smooth and rough circular cylinders at high Reynolds numbers. Tech. Rep. NPS-59SL7602. Naval Postgraduate School, Monterey, CA.Google Scholar
Sarpkaya, T. 2002 Experiments on the stability of sinusoidal flow over a circular cylinder. J. Fluid Mech. 457, 157–180.Google Scholar
Scolan, Y.-M. & Faltinsen, O. M. 1994 Numerical studies of separated flow from bodies with sharp corners by the vortex in cell method. J. Fluids Struct. 8 (2), 201–230.Google Scholar
Smith, P. A. & Stansby, P. K. 1991 Viscous oscillatory flows around cylindrical bodies at low Keulegan–Carpenter numbers using the vortex method. J. Fluids Struct. 5 (4), 339–361.Google Scholar
Sohankar, A., Norberg, C. & Davidson, L. 1997 Numerical simulation of unsteady low-Reynolds number flow around rectangular cylinders at incidence. J. Wind Engng Ind. Aerodyn. 69, 189–201.Google Scholar
Sohankar, A., Norberg, C. & Davidson, L. 1998 Low-Reynolds-number flow around a square cylinder at incidence: study of blockage, onset of vortex shedding and outlet boundary condition. Intl J. Numer. Meth. Fluids 26 (1), 39–56.Google Scholar
Stokes, G. G. 1851 On the effect of the internal friction of fluids on the motion of pendulums. Trans. Camb. Phil. Soc. 9, 8–106.Google Scholar
Tatsuno, M. & Bearman, P. W. 1990 A visual study of the flow around an oscillating circular cylinder at low Keulegan–Carpenter numbers and low Stokes numbers. J. Fluid Mech. 211, 157–182.Google Scholar
Tong, F., Cheng, L., Zhao, M. & An, H. 2015 Oscillatory flow regimes around four cylinders in a square arrangement under small KC and Re conditions. J. Fluid Mech. 769, 298–336.Google Scholar
Troesch, A. W. & Kim, S. K. 1991 Hydrodynamic forces acting on cylinders oscillating at small amplitudes. J. Fluids Struct. 5 (1), 113–126.Google Scholar
Venugopal, V., Varyani, K. S. & Barltrop, N. D. 2006 Wave force coefficients for horizontally submerged rectangular cylinders. Ocean Engng 33 (11), 1669–1704.Google Scholar
Wang, C.-Y. 1968 On high-frequency oscillatory viscous flows. J. Fluid Mech. 32 (01), 55–68.Google Scholar
Williamson, C. H. K. 1985 Sinusoidal flow relative to circular cylinders. J. Fluid Mech. 155, 141–174.Google Scholar
Williamson, C. H. K. 1996 Vortex dynamics in the cylinder wake. Annu. Rev. Fluid. Mech. 28 (1), 477–539.Google Scholar
Williamson, C. H. K. & Brown, G. L. 1998 A series in 1/√Re to represent the Strouhal–Reynolds number relationship of the cylinder wake. J. Fluids Struct. 12 (8), 1073–1085.CrossRefGoogle Scholar
Zhao, M. & Cheng, L. 2014 Two-dimensional numerical study of vortex shedding regimes of oscillatory flow past two circular cylinders in side-by-side and tandem arrangements at low Reynolds numbers. J. Fluid Mech. 751, 1–37.Google Scholar
Zheng, W. & Dalton, C. 1999 Numerical prediction of force on rectangular cylinders in oscillating viscous flow. J. Fluids Struct. 13 (2), 225–249.CrossRefGoogle Scholar