Hostname: page-component-78c5997874-94fs2 Total loading time: 0 Render date: 2024-11-16T16:58:37.451Z Has data issue: false hasContentIssue false
  • English
  • Français

Flight-crash events in superfluid turbulence

Published online by Cambridge University Press:  01 August 2019

P. Švančara  and
M. La Mantia Open the ORCID record for M. La Mantia [Opens in a new window]
P. Švančara
Affiliation:
Faculty of Mathematics and Physics, Charles University, Ke Karlovu 3, 121 16 Prague, Czech Republic
M. La Mantia*
Affiliation:
Faculty of Mathematics and Physics, Charles University, Ke Karlovu 3, 121 16 Prague, Czech Republic
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

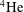We show experimentally that the mechanisms of energy transport in turbulent flows of superfluid $^{4}\text{He}$ are strikingly different from those occurring in turbulent flows of viscous fluids. We argue that the result can be related to the role played by quantized vortices in this unique type of turbulence. The flow-induced motions of relatively small particles suspended in the liquid reveal that, for scales of the order of the mean distance between the vortices, the particles do not tend on average to decelerate faster than they accelerate, whereas, at larger scales, a classical-like asymmetry is recovered. It follows that, in the range of investigated parameters, flight-crash events are less apparent than in classical turbulence. We specifically link the outcome to the time symmetry of quantized vortex reconnections observed at scales comparable to the typical particle size.

JFM classification

Complex Fluids: Quantum fluids Turbulent Flows: Turbulent Flows
Type
JFM Rapids
Information
Journal of Fluid Mechanics , Volume 876 , 10 October 2019 , R2
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Barenghi, C. F., Skrbek, L. & Sreenivasan, K. R. 2014 Introduction to quantum turbulence. Proc. Natl Acad. Sci. USA 111, 4647–4652.Google Scholar
Bhatnagar, A., Gupta, A., Mitra, D. & Pandit, R. 2018 Heavy inertial particles in turbulent flows gain energy slowly but lose it rapidly. Phys. Rev. E 97, 033102.Google Scholar
Clark di Leoni, P., Mininni, P. D. & Brachet, M. E. 2017 Dual cascade and dissipation mechanisms in helical quantum turbulence. Phys. Rev. A 95, 053636.Google Scholar
Guo, W., La Mantia, M., Lathrop, D. P. & Van Sciver, S. W. 2014 Visualization of two-fluid flows of superfluid helium-4. Proc. Natl Acad. Sci. USA 111, 4653–4658.Google Scholar
Hussain, F. & Duraisamy, K. 2011 Mechanics of viscous vortex reconnection. Phys. Fluids 23, 021701.Google Scholar
La Mantia, M. 2016 Particle trajectories in thermal counterflow of superfluid helium in a wide channel of square cross section. Phys. Fluids 28, 024102.Google Scholar
La Mantia, M. 2017 Particle dynamics in wall-bounded thermal counterflow of superfluid helium. Phys. Fluids 29, 065102.Google Scholar
La Mantia, M. & Skrbek, L. 2014 Quantum, or classical turbulence? Europhys. Lett. 105, 46002.Google Scholar
La Mantia, M., Švančara, P., Duda, D. & Skrbek, L. 2016 Small-scale universality of particle dynamics in quantum turbulence. Phys. Rev. B 94, 184512.Google Scholar
Lévêque, E. & Naso, A. 2014 Introduction of longitudinal and transverse Lagrangian velocity increments in homogeneous and isotropic turbulence. Europhys. Lett. 108, 54004.Google Scholar
Loisy, A. & Naso, A. 2017 Interaction between a large buoyant bubble and turbulence. Phys. Rev. Fluids 2, 014606.Google Scholar
McKeown, R., Ostilla-Mónico, R., Pumir, A., Brenner, M. P. & Rubinstein, S. M. 2018 Cascade leading to the emergence of small structures in vortex ring collisions. Phys. Rev. Fluids 3, 124702.Google Scholar
Mongiovì, M. S., Jou, D. & Sciacca, M. 2018 Non-equilibrium thermodynamics, heat transport and thermal waves in laminar and turbulent superfluid helium. Phys. Rep. 726, 1–71.Google Scholar
Paoletti, M. S., Fisher, M. E. & Lathrop, D. P. 2010 Reconnection dynamics for quantized vortices. Physica D 239, 1367–1377.Google Scholar
Pumir, A., Xu, H., Bodenschatz, E. & Grauer, R. 2016 Single-particle motion and vortex stretching in three-dimensional turbulent flows. Phys. Rev. Lett. 116, 124502.Google Scholar
Stelzenmuller, N.2018 A Lagrangian study of inhomogeneous turbulence PhD thesis, Université de Grenoble-Alpes.Google Scholar
Švančara, P., Hrubcová, P., Rotter, M. & La Mantia, M. 2018 Visualization study of thermal counterflow of superfluid helium in the proximity of the heat source by using solid deuterium hydride particles. Phys. Rev. Fluids 3, 114701.Google Scholar
Švančara, P. & La Mantia, M. 2017 Flows of liquid 4He due to oscillating grids. J. Fluid Mech. 832, 578–599.Google Scholar
Villois, A., Proment, D. & Krstulovic, G. 2017 Universal and nonuniversal aspects of vortex reconnections in superfluids. Phys. Rev. Fluids 2, 044701.Google Scholar
Xu, H., Pumir, A., Falkovich, G., Bodenschatz, E., Shats, M., Xia, H., Francois, N. & Boffetta, G. 2014 Flight-crash events in turbulence. Proc. Natl Acad. Sci. USA 111, 7558–7563.Google Scholar
Zuccher, S., Caliari, M., Baggaley, A. W. & Barenghi, C. F. 2012 Quantum vortex reconnections. Phys. Fluids 24, 125108.Google Scholar