Hostname: page-component-745bb68f8f-lrblm Total loading time: 0 Render date: 2025-01-09T21:37:05.374Z Has data issue: false hasContentIssue false
  • English
  • Français

Film spreading from a miscible drop on a deep liquid layer

Published online by Cambridge University Press:  14 September 2017

Raj Dandekar ,
Anurag Pant  and
Baburaj A. Puthenveettil Open the ORCID record for Baburaj A. Puthenveettil [Opens in a new window]
Raj Dandekar
Affiliation:
Department of Mechanical Engineering, Indian Institute of Technology Madras, Chennai, 600036, India
Anurag Pant
Affiliation:
Department of Applied Mechanics, Indian Institute of Technology Madras, Chennai, 600036, India
Baburaj A. Puthenveettil*
Affiliation:
Department of Applied Mechanics, Indian Institute of Technology Madras, Chennai, 600036, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We study the spreading of a film from ethanol–water droplets of radii 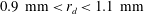$0.9~\text{mm}<r_{d}<1.1~\text{mm}$ on the surface of a deep water layer for various concentrations of ethanol in the drop. Since the drop is lighter ($\unicode[STIX]{x1D709}=\unicode[STIX]{x1D70C}_{l}/\unicode[STIX]{x1D70C}_{d}>1.03$), it stays at the surface of the water layer during the spreading of the film from the drop; the film is more viscous than the underlying water layer since 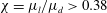$\unicode[STIX]{x1D712}=\unicode[STIX]{x1D707}_{l}/\unicode[STIX]{x1D707}_{d}>0.38$. Inertial forces are not dominant in the spreading since the Reynolds numbers based on the film thickness 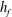$h_{f}$ are in the range 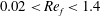$0.02<Re_{f}<1.4$. The spreading is surface-tension-driven since the film capillary numbers are in the range 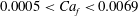$0.0005<Ca_{f}<0.0069$ and the drop Bond numbers are in the range 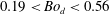$0.19<Bo_{d}<0.56$. We observe that, when the drop is brought in contact with the water surface, capillary waves propagate from the point of contact, followed by a radially expanding, thin circular film of ethanol–water mixture. The film develops instabilities at some radius to form outward-moving fingers at its periphery while it is still expanding, till the expansion stops at a larger radius. The film then retracts, during which time the remaining major part of the drop, which stays at the centre of the expanding film, thins and develops holes and eventually mixes completely with water. The radius of the expanding front of the film scales as 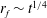$r_{f}\sim t^{1/4}$ and shows a dependence on the concentration of ethanol in the drop as well as on 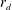$r_{d}$, and is independent of the layer height $h_{l}$. Using a balance of surface tension and viscous forces within the film, along with a model for the fraction of the drop that forms the thin film, we obtain an expression for the dimensionless film radius $r_{f}^{\ast }=r_{f}/r_{d}$, in the form 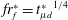$fr_{f}^{\ast }={t_{\unicode[STIX]{x1D707}d}^{\ast }}^{1/4}$, where 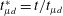$t_{\unicode[STIX]{x1D707}d}^{\ast }=t/t_{\unicode[STIX]{x1D707}d}$, with the time scale 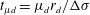$t_{\unicode[STIX]{x1D707}d}=\unicode[STIX]{x1D707}_{d}r_{d}/\unicode[STIX]{x0394}\unicode[STIX]{x1D70E}$ and 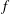$f$ is a function of 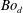$Bo_{d}$. Similarly, we show that the dimensionless velocity of film spreading, $Ca_{d}=u_{f}\unicode[STIX]{x1D707}_{d}/\unicode[STIX]{x0394}\unicode[STIX]{x1D70E}$, scales as 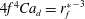$4f^{4}Ca_{d}={r_{f}^{\ast }}^{-3}$.

JFM classification

Drops and Bubbles: Drops Interfacial Flows (free surface): Interfacial Flows (free surface) Interfacial Flows (free surface): Thin films
Type
Papers
Information
Journal of Fluid Mechanics , Volume 829 , 25 October 2017 , pp. 304 - 327
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Afsar-Siddiqui, A., Luckham, P. F. & Matar, O. M. 2003 Unstable spreading of aqueous anionic surfactant solutions on liquid films. 2. Highly soluble surfactant. Langmuir 19, 703–708.Google Scholar
Bacri, L., Debregeas, G. & Brochard-Wyart, F. 1996 Experimental study of the spreading of a viscous droplet on a non viscous liquid. Langmuir 12, 6708–6711.Google Scholar
Blanchette, F., Messio, L. & Bush, J. W. M. 2009 The influence of surface tension gradients on drop coalescence. Phys. Fluids 21 (7), 072107.Google Scholar
Chen, X., Mandre, S. & Feng, J. J. 2006 Partial coalescence between a drop and a liquid–liquid interface. Phys. Fluids 18 (5), 051705.Google Scholar
Clanet, C. & Lasheras, J. C. 1999 Transition from dripping to jetting. J. Fluid Mech. 383, 307–326.Google Scholar
Dong, L. & Johnson, D. 2003 Surface tension of charge-stabilized colloidal suspensions at the water–air interface. Langmuir 19 (24), 10205–10209.CrossRefGoogle Scholar
Dussaud, A. D., Matar, O. K. & Troian, S. M. 2005 Spreading characteristics of an insoluble surfactant film on a thin liquid layer: comparison between theory and experiment. J. Fluid Mech. 544, 23–51.CrossRefGoogle Scholar
Dussaud, A. D. & Troian, S. M. 1998 Dynamics of spontaneous spreading with evaporation on a deep fluid layer. Phys. Fluids 10 (1), 23–38.Google Scholar
Eggers, J., Lister, J. R. & Stone, H. A. 1999 Coalescence of liquid drops. J. Fluid Mech. 401, 293–310.CrossRefGoogle Scholar
Ernst, R. C., Watkins, C. H. & Ruwe, H. H. 1936 The physical properties of the ternary system ethyl–alcohol–glycerin–water. J. Phys. Chem. 40 (5), 627–635.Google Scholar
Fay, J. A. 1969 The spread of oil slicks on a calm sea. In Oil on the Sea, pp. 53–63. Springer.CrossRefGoogle Scholar
Foda, M. & Cox, R. G. 1980 The spreading of thin liquid films on a water–air interface. J. Fluid Mech. 101 (1), 33–51.Google Scholar
Fraaije, J. G. E. M. & Cazabat, A. M. 1989 Dynamics of spreading on a liquid substrate. J. Colloid Interface Sci. 133 (2), 452–460.Google Scholar
Grotberg, J. B. 1994 Pulmonary flow and transport phenomena. Annu. Rev. Fluid Mech. 26 (1), 529–571.Google Scholar
Grotberg, J. B. & Gaver, D. P. III 1996 A synopsis of surfactant spreading research. J. Colloid Interface Sci. 178 (1), 377–378.Google Scholar
Halpern, D. & Grotberg, J. B. 1992 Dynamics of transport of a localised soluble surfactant on a thin film. J. Fluid Mech. 237, 1–11.Google Scholar
Hernández-Sánchez, J. F., Eddi, A. & Snoeijer, J. H. 2015 Marangoni spreading due to a localized alcohol supply on a thin water film. Phys. Fluids 27 (3), 032003.Google Scholar
Jensen, O. E. 1995 The spreading of insoluble surfactant at the free surface of a deep fluid layer. J. Fluid Mech. 293, 349–378.Google Scholar
Jensen, O. E. & Grotberg, J. B. 1992 Insoluble surfactant spreading on a thin viscous film: shock evolution and film rupture. J. Fluid Mech. 240, 259–288.CrossRefGoogle Scholar
Jensen, O. E. & Grotberg, J. B. 1993 The spreading of heat or soluble surfactant along a thin liquid film. Phys. Fluids A 5 (1), 58–68.CrossRefGoogle Scholar
Jensen, O. E. & Halpern, D. 1998 The stress singularity in surfactant-driven thin-film flows. Part 1. Viscous effects. J. Fluid Mech. 372, 273–300.Google Scholar
Joos, P. & Pintens, J. 1977 Spreading kinetics of liquids on liquids. J. Colloid Interfacial Sci. 60, 507–513.CrossRefGoogle Scholar
Joos, P. & Van Hunsel, J. 1985 Spreading of aqueous surfactant solutions on organic liquids. J. Colloid Interfacial Sci. 106 (1), 161–167.Google Scholar
Khattab, I. S., Bandarkar, F., Fakhree, M. A. & Jouban, A. 2012 Density, viscosity and surface tension of water–ethanol mixtures from 293 to 323 K. Korean J. Chem. Engng 29 (6), 812–817.CrossRefGoogle Scholar
La Due, J., Muller, M. R. & Swangler, M. J. 1996 Cratering phenomena on aircraft anti-icing films. J. Aircraft 33, 131–138.CrossRefGoogle Scholar
Landt, E. & Volmer, M. 1926 Spreading velocity of oil on H2 O. Z. Phys. Chem. 122, 398.Google Scholar
Le, H. P. 1998 Progress and trends in ink-jet printing technology. J. Imaging Sci. Technol. 42 (1), 42.Google Scholar
Lock, G. S. H. 1996 Latent Heat Transfer: An Introduction to Fundamentals, No. 43, pp. 124–126. Oxford University Press.Google Scholar
Warner, M. R. E., Craster, R. V. & Matar, O. K. 2004 Fingering phenomena created by a soluble surfactant deposition on a thin liquid film. Phys. Fluids 16 (8), 2933–2951.CrossRefGoogle Scholar

Dandekar et al. supplementary material movie 1

Top view of the coalescence and film spreading of an ethanol drop of 100% concentration and radius rd=0.97 mm mixes with a water layer at Delta sigma =. 31.9 mN m -1. The area of imaging is of size 87.75 mm x 35.50 mm. The movie is at 25fps of the phenomena that was captured at 800fps

Download Dandekar et al. supplementary material movie 1(Video)
Video 10.2 MB

Dandekar et al. supplementary material movie 2

Side view of the coalescence and film spreading when an ethanol drop of 100% concentration and radius rd=1.1 mm mixes with a water layer of height hl=5 cm. The movie is at 25 FPS of the phenomena that was captured at 2000FPS.

Download Dandekar et al. supplementary material movie 2(Video)
Video 9.9 MB