Hostname: page-component-586b7cd67f-l7hp2 Total loading time: 0 Render date: 2024-11-26T08:41:06.883Z Has data issue: false hasContentIssue false
  • English
  • Français

Falkner–Skan boundary layer approximation in Rayleigh–Bénard convection

Published online by Cambridge University Press:  01 August 2013

Olga Shishkina ,
Susanne Horn  and
Sebastian Wagner
Olga Shishkina*
Affiliation:
DLR - Institute for Aerodynamics and Flow Technology, Bunsenstraße 10, 37073 Göttingen, Germany
Susanne Horn
Affiliation:
DLR - Institute for Aerodynamics and Flow Technology, Bunsenstraße 10, 37073 Göttingen, Germany
Sebastian Wagner
Affiliation:
DLR - Institute for Aerodynamics and Flow Technology, Bunsenstraße 10, 37073 Göttingen, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

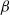To approximate the velocity and temperature within the boundary layers in turbulent thermal convection at moderate Rayleigh numbers, we consider the Falkner–Skan ansatz, which is a generalization of the Prandtl–Blasius one to a non-zero-pressure-gradient case. This ansatz takes into account the influence of the angle of attack $\beta $ of the large-scale circulation of a fluid inside a convection cell against the heated/cooled horizontal plate. With respect to turbulent Rayleigh–Bénard convection, we derive several theoretical estimates, among them the limiting cases of the temperature profiles for all angles $\beta $, for infinite and for infinitesimal Prandtl numbers 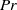$\mathit{Pr}$. Dependences on 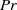$\mathit{Pr}$ and 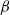$\beta $ of the ratio of the thermal to viscous boundary layers are obtained from the numerical solutions of the boundary layers equations. For particular cases of 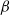$\beta $, accurate approximations are developed as functions on $\mathit{Pr}$. The theoretical results are corroborated by our direct numerical simulations for 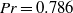$\mathit{Pr}= 0. 786$ (air) and 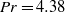$\mathit{Pr}= 4. 38$ (water). The angle of attack $\beta $ is estimated based on the information on the locations within the plane of the large-scale circulation where the time-averaged wall shear stress vanishes. For the fluids considered it is found that 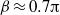$\beta \approx 0. 7\mathrm{\pi} $ and the theoretical predictions based on the Falkner–Skan approximation for this 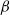$\beta $ leads to better agreement with the DNS results, compared with the Prandtl–Blasius approximation for $\beta = \mathrm{\pi} $.

JFM classification

Boundary Layers: Boundary layer structure Turbulent Flows: Turbulent convection
Type
Papers
Information
Journal of Fluid Mechanics , Volume 730 , 10 September 2013 , pp. 442 - 463
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., Bodenschatz, E., Funfschilling, D., Grossmann, S., He, X., Lohse, D., Stevens, R. J. A. M. & Verzicco, R. 2012 Logarithmic temperature profiles in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 109, 114501.Google Scholar
Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large-scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 82, 503–537.CrossRefGoogle Scholar
Bailon-Cuba, J., Shishkina, O., Wagner, C. & Schumacher, J. 2012 Low-dimensional model of turbulent mixed convection in a complex domain. Phys. Fluids 24, 107101.CrossRefGoogle Scholar
Blasius, H. 1908 Grenzschichten in Flüssigkeiten mit kleiner Reibung. Z. Math. Phys. 56, 1–37.Google Scholar
Brown, E. & Ahlers, G. 2006 Rotations and cessations of the large-scale circulation in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 568, 351–386.Google Scholar
Calzavarini, E., Doering, C. R., Gibbon, J. D., Lohse, D., Tanabe, A. & Toschi, F. 2006 Exponentially growing solutions in homogeneous Rayleigh–Bénard convection. Phys. Rev. E 73, 035301.CrossRefGoogle ScholarPubMed
Chillà, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh–Bénard convection. Eur. Phys. J. E 35, 58.Google Scholar
Ching, E. S. C. 1997 Heat flux and shear rate in turbulent convection. Phys. Rev. E 55, 1189–1192.Google Scholar
Falkner, V. M. & Skan, S. W. 1931 Some approximate solutions of the boundary layer equations. Phyl. Mag. 12, 865–896.CrossRefGoogle Scholar
Funfschilling, D. & Ahlers, G. 2004 Plume motion and large-scale dynamics in a cylindrical Rayleigh–Bénard cell. Phys. Rev. Lett. 92, 194502.Google Scholar
Gauthier, F. & Roche, P.-E. 2008 Evidence of a boundary layer instability at very high Rayleigh number. Europhys. Lett. 83, 24005.CrossRefGoogle Scholar
Grossmann, S. & Lohse, D. 2000 Scaling in thermal convection: a unifying view. J. Fluid Mech. 407, 27–56.Google Scholar
Grossmann, S. & Lohse, D. 2001 Thermal convection for large Prandtl numbers. Phys. Rev. Lett. 86, 3316–3319.Google Scholar
Grossmann, S. & Lohse, D. 2011 Multiple scaling in the ultimate regime of thermal convection. Phys. Fluids 23, 045108.CrossRefGoogle Scholar
Grossmann, S. & Lohse, D. 2012 Logarithmic temperature profiles in the ultimate regime of thermal convection. Phys. Fluids 24, 125103.Google Scholar
Horn, S., Shishkina, O. & Wagner, C. 2011 The influence of non-Oberbeck–Boussinesq effects on rotating turbulent Rayleigh–Bénard convection. J. Phys.: Conf. Ser. 318, 082005.Google Scholar
Horn, S., Shishkina, O. & Wagner, C. 2013 On non-Oberbeck–Boussinesq effects in three-dimensional Rayleigh–Bénard convection in glycerol. J. Fluid Mech. 724, 175–202.Google Scholar
Kaczorowski, M., Shishkina, O., Shishkin, A., Wagner, C. & Xia, K.-Q. 2011 Analysis of the large-scale circulation and the boundary layers in turbulent Rayleigh–Bénard convection. In Direct and Large-Eddy Simulation VIII (ed. Kuerten, H., Geurts, B. & Armenio, V.), pp. 383–388. Springer.Google Scholar
Koerner, M., Shishkina, O., Wagner, C. & Thess, A. 2013 Properties of large-scale flow structures in an isothermal ventilated room. Build. Environ. 59, 563–574.Google Scholar
Lohse, D. & Xia, K.-Q. 2010 Small-scale properties of turbulent Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 42, 335–364.Google Scholar
Pohlhausen, K. 1921 Zur nährungsweisen Integration der Differentialgleichung der laminaren Grenzschicht. Z. Angew. Math. Mech. 1, 252–268.Google Scholar
Prandtl, L. 1905 Über Flüssigkeitsbewegung bei sehr kleiner Reibung. In Verhandlungen des III. Int. Math. Kongr., Heidelberg, 1904, pp. 484–491. Teubner.Google Scholar
du Puits, R., Resagk, C., Tilgner, A., Busse, F. H. & Thess, A. 2007 Structure of thermal boundary layers in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 572, 231–254.CrossRefGoogle Scholar
Scheel, J. D., Kim, E. & White, K. R. 2012 Thermal and viscous boundary layers in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 711, 281–305.Google Scholar
Schlichting, H. & Gersten, K. 2000 Boundary-Layer Theory, 8th edn. Springer.Google Scholar
Schmalzl, J., Breuer, M. & Hansen, U. 2004 On the validity of two-dimensional numerical approaches to time-dependent thermal convection. Europhys. Lett. 67, 390–396.Google Scholar
Shi, N., Emran, M. S. & Schumacher, J. 2012 Boundary layer structure in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 706, 5–33.Google Scholar
Shishkina, O., Stevens, R. J. A. M., Grossmann, S. & Lohse, D. 2010 Boundary layer structure in turbulent thermal convection and its consequences for the required numerical resolution. New J. Phys. 12, 075022.Google Scholar
Shishkina, O. & Thess, A. 2009 Mean temperature profiles in turbulent Rayleigh–Bénard convection of water. J. Fluid Mech. 663, 449–460.Google Scholar
Shishkina, O. & Wagner, C. 2005 A fourth order accurate finite volume scheme for numerical simulations of turbulent Rayleigh–Bénard convection in cylindrical containers. C. R. Mecanique 333, 17–28.Google Scholar
Shishkina, O. & Wagner, C. 2011 Modelling the influence of wall roughness on heat transfer in thermal convection. J. Fluid Mech. 686, 568–582.Google Scholar
Shishkina, O. & Wagner, C. 2012 A numerical study of turbulent mixed convection in an enclosure with heated rectangular elements. J. Turbul. 13, 1–21.Google Scholar
Siggia, E. 1994 High Rayleigh number convection. Annu. Rev. Fluid Mech. 26, 137–168.Google Scholar
Stevens, R. J. A. M., van der Poel, E. P., Grossmann, S. & Lohse, D. 2013 The unifying theory of scaling in thermal convection: the updated prefactors. J. Fluid Mech. (in press).Google Scholar
Stevens, R. J. A. M., Verzicco, R. & Lohse, D. 2010 Radial boundary layer structure and Nusselt number in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 643, 495–507.Google Scholar
Stevens, R. J. A. M., Zhou, Q., Grossmann, S., Verzicco, R., Xia, K.-Q. & Lohse, D. 2012 Thermal boundary layer profiles in turbulent Rayleigh–Bénard convection in a cylindrical sample. Phys. Rev. E 85, 027301.Google Scholar
Stewartson, K. 1958 On the free convection from a horizontal plate. Z. Angew. Math. Phys. 9a, 276–282.Google Scholar
Sun, C., Cheung, Y.-H. & Xia, K.-Q. 2008 Experimental studies of the viscous boundary layer properties in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 605, 79–113.Google Scholar
Tilgner, A., Belmonte, A. & Libchaber, A. 1993 Temperature and velocity profiles of turbulent convection in water. Phys. Rev. E 47, 2253–2257.Google Scholar
Verzicco, R. 2012 Boundary layer structure in confined turbulent thermal convection. J. Fluid Mech. 706, 1–4.Google Scholar
Wagner, S., Shishkina, O. & Wagner, C. 2012 Boundary layers and wind in cylindrical Rayleigh–Bénard cells. J. Fluid Mech. 697, 336–366.CrossRefGoogle Scholar
Weiss, S. & Ahlers, G. 2011 The large-scale flow structure in turbulent rotating Rayleigh–Bénard convection. J. Fluid Mech. 688, 461–492.Google Scholar
Wilcox, D. C. 2010 Basic Fluid Mechanics, 4th edn. DCW Industries.Google Scholar
Xi, H.-D. & Xia, K.-Q. 2007 Cessations and reversals of the large-scale circulation in turbulent thermal convection. Phys. Rev. E 76, 036301.Google Scholar
Xia, K.-Q. 2011 How heat transfer efficiencies in turbulent thermal convection depend on internal flow modes. J. Fluid Mech. 676, 1–4.Google Scholar
Zhou, Q. & Xia, K.-Q. 2010 Measured instantaneous viscous boundary layer in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 104, 104301.Google Scholar