Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Cardin, P.
and
Olson, P.
2007.
Treatise on Geophysics.
p.
319.
Pais, M. A.
and
Jault, D.
2008.
Quasi-geostrophic flows responsible for the secular variation of the Earth's magnetic field.
Geophysical Journal International,
Vol. 173,
Issue. 2,
p.
421.
NET, MARTA
GARCIA, FERRAN
and
SÁNCHEZ, JUAN
2008.
On the onset of low-Prandtl-number convection in rotating spherical shells: non-slip boundary conditions.
Journal of Fluid Mechanics,
Vol. 601,
Issue. ,
p.
317.
Garcia, Ferran
Sánchez, Juan
and
Net, Marta
2008.
Antisymmetric Polar Modes of Thermal Convection in Rotating Spherical Fluid Shells at High Taylor Numbers.
Physical Review Letters,
Vol. 101,
Issue. 19,
PLAUT, E.
LEBRANCHU, Y.
SIMITEV, R.
and
BUSSE, F. H.
2008.
Reynolds stresses and mean fields generated by pure waves: applications to shear flows and convection in a rotating shell.
Journal of Fluid Mechanics,
Vol. 602,
Issue. ,
p.
303.
Fournier, Alexandre
Hulot, Gauthier
Jault, Dominique
Kuang, Weijia
Tangborn, Andrew
Gillet, Nicolas
Canet, Elisabeth
Aubert, Julien
and
Lhuillier, Florian
2010.
An Introduction to Data Assimilation and Predictability in Geomagnetism.
Space Science Reviews,
Vol. 155,
Issue. 1-4,
p.
247.
Sukhanovsky, A.N.
2010.
Formation of differential rotation in a cylindrical fluid layer.
Computational Continuum Mechanics,
Vol. 3,
Issue. 2,
p.
103.
Futterer, B.
Egbers, C.
Dahley, N.
Koch, S.
and
Jehring, L.
2010.
First identification of sub- and supercritical convection patterns from ‘GeoFlow’, the geophysical flow simulation experiment integrated in Fluid Science Laboratory.
Acta Astronautica,
Vol. 66,
Issue. 1-2,
p.
193.
Fournier, Alexandre
Hulot, Gauthier
Jault, Dominique
Kuang, Weijia
Tangborn, Andrew
Gillet, Nicolas
Canet, Elisabeth
Aubert, Julien
and
Lhuillier, Florian
2010.
Terrestrial Magnetism.
Vol. 36,
Issue. ,
p.
247.
Miyagoshi, Takehiro
Kageyama, Akira
and
Sato, Tetsuya
2010.
Zonal flow formation in the Earth’s core.
Nature,
Vol. 463,
Issue. 7282,
p.
793.
Batalov, Vladimir
Sukhanovsky, Andrey
and
Frick, Peter
2010.
Laboratory study of differential rotation in a convective rotating layer.
Geophysical & Astrophysical Fluid Dynamics,
Vol. 104,
Issue. 4,
p.
349.
Olson, Peter
2011.
Laboratory Experiments on the Dynamics of the Core.
Physics of the Earth and Planetary Interiors,
Vol. 187,
Issue. 3-4,
p.
139.
Sukhanovsky, A. N.
2011.
Formation of differential rotation in a cylindrical fluid layer.
Fluid Dynamics,
Vol. 46,
Issue. 1,
p.
158.
Brito, D.
Alboussière, T.
Cardin, P.
Gagnière, N.
Jault, D.
La Rizza, P.
Masson, J.-P.
Nataf, H.-C.
and
Schmitt, D.
2011.
Zonal shear and super-rotation in a magnetized spherical Couette-flow experiment.
Physical Review E,
Vol. 83,
Issue. 6,
Olson, Peter
2011.
Laboratory experiments on the dynamics of the core.
Physics of the Earth and Planetary Interiors,
Vol. 187,
Issue. 1-2,
p.
1.
Teed, Robert J.
Jones, Chris A.
and
Hollerbach, Rainer
2012.
On the necessary conditions for bursts of convection within the rapidly rotating cylindrical annulus.
Physics of Fluids,
Vol. 24,
Issue. 6,
Calkins, Michael A.
Noir, Jérôme
Eldredge, Jeff D.
and
Aurnou, Jonathan M.
2012.
The effects of boundary topography on convection in Earth’s core.
Geophysical Journal International,
Vol. 189,
Issue. 2,
p.
799.
Calkins, Michael A.
Aurnou, Jonathan M.
Eldredge, Jeff D.
and
Julien, Keith
2012.
The influence of fluid properties on the morphology of core turbulence and the geomagnetic field.
Earth and Planetary Science Letters,
Vol. 359-360,
Issue. ,
p.
55.
Liao, Xinhao
Zhang, Keke
and
Li, Ligang
2012.
Asymptotic solutions of differential rotation driven by convection in rapidly rotating fluid spheres with the non-slip boundary condition.
Geophysical & Astrophysical Fluid Dynamics,
Vol. 106,
Issue. 6,
p.
643.
2012.
Rotating Thermal Flows in Natural and Industrial Processes.
p.
473.
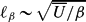 of zonal jets emerges, and the boundary friction becomes the main brake on the growth of the zonal flow. A scaling law U ∼ Ũ4/3 is then derived and verified both numerically and experimentally.
of zonal jets emerges, and the boundary friction becomes the main brake on the growth of the zonal flow. A scaling law U ∼ Ũ4/3 is then derived and verified both numerically and experimentally.

