Article contents
Exact coherent structures in stably stratified plane Couette flow
Published online by Cambridge University Press: 08 August 2017
Abstract
The existence of exact coherent structures in stably stratified plane Couette flow (gravity perpendicular to the plates) is investigated over Reynolds–Richardson number (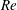 $Re$–
$Re$– $Ri_{b}$) space for a fluid of unit Prandtl number
$Ri_{b}$) space for a fluid of unit Prandtl number 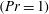 $(Pr=1)$ using a combination of numerical and asymptotic techniques. Two states are repeatedly discovered using edge tracking – EQ7 and EQ7-1 in the nomenclature of Gibson & Brand (J. Fluid Mech., vol. 745, 2014, pp. 25–61) – and found to connect with two-dimensional convective roll solutions when tracked to negative
$(Pr=1)$ using a combination of numerical and asymptotic techniques. Two states are repeatedly discovered using edge tracking – EQ7 and EQ7-1 in the nomenclature of Gibson & Brand (J. Fluid Mech., vol. 745, 2014, pp. 25–61) – and found to connect with two-dimensional convective roll solutions when tracked to negative 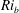 $Ri_{b}$ (the Rayleigh–Bénard problem with shear). Both these states and Nagata’s (J. Fluid Mech., vol. 217, 1990, pp. 519–527) original exact solution feel the presence of stable stratification when
$Ri_{b}$ (the Rayleigh–Bénard problem with shear). Both these states and Nagata’s (J. Fluid Mech., vol. 217, 1990, pp. 519–527) original exact solution feel the presence of stable stratification when  $Ri_{b}=O(Re^{-2})$ or equivalently when the Rayleigh number
$Ri_{b}=O(Re^{-2})$ or equivalently when the Rayleigh number 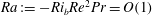 $Ra:=-Ri_{b}Re^{2}Pr=O(1)$. This is confirmed via a stratified extension of the vortex wave interaction theory of Hall & Sherwin (J. Fluid Mech., vol. 661, 2010, pp. 178–205). If the stratification is increased further, EQ7 is found to progressively spanwise and cross-stream localise until a second regime is entered at
$Ra:=-Ri_{b}Re^{2}Pr=O(1)$. This is confirmed via a stratified extension of the vortex wave interaction theory of Hall & Sherwin (J. Fluid Mech., vol. 661, 2010, pp. 178–205). If the stratification is increased further, EQ7 is found to progressively spanwise and cross-stream localise until a second regime is entered at  $Ri_{b}=O(Re^{-2/3})$. This corresponds to a stratified version of the boundary region equations regime of Deguchi, Hall & Walton (J. Fluid Mech., vol. 721, 2013, pp. 58–85). Increasing the stratification further appears to lead to a third, ultimate regime where
$Ri_{b}=O(Re^{-2/3})$. This corresponds to a stratified version of the boundary region equations regime of Deguchi, Hall & Walton (J. Fluid Mech., vol. 721, 2013, pp. 58–85). Increasing the stratification further appears to lead to a third, ultimate regime where 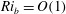 $Ri_{b}=O(1)$ in which the flow fully localises in all three directions at the minimal Kolmogorov scale which then corresponds to the Osmidov scale. Implications for the laminar–turbulent boundary in the (
$Ri_{b}=O(1)$ in which the flow fully localises in all three directions at the minimal Kolmogorov scale which then corresponds to the Osmidov scale. Implications for the laminar–turbulent boundary in the (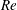 $Re$–
$Re$–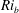 $Ri_{b}$) plane are briefly discussed.
$Ri_{b}$) plane are briefly discussed.
- Type
- Papers
- Information
- Copyright
- © 2017 Cambridge University Press
References
- 10
- Cited by



