1. Introduction
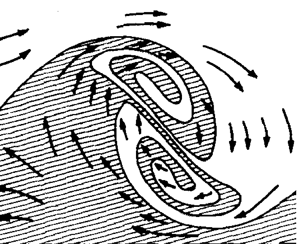
Entrainment occurs mainly by engulfment of ambient fluid by large-scale eddies (figure 1a) and it is ubiquitous in our daily lives. See for example the plumes leaving a chimney stack and getting wider as they rise vertically, the clouds that develop vertically increasing their horizontal size, hot gases from volcanic eruptions which rise into the atmosphere to reach horizontal sizes that are 10–100 times that of the crater (figure 1b). Taylor (Reference Taylor1946) was the first to introduce the entrainment hypothesis during World War II, when investigating the use of oil drum fires to clear the fog from airplane runways. This hypothesis was then revisited by Batchelor (Reference Batchelor1954) and his two PhD students in Cambridge, focusing on theoretical development and laboratory experiments on entrainment. Their results again attracted G. I. Taylor's interest in entrainment and this collaboration flourished into another seminal paper (Morton, Taylor & Turner Reference Morton, Taylor and Turner1956).
Figure 1. (a) Illustration showing the successive stages of the interface and engulfing process, with the fluid on one side shaded. Arrows represent the direction of the flow; not to scale. Image from Corcos & Sherman (Reference Corcos and Sherman1984). (b) Plume of steam, gas and ash at Mount St. Helens on 19 May 1982. Credit, USA Geological Survey (photograph by Lyn Topinka).
By engulfing ambient water, entrainment modifies the density and hence the dynamics of a turbulent flow and a correct prediction of these changes requires an accurate quantification of the entrainment flux. However, the large-scale eddies are too small to be represented in numerical models of geophysical flows. For example, general circulation models (GCM) of the ocean can now resolve dense water overflows ( $\sim$100 m in height), but resolving the eddies (
$\sim$100 m in height), but resolving the eddies ( $\sim$0.1–1 m) at the interface is still prohibitive. A large amount of literature has investigated different ways to accurately parameterize entrainment in dense overflows in GCM. The entrainment hypothesis can be written as
$\sim$0.1–1 m) at the interface is still prohibitive. A large amount of literature has investigated different ways to accurately parameterize entrainment in dense overflows in GCM. The entrainment hypothesis can be written as  $W_e=EU$, where
$W_e=EU$, where  $W_e$ is the entrainment velocity,
$W_e$ is the entrainment velocity,  $U$ is a characteristic velocity scale and
$U$ is a characteristic velocity scale and  $E$ is a constant of proportionality usually named the entrainment coefficient. The value of
$E$ is a constant of proportionality usually named the entrainment coefficient. The value of  $E$ is constant when the boundary of the turbulent flow is primarily parallel to gravity, e.g. plumes. However, when the flow is buoyancy-driven and the boundary is significantly orthogonal to gravity, e.g. gravity currents, to include the buoyancy stabilizing effect the entrainment coefficient depends on the Richardson number
$E$ is constant when the boundary of the turbulent flow is primarily parallel to gravity, e.g. plumes. However, when the flow is buoyancy-driven and the boundary is significantly orthogonal to gravity, e.g. gravity currents, to include the buoyancy stabilizing effect the entrainment coefficient depends on the Richardson number  $E({Ri})$, where
$E({Ri})$, where  ${Ri}=g'h\cos \theta /U^2$ represents the relative importance of buoyancy and inertial forces,
${Ri}=g'h\cos \theta /U^2$ represents the relative importance of buoyancy and inertial forces,  $g'$ is the reduced gravity,
$g'$ is the reduced gravity,  $h$ the flow depth and
$h$ the flow depth and  $\theta$ the bottom slope. Although different expressions of
$\theta$ the bottom slope. Although different expressions of  $E$ for dense water overflows exist (see Cenedese & Adduce (Reference Cenedese and Adduce2010) for a few examples), they all include a dependence on
$E$ for dense water overflows exist (see Cenedese & Adduce (Reference Cenedese and Adduce2010) for a few examples), they all include a dependence on  ${Ri}$, similar to the one based on the experiments of Ellison & Turner (Reference Ellison and Turner1959) and subsequent analysis by Turner (Reference Turner1986):
${Ri}$, similar to the one based on the experiments of Ellison & Turner (Reference Ellison and Turner1959) and subsequent analysis by Turner (Reference Turner1986):
The above parameterization is often used only for  ${Ri}<1/4$, a necessary condition for shear instability (Howard Reference Howard1961; Miles Reference Miles1961), and
${Ri}<1/4$, a necessary condition for shear instability (Howard Reference Howard1961; Miles Reference Miles1961), and  $E=0$ for larger
$E=0$ for larger  ${Ri}$. Although this condition is correct at the scale of the instability, one needs to be careful when using bulk values of
${Ri}$. Although this condition is correct at the scale of the instability, one needs to be careful when using bulk values of  ${Ri}$ averaged over larger scales.
${Ri}$ averaged over larger scales.
2. Overview
In his very comprehensive paper, Turner (Reference Turner1986) applies the entrainment hypothesis to plumes/jets, thermals, gravity currents and density interfaces, both in homogeneous and stratified ambient fluids. He starts by reviewing the similarity solutions for the equations of mass, momentum and buoyancy fluxes for turbulent jets (sources of momentum) and plumes (sources of buoyancy) in a uniform environment, following Fischer et al. (Reference Fischer, List, Koh, Imberger and Brooks1979) and List (Reference List1982). He shows that, for both, the entrainment flux is a function of the local specific momentum flux and that the entrainment coefficient is larger for plumes. The equation  $W_e=EU$ is integral to the similarity solutions which predict a linear increase of the radius with height, and is not an independent assumption (Batchelor Reference Batchelor1954). Given that the main entrainment mechanism is the engulfment of ambient fluid by the large eddies (figure 1), the characteristic velocity should be that of the largest scale of motion, i.e. a mean of the maximum velocity. Then the similarity assumption means that the relationship between the large eddies and the mean flow is unchanged regardless of the scale of motion and that the turbulent energy at smaller scales plays a secondary role.
$W_e=EU$ is integral to the similarity solutions which predict a linear increase of the radius with height, and is not an independent assumption (Batchelor Reference Batchelor1954). Given that the main entrainment mechanism is the engulfment of ambient fluid by the large eddies (figure 1), the characteristic velocity should be that of the largest scale of motion, i.e. a mean of the maximum velocity. Then the similarity assumption means that the relationship between the large eddies and the mean flow is unchanged regardless of the scale of motion and that the turbulent energy at smaller scales plays a secondary role.
These similarity solutions are not applicable when the ambient fluid is stratified, since the plume will come to rest at the level of neutral buoyancy. However, Turner (Reference Turner1986) shows that the applicability of the entrainment hypothesis is more general and, up to a critical height, the plume spreads nearly linearly and the solutions are close to those where the ambient fluid has constant density. The maximum height reached by the plume is  $z_{max}=3.8 B^{{1}/{4}}N^{-{3}/{4}}$, given in terms of buoyancy flux,
$z_{max}=3.8 B^{{1}/{4}}N^{-{3}/{4}}$, given in terms of buoyancy flux,  $B$, and stratification,
$B$, and stratification,  $N$. This expression predicts correctly not only the maximum rise of plumes in the laboratory (Briggs Reference Briggs1969), but also of plumes from erupting volcanoes (Wilson et al. Reference Wilson, Sparks, Huang and Watkins1978), the rise of large convective clouds (Morton Reference Morton1957; Squires & Turner Reference Squires and Turner1962), black-smokers hot water plumes (Campbell, McDougall & Turner Reference Campbell, McDougall and Turner1984) and less dense magma intrusion and in a stratified magma chamber (Campbell, Naldrett & Barnes Reference Campbell, Naldrett and Barnes1983).
$N$. This expression predicts correctly not only the maximum rise of plumes in the laboratory (Briggs Reference Briggs1969), but also of plumes from erupting volcanoes (Wilson et al. Reference Wilson, Sparks, Huang and Watkins1978), the rise of large convective clouds (Morton Reference Morton1957; Squires & Turner Reference Squires and Turner1962), black-smokers hot water plumes (Campbell, McDougall & Turner Reference Campbell, McDougall and Turner1984) and less dense magma intrusion and in a stratified magma chamber (Campbell, Naldrett & Barnes Reference Campbell, Naldrett and Barnes1983).
A gravity current on a sloping bottom or roof can be regarded as a plume with a component of gravity in the downslope direction and one normal to the plume interface, hence buoyancy will hinder the entrainment into the current. The entrainment still depends on the large-scale eddies, but  $E$ is no longer constant and depends on
$E$ is no longer constant and depends on  ${Ri}$ (see (1.1)). The expression of
${Ri}$ (see (1.1)). The expression of  $z_{max}$, the depth at which a gravity current reaches the level of neutral buoyancy in a stratified ambient, is then
$z_{max}$, the depth at which a gravity current reaches the level of neutral buoyancy in a stratified ambient, is then  $z_{max}\propto E^{-{1}/{3}} A^{{1}/{3}}N^{-1}$, where
$z_{max}\propto E^{-{1}/{3}} A^{{1}/{3}}N^{-1}$, where  $A$ is the buoyancy flux per unit width. The entrainment theory for gravity currents has been applied successfully to a wide variety of geophysical and environmental flows: methane roof layers in mines (Ellison & Turner Reference Ellison and Turner1959), spilling breakers on the surface of shoaling water (Longuet-Higgins & Turner Reference Longuet-Higgins and Turner1974), katabatic winds generated by cooling over a slope, powder-snow avalanches (Hopfinger Reference Hopfinger1983; Meiburg, McElwaine & Kneller Reference Meiburg, McElwaine and Kneller2012), turbidity currents (Meiburg et al. Reference Meiburg, McElwaine and Kneller2012), pyroclastic flows, gravity currents driven by dust particles suspended in Mars's atmosphere (Simpson Reference Simpson1982).
$A$ is the buoyancy flux per unit width. The entrainment theory for gravity currents has been applied successfully to a wide variety of geophysical and environmental flows: methane roof layers in mines (Ellison & Turner Reference Ellison and Turner1959), spilling breakers on the surface of shoaling water (Longuet-Higgins & Turner Reference Longuet-Higgins and Turner1974), katabatic winds generated by cooling over a slope, powder-snow avalanches (Hopfinger Reference Hopfinger1983; Meiburg, McElwaine & Kneller Reference Meiburg, McElwaine and Kneller2012), turbidity currents (Meiburg et al. Reference Meiburg, McElwaine and Kneller2012), pyroclastic flows, gravity currents driven by dust particles suspended in Mars's atmosphere (Simpson Reference Simpson1982).
Regions of mixed fluid, where the density is approximately constant, are often encountered near boundaries in an otherwise stratified fluid, e.g. the thermocline in the ocean and the inversion layer in the atmosphere. The transport of mass and heat across these stable layers is then impeded and the mechanism of entrainment at the interface dictates the depth, for example, of the ocean mixed layer. Turner (Reference Turner1986) describes a series of integral models in which the mixing (both mechanical and convective) is assumed to be uniform in the horizontal, and which use modifications of the entrainment hypothesis. For example, the oscillating grid experiments of Turner (Reference Turner1973) show that turbulence may mix a stratified fluid with an entrainment coefficient  $E \propto {Ri_0}^{-{3}/{2}}$, where
$E \propto {Ri_0}^{-{3}/{2}}$, where  $Ri_0$ is estimated at the interface of the mixed region, and the
$Ri_0$ is estimated at the interface of the mixed region, and the  $-{3}/{2}$ power dependence is explained by the eddy recoil mechanism proposed by Linden (Reference Linden1973).
$-{3}/{2}$ power dependence is explained by the eddy recoil mechanism proposed by Linden (Reference Linden1973).
The engulfment entrainment mechanism is no longer the only player when the fluid is stratified and in particular when there is an interface. For strong stratifications,  ${Ri} \gg 1$, the interfacial perturbations become small and the entrainment occurs either via the eddy recoil mechanism (Linden Reference Linden1973) or internal wave breaking. In this scenario, wisps of fluid are injected into the turbulent layer to be mixed by turbulence first and diffusion later. Another classical measure of mixing is the flux Richardson number
${Ri} \gg 1$, the interfacial perturbations become small and the entrainment occurs either via the eddy recoil mechanism (Linden Reference Linden1973) or internal wave breaking. In this scenario, wisps of fluid are injected into the turbulent layer to be mixed by turbulence first and diffusion later. Another classical measure of mixing is the flux Richardson number  ${Ri_f}$, the ratio of the density flux to turbulence production (Turner Reference Turner1973), which increases from zero as
${Ri_f}$, the ratio of the density flux to turbulence production (Turner Reference Turner1973), which increases from zero as  ${Ri}$ increases, presents a maximum value around 0.2, and then decreases for larger values of
${Ri}$ increases, presents a maximum value around 0.2, and then decreases for larger values of  ${Ri}$ (Linden Reference Linden1979, Reference Linden1980). This dependence is general for a wide range of mechanical and convective mixing processes.
${Ri}$ (Linden Reference Linden1979, Reference Linden1980). This dependence is general for a wide range of mechanical and convective mixing processes.
3. Impact
The work presented in Turner (Reference Turner1986) had, and continues to have, a profound impact on many disciplines, from engineering where the entrainment in plumes is fundamental for offshore outfall diffusers, to oceanography where the accurate representation of the density evolution in dense currents is fundamental to representing the global overturning circulation. Current GCM use entrainment parameterizations based on (1.1), and several modifications have been proposed. For example, Cenedese & Adduce (Reference Cenedese and Adduce2010) proposed a modification that allows for entrainment at any  ${Ri}$. Indeed, the entrainment becomes very small when
${Ri}$. Indeed, the entrainment becomes very small when  ${Ri}$ is larger than unity. However, if this small entrainment occurs over a long time, as is the case for some dense currents that travel for several hundreds of kilometres before reaching their neutrally buoyant level or the ocean bottom, it can substantially modify the final properties of these water masses. The dependence of
${Ri}$ is larger than unity. However, if this small entrainment occurs over a long time, as is the case for some dense currents that travel for several hundreds of kilometres before reaching their neutrally buoyant level or the ocean bottom, it can substantially modify the final properties of these water masses. The dependence of  $E$ on the flux Richardson number,
$E$ on the flux Richardson number,  ${Ri_f}$, or the closely related parameter used in the oceanographic community referred to as the flux coefficient
${Ri_f}$, or the closely related parameter used in the oceanographic community referred to as the flux coefficient  $\varGamma$, has been the focus of Wells, Cenedese & Caulfield (Reference Wells, Cenedese and Caulfield2010). Another recent application of the work in Turner (Reference Turner1986) is the use of the similarity solutions to predict the evolution of subglacial discharge plumes that rise along the glacier/ocean interface (Mankoff et al. Reference Mankoff, Straneo, Cenedese, Das, Richards and Singh2016) and the effect the entrainment has on the submarine melt rates (Hewitt Reference Hewitt2020; Jenkins Reference Jenkins2011).
$\varGamma$, has been the focus of Wells, Cenedese & Caulfield (Reference Wells, Cenedese and Caulfield2010). Another recent application of the work in Turner (Reference Turner1986) is the use of the similarity solutions to predict the evolution of subglacial discharge plumes that rise along the glacier/ocean interface (Mankoff et al. Reference Mankoff, Straneo, Cenedese, Das, Richards and Singh2016) and the effect the entrainment has on the submarine melt rates (Hewitt Reference Hewitt2020; Jenkins Reference Jenkins2011).
In summary, the fundamental entrainment dynamics discussed in Turner (Reference Turner1986) constitutes the basis for understanding the role of turbulence in the irreversible transport and mixing of scalars in stratified fluid (Caulfield Reference Caulfield2021), still a grand challenge in geophysical and environmental fluid dynamics (Dauxois et al. Reference Dauxois2021).

1. Introduction
Entrainment occurs mainly by engulfment of ambient fluid by large-scale eddies (figure 1a) and it is ubiquitous in our daily lives. See for example the plumes leaving a chimney stack and getting wider as they rise vertically, the clouds that develop vertically increasing their horizontal size, hot gases from volcanic eruptions which rise into the atmosphere to reach horizontal sizes that are 10–100 times that of the crater (figure 1b). Taylor (Reference Taylor1946) was the first to introduce the entrainment hypothesis during World War II, when investigating the use of oil drum fires to clear the fog from airplane runways. This hypothesis was then revisited by Batchelor (Reference Batchelor1954) and his two PhD students in Cambridge, focusing on theoretical development and laboratory experiments on entrainment. Their results again attracted G. I. Taylor's interest in entrainment and this collaboration flourished into another seminal paper (Morton, Taylor & Turner Reference Morton, Taylor and Turner1956).
Figure 1. (a) Illustration showing the successive stages of the interface and engulfing process, with the fluid on one side shaded. Arrows represent the direction of the flow; not to scale. Image from Corcos & Sherman (Reference Corcos and Sherman1984). (b) Plume of steam, gas and ash at Mount St. Helens on 19 May 1982. Credit, USA Geological Survey (photograph by Lyn Topinka).
By engulfing ambient water, entrainment modifies the density and hence the dynamics of a turbulent flow and a correct prediction of these changes requires an accurate quantification of the entrainment flux. However, the large-scale eddies are too small to be represented in numerical models of geophysical flows. For example, general circulation models (GCM) of the ocean can now resolve dense water overflows ( $\sim$100 m in height), but resolving the eddies (
$\sim$100 m in height), but resolving the eddies ( $\sim$0.1–1 m) at the interface is still prohibitive. A large amount of literature has investigated different ways to accurately parameterize entrainment in dense overflows in GCM. The entrainment hypothesis can be written as
$\sim$0.1–1 m) at the interface is still prohibitive. A large amount of literature has investigated different ways to accurately parameterize entrainment in dense overflows in GCM. The entrainment hypothesis can be written as  $W_e=EU$, where
$W_e=EU$, where  $W_e$ is the entrainment velocity,
$W_e$ is the entrainment velocity,  $U$ is a characteristic velocity scale and
$U$ is a characteristic velocity scale and  $E$ is a constant of proportionality usually named the entrainment coefficient. The value of
$E$ is a constant of proportionality usually named the entrainment coefficient. The value of  $E$ is constant when the boundary of the turbulent flow is primarily parallel to gravity, e.g. plumes. However, when the flow is buoyancy-driven and the boundary is significantly orthogonal to gravity, e.g. gravity currents, to include the buoyancy stabilizing effect the entrainment coefficient depends on the Richardson number
$E$ is constant when the boundary of the turbulent flow is primarily parallel to gravity, e.g. plumes. However, when the flow is buoyancy-driven and the boundary is significantly orthogonal to gravity, e.g. gravity currents, to include the buoyancy stabilizing effect the entrainment coefficient depends on the Richardson number  $E({Ri})$, where
$E({Ri})$, where  ${Ri}=g'h\cos \theta /U^2$ represents the relative importance of buoyancy and inertial forces,
${Ri}=g'h\cos \theta /U^2$ represents the relative importance of buoyancy and inertial forces,  $g'$ is the reduced gravity,
$g'$ is the reduced gravity,  $h$ the flow depth and
$h$ the flow depth and  $\theta$ the bottom slope. Although different expressions of
$\theta$ the bottom slope. Although different expressions of  $E$ for dense water overflows exist (see Cenedese & Adduce (Reference Cenedese and Adduce2010) for a few examples), they all include a dependence on
$E$ for dense water overflows exist (see Cenedese & Adduce (Reference Cenedese and Adduce2010) for a few examples), they all include a dependence on  ${Ri}$, similar to the one based on the experiments of Ellison & Turner (Reference Ellison and Turner1959) and subsequent analysis by Turner (Reference Turner1986):
${Ri}$, similar to the one based on the experiments of Ellison & Turner (Reference Ellison and Turner1959) and subsequent analysis by Turner (Reference Turner1986):
The above parameterization is often used only for ${Ri}<1/4$, a necessary condition for shear instability (Howard Reference Howard1961; Miles Reference Miles1961), and
${Ri}<1/4$, a necessary condition for shear instability (Howard Reference Howard1961; Miles Reference Miles1961), and  $E=0$ for larger
$E=0$ for larger  ${Ri}$. Although this condition is correct at the scale of the instability, one needs to be careful when using bulk values of
${Ri}$. Although this condition is correct at the scale of the instability, one needs to be careful when using bulk values of  ${Ri}$ averaged over larger scales.
${Ri}$ averaged over larger scales.
2. Overview
In his very comprehensive paper, Turner (Reference Turner1986) applies the entrainment hypothesis to plumes/jets, thermals, gravity currents and density interfaces, both in homogeneous and stratified ambient fluids. He starts by reviewing the similarity solutions for the equations of mass, momentum and buoyancy fluxes for turbulent jets (sources of momentum) and plumes (sources of buoyancy) in a uniform environment, following Fischer et al. (Reference Fischer, List, Koh, Imberger and Brooks1979) and List (Reference List1982). He shows that, for both, the entrainment flux is a function of the local specific momentum flux and that the entrainment coefficient is larger for plumes. The equation $W_e=EU$ is integral to the similarity solutions which predict a linear increase of the radius with height, and is not an independent assumption (Batchelor Reference Batchelor1954). Given that the main entrainment mechanism is the engulfment of ambient fluid by the large eddies (figure 1), the characteristic velocity should be that of the largest scale of motion, i.e. a mean of the maximum velocity. Then the similarity assumption means that the relationship between the large eddies and the mean flow is unchanged regardless of the scale of motion and that the turbulent energy at smaller scales plays a secondary role.
$W_e=EU$ is integral to the similarity solutions which predict a linear increase of the radius with height, and is not an independent assumption (Batchelor Reference Batchelor1954). Given that the main entrainment mechanism is the engulfment of ambient fluid by the large eddies (figure 1), the characteristic velocity should be that of the largest scale of motion, i.e. a mean of the maximum velocity. Then the similarity assumption means that the relationship between the large eddies and the mean flow is unchanged regardless of the scale of motion and that the turbulent energy at smaller scales plays a secondary role.
These similarity solutions are not applicable when the ambient fluid is stratified, since the plume will come to rest at the level of neutral buoyancy. However, Turner (Reference Turner1986) shows that the applicability of the entrainment hypothesis is more general and, up to a critical height, the plume spreads nearly linearly and the solutions are close to those where the ambient fluid has constant density. The maximum height reached by the plume is $z_{max}=3.8 B^{{1}/{4}}N^{-{3}/{4}}$, given in terms of buoyancy flux,
$z_{max}=3.8 B^{{1}/{4}}N^{-{3}/{4}}$, given in terms of buoyancy flux,  $B$, and stratification,
$B$, and stratification,  $N$. This expression predicts correctly not only the maximum rise of plumes in the laboratory (Briggs Reference Briggs1969), but also of plumes from erupting volcanoes (Wilson et al. Reference Wilson, Sparks, Huang and Watkins1978), the rise of large convective clouds (Morton Reference Morton1957; Squires & Turner Reference Squires and Turner1962), black-smokers hot water plumes (Campbell, McDougall & Turner Reference Campbell, McDougall and Turner1984) and less dense magma intrusion and in a stratified magma chamber (Campbell, Naldrett & Barnes Reference Campbell, Naldrett and Barnes1983).
$N$. This expression predicts correctly not only the maximum rise of plumes in the laboratory (Briggs Reference Briggs1969), but also of plumes from erupting volcanoes (Wilson et al. Reference Wilson, Sparks, Huang and Watkins1978), the rise of large convective clouds (Morton Reference Morton1957; Squires & Turner Reference Squires and Turner1962), black-smokers hot water plumes (Campbell, McDougall & Turner Reference Campbell, McDougall and Turner1984) and less dense magma intrusion and in a stratified magma chamber (Campbell, Naldrett & Barnes Reference Campbell, Naldrett and Barnes1983).
A gravity current on a sloping bottom or roof can be regarded as a plume with a component of gravity in the downslope direction and one normal to the plume interface, hence buoyancy will hinder the entrainment into the current. The entrainment still depends on the large-scale eddies, but $E$ is no longer constant and depends on
$E$ is no longer constant and depends on  ${Ri}$ (see (1.1)). The expression of
${Ri}$ (see (1.1)). The expression of  $z_{max}$, the depth at which a gravity current reaches the level of neutral buoyancy in a stratified ambient, is then
$z_{max}$, the depth at which a gravity current reaches the level of neutral buoyancy in a stratified ambient, is then  $z_{max}\propto E^{-{1}/{3}} A^{{1}/{3}}N^{-1}$, where
$z_{max}\propto E^{-{1}/{3}} A^{{1}/{3}}N^{-1}$, where  $A$ is the buoyancy flux per unit width. The entrainment theory for gravity currents has been applied successfully to a wide variety of geophysical and environmental flows: methane roof layers in mines (Ellison & Turner Reference Ellison and Turner1959), spilling breakers on the surface of shoaling water (Longuet-Higgins & Turner Reference Longuet-Higgins and Turner1974), katabatic winds generated by cooling over a slope, powder-snow avalanches (Hopfinger Reference Hopfinger1983; Meiburg, McElwaine & Kneller Reference Meiburg, McElwaine and Kneller2012), turbidity currents (Meiburg et al. Reference Meiburg, McElwaine and Kneller2012), pyroclastic flows, gravity currents driven by dust particles suspended in Mars's atmosphere (Simpson Reference Simpson1982).
$A$ is the buoyancy flux per unit width. The entrainment theory for gravity currents has been applied successfully to a wide variety of geophysical and environmental flows: methane roof layers in mines (Ellison & Turner Reference Ellison and Turner1959), spilling breakers on the surface of shoaling water (Longuet-Higgins & Turner Reference Longuet-Higgins and Turner1974), katabatic winds generated by cooling over a slope, powder-snow avalanches (Hopfinger Reference Hopfinger1983; Meiburg, McElwaine & Kneller Reference Meiburg, McElwaine and Kneller2012), turbidity currents (Meiburg et al. Reference Meiburg, McElwaine and Kneller2012), pyroclastic flows, gravity currents driven by dust particles suspended in Mars's atmosphere (Simpson Reference Simpson1982).
Regions of mixed fluid, where the density is approximately constant, are often encountered near boundaries in an otherwise stratified fluid, e.g. the thermocline in the ocean and the inversion layer in the atmosphere. The transport of mass and heat across these stable layers is then impeded and the mechanism of entrainment at the interface dictates the depth, for example, of the ocean mixed layer. Turner (Reference Turner1986) describes a series of integral models in which the mixing (both mechanical and convective) is assumed to be uniform in the horizontal, and which use modifications of the entrainment hypothesis. For example, the oscillating grid experiments of Turner (Reference Turner1973) show that turbulence may mix a stratified fluid with an entrainment coefficient $E \propto {Ri_0}^{-{3}/{2}}$, where
$E \propto {Ri_0}^{-{3}/{2}}$, where  $Ri_0$ is estimated at the interface of the mixed region, and the
$Ri_0$ is estimated at the interface of the mixed region, and the  $-{3}/{2}$ power dependence is explained by the eddy recoil mechanism proposed by Linden (Reference Linden1973).
$-{3}/{2}$ power dependence is explained by the eddy recoil mechanism proposed by Linden (Reference Linden1973).
The engulfment entrainment mechanism is no longer the only player when the fluid is stratified and in particular when there is an interface. For strong stratifications, ${Ri} \gg 1$, the interfacial perturbations become small and the entrainment occurs either via the eddy recoil mechanism (Linden Reference Linden1973) or internal wave breaking. In this scenario, wisps of fluid are injected into the turbulent layer to be mixed by turbulence first and diffusion later. Another classical measure of mixing is the flux Richardson number
${Ri} \gg 1$, the interfacial perturbations become small and the entrainment occurs either via the eddy recoil mechanism (Linden Reference Linden1973) or internal wave breaking. In this scenario, wisps of fluid are injected into the turbulent layer to be mixed by turbulence first and diffusion later. Another classical measure of mixing is the flux Richardson number  ${Ri_f}$, the ratio of the density flux to turbulence production (Turner Reference Turner1973), which increases from zero as
${Ri_f}$, the ratio of the density flux to turbulence production (Turner Reference Turner1973), which increases from zero as  ${Ri}$ increases, presents a maximum value around 0.2, and then decreases for larger values of
${Ri}$ increases, presents a maximum value around 0.2, and then decreases for larger values of  ${Ri}$ (Linden Reference Linden1979, Reference Linden1980). This dependence is general for a wide range of mechanical and convective mixing processes.
${Ri}$ (Linden Reference Linden1979, Reference Linden1980). This dependence is general for a wide range of mechanical and convective mixing processes.
3. Impact
The work presented in Turner (Reference Turner1986) had, and continues to have, a profound impact on many disciplines, from engineering where the entrainment in plumes is fundamental for offshore outfall diffusers, to oceanography where the accurate representation of the density evolution in dense currents is fundamental to representing the global overturning circulation. Current GCM use entrainment parameterizations based on (1.1), and several modifications have been proposed. For example, Cenedese & Adduce (Reference Cenedese and Adduce2010) proposed a modification that allows for entrainment at any ${Ri}$. Indeed, the entrainment becomes very small when
${Ri}$. Indeed, the entrainment becomes very small when  ${Ri}$ is larger than unity. However, if this small entrainment occurs over a long time, as is the case for some dense currents that travel for several hundreds of kilometres before reaching their neutrally buoyant level or the ocean bottom, it can substantially modify the final properties of these water masses. The dependence of
${Ri}$ is larger than unity. However, if this small entrainment occurs over a long time, as is the case for some dense currents that travel for several hundreds of kilometres before reaching their neutrally buoyant level or the ocean bottom, it can substantially modify the final properties of these water masses. The dependence of  $E$ on the flux Richardson number,
$E$ on the flux Richardson number,  ${Ri_f}$, or the closely related parameter used in the oceanographic community referred to as the flux coefficient
${Ri_f}$, or the closely related parameter used in the oceanographic community referred to as the flux coefficient  $\varGamma$, has been the focus of Wells, Cenedese & Caulfield (Reference Wells, Cenedese and Caulfield2010). Another recent application of the work in Turner (Reference Turner1986) is the use of the similarity solutions to predict the evolution of subglacial discharge plumes that rise along the glacier/ocean interface (Mankoff et al. Reference Mankoff, Straneo, Cenedese, Das, Richards and Singh2016) and the effect the entrainment has on the submarine melt rates (Hewitt Reference Hewitt2020; Jenkins Reference Jenkins2011).
$\varGamma$, has been the focus of Wells, Cenedese & Caulfield (Reference Wells, Cenedese and Caulfield2010). Another recent application of the work in Turner (Reference Turner1986) is the use of the similarity solutions to predict the evolution of subglacial discharge plumes that rise along the glacier/ocean interface (Mankoff et al. Reference Mankoff, Straneo, Cenedese, Das, Richards and Singh2016) and the effect the entrainment has on the submarine melt rates (Hewitt Reference Hewitt2020; Jenkins Reference Jenkins2011).
In summary, the fundamental entrainment dynamics discussed in Turner (Reference Turner1986) constitutes the basis for understanding the role of turbulence in the irreversible transport and mixing of scalars in stratified fluid (Caulfield Reference Caulfield2021), still a grand challenge in geophysical and environmental fluid dynamics (Dauxois et al. Reference Dauxois2021).
Acknowledgements
C.C. thanks the editor J. Neufeld for feedback.
Declaration of interests
The author reports no conflict of interest.