Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
FitzMaurice, A.
Cenedese, C.
and
Straneo, F.
2017.
Nonlinear response of iceberg side melting to ocean currents.
Geophysical Research Letters,
Vol. 44,
Issue. 11,
p.
5637.
McConnochie, C. D.
and
Kerr, R. C.
2017.
Testing a common ice‐ocean parameterization with laboratory experiments.
Journal of Geophysical Research: Oceans,
Vol. 122,
Issue. 7,
p.
5905.
Ezhova, Ekaterina
Cenedese, Claudia
and
Brandt, Luca
2017.
Dynamics of a Turbulent Buoyant Plume in a Stratified Fluid: An Idealized Model of Subglacial Discharge in Greenland Fjords.
Journal of Physical Oceanography,
Vol. 47,
Issue. 10,
p.
2611.
McConnochie, Craig D.
and
Kerr, Ross C.
2018.
Dissolution of a sloping solid surface by turbulent compositional convection.
Journal of Fluid Mechanics,
Vol. 846,
Issue. ,
p.
563.
Mondal, Mainak
Gayen, Bishakhdatta
Griffiths, Ross W.
and
Kerr, Ross C.
2019.
Ablation of sloping ice faces into polar seawater.
Journal of Fluid Mechanics,
Vol. 863,
Issue. ,
p.
545.
Hewitt, Ian J.
2020.
Subglacial Plumes.
Annual Review of Fluid Mechanics,
Vol. 52,
Issue. 1,
p.
145.
Wilson, Earle A.
Wells, Andrew J.
Hewitt, Ian J.
and
Cenedese, Claudia
2020.
The dynamics of a subglacial salt wedge.
Journal of Fluid Mechanics,
Vol. 895,
Issue. ,
McConnochie, Craig D.
Cenedese, Claudia
and
McElwaine, Jim N.
2020.
Surface Expression of a Wall Fountain: Application to Subglacial Discharge Plumes.
Journal of Physical Oceanography,
Vol. 50,
Issue. 5,
p.
1245.
Rosevear, Madelaine Gamble
Gayen, Bishakhdatta
and
Galton-Fenzi, Benjamin Keith
2021.
The role of double-diffusive convection in basal melting of Antarctic ice shelves.
Proceedings of the National Academy of Sciences,
Vol. 118,
Issue. 6,
Rosevear, Madelaine
Galton-Fenzi, Benjamin
and
Stevens, Craig
2022.
Evaluation of basal melting parameterisations using in situ ocean and melting observations from the Amery Ice Shelf, East Antarctica.
Ocean Science,
Vol. 18,
Issue. 4,
p.
1109.
Wells, Andrew J.
2023.
From classical to ultimate heat fluxes for convection at a vertical wall.
Journal of Fluid Mechanics,
Vol. 970,
Issue. ,
Wengrove, Meagan E.
Pettit, Erin C.
Nash, Jonathan D.
Jackson, Rebecca H.
and
Skyllingstad, Eric D.
2023.
Melting of glacier ice enhanced by bursting air bubbles.
Nature Geoscience,
Vol. 16,
Issue. 10,
p.
871.
McConnochie, C. D.
and
Cenedese, C.
2023.
Increased Melting of Marine‐Terminating Glaciers by Sediment‐Laden Plumes.
Geophysical Research Letters,
Vol. 50,
Issue. 20,
Bennetts, Luke G.
Shakespeare, Callum J.
Vreugdenhil, Catherine A.
Foppert, Annie
Gayen, Bishakhdatta
Meyer, Amelie
Morrison, Adele K.
Padman, Laurie
Phillips, Helen E.
Stevens, Craig L.
Toffoli, Alessandro
Constantinou, Navid C.
Cusack, Jesse M.
Cyriac, Ajitha
Doddridge, Edward W.
England, Matthew H.
Evans, D. Gwyn
Heil, Petra
Hogg, Andrew McC.
Holmes, Ryan M.
Huneke, Wilma G. C.
Jones, Nicole L.
Keating, Shane R.
Kiss, Andrew E.
Kraitzman, Noa
Malyarenko, Alena
McConnochie, Craig D.
Meucci, Alberto
Montiel, Fabien
Neme, Julia
Nikurashin, Maxim
Patel, Ramkrushnbhai S.
Peng, Jen‐Ping
Rayson, Matthew
Rosevear, Madelaine G.
Sohail, Taimoor
Spence, Paul
and
Stanley, Geoffrey J.
2024.
Closing the Loops on Southern Ocean Dynamics: From the Circumpolar Current to Ice Shelves and From Bottom Mixing to Surface Waves.
Reviews of Geophysics,
Vol. 62,
Issue. 3,
McCutchan, Aubrey L.
Meyer, Colin R.
and
Johnson, Blair A.
2024.
Enhancement of ice melting in isotropic turbulence.
Physical Review Fluids,
Vol. 9,
Issue. 7,
Zhao, Ken X.
Skyllingstad, Eric D.
and
Nash, Jonathan D.
2024.
Improved Parameterizations of Vertical Ice‐Ocean Boundary Layers and Melt Rates.
Geophysical Research Letters,
Vol. 51,
Issue. 4,
Rosevear, Madelaine G.
Gayen, Bishakhdatta
Vreugdenhil, Catherine A.
and
Galton-Fenzi, Benjamin K.
2025.
How Does the Ocean Melt Antarctic Ice Shelves?.
Annual Review of Marine Science
,
Vol. 17,
Issue. 1,
p.
325.
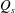 $Q_{s}$, is varied between experiments to determine where the resultant wall plume transitions from being dominated by the distributed buoyancy flux due to dissolution of the ice, to being dominated by the initial buoyancy flux,
$Q_{s}$, is varied between experiments to determine where the resultant wall plume transitions from being dominated by the distributed buoyancy flux due to dissolution of the ice, to being dominated by the initial buoyancy flux, 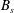 $B_{s}$. We find that when
$B_{s}$. We find that when 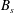 $B_{s}$ is significantly larger than the distributed buoyancy flux from dissolution, the plume velocity is uniform with height and is proportional to
$B_{s}$ is significantly larger than the distributed buoyancy flux from dissolution, the plume velocity is uniform with height and is proportional to 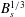 $B_{s}^{1/3}$, the interface temperature is independent of
$B_{s}^{1/3}$, the interface temperature is independent of 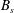 $B_{s}$, and the ablation velocity increases with
$B_{s}$, and the ablation velocity increases with 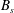 $B_{s}$.
$B_{s}$.


