Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-25T23:46:40.413Z Has data issue: false hasContentIssue false
  • English
  • Français

Electric field mediated von Kármán vortices in stratified microflows: transition from linear instabilities to coherent mixing

Published online by Cambridge University Press:  18 February 2019

Satarupa Dutta Open the ORCID record for Satarupa Dutta [Opens in a new window] ,
Abir Ghosh ,
Partho Sarathi Gooh Pattader  and
Dipankar Bandyopadhyay Open the ORCID record for Dipankar Bandyopadhyay [Opens in a new window]
Satarupa Dutta
Affiliation:
Department of Chemical Engineering, Indian Institute of Technology Guwahati, Assam 781039, India
Abir Ghosh
Affiliation:
Centre for Nanotechnology, Indian Institute of Technology Guwahati, Assam 781039, India
Partho Sarathi Gooh Pattader
Affiliation:
Department of Chemical Engineering, Indian Institute of Technology Guwahati, Assam 781039, India Centre for Nanotechnology, Indian Institute of Technology Guwahati, Assam 781039, India
Dipankar Bandyopadhyay*
Affiliation:
Department of Chemical Engineering, Indian Institute of Technology Guwahati, Assam 781039, India Centre for Nanotechnology, Indian Institute of Technology Guwahati, Assam 781039, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Application of an electric field across the pressure-driven stratified flow of a pair of miscible fluids inside a microchannel manifests interesting electrohydrodynamic (EHD) instabilities. Experiments uncover distinctive instability regimes with an increase in electric field Rayleigh number (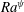$Ra^{\unicode[STIX]{x1D713}}$) – a linear-onset regime, a time-periodic nonlinear regime analogous to the von Kármán vortex street in the downstream and a regime with coherent flow patterns. The experiments also reveal that such linear and nonlinear instabilities can be stimulated non-invasively in a microchannel to mix or de-mix fluids simply by turning the electric field on or off, indicating the suitability of the process for on-demand micromixing. The characteristics of these instabilities have been theoretically investigated with the help of an Orr–Sommerfeld framework, which discloses the possibility of five distinctive finite-wavenumber modes for the instability. The EHD stresses originating due to the application of electric field stimulate a pair of shorter-wavelength electric field modes beyond a critical value of $Ra^{\unicode[STIX]{x1D713}}$. Increase in the levels of charge injection and EHD stresses lower the critical $Ra^{\unicode[STIX]{x1D713}}$ of these modes. The relatively longer-wavelength viscous mode is found to appear when the viscosity stratification between the fluid layers is high. Beyond a threshold Schmidt number ($Sc$), a diffusive mode is also found to appear near the mixed interfacial region. A thinner interface between the fluids at a higher $Sc$ helps this mode to behave as the interfacial mode of immiscible fluids. Contrast of ionic mobility in the fluids leads to the appearance of the K-mode of instability at much shorter wavelengths. The reported phenomena can be of significance in the domains of microscale mixing, pumping, heat exchange, mass transfer and reaction engineering.

JFM classification

Instability: Absolute/convective instability Mixing: Chaotic advection Micro-/Nano-fluid dynamics: Microfluidics
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 865 , 25 April 2019 , pp. 169 - 211
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alj, A., Denat, A., Gosse, J. P., Gosse, B. & Nakamura, I. 1985 Creation of charge carriers in nonpolar liquids. IEEE Trans. Electr. Insul. EI‐20 (2), 221–231.Google Scholar
Allen, P. H. G. & Karayiannis, T. G. 1995 Electrohydrodynamic enhancement of heat transfer and fluid flow. Heat Recov. Syst. CHP 15 (5), 389–423.10.1016/0890-4332(95)90050-0Google Scholar
Atten, P. 1974 Electrohydrodynamic stability of dielectric liquids during transient regime of space-charge-limited injection. Phys. Fluids 17 (10), 1822–1827.10.1063/1.1694623Google Scholar
Atten, P. & Gosse, J. P. 1969 Transient of one-carrier injections in polar liquids. J. Chem. Phys. 51 (7), 2804–2811.Google Scholar
Atten, P. & Haidara, M. 1985 Electrical conduction and EHD motion of dielectric liquids in a knife-plane electrode assembly. IEEE Trans. Electr. Insul. E1‐20 (2), 187–198.Google Scholar
Atten, P. & Lacroix, J. C. 1979 Non-linear hydrodynamic stability of liquids subjected to unipolar injection. J. Méc. 18, 469–510.Google Scholar
Atten, P. & Moreau, R. 1972 Stabilité électrohydrodynamique des liquides isolants soumis à une injection unipolaire. J. Méc. 11 (3), 471–521.Google Scholar
Bart, S. F., Tavrow, L. S., Mehregany, M. & Lang, J. H. 1990 Microfabricated electrohydrodynamic pumps. Sensor Actuators A 21 (1), 193–197.Google Scholar
Bertsch, A., Heimgartner, S., Cousseau, P. & Renaud, P. 2001 Static micromixers based on large-scale industrial mixer geometry. Lab on a Chip 1 (1), 56–60.Google Scholar
Bobyl, V. G., Romanets, R. G. & Alyab’ev, V. A. 1965 The electrical conductivity of benzene and its monohalide derivatives in an ultrasonic field. Sov. Phys. J. 8, 30–34.10.1007/BF00818399Google Scholar
Bromberg, A. & Mathies, R. A. 2003 Homogeneous immunoassay for detection of TNT and its analogues on a microfabricated capillary electrophoresis chip. Anal. Chem. 75 (5), 1188–1195.Google Scholar
Castellanos, A 1991 Coulomb-driven convection in electrohydrodynamics. IEEE Trans. Electr. Insul. 26 (6), 1201–1215.Google Scholar
Castellanos, A. & Agrait, N. 1992 Unipolar injection induced instabilities in plane parallel flows. IEEE Trans Ind. Applics. 28 (3), 513–519.Google Scholar
Chakraborty, S., Liao, I.-C., Adler, A. & Leong, K. W. 2009 Electrohydrodynamics: a facile technique to fabricate drug delivery systems. Adv. Drug Deliv. Rev. 61 (12), 1043–1054.Google Scholar
Chen, C.-H., Lin, H., Lele, S. K. & Santiago, J. G. 2005 Convective and absolute electrokinetic instability with conductivity gradients. J. Fluid Mech. 524, 263–303.10.1017/S0022112004002381Google Scholar
Chen, C.-Y. & Meiburg, E. 1996 Miscible displacements in capillary tubes. Part 2. Numerical simulations. J. Fluid Mech. 326, 57–90.Google Scholar
Christodoulides, N., Tran, M., Floriano, P. N., Rodriguez, M., Goodey, A., Ali, M., Neikirk, D. & McDevitt, J. T. 2002 A microchip-based multianalyte assay system for the assessment of cardiac risk. Anal. Chem. 74 (13), 3030–3036.10.1021/ac011150aGoogle Scholar
Craik, A. D. D. 1969 The stability of plane Couette flow with viscosity stratification. J. Fluid Mech. 36 (4), 685–693.10.1017/S0022112069001935Google Scholar
Denat, A., Gosse, B. & Gosse, J. P. 1979 Ion injections in hydrocarbons. J. Electrostat. 7, 205–225.10.1016/0304-3886(79)90073-1Google Scholar
Ding, Z. & Wong, T. N. 2015 Electrohydrodynamic instability of miscible coreannular flows with electrical conductivity stratification. J. Fluid Mech. 764, 488–512.10.1017/jfm.2014.720Google Scholar
Doyle, P. S., Bibette, J., Bancaud, A. & Viovy, J.-L. 2002 Self-assembled magnetic matrices for dna separation chips. Science 295 (5563), 2237.Google Scholar
El Moctar, A. O., Aubry, N. & Batton, J. 2003 Electro-hydrodynamic micro-fluidic mixer. Lab on a Chip 3 (4), 273–280.10.1039/b306868bGoogle Scholar
Ern, P., Charru, F. & Luchini, P. 2003 Stability analysis of a shear flow with strongly stratified viscosity. J. Fluid Mech. 496, 295–312.10.1017/S0022112003006372Google Scholar
Ghosh, S. & Usha, R. 2016 Stability of viscosity stratified flows down an incline: role of miscibility and wall slip. Phys. Fluids 28, 104101.Google Scholar
Govindarajan, R. 2004 Effect of miscibility on the linear instability of two-fluid channel flow. Intl J. Multiphase Flow 30 (10), 1177–1192.Google Scholar
Haeberle, S. & Zengerle, R. 2007 Microfluidic platforms for lab-on-a-chip applications. Lab on a Chip 7, 1094–1110.Google Scholar
Harnett, C. K, Templeton, J., Dunphy-Guzman, K. A., Senousy, Y. M. & Kanouff, M. P. 2008 Model based design of a microfluidic mixer driven by induced charge electroosmosis. Lab on a Chip 8 (4), 565–572.10.1039/b717416kGoogle Scholar
Hertzog, D. E., Ivorra, B., Mohammadi, B., Bakajin, O. & Santiago, J. G. 2006 Optimization of a microfluidic mixer for studying protein folding kinetics. Anal. Chem. 78 (13), 4299–4306.10.1021/ac051903jGoogle Scholar
Higuera, F. J. 2002 Electrohydrodynamic flow of a dielectric liquid due to autonomous injection of charge by a needle electrode. Phys. Fluids 14 (1), 423–426.Google Scholar
Hinsmann, P., Frank, J., Svasek, P., Harasek, M. & Lendl, B. 2001 Design, simulation and application of a new micromixing device for time resolved infrared spectroscopy of chemical reactions in solution. Lab on a Chip 1 (1), 16–21.Google Scholar
Hopfinger, E. J. & Gosse, J. P. 1971 Charge transport by selfgenerated turbulence in insulating liquids submitted to unipolar injection. Phys. Fluids 14 (8), 1671–1682.10.1063/1.1693663Google Scholar
Janasek, D., Franzke, J. & Manz, A. 2006 Scaling and the design of miniaturized chemical-analysis systems. Nature 442 (7101), 374.Google Scholar
Jha, B., Cueto-Felgueroso, L. & Juanes, R. 2011 Fluid mixing from viscous fingering. Phys. Rev. Lett. 106 (19), 194502.Google Scholar
Ko, S. H., Lee, H. & Kang, K. H. 2008 Hydrodynamic flows in electrowetting. Langmuir 24 (3), 1094–1101.Google Scholar
Lacroix, J. C., Atten, P. & Hopfinger, E. J. 1975 Electro-convection in a dielectric liquid layer subjected to unipolar injection. J. Fluid Mech. 69 (3), 539–563.10.1017/S0022112075001553Google Scholar
Lajeunesse, E., Martin, J., Rakotomalala, N., Salin, D. & Yortsos, Y. C. 1999 Miscible displacement in a Hele-Shaw cell at high rates. J. Fluid Mech. 398, 299–319.Google Scholar
Lara, J. L., Castellanos, A. & Pontiga, F. 1997 Destabilization of plane Poiseuille flow of insulating liquids by unipolar charge injection. Phys. Fluids 9 (2), 399–406.Google Scholar
van der Maesen, F. 1949 The absolute dielectric constant of benzene. Physica 15 (5–6), 481–483.Google Scholar
Malraison, B. & Atten, P. 1982 Chaotic behavior of instability due to unipolar ion injection in a dielectric liquid. Phys. Rev. Lett. 49 (10), 723–726.10.1103/PhysRevLett.49.723Google Scholar
Mark, D., Haeberle, S., Roth, G., von Stetten, F. & Zengerle, R. 2010 Microfluidic lab-on-a-chip platforms: requirements, characteristics and applications. Chem. Soc. Rev. 39, 1153–1182.Google Scholar
McCluskey, F. M. J., Atten, P. & Perez, A. T. 1991 Heat transfer enhancement by electroconvection resulting from an injected space charge between parallel plates. Intl J. Heat Mass Transfer 34 (9), 2237–2250.Google Scholar
McCluskey, F. M. J. & Atten, P. 1988 Modifications to the wake of a wire across Poiseuille flow due to a unipolar space charge. J. Fluid Mech. 197, 81–104.Google Scholar
Oddy, M. H., Santiago, J. G. & Mikkelsen, J. C. 2001 Electrokinetic instability micromixing. Anal. Chem. 73 (24), 5822–5832.Google Scholar
d’Olce, M., Martin, J., Rakotomalala, N., Salin, D. & Talon, L. 2009 Convective/absolute instability in miscible core-annular flow. Part 1. Experiments. J. Fluid Mech. 618, 305–322.10.1017/S0022112008004230Google Scholar
Oliveri, S., Atten, P. & Castellanos, A. 1987 The stability of a planar layer of insulating liquid subjected to charge injection and rotation. Phys. Fluids 30 (7), 1948–1955.10.1063/1.866209Google Scholar
Orszag, S. A. 1971 Accurate solution of the Orr-Sommerfeld stability equation. J. Fluid Mech. 50 (4), 689–703.Google Scholar
Otsubo, Y. & Edamura, K. 1998 Viscoelasticity of a dielectric fluid in nonuniform electric fields generated by electrodes with flocked fabrics. Rheol. Acta 37 (5), 500–507.10.1007/s003970050136Google Scholar
Petitjeans, P. & Maxworthy, T. 1996 Miscible displacements in capillary tubes. Part 1. Experiments. J. Fluid Mech. 326, 37–56.Google Scholar
Pontiga, F., Castellanos, A. & Malraison, B. 1995 Some considerations on the instabilities of nonpolar liquids subjected to charge injection. Phys. Fluids 7 (6), 1348–1356.Google Scholar
Posner, J. D., Pérez, C. L. & Santiago, J. G. 2012 Electric fields yield chaos in microflows. Proc. Natl Acad. Sci. USA 109 (36), 14353–14356.Google Scholar
Posner, J. D. & Santiago, J. G. 2006 Convective instability of electrokinetic flows in a cross-shaped microchannel. J. Fluid Mech. 555, 1–42.Google Scholar
Preziosi, L., Chen, K. & Joseph, D. D. 1989 Lubricated pipelining: stability of core-annular flow. J. Fluid Mech. 201, 323–356.Google Scholar
Ranganathan, B. T. & Govindarajan, R. 2001 Stabilization and destabilization of channel flow by location of viscosity-stratified fluid layer. Phys. Fluids 13 (1), 1–3.10.1063/1.1329651Google Scholar
Ren, Q. Y., Wang, L. F. & Huang, Q. A. 2016 A new method for real-time measuring the temperature-dependent dielectric constant of the silicone oil. IEEE Sens. J. 16 (24), 8792–8797.10.1109/JSEN.2016.2580158Google Scholar
Rhee, S. W., Taylor, A. M., Tu, C. H., Cribbs, D. H., Cotman, C. W. & Jeon, N. L. 2005 Patterned cell culture inside microfluidic devices. Lab on a Chip 5, 102–107.Google Scholar
Rife, J. C., Bell, M. I., Horwitz, J. S., Kabler, M. N., Auyeung, R. C. Y. & Kim, W. J. 2000 Miniature valveless ultrasonic pumps and mixers. Sens. Actuat. A: Phys. 86 (1), 135–140.Google Scholar
Sahu, K. C. & Govindarajan, R. 2016 Linear stability analysis and direct numerical simulation of two-layer channel flow. J. Fluid Mech. 798, 889–909.10.1017/jfm.2016.346Google Scholar
Samiei, E., Tabrizian, M. & Hoorfar, M. 2016 A review of digital microfluidics as portable platforms for lab-on a-chip applications. Lab on a Chip 16, 2376–2396.10.1039/C6LC00387GGoogle Scholar
Schmid, P. J. & Henningson, D. S. 2001 Stability and Transition in Shear Flows. Springer.Google Scholar
Schmidt, W. F. & Yoshino, K. 2015 Ion mobilities in non-polar dielectric liquids: silicone oils. IEEE Trans. Dielec. Elec. Insul. 22 (5), 2424–2427.10.1109/TDEI.2015.005036Google Scholar
Schneider, J. M. & Watson, P. K. 1970 Electrohydrodynamic stability of space-charge-limited currents in dielectric liquids. I. Theoretical study. Phys. Fluids 13 (8), 1948–1954.Google Scholar
Scoffoni, J., Lajeunesse, E. & Homsy, G. M. 2001 Interface instabilities during displacements of two miscible fluids in a vertical pipe. Phys. Fluids 13 (3), 553–556.Google Scholar
Selvam, B., Merk, S., Govindarajan, R. & Meiburg, E. 2007 Stability of miscible core–annular flows with viscosity stratification. J. Fluid Mech. 592, 23–49.Google Scholar
Selvam, B., Talon, L., Lesshafft, L. & Meiburg, E. 2009 Convective/absolute instability in miscible core-annular flow. Part 2. Numerical simulations and nonlinear global modes. J. Fluid Mech. 618, 323–348.Google Scholar
Simonnet, C. & Groisman, A. 2005 Chaotic mixing in a steady flow in a microchannel. Phys. Rev. Lett. 94 (13), 134501.Google Scholar
Skotak, M. & Larsen, G. 2006 Solution chemistry control to make well defined submicron continuous fibres by electrospinning: the (CH3 CH2 CH2 O)4 Ti/AcOH/poly(N-vinylpyrrolidone) system. J. Mater. Chem. 16, 3031–3039.10.1039/b601960aGoogle Scholar
de Sousa, F. F., Moreira, S. G. C., Shirsley, J., Nero, J. D. & Alcantara, P. Jr. 2009 Dielectric properties of oleic acid in liquid phase. J. Bionanoscience 3 (2), 139–142.Google Scholar
Spohner, M. 2016 Study of the dielectric properties of vegetable oils and their constituents. In Diagnostic of Electrical Machines and Insulating Systems in Electrical Engineering (DEMISEE), pp. 16–19. IEEE.10.1109/DEMISEE.2016.7530478Google Scholar
Stone, H. A., Stroock, A. D. & Ajdari, A. 2004 Engineering flows in small devices: microfluidics toward a lab-on-a-chip. Annu. Rev. Fluid Mech. 36, 381–411.10.1146/annurev.fluid.36.050802.122124Google Scholar
Stroock, A. D., Dertinger, S. K. W., Ajdari, A., Mezić, I., Stone, H. A. & Whitesides, G. M. 2002 Chaotic mixer for microchannels. Science 295 (5555), 647–651.Google Scholar
Suh, Y. K. 2012 Modeling and simulation of ion transport in dielectric liquids – fundamentals and review. IEEE Trans. Dielec. Elec. Insul. 19 (3), 831–848.Google Scholar
Talon, L., Goyal, N. & Meiburg, E. 2013 Variable density and viscosity, miscible displacements in horizontal Hele-Shaw cells. Part 1. Linear stability analysis. J. Fluid Mech. 721, 268–294.10.1017/jfm.2013.63Google Scholar
Talon, L. & Meiburg, E. 2011 Plane poiseuille flow of miscible layers with different viscosities: instabilities in the Stokes flow regime. J. Fluid Mech. 686, 484–506.Google Scholar
Tan, C. T. & Homsy, G. M. 1986 Stability of miscible displacements in porous media: rectilinear flow. Phys. Fluids 29 (11), 3549–3556.Google Scholar
Timung, S., Chaudhuri, J., Borthakur, M. P., Mandal, T. K., Biswas, G. & Bandyopadhyay, D. 2017 Electric field mediated spraying of miniaturized droplets inside microchannel. Electrophoresis 38 (11), 1450–1457.Google Scholar
Tobazeon, R., Haidara, M. & Atten, P. 1984 Ion injection and kerr plots in liquids with blade-plane electrodes. J. Phys. D: Appl. Phys. 17 (6), 1293–1301.Google Scholar
Traoré, P. & Pérez, A. T. 2012 Two-dimensional numerical analysis of electroconvection in a dielectric liquid subjected to strong unipolar injection. Phys. Fluids 24 (3), 037102.Google Scholar
Tsukahara, Y., Hirose, Y. & Otsubo, Y. 2013 Effect of electrode materials on electrohydrodynamic flows of ethanol. Colloids Surf. A 425, 76–82.10.1016/j.colsurfa.2013.02.060Google Scholar
Vasilkov, S. A., Chirkov, V. A. & Stishkov, Y. K. 2017 Study on high-voltage conductivity provided solely by field-enhanced dissociation in liquid dielectrics. J. Electrostat. 88, 81–87.10.1016/j.elstat.2016.12.001Google Scholar
Vázquez, P. A., Georghiou, G. E. & Castellanos, A. 2006 Characterization of injection instabilities in electrohydrodynamics by numerical modelling: comparison of particle in cell and flux corrected transport methods for electroconvection between two plates. J. Phys. D: Appl. Phys. 39 (13), 2754–2763.Google Scholar
Verma, M. K. S., Ganneboyina, S. R., Vinayak, R. R. & Ghatak, A. 2008 Three-dimensional multihelical microfluidic mixers for rapid mixing of liquids. Langmuir 24 (5), 2248–2251.Google Scholar
Vilkner, T., Janasek, D. & Manz, A. 2004 Micro total analysis systems. Recent developments. Anal. Chem. 76 (12), 3373–3386.Google Scholar
Wall, D. P. & Wilson, S. K. 1996 The linear stability of channel flow of fluid with temperature-dependent viscosity. J. Fluid Mech. 323, 107–132.Google Scholar
Wang, B. F. & Sheu, T. W. H. 2016 Numerical investigation of electrohydrodynamic instability and bifurcation in a dielectric liquid subjected to unipolar injection. Comput. Fluids 136, 1–10.Google Scholar
Wang, G., Yang, F., Zhao, W. & Chen, C.-P. 2016 On micro-electrokinetic scalar turbulence in microfluidics at a low Reynolds number. Lab on a Chip 16 (6), 1030–1038.Google Scholar
Wang, G. R., Yang, F. & Zhao, W. 2014 There can be turbulence in microfluidics at low Reynolds number. Lab on a Chip 14 (8), 1452–1458.10.1039/C3LC51403JGoogle Scholar
Wang, J., Ibáñez, A., Chatrathi, M. P. & Escarpa, A. 2001 Electrochemical enzyme immunoassays on microchip platforms. Anal. Chem. 73 (21), 5323–5327.10.1021/ac010808hGoogle Scholar
Watson, P. K., Schneider, J. M. & Till, H. R. 1970 Electrohydrodynamic stability of space-charge-limited currents in dielectric liquids. II. Experimental study. Phys. Fluids 13 (8), 1955–1961.10.1063/1.1693191Google Scholar
Weideman, J. A. & Reddy, S. C. 2000 A matlab differentiation matrix suite. ACM Trans. Math. Softw. 26 (4), 465–519.10.1145/365723.365727Google Scholar
Wu, J., Pérez, A. T., Traoré, P. & Vázquez, P. A. 2015 Complex flow patterns at the onset of annular electroconvection in a dielectric liquid subjected to an arbitrary unipolar injection. IEEE Trans. Dielec. Elec. Insul. 22 (5), 2637–2645.Google Scholar
Wu, J., Traoré, P., Vázquez, P. A. & Pérez, A. T. 2013 Onset of convection in a finite two-dimensional container due to unipolar injection of ions. Phys. Rev. E 88, 053018.Google Scholar
Yi, M., Qian, S. & Bau, H. H. 2002 A magnetohydrodynamic chaotic stirrer. J. Fluid Mech. 468, 153–177.Google Scholar
Zhang, H. B., Edirisinghe, M. J. & Jayasinghe, S. N. 2006 Flow behaviour of dielectric liquids in an electric field. J. Fluid Mech. 558, 103–111.10.1017/S0022112006000188Google Scholar
Zhang, M. 2016 Weakly nonlinear stability analysis of subcritical electrohydrodynamic flow subject to strong unipolar injection. J. Fluid Mech. 792, 328–363.Google Scholar
Zhang, M., Martinelli, F., Wu, J., Schmid, P. J. & Quadrio, M. 2015 Modal and non-modal stability analysis of electrohydrodynamic flow with and without cross-flow. J. Fluid Mech. 770, 319–349.10.1017/jfm.2015.134Google Scholar
Zhao, H. & Bau, H. H. 2007 Microfluidic chaotic stirrer utilizing induced-charge electro-osmosis. Phys. Rev. E 75 (6), 066217.Google Scholar

Dutta et al. supplementary material 1

Experimental video depicting linear regime I of instability developed in a benzenesilicone oil stratified flow through a 420 μm diameter channel, upon application of 0 – 300 V DC voltage through 420 μm diameter copper wire electrodes. The arrow indicates the direction of flow.

Download Dutta et al. supplementary material 1(Video)
Video 3.5 MB

Dutta et al. supplementary material 2

Experimental video depicting non-linear regime II of instability developed in a benzene-silicone oil stratified flow through a 420 μm diameter channel, upon application of 300 – 600 V DC voltage through 420 μm diameter copper wire electrodes. The arrow indicates the direction of flow.

Download Dutta et al. supplementary material 2(Video)
Video 7.6 MB

Dutta et al. supplementary material 3

Experimental video depicting non-linear regime III of instability developed in a benzene-silicone oil stratified flow through a 420 μm diameter channel, upon application of 600 – 900 V DC voltage through 420 μm diameter copper wire electrodes. The arrow indicates the direction of flow.

Download Dutta et al. supplementary material 3(Video)
Video 5.6 MB

Dutta et al. supplementary material 4

Experimental video depicting non-linear chaotic regime IV of instability developed in a benzene-silicone oil stratified flow through a 420 μm diameter channel, upon application of 900 – 1000 V DC voltage through 420 μm diameter copper wire electrodes. The arrow indicates the direction of flow.

Download Dutta et al. supplementary material 4(Video)
Video 16.8 MB

Dutta et al. supplementary material 5

Experimental video depicting response of benzene-silicone oil stratified flow through a 420 μm diameter channel, upon application of 0 – 500 V DC voltage through multiple copper wire electrodes of 420 μm diameter. The arrow indicates the direction of flow.

Download Dutta et al. supplementary material 5(Video)
Video 11.5 MB

Dutta et al. supplementary material 6

CFD simulation video (corresponding to figure 19) depicting response of benzenesilicone oil stratified flow through a 420 μm diameter channel, upon application of 300 V DC voltage through copper wire electrodes of 420 μm diameter. The arrow indicates the direction of flow.

Download Dutta et al. supplementary material 6(Video)
Video 690.8 KB

Dutta et al. supplementary material 7

CFD simulation depicting response of benzene-oleic acid stratified flow through a 420 μm diameter channel, upon application of 300 V DC voltage through copper wire electrodes of 420 μm diameter. The arrow indicates the direction of flow. The parameters used for the simulation are Sc = 500, KL=-4, EL=-0.15, and Re = 0.5.

Download Dutta et al. supplementary material 7(Video)
Video 743.4 KB

Dutton et al. supplementary movie 8

CFD simulation depicting response of benzene-silicone oil stratified flow through a 420 μm diameter channel, upon application of 500 V DC voltage through copper wire electrodes of 420 μm diameter. The arrow indicates the direction of flow. The parameters used for the simulation are Sc = 500, KL=-0, EL=-0.15, and Re = 0.5.

Download Dutton et al. supplementary movie 8(Video)
Video 552.4 KB