Hostname: page-component-78c5997874-lj6df Total loading time: 0 Render date: 2024-11-05T02:40:30.308Z Has data issue: false hasContentIssue false
  • English
  • Français

The elastic Landau–Levich problem

Published online by Cambridge University Press:  30 August 2013

Harish N. Dixit  and
G. M. Homsy
Harish N. Dixit*
Affiliation:
Department of Mathematics, University of British Columbia, 1984 Mathematics Road, Vancouver, BC, V6T 1Z2, Canada
G. M. Homsy
Affiliation:
Department of Mathematics, University of British Columbia, 1984 Mathematics Road, Vancouver, BC, V6T 1Z2, Canada
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We study the classical Landau–Levich dip-coating problem in the case where the interface has significant elasticity. One aim of this work is to unravel the effect of surface-adsorbed hydrophobic particles on Landau–Levich flow. Motivated by recent findings (Vella, Aussillous & Mahadevan, Europhys. Lett., vol. 68, 2004, pp. 212–218) that a jammed monolayer of adsorbed particles on a fluid interface makes it respond akin to an elastic solid, we use the Helfrich elasticity model to study the effect of interfacial elasticity on Landau–Levich flow. We define an elasticity number, $\mathit{El}$, which represents the relative strength of viscous forces to elasticity. The main assumptions of the theory are that 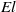$\mathit{El}$ be small, and that surface tension effects are negligible. The shape of the free surface is formulated as a nonlinear boundary value problem: we develop the solution as an asymptotic expansion in the small parameter 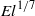${\mathit{El}}^{1/ 7} $ and use the method of matched asymptotic expansions to determine the film thickness as a function of 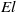$\mathit{El}$. The solution to the shape of the static meniscus is not as straightforward as in the classical Landau–Levich problem, as evaluation of higher-order effects is necessary in order to close the problem. A remarkable aspect of the problem is the occurrence of multiple solutions, and five of these are found numerically. In any event, the film thickness varies as 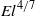${\mathit{El}}^{4/ 7} $ in qualitative agreement with the experiments of Ouriemi & Homsy (Phys. Fluids, 2013, in press).

JFM classification

Interfacial Flows (free surface): Capillary flows Low-Reynolds-number flows: Lubrication theory Materials Processing Flows: Coating
Type
Papers
Information
Journal of Fluid Mechanics , Volume 732 , 10 October 2013 , pp. 5 - 28
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Audoly, B. 2011 Localized buckling of a floating elastica. Phys. Rev. E 84, 011605.CrossRefGoogle ScholarPubMed
Aussillous, P. & Quéré, D. 2001 Liquid marbles. Nature 411, 924–927.CrossRefGoogle ScholarPubMed
Binks, B. P. 2002 Particles as surfactants - similarities and differences. Curr. Opin. Colloid Interface Sci. 7, 21–41.CrossRefGoogle Scholar
Bretherton, F. P. 1961 The motion of long bubbles in tubes. J. Fluid Mech. 10, 166–188.CrossRefGoogle Scholar
Campana, D. M., Ubal, S., Giavedon, M. D. & Saita, F. A. 2010 Numerical prediction of the film thickening due to surfactants in the Landau–Levich problem. Phys. Fluids 22, 032103.CrossRefGoogle Scholar
Campana, D. M., Ubal, S., Giavedon, M. D. & Saita, F. A. 2011 A deeper insight into the dip coating process in the presence of insoluble surfactants: a numerical analysis. Phys. Fluids 23, 052102.CrossRefGoogle Scholar
Chan, D. Y. C., Henry, J. D. Jr. & White, L. R. 1981 The interaction of colloidal particles collected at a fluid interface. J. Colloid Interface Sci. 79, 410–418.CrossRefGoogle Scholar
Daicic, J., Fogden, A., Carlsson, I., Wennerström, H. & Jönsson, B. 1996 Bending of ionic surfactant monolayers. Phys. Rev. E 54, 3984–3998.CrossRefGoogle ScholarPubMed
Danov, K. D. & Kralchevsky, P. A. 2010 Capillary forces between particles at a liquid interface: general theoretical approach and interactions between capillary multipoles. Adv. Colloid Interface Sci. 154, 91–103.CrossRefGoogle Scholar
Derjaguin, B. V. 1943 On the thickness of the liquid film adhering to the walls of a vessel after emptying. Acta Physicochim. USSR 20, 349–352.Google Scholar
Diamant, H. & Witten, T. A. 2011 Compression induced folding of a sheet: an integrable system. Phys. Rev. Lett. 107, 164302.CrossRefGoogle ScholarPubMed
Dinsmore, A. D., Hsu, M. F., Nikolaides, M. G., Marquez, M., Bausch, A. R. & Weitz, D. A. 2002 Colloidosomes: selectively permeable capsules composed of colloidal particles. Science 298, 1006–1009.CrossRefGoogle ScholarPubMed
Fainerman, V. B., Kovalchuk, V. I., Lucassen-Reynders, E. H., Grigouriev, D. O., Ferri, J. K., Leser, M. E., Michel, M., Miller, R. & Möhwald, H. 2006 Surface-pressure isotherms of monolayers formed by microsize and nanosize particles. Langmuir 22, 1701–1705.CrossRefGoogle ScholarPubMed
Fuller, G. G. & Vermant, J. 2012 Complex fluid–fluid interfaces: rheology and structure. Ann. Rev. Chem. Biomol. Engng 3, 519–543.CrossRefGoogle ScholarPubMed
Gaskell, P. H., Savage, M. D., Summers, J. L. & Thompson, H. M. 1995 Modelling and analysis of meniscus roll coating. J. Fluid Mech. 298, 113–137.CrossRefGoogle Scholar
Gaver, D. P. III, Halpern, D., Jensen, O. E. & Grotberg, J. B. 1996 The steady motion of a semi-infinite bubble through a flexible-walled channel. J. Fluid Mech. 319, 25–65.CrossRefGoogle Scholar
Gaver, D. P. III, Samsel, R. W. & Solway, J. 1990 Effects of surface tension and viscosity on airway reopening. J. Appl. Physiol. 69, 74–85.CrossRefGoogle ScholarPubMed
Gifford, W. A. & Scriven, L. E. 1971 On the attraction of floating particles. Chem. Engng Sci. 26, 287–297.CrossRefGoogle Scholar
Groenveld, P. 1970a Dip-coating by withdrawal of liquid films. PhD thesis, Delft University.Google Scholar
Groenveld, P. 1970b Low capillary number withdrawal. Chem. Engng Sci. 25, 1259–1266.CrossRefGoogle Scholar
Grotberg, J. B. & Jensen, O. E. 2004 Biofluid mechanics in flexible tubes. Ann. Rev. Fluid Mech. 36, 121–147.CrossRefGoogle Scholar
Helfrich, W. 1973 Elastic properties of lipid bilayers – theory and possible experiments. Z. Naturforsch. 28, 693–703.CrossRefGoogle ScholarPubMed
Jensen, O. E., Horsburgh, M. K., Halpern, D. & Gaver, D. P. III 2002 The steady propagation of a bubble in a flexible-walled channel: asymptotic and computational models. Phys. Fluids 14, 443–457.CrossRefGoogle Scholar
Kaoui, B., Ristow, G. H., Cantat, I., Misbah, C. & Zimmermann, W. 2008 Lateral migration of a two-dimensional vesicle in unbounded poiseuille flow. Phys. Rev. E 77, 021903.CrossRefGoogle ScholarPubMed
Krechetnikov, R. & Homsy, G. M. 2005 Experimental study of substrate roughness and surfactant effects on the Landau–Levich law. Phys. Fluids 17, 1021108.CrossRefGoogle Scholar
Kruglyakov, P. & Nushtayeva, A. 2004 Emulsions stabilizied by solid particles: the role of capillary pressure in the emulsion films. In Emulsions: Structure, Stability and Interactions (ed. Petsev, D. N.), Interface Science and Technology, vol. 4, pp. 641–676. Elsevier.CrossRefGoogle Scholar
Landau, L. & Levich, B. 1942 Dragging of a liquid by a moving plate. Acta Physicochim. USSR 7, 42–54.Google Scholar
Mayer, H. C. & Krechetnikov, R. 2012 Landau–Levich flow visualization: revealing the flow topology responsible for the film thickening phenomena. Phys. Fluids 24, 052103.CrossRefGoogle Scholar
McHale, G. & Newton, M. I. 2011 Liquid marbles: principles and applications. Soft Matt. 7, 5473–5481.CrossRefGoogle Scholar
McLean, J. W. & Saffman, P. G. 1981 The effect of surface tension on the shape of fingers in a Hele Shaw cell. J. Fluid Mech. 102, 455–469.CrossRefGoogle Scholar
Monteux, C., Kirkwood, J., Xu, H., Jung, E. & Fuller, G. G. 2007 Determining the mechanical response of particle-laden fluid interfaces using surface pressure isotherms and bulk pressure measurements of droplets. Phys. Chem. Phys. 9, 6344–6350.CrossRefGoogle ScholarPubMed
Nicolson, M. M. 1949 The interaction between floating particles. Proc. Camb. Phil. Soc. 45, 288–295.CrossRefGoogle Scholar
Okubo, T. 1995 Surface tension of structured colloidal suspensions of polystyrene and silica spheres at air–water interface. J. Colloid Interface Sci. 171, 55–62.CrossRefGoogle Scholar
Ouriemi, M. & Homsy, G. M. 2013 Experimental study of the effect of surface-adsorbed hydrophobic particles on the Landau–Levich law. Phys. Fluids (in press).CrossRefGoogle Scholar
Park, C.-W. 1991 Effects of insoluble surfactants on dip coating. J. Colloid Interface Sci. 146, 382–394.CrossRefGoogle Scholar
Park, C.-W. & Homsy, G. M. 1984 Two-phase displacement in Hele-Shaw cells: theory. J. Fluid Mech. 139, 291–308.CrossRefGoogle Scholar
Pickering, S. U. 1907 Emulsions. J. Chem. Soc. Trans. 91, 2001–2021.CrossRefGoogle Scholar
Planchette, C., Lorenceau, E. & Biance, A.-L. 2012 Surface wave on a particle raft. Soft Matt. 8, 2444–2451.CrossRefGoogle Scholar
Pocivavsek, L., Dellsy, R., Kern, A., Johnson, S., Lin, B., Lee, K. Y. C. & Cerda, E. 2008 Stress and fold localization in thin elastic membranes. Science 320, 912–916.CrossRefGoogle ScholarPubMed
Py, C., Reverdy, P., Doppler, L., Bico, José, Roman, B. & Baroud, C. N. 2007 Capillary origami: spontaneous wrapping of a droplet with an elastic sheet. Phys. Rev. Lett. 98, 156103.CrossRefGoogle ScholarPubMed
Quéré, D. 1999 Fluid coating on a fibre. Ann. Rev. Fluid Mech. 31, 347–384.CrossRefGoogle Scholar
Ramsden, W. 1903 Separation of solids in the surface-layers of solutions and ‘suspensions’ (Observations on surface-membranes, bubbles, emulsions and mechanical coagulation) – preliminary account. Proc. R. Soc. Lond. 72, 156–164.Google Scholar
Ratulowski, J. & Chang, H.-C. 1990 Marangoni effects of trace impurities on the motion of long gas bubbles in capillaries. J. Fluid Mech. 210, 303–328.CrossRefGoogle Scholar
Reynaert, S., Moldenaers, P. & Vermant, J. 2007 Interfacial rheology of stable and weakly aggregated two-dimensional suspensions. Phys. Chem. Phys. 9, 6463–6475.CrossRefGoogle ScholarPubMed
Rivetti, M. & Antkowiak, A. 2013Elasto-capillary meniscus: pulling out a soft strip sticking to a liquid surface. Preprint.CrossRefGoogle Scholar
Saffman, P. G. & Taylor, G. I. 1958 The penetration of a fluid into a porous medum or Hele-Shaw cell containing a more viscous liquid. Proc. R. Soc. Lond. A 245, 312–329.Google Scholar
Snoeijer, J. H., Ziegler, J., Andreotti, B., Fermigier, M. & Eggers, J. 2008 Thick films of viscous fluid coating a plate withdrawn from a liquid reservoir. Phys. Rev. Lett. 100, 244502.CrossRefGoogle ScholarPubMed
Stebe, K. J. & Barthès-Biesel, D. 1995 Marangoni effects of adsorption–desorption controlled surfactants on the leading end of an infinitely long bubble in a capillary. J. Fluid Mech. 286, 25–48.CrossRefGoogle Scholar
Subramaniam, A. B., Abkarian, M., Mahadevan, L. & Stone, H. A. 2005a Non-spherical bubbles. Nature 438, 938.Google ScholarPubMed
Subramaniam, A. B., Abkarian, M. & Stone, H. A. 2005b Controlled assembly of jammed colloidal shells on fluid droplets. Nature Mater. 4, 553–556.CrossRefGoogle ScholarPubMed
Szleifer, I., Kramer, D., Ben-Shaul, A., Gelbart, W. M. & Safran, S. A. 1990 Molecular theory of curvature elasticity in surfactant films. J. Chem. Phys. 92, 6800–6817.CrossRefGoogle Scholar
Van Dyke, M. D. 1975 Perturbation Methods in Fluid Mechanics. Parabolic.Google Scholar
Vanden-Broeck, J. M. 1984 Rising bubbles in a two-dimensional tube with surface tension. Phys. Fluids 27, 2604–2607.CrossRefGoogle Scholar
Varshney, A., Sane, A., Ghosh, S. & Bhattacharya, S. 2012 Amorphous to amorphous transition in particle rafts. Phys. Rev. E 86, 031402.CrossRefGoogle ScholarPubMed
Vella, D., Aussillous, P. & Mahadevan, L. 2004 Elasticity of an interfacial particle raft. Europhys. Lett. 68, 212–218.CrossRefGoogle Scholar
Würger, A. 2000 Bending elasticity of surfactant films: the role of the hydrophobic tails. Phys. Rev. Lett. 85, 337–340.CrossRefGoogle ScholarPubMed
Yunker, P. J., Gratale, M., Lohr, M. A., Still, T., Lubensky, T. C. & Yodh, A. G. 2012 Influence of particle shape on bending rigidity of colloidal monolayer membranes and particle deposition during droplet evapouration in confined geometries. Phys. Rev. Lett. 108, 228303.CrossRefGoogle Scholar