Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-23T08:29:34.309Z Has data issue: false hasContentIssue false
  • English
  • Français

Effects of fluctuating energy input on the small scales in turbulence

Published online by Cambridge University Press:  27 November 2013

Chen-Chi Chien ,
Daniel B. Blum  and
Greg A. Voth
Chen-Chi Chien
Affiliation:
Department of Physics, Wesleyan University, Middletown, Connecticut 06459, USA
Daniel B. Blum
Affiliation:
Department of Physics, Wesleyan University, Middletown, Connecticut 06459, USA
Greg A. Voth*
Affiliation:
Department of Physics, Wesleyan University, Middletown, Connecticut 06459, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In the standard cascade picture of three-dimensional turbulent fluid flows, energy is input at a constant rate at large scales. Energy is then transferred to smaller scales by an intermittent process that has been the focus of a vast literature. However, the energy input at large scales is not constant in most real turbulent flows. We explore the signatures of these fluctuations of large-scale energy input on small-scale turbulence statistics. 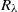Measurements were made in a flow between oscillating grids, with ${R}_{\lambda } $ up to 262, in which temporal variations in the large-scale energy input can be introduced by modulating the oscillating grid frequency. We find that the Kolmogorov constant for second-order longitudinal structure functions depends on the magnitude of the fluctuations in the large-scale energy input. We can quantitatively predict the measured change with a model based on Kolmogorov’s refined similarity theory. The effects of fluctuations of the energy input can also be observed using structure functions conditioned on the instantaneous large-scale velocity. A linear parametrization using the curvature of the conditional structure functions provides a fairly good match with the measured changes in the Kolmogorov constant. Conditional structure functions are found to provide a more sensitive measure of the presence of fluctuations in the large-scale energy input than inertial range scaling coefficients.

JFM classification

Turbulent Flows: Homogeneous turbulence Turbulent Flows: Intermittency Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 737 , 25 December 2013 , pp. 527 - 551
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Antonia, R. A. & Burattini, P. 2006 Approach to the 4/5 law in homogeneous isotropic turbulence. J. Fluid Mech. 550, 175–184.CrossRefGoogle Scholar
Batchelor, G. K. & Townsend, A. A. 1949 The nature of turbulent motion at large wave-numbers. Proc. R. Soc. Lond. A 199, 238–255.Google Scholar
Blum, D. B., Bewley, G. P., Bodenschatz, E., Gibert, M., Gylfason, A., Mydlarski, L., Voth, G. A., Xu, H. & Yeung, P. K. 2011 Signatures of non-universal large scales in conditional structure functions from various turbulent flows. New J. Phys. 34, 114020.Google Scholar
Blum, D. B., Kunwar, S. B., Johnson, J. & Voth, G. A. 2010 Effects of nonuniversal large scales on conditional structure functions in turbulence. Phys. Fluids 22, 015107.CrossRefGoogle Scholar
Bos, W. J. T., Clark, T. T. & Rubinstein, R. 2007a Small scale response and modelling of periodically forced turbulence. Phys. Fluids 19, 055107.CrossRefGoogle Scholar
Bos, W. J. T., Shao, L. & Bertoglio, J.-P. 2007b Spectral imbalance and the normalized dissipation rate of turbulence. Phys. Fluids 19, 045101.CrossRefGoogle Scholar
Cadot, O., Titon, J. H. & Bonn, D. 2003 Observation of resonances in modulated turbulence. J. Fluid Mech. 485, 161–170.CrossRefGoogle Scholar
Chan, D., Stich, D. & Voth, G. A. 2007 Real time image compression for high-speed particle tracking. Rev. Sci. Instrum. 78, 023704.CrossRefGoogle ScholarPubMed
Davidson, P. A. 2004 Turbulence: An Introduction for Scientists and Engineers. Oxford University Press.Google Scholar
Donzis, D. A. & Yeung, P. K. 2010 Resolution effects and scaling in numerical simulations of passive scalar mixing in turbulence. Physica D 239, 1278–1287.CrossRefGoogle Scholar
Frisch, U. 1995 Turbulence: The legacy of A. N. Kolmogorov. Cambridge University Press.CrossRefGoogle Scholar
von der Heydt, A., Grossmann, S. & Lohse, D. 2003 Response maxima in modulated turbulence. Phys. Rev. E 67, 046308.CrossRefGoogle ScholarPubMed
Hinze, J. O. 1959 Turbulence: An Introduction to its Mechanism and Theory. McGraw-Hill.Google Scholar
Hinze, J. O. 1975 Turbulence, 2nd edn. McGraw-Hill.Google Scholar
Ishihara, T., Gotoh, T. & Kaneda, Y. 2009 Study of high-Reynolds number isotropic turbulence by direct numerical simulation. Annu. Rev. Fluid Mech. 41, 165–180.CrossRefGoogle Scholar
Jin, X.-L. & Xia, K.-Q. 2008 An experimental study of kicked thermal turbulence. J. Fluid Mech. 606, 133–151.Google Scholar
Kang, H. S. & Meneveau, C. 2008 Experimental study of an active grid-generated shearless mixing layer and comparisons with large-eddy simulation. Phys. Fluids 20, 125102.CrossRefGoogle Scholar
Kolmogorov, A. N. 1941 The local structure of turbulence in incompressible viscous fluid for very large Reynolds numbers. Dokl. Akad. Nauk SSSR 30, 301–305.Google Scholar
Kolmogorov, A. N. 1962 A refinement of previous hypotheses concerning the local structure of turbulence in a viscous incompressible fluid at high Reynolds number. J. Fluid Mech. 13, 82–85.CrossRefGoogle Scholar
Kuczaj, A. K., Geurts, B. J. & Lohse, D. 2006 Response maxima in time-modulated turbulence: direct numerical simulations. Europhys. Lett. 73, 851–857.CrossRefGoogle Scholar
Kuczaj, A. K., Geurts, B. J., Lohse, D & van de Water, W. 2008 Turbulence modification by periodically modulated scale-dependent forcing. Comput. Fluids 37, 816–824.CrossRefGoogle Scholar
Landau, L. D. & Lifschitz, E. M. 1944 Fluid Mechanics (in Russian) USSR. (Also 2nd edition, Pergamon Press, 1987).Google Scholar
Mi, J. & Antonia, R. A. 2001 Effects of large-scale intermittency and mean shear on scaling-range exponents in a turbulent jet. Phys. Rev. E 64, 026302.CrossRefGoogle Scholar
Moisy, F., Tabeling, P. & Willaime, H. 1999 Kolmogorov equation in a fully developed turbulence experiment. Phys. Rev. Lett. 82, 3994–3997.CrossRefGoogle Scholar
Monin, A. S. & Yaglom, A. M. 1971 Statistical Fluid Mechanics: Mechanics of Turbulence. MIT Press.Google Scholar
Mouri, H., Takaoka, M., Hori, A. & Kawashima, Y. 2006 On Landau’s prediction for large-scale fluctuation of turbulence energy dissipation. Phys. Fluids 18, 015103.CrossRefGoogle Scholar
Obukhov, A. M. 1962 Some specific features of atmospheric turbulence. J. Fluid Mech 13, 77–81.CrossRefGoogle Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.CrossRefGoogle Scholar
Praskovsky, A. A., Gledzer, E. B., Karyakin, M. Y. & Zhou, Y. 1993 The sweeping decorrelation hypothesis and energy-inertial scale interaction in high Reynolds number flows. J. Fluid Mech. 248, 493–511.CrossRefGoogle Scholar
Sreenivasan, K. R. 1995 On the universality of the Kolmogorov constant. Phys. Fluids 7, 2778–2784.CrossRefGoogle Scholar
Sreenivasan, K. R. & Antonia, R. A. 1997 The phenomenology of small-scale turbulence. Annu. Rev. Fluid Mech. 29, 435–472.CrossRefGoogle Scholar
Sreenivasan, K. R. & Dhruva, B. 1998 Is there scaling in high-Reynolds-number turbulence? Progr. Theor. Phys. 130, 103–120.CrossRefGoogle Scholar
Sreenivasan, K. R. & Stolovitzky, G. 1996 Statistical dependence of inertial range properties on large scales in a high-Reynolds-number shear flow. Phys. Rev. Lett. 77, 2218–2221.CrossRefGoogle Scholar
Veeravalli, S. & Warhaft, Z. 1989 The shearless turbulent mixing layer. J. Fluid Mech. 207, 191–229.CrossRefGoogle Scholar
Yeung, P. K. & Zhou, Y. 1997 Universality of the Kolmogorov constant in numerical simulations of turbulence. Phys. Rev. E 56, 1746–1752.CrossRefGoogle Scholar