Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Sánchez Umbría, J.
and
Net, M.
2021.
Three-dimensional quasiperiodic torsional flows in rotating spherical fluids at very low Prandtl numbers.
Physics of Fluids,
Vol. 33,
Issue. 11,
Guervilly, Céline
2022.
Fingering Convection in the Stably Stratified Layers of Planetary Cores.
Journal of Geophysical Research: Planets,
Vol. 127,
Issue. 11,
Sánchez Umbría, J.
and
Net, M.
2022.
Bifurcations to quasiperiodicity of the torsional solutions of convection in rotating fluid spheres: Techniques and results.
Physics of Fluids,
Vol. 34,
Issue. 11,
Barrois, O
Gastine, T
and
Finlay, C C
2022.
Comparison of quasi-geostrophic, hybrid and 3-D models of planetary core convection.
Geophysical Journal International,
Vol. 231,
Issue. 1,
p.
129.
Kolhey, Patrick
Stellmach, Stephan
and
Heyner, Daniel
2022.
Influence of boundary conditions on rapidly rotating convection and its dynamo action in a plane fluid layer.
Physical Review Fluids,
Vol. 7,
Issue. 4,
Sánchez Umbría, J.
and
Net, M.
2022.
Thermal convection in rotating spherical geometry: A numerical overview of the transitions from periodic axisymmetric to temporally complex three-dimensional flows.
Physical Review E,
Vol. 106,
Issue. 6,
Rein, F.
Carénini, L.
Fichot, F.
Favier, B.
and
Le Bars, M.
2023.
Interaction between forced and natural convection in a thin cylindrical fluid layer at low Prandtl number.
Journal of Fluid Mechanics,
Vol. 977,
Issue. ,
Olsthoorn, Jason
2023.
Accounting for surface temperature variations in Rayleigh-Bénard convection.
Physical Review Fluids,
Vol. 8,
Issue. 3,
Folch, Arnau
Abril, Claudia
Afanasiev, Michael
Amati, Giorgio
Bader, Michael
Badia, Rosa M.
Bayraktar, Hafize B.
Barsotti, Sara
Basili, Roberto
Bernardi, Fabrizio
Boehm, Christian
Brizuela, Beatriz
Brogi, Federico
Cabrera, Eduardo
Casarotti, Emanuele
Castro, Manuel J.
Cerminara, Matteo
Cirella, Antonella
Cheptsov, Alexey
Conejero, Javier
Costa, Antonio
de la Asunción, Marc
de la Puente, Josep
Djuric, Marco
Dorozhinskii, Ravil
Espinosa, Gabriela
Esposti-Ongaro, Tomaso
Farnós, Joan
Favretto-Cristini, Nathalie
Fichtner, Andreas
Fournier, Alexandre
Gabriel, Alice-Agnes
Gallard, Jean-Matthieu
Gibbons, Steven J.
Glimsdal, Sylfest
González-Vida, José Manuel
Gracia, Jose
Gregorio, Rose
Gutierrez, Natalia
Halldorsson, Benedikt
Hamitou, Okba
Houzeaux, Guillaume
Jaure, Stephan
Kessar, Mouloud
Krenz, Lukas
Krischer, Lion
Laforet, Soline
Lanucara, Piero
Li, Bo
Lorenzino, Maria Concetta
Lorito, Stefano
Løvholt, Finn
Macedonio, Giovanni
Macías, Jorge
Marín, Guillermo
Martínez Montesinos, Beatriz
Mingari, Leonardo
Moguilny, Geneviève
Montellier, Vadim
Monterrubio-Velasco, Marisol
Moulard, Georges Emmanuel
Nagaso, Masaru
Nazaria, Massimo
Niethammer, Christoph
Pardini, Federica
Pienkowska, Marta
Pizzimenti, Luca
Poiata, Natalia
Rannabauer, Leonhard
Rojas, Otilio
Rodriguez, Juan Esteban
Romano, Fabrizio
Rudyy, Oleksandr
Ruggiero, Vittorio
Samfass, Philipp
Sánchez-Linares, Carlos
Sanchez, Sabrina
Sandri, Laura
Scala, Antonio
Schaeffer, Nathanael
Schuchart, Joseph
Selva, Jacopo
Sergeant, Amadine
Stallone, Angela
Taroni, Matteo
Thrastarson, Solvi
Titos, Manuel
Tonelllo, Nadia
Tonini, Roberto
Ulrich, Thomas
Vilotte, Jean-Pierre
Vöge, Malte
Volpe, Manuela
Aniko Wirp, Sara
and
Wössner, Uwe
2023.
The EU Center of Excellence for Exascale in Solid Earth (ChEESE): Implementation, results, and roadmap for the second phase.
Future Generation Computer Systems,
Vol. 146,
Issue. ,
p.
47.
Sánchez Umbría, J.
and
Net, M.
2023.
Effect of Robin boundary conditions on the onset of convective torsional flows in rotating fluid spheres.
Physics of Fluids,
Vol. 35,
Issue. 10,
Olsthoorn, Jason
2024.
Atmospheric cooling of freshwater near the temperature of maximum density.
Physical Review Fluids,
Vol. 9,
Issue. 11,
Marušić-Paloka, Eduard
and
Pažanin, Igor
2024.
The Robin boundary condition for modelling heat transfer.
Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 480,
Issue. 2286,
Schneider, Baruch
Schneiderová, Diana
and
Zhang, Yifan
2025.
Optimization of Robin Laplacian Eigenvalue With Indefinite Weight in Spherical Shell.
Mathematical Methods in the Applied Sciences,
Vol. 48,
Issue. 6,
p.
6586.
Figueroa, Pablo
Iturriaga, Leonelo
and
Topp, Erwin
2025.
Sign-changing solutions for the sinh–Poisson equation with Robin boundary condition.
Journal d'Analyse Mathématique,
Horn, Susanne
and
Aurnou, Jonathan M.
2025.
The Elbert range of magnetostrophic convection. II. Comparing linear theory to nonlinear low-
Rm
simulations
.
Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 481,
Issue. 2310,
Guervilly, C.
and
Dormy, E.
2025.
The Cross‐Over From Viscous to Inertial Lengthscales in Rapidly‐Rotating Convection.
Geophysical Research Letters,
Vol. 52,
Issue. 7,
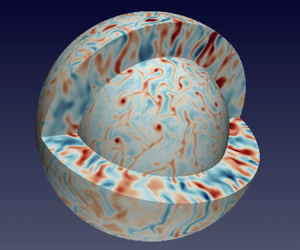
 ${{\textit {Bi}}}$ as a proportionality factor in non-dimensional form. Varying
${{\textit {Bi}}}$ as a proportionality factor in non-dimensional form. Varying  ${{\textit {Bi}}}$ allows us to transition from fixed temperature for
${{\textit {Bi}}}$ allows us to transition from fixed temperature for  ${{\textit {Bi}}}=+\infty$, to imposed heat flux for
${{\textit {Bi}}}=+\infty$, to imposed heat flux for  ${{\textit {Bi}}}=0$. We find that the onset of convection is only affected by
${{\textit {Bi}}}=0$. We find that the onset of convection is only affected by  ${{\textit {Bi}}}$ in the non-rotating case. Far from onset, considering an effective Rayleigh number and a generalized Nusselt number, we show that the Nusselt and Péclet numbers follow standard universal scaling laws, independent of
${{\textit {Bi}}}$ in the non-rotating case. Far from onset, considering an effective Rayleigh number and a generalized Nusselt number, we show that the Nusselt and Péclet numbers follow standard universal scaling laws, independent of  ${{\textit {Bi}}}$ in all cases considered. However, for the non-rotating limit, the large-scale flow structure keeps the signature of the boundary condition with more vigorous large scales for smaller
${{\textit {Bi}}}$ in all cases considered. However, for the non-rotating limit, the large-scale flow structure keeps the signature of the boundary condition with more vigorous large scales for smaller  ${{\textit {Bi}}}$, even though the global heat transfer and kinetic energy are the same. For all practical purposes, the Robin condition can be safely replaced by a fixed flux when
${{\textit {Bi}}}$, even though the global heat transfer and kinetic energy are the same. For all practical purposes, the Robin condition can be safely replaced by a fixed flux when  ${{\textit {Bi}}} \lesssim 0.03$ and by a fixed temperature for
${{\textit {Bi}}} \lesssim 0.03$ and by a fixed temperature for  ${{\textit {Bi}}}\gtrsim 30$. For turbulent rapidly rotating convection, the thermal boundary condition does not seem to have any impact, once the effective numbers are considered and a reference temperature profile has been chosen.
${{\textit {Bi}}}\gtrsim 30$. For turbulent rapidly rotating convection, the thermal boundary condition does not seem to have any impact, once the effective numbers are considered and a reference temperature profile has been chosen.


