Published online by Cambridge University Press: 06 November 2017
We explore the effect of an applied homogeneous magnetic field on the electrospraying characteristics of a ferrofluid in the cone-jet mode. A sulfolane-based ferrofluid mixed with the ionic liquid ethyl ammonium nitrate has been synthesized. These mixtures have negligible volatility under ambient conditions and remain stable under a very wide range of electrical conductivities 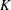 $K$. Magnetized Taylor cones spray with the same current emission characteristics as their non-magnetized counterparts in the shared voltage and flow rate parameter space. However, the magnetized Taylor cones studied remained stable at voltages 23 % lower than the non-magnetized spray; they also access flow rates 30 % and 40 % lower in ferrofluids with
$K$. Magnetized Taylor cones spray with the same current emission characteristics as their non-magnetized counterparts in the shared voltage and flow rate parameter space. However, the magnetized Taylor cones studied remained stable at voltages 23 % lower than the non-magnetized spray; they also access flow rates 30 % and 40 % lower in ferrofluids with  $K=0.3$ and
$K=0.3$ and 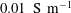 $0.01~\text{S}~\text{m}^{-1}$. In the lower voltage ranges available only to magnetized tips, unusually long stable cones are observed. The magnetic stabilization mechanism responsible for these two effects remains unclear. It is noteworthy that these strong effects arise even when the tip curvature of the strictly magnetized liquid is orders of magnitude smaller than that for the strictly electrified liquid.
$0.01~\text{S}~\text{m}^{-1}$. In the lower voltage ranges available only to magnetized tips, unusually long stable cones are observed. The magnetic stabilization mechanism responsible for these two effects remains unclear. It is noteworthy that these strong effects arise even when the tip curvature of the strictly magnetized liquid is orders of magnitude smaller than that for the strictly electrified liquid.