Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-27T01:43:01.831Z Has data issue: false hasContentIssue false
  • English
  • Français

Educing the source mechanism associated with downstream radiation in subsonic jets

Published online by Cambridge University Press:  31 August 2012

F. Kerhervé ,
P. Jordan ,
A. V. G. Cavalieri ,
J. Delville ,
C. Bogey  and
D. Juvé
F. Kerhervé*
Affiliation:
Institut PPRIME, CNRS UPR 3346, Université de Poitiers, ENSMA 86000, France
P. Jordan
Affiliation:
Institut PPRIME, CNRS UPR 3346, Université de Poitiers, ENSMA 86000, France
A. V. G. Cavalieri
Affiliation:
Institut PPRIME, CNRS UPR 3346, Université de Poitiers, ENSMA 86000, France Divisão de Engenharia Aeronáutica, Instituto Tecnológico de Aeronáutica, 12228-900 São José dos Campos, SP, Brazil
J. Delville
Affiliation:
Institut PPRIME, CNRS UPR 3346, Université de Poitiers, ENSMA 86000, France
C. Bogey
Affiliation:
Laboratoire Mécanique des Fluides et d’Acoustique, CNRS UMR 5509, Ecole Centrale de Lyon 69000, France
D. Juvé
Affiliation:
Laboratoire Mécanique des Fluides et d’Acoustique, CNRS UMR 5509, Ecole Centrale de Lyon 69000, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

This work belongs to the ongoing debate surrounding the mechanism responsible for low-angle sound emission from subsonic jets. The flow, simulated by large eddy simulation (Bogey & Bailly, Comput. Fluids, vol. 35 (10), 2006a, pp. 1344–1358), is a Mach 0.9 jet with Reynolds number, based on the exit diameter, of 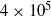. A methodology is implemented to educe, explore and model the flow motions associated with low-angle sound radiation. The eduction procedure, which is based on frequency–wavenumber filtering of the sound field and subsequent conditional analysis of the turbulent jet, provides access to space- and time-dependent (hydrodynamic) pressure and velocity fields. Analysis of these shows the low-angle sound emission to be underpinned by dynamics comprising space and time modulation of axially coherent wavepackets: temporally localized energization of wavepackets is observed to be correlated with the generation of high-amplitude acoustic bursts. Quantitative validation is provided by means of a simplified line-source Ansatz (Cavalieri et al. J. Sound Vib., vol. 330, 2011b, pp. 4474–4492). The dynamic nature of the educed field is then assessed using linear stability theory (LST). The educed pressure and velocity fields are found to compare well with LST: the radial structures of these match the corresponding LST eigenfunctions; the axial evolutions of their fluctuation energy are consistent with the LST amplification rates; and the relative amplitudes of the pressure and velocity fluctuations, which are educed independently of one another, are consistent with LST.

JFM classification

Acoustics: Aeroacoustics Acoustics: Jet noise
Type
Papers
Information
Journal of Fluid Mechanics , Volume 710 , 10 November 2012 , pp. 606 - 640
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Adrian, R. J. 1977 On the role of conditional averages in turbulent theory. In Turbulence in Liquids: Proceedings of the Fourth Biennial Symposium on Turbulence in Liquids (ed. Patterson, G. & Zakin, J. ), pp. 322–332. Science Press.Google Scholar
2. Adrian, R. J. 1978 Structural information obtained from analysis using conditional vector events: a potential tool for the study of coherent structures. In Coherent Structures of Turbulent Boundary Layers, vol. 22 (ed. Smith, C. R. & Abbot, D. E. ). pp. 2065–2070.Google Scholar
3. Adrian, R. J. 1996 Stochastic estimation of the structure of turbulent fields. In Courses and Lectures-International Centre for Mechanical Sciences, pp. 145–196. Springer.Google Scholar
4. Bailly, C., Bogey, C. & Marsden, O. 2010 Progress in direct noise computation. Intl J. Aeroacoust. 9 (1–2), 123–143.CrossRefGoogle Scholar
5. Bodony, D. J. & Lele, S. K. 2008 Low-frequency sound sources in high-speed turbulent jets. J. Fluid Mech. 617 (1), 231–253.CrossRefGoogle Scholar
6. Bogey, C. & Bailly, C. 2006a Computation of a high Reynolds number jet and its radiated noise using large eddy simulation based on explicit filtering. Comput. Fluids 35 (10), 1344–1358.CrossRefGoogle Scholar
7. Bogey, C. & Bailly, C. 2006b Investigation of downstream and sideline subsonic jet noise using large eddy simulations. Theor. Comput. Fluid Dyn. 20 (1), 23–40.CrossRefGoogle Scholar
8. Bogey, C. & Bailly, C. 2006c Large eddy simulations of round jets using explicit filtering with/without dynamic Smagorinsky model. Intl J. Heat Fluid Flow 27, 603–610.CrossRefGoogle Scholar
9. Bogey, C. & Bailly, C. 2006d Large eddy simulations of transitional round jets: influence of the Reynolds number on flow development and energy dissipation. Phys. Fluids 18, 1–14.Google Scholar
10. Bogey, C. & Bailly, C. 2007 An analysis of the correlations between the turbulent flow and the sound pressure fields of subsonic jets. J. Fluid Mech. 583, 71–97.CrossRefGoogle Scholar
11. Broze, G. & Hussain, F. 1994 Nonlinear dynamics of forced transitional jets: periodic and chaotic attractors. J. Fluid Mech. 263 (1), 93–132.CrossRefGoogle Scholar
12. Cavalieri, A. V. G., Daviller, G., Comte, P., Jordan, P., Tadmor, G. & Gervais, Y. 2011a Using large eddy simulation to explore sound-source mechanisms in jets. J. Sound Vib. 330, 4098–4113.Google Scholar
13. Cavalieri, A. V. G., Jordan, P., Agarwal, A. & Gervais, Y. 2011b Jittering wave-packet models for subsonic jet noise. J. Sound Vib. 330, 4474–4492.CrossRefGoogle Scholar
14. Cavalieri, A. V. G., Jordan, P., Gervais, Y., Wei, M. & Freund, J. B. 2010 Intermittent sound generation and its control in a free-shear flow. Phys. Fluids 22.CrossRefGoogle Scholar
15. Cavalieri, A. V. G., Jordan, P., Colonius, T. & Gervais, Y. 2012a Axisymmetric superdirectivity in subsonic jets. J. Fluid Mech. 704, 388–420.CrossRefGoogle Scholar
16. Cavalieri, A. V. G., Rodriguez, D., Jordan, P., Colonius, T. & Gervais, Y. 2012bWavepackets in the velocity field of turbulent jets. In 18th AIAA/CEAS Aeroacoustics Conference, Paper 2011-2743.Google Scholar
17. Chandrsuda, C., Mehta, R. D., Weir, A. D. & Bradshaw, P. 1978 Effect of free-stream turbulence on large structure in turbulent mixing layers. J. Fluid Mech. 85 (4), 603–704.CrossRefGoogle Scholar
18. Christensen, K. T. & Adrian, R. J. 2001 Statistical evidence of hairpin vortex packets in wall turbulence. J. Fluid Mech. 431, 433–443.Google Scholar
19. Cordier, L., Abou El Majd, B. & Favier, J. 2010 Calibration of POD reduced-order models using Tikhonov regularization. Intl J. Numer. Meth. Fluids 63 (2), 269–296.CrossRefGoogle Scholar
20. Crighton, D. G. & Gaster, M. 1976 Stability of slowly diverging jet flow. J. Fluid Mech. 77 (2), 387–413.CrossRefGoogle Scholar
21. Crighton, D. G. & Huerre, P. 1990 Shear-layer pressure fluctuations and superdirective acoustic sources. J. Fluid Mech. 220 (1), 355–368.CrossRefGoogle Scholar
22. Crow, S. C. & Champagne, F. H. 1971 Orderly structures in jet turbulence. J. Fluid Mech. 48 (3), 547–591.Google Scholar
23. Delville, J., Ukeyley, L., Cordier, L., Bonnet, J. P. & Glauser, M. 1999 Examination of large-scale structures in a turbulent plane mixing layer. Part 1. Proper orthogonal decomposition. J. Fluid Mech. 391, 91–122.CrossRefGoogle Scholar
24. Dimotakis, P. E. & Brown, G. L. 1976 The mixing layer at high Reynolds number: large-structure dynamics and entrainment. J. Fluid Mech. 78 (3), 535–560.CrossRefGoogle Scholar
25. Druault, P., Yu, M. & Sagaut, P. 2010 Quadratic stochastic estimation of far-field acoustic pressure with coherent structure events in a 2D compressible plane mixing layer. Intl J. Numer. Meth. Fluids 62 (8), 906–926.CrossRefGoogle Scholar
26. Farge, M. 1992 Wavelet transforms and their applications to turbulence. Annu. Rev. Fluid Mech. 24 (1), 395–458.CrossRefGoogle Scholar
27. Ffowcs Williams, J. E. & Kempton, A. J. 1978 The noise from the large-scale structure of a jet. J. Fluid Mech. 84, 673–694.CrossRefGoogle Scholar
28. Freund, J. B. 2003 Noise-source turbulence statistics and the noise from a Mach 0.9 jet. Phys. Fluids 15, 1788.Google Scholar
29. Freund, J. B. & Colonius, T. 2009 Turbulence and source-field POD analysis of a turbulent jet. Intl J. Aeroacoust. 8 (4), 337–354.CrossRefGoogle Scholar
30. Fuchs, H. V. & Michel, U. 1978 Experimental evidence of turbulent source coherence affecting jet noise. AIAA J. 16, 871–872.CrossRefGoogle Scholar
31. Gudmundsson, K. & Colonius, T. 2009 Parabolized stability equation models for turbulent jets and their radiated sound. In 15th AIAA/CEAS Aeroacoustics Conference, Paper 2009-3380.Google Scholar
32. Gudmundsson, K. & Colonius, T. 2011 Instability wave models for the near-field fluctuations of turbulent jets. J. Fluid Mech. 689 (1), 97–128.Google Scholar
33. Guezennec, Y. G. 1989 Stochastic estimation of coherent structure in turbulent boundary layers. Phys. Fluids A 1 (1), 1054–1060.CrossRefGoogle Scholar
34. Hudy, L. M. & Naguib, A. 2007 Stochastic estimation of a separated-flow field using wall-pressure-array measurements. Phy. Fluids 19.CrossRefGoogle Scholar
35. Hussain, A. K. M. F. 1983 Coherent structures: reality and myth. Phys. Fluids 26 (10), 2816–2850.CrossRefGoogle Scholar
36. Hussain, A. K. M. F. & Zaman, K. B. M. Q 1981 The ‘preferred mode’ of the axisymmetric jet. J. Fluid Mech. 110, 39–71.CrossRefGoogle Scholar
37. Jordan, P. & Colonius, T. 2013 Wavepackets and turbulent jet noise. Annu. Rev. Fluid Mech. 45.CrossRefGoogle Scholar
38. Jordan, P. & Gervais, Y. 2008 Subsonic jet aeroacoustics: associating experiment, modelling and simulation. Exp. Fluids 44 (1), 1–21.CrossRefGoogle Scholar
39. Jordan, P., Schlegel, M., Stalnov, O., Noack, B. R. & Tinney, C. E. 2007 Identifying noisy and quiet modes in a jet. In 13th AIAA/CEAS Aeroacoustics Conference, Paper 2007-3602.Google Scholar
40. Jordan, P. & Tinney, C. E. 2008 The near-field pressure of co-axial subsonic jets. J. Fluid Mech. 611, 175–204.Google Scholar
41. Jordan, P., Tinney, C. E., Delville, J., Coiffet, F., Glauser, M. & Hall, A. 2005 Low-dimensional signatures of the sound production mechanism in subsonic jets: towards identification and control. In 35th AIAA Fluid Dynamics Conference, Paper 2005-4647.Google Scholar
42. Juvé, D., Sunyach, M. & Comte-Bellot, G. 1980 Intermittency of the noise emission in subsonic cold jets. J. Sound Vib. 71 (3), 319–332.Google Scholar
43. Koenig, M., Cavalieri, A. V. G., Jordan, P., Delville, J., Gervais, Y. & Papamoschou, D. 2011 Farfield filtering of subsonic jet noise: Mach and temperature effects. In 17th AIAA/CEAS Aeroacoustics Conference, Paper 2011-2926.Google Scholar
44. Lau, J. C., Fisher, M. J. & Fuchs, H. V. 1972 The intrinsic structure of turbulent jets. J. Sound Vib. 22 (4), 379–406.Google Scholar
45. Mankbadi, R. & Liu, J. T. C. 1984 Sound generated aerodynamically revisited: large-scale structures in a turbulent jet as a source of sound. Phil. Trans. R. Soc. Lond. A 311 (1516), 183–217.Google Scholar
46. Michalke, A. 1984 Survey on jet instability theory. Prog. Aeronaut. Sci. 21, 159–199.Google Scholar
47. Michalke, A. & Fuchs, H. V. 1975 On turbulence and noise of an axisymmetric shear flow. J. Fluid Mech. 70 (1), 179–205.CrossRefGoogle Scholar
48. Michalke, A. & Hermann, G. 1982 On the inviscid instability of a circular jet with external flow. J. Fluid Mech. 114 (1), 343–359.CrossRefGoogle Scholar
49. Mollo-Christensen, E. 1963 Measurements of near-field pressure of subsonic jets. Tech. rep. Advisory Group for Aeronautical Research and Development, Paris (France).Google Scholar
50. Mollo-Christensen, E. 1967 Jet noise and shear flow instability seen from an experimenter’s viewpoint. Trans. ASME: J. Appl. Mech. 34, 1.CrossRefGoogle Scholar
51. Moore, C. J. 1977 The role of shear-layer instability waves in jet exhaust noise. J. Fluid Mech. 80 (2), 321–367.Google Scholar
52. Morris, P. J. 2009 A note on noise generation by large-scale turbulent structures in subsonic and supersonic jets. Intl J. Aeroacoust. 8 (4), 301–315.CrossRefGoogle Scholar
53. Murray, L. & Ukeiley, N. 2005 Velocity and surface pressure measurements in an open cavity. Exp. Fluids J. 38 (5), 656–671.Google Scholar
54. Murray, L. & Ukeiley, N. 2007 Modified quadratic stochastic estimation of resonating subsonic cavity flow. J. Turbul. 8 (53), 1–23.CrossRefGoogle Scholar
55. Olsen, M. G. & Dutton, J. C. 2002 Stochastic estimation of large structures in an incompressible mixing layer. AIAA J. 40, 2431–2438.CrossRefGoogle Scholar
56. Papamoschou, D. 2008 Imaging of directional distributed noise sources. In 14th AIAA/CEAS Aeroacoustics Conference and Exhibit, Paper 2008-2885.Google Scholar
57. Papamoschou, D. 2011Wavepacket modelling of the jet noise source. In 17th AIAA/CEAS Aeroacoustics Conference, Paper 2011-2835.Google Scholar
58. Picard, C. & Delville, J. 2000 Pressure velocity coupling in a subsonic round jet. Heat Fluid Flow 21, 359–364.CrossRefGoogle Scholar
59. Reba, R., Narayanan, S. & Colonius, T. 2010 Wave-packet models for large-scale mixing noise. Intl J. Aeroacoust. 9 (4), 533–558.Google Scholar
60. Reba, R., Simonich, J. & Schlinker, T. 2008 Measurement of source wave-packets in high-speed jets and connection to far-field. In 14th AIAA/CEAS Aeroacoustics Conference, Paper 2008-2091.Google Scholar
61. Sandham, N. D., Morfey, C. L. & Hu, Z. W. 2006 Sound radiation from exponentially growing and decaying surface waves. J. Sound Vib. 294 (1–2), 355–361.Google Scholar
62. Sandham, N. D. & Salgado, A. M. 2008 Nonlinear interaction model of subsonic jet noise. Phil. Trans. R. Soc. A 366 (1876), 2745–2760.CrossRefGoogle ScholarPubMed
63. Schlegel, M., Noack, B. R., Jordan, P., Dillmann, A., Groschel, E., Schroder, W., Wei, M., Freund, J. B., Lehmann, O. & Tadmor, G. 2012 On least-order flow representations for flow aerodynamics and acoustics. J. Fluid Mech. 697, 367–398.CrossRefGoogle Scholar
64. Sinayoko, S., Agarwal, A. & Hu, Z. 2011 Flow decomposition and aerodynamic sound generation. J. Fluid Mech. 668, 335–350.CrossRefGoogle Scholar
65. Sirovich, L. 1987 Turbulence and the dynamics of coherent structures. Parts 1–3. Q. Appl. Math. 45, 561–590.CrossRefGoogle Scholar
66. Stanislas, M., Perret, L. & Foucault, J. M. 2008 Vortical structures in the turbulent boundary layer: a possible route to universal representation. J. Fluid Mech. 602, 327–382.Google Scholar
67. Suzuki, T. & Colonius, T. 2006 Instability waves in a subsonic jet detected using a near-field phased microphone array. J. Fluid Mech. 565, 197–226.CrossRefGoogle Scholar
68. Tadmor, G., Bissex, D., Noack, B., Morzynski, M., Colonius, T. & Taira, K. 2008 Temporal-harmonic specific POD mode extraction. In 4th AIAA Flow Control Conference, Paper 4190.Google Scholar
69. Tam, C. K. W. & Burton, D. E. 1984a Sound generated by instability waves of supersonic flows. Part 1. Two-dimensional mixing layers. J. Fluid Mech. 138, 249–272.CrossRefGoogle Scholar
70. Tam, C. K. W. & Burton, D. E. 1984b Sound generated by instability waves of supersonic flows. Part 2. Axisymmetric jets. J. Fluid Mech. 138 (1), 273–295.Google Scholar
71. Tam, C. K. W. & Morris, P. J. 1980 The radiation of sound by the instability waves of a compressible plane turbulent shear layer. J. Fluid Mech. 98 (2), 349–381.Google Scholar
72. Tinney, C. E., Glauser, M. N. & Ukeiley, L. 2005 The evolution of the most energetic modes in high subsonic Mach number turbulent jets. In 43rd AIAA Aerospace Science, Paper 2005-0417.Google Scholar
73. Tinney, C. E. & Jordan, P. 2008 The near-field pressure surrounding co-axial subsonic jets. J. Fluid Mech. 611, 175–204.Google Scholar
74. Tinney, C. E., Jordan, P., Hall, A., Delville, J. & Glauser, M. N. 2006 A study in the near pressure field of co-axial subsonic jets. In 12th AIAA/CEAS Aeroacoustics Conference, Paper 2006-2589.Google Scholar
75. Tinney, C. E., Jordan, P., Hall, A. M., Delville, J. & Glauser, M. N. 2007 A time-resolved estimate of the turbulence and sound source mechanisms in a subsonic jet flow. J. Turbul. 8 (7), 1–20.Google Scholar
76. Torrence, C. & Compo, G. 1998 A practical guide to wavelet analysis. Bull. Am. Meteorol. Soc. 79 (1), 61–78.Google Scholar
77. Tung, T. C. & Adrian, R. J. 1980 Higher-order estimates of conditional eddies in isotropic turbulence. Phys. Fluids 23, 1469–1470.Google Scholar
78. Ukeiley, L., Cordier, L., Manceau, R., Delville, J., Glauser, M. & Bonnet, J. P. 2001 Examination of large-scale structures in a turbulent plane mixing layer. Part 2. Dynamical systems model. J. Fluid Mech. 441.Google Scholar
79. Yule, A. J. 1978 Large-scale structure in the mixing layer of a round jet. J. Fluid Mech. 89, 413–432.CrossRefGoogle Scholar
80. Zhou, J., Adrian, R. J., Balachandar, S. & Kendall, T. M. 1999 Mechanisms for generating coherent packets of hairpin vortices in channel flow. J. Fluid Mech. 387, 353–396.Google Scholar