Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-23T17:07:54.791Z Has data issue: false hasContentIssue false
  • English
  • Français

Dynamics of revolving wings for various aspect ratios

Published online by Cambridge University Press:  12 May 2014

D. J. Garmann  and
M. R. Visbal
D. J. Garmann*
Affiliation:
Air Force Research Laboratory, Wright–Patterson AFB, OH 45433, USA
M. R. Visbal
Affiliation:
Air Force Research Laboratory, Wright–Patterson AFB, OH 45433, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

High-fidelity, direct numerical simulations (DNSs) are conducted to examine the vortex structure and aerodynamic loading of unidirectionally revolving wings in quiescent fluid. Wings with aspect ratios 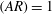$({\mathit{AR}}) = 1$, 2 and 4 are considered at a fixed root-based Reynolds number of 1000. Each wing is shown to generate a coherent leading-edge vortex (LEV) that remains in close proximity to the surface and provides persistent suction throughout the motion. Towards the tip, the LEV lifts off as an arch-like structure and reorients itself along the chord through its connection with the tip vortex. The substantial and sustained aerodynamic loads achieved during the motion saturate with aspect ratio resulting from the chordwise growth of the LEV along the span eventually becoming geometrically constrained by the trailing edge. Further, for 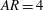${\mathit{AR}}=4$, substructures develop in the feeding sheet of the LEV, which appear to directly correlate with the local, span-based Reynolds number achieved during rotation. The lower-aspect-ratio wings do not have sufficient spans for these transitional elements to manifest. In contrast, vortex breakdown, which occurs around midspan for each aspect ratio, shows a strong dependence on the spanwise pressure gradient established between the root and tip of the wing and not local Reynolds number. This independent development of shear-layer substructures and vortex breakdown parallels very closely with what has been observed in delta wing flow. Next, the centrifugal, Coriolis and pressure gradient forces are also analysed at several spanwise locations across each wing, and the centrifugal and pressure gradient forces are shown to be responsible for the spanwise flow above the wing. The Coriolis force is directed away from the surface at the base of the LEV, indicating that it is not a contributor to LEV attachment, which is contrary to previous hypotheses. Finally, as a means of emphasizing the importance of the centrifugal force on LEV attachment, the 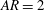${\mathit{AR}}=2$ wing is simulated with the addition of a source term in the governing equations to oppose and eliminate the centrifugal force near the surface. The initial formation and development of the LEV is unhindered by the absence of this force; however, later in the motion, the outboard lift-off of the LEV moves inboard. Without the opposing outboard-directed centrifugal force to keep the separation past midspan, the entire vortex eventually separates and moves away from the surface.

JFM classification

Low-Reynolds-number flows: Low-Reynolds-number flows Vortex Flows: Vortex breakdown Vortex Flows: Vortex Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 748 , 10 June 2014 , pp. 932 - 956
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alpert, P. 1981 Implicit filtering in conjunction with explicit filtering. J. Comput. Phys. 44 (1), 212–219.CrossRefGoogle Scholar
Ansari, S., Phillips, N., Stabler, G., Wilkins, P. C., Żbikowski, R. & Knowles, K. 2009 Experimental investigation of some aspects of insect-like flapping flight aerodynamics for application to micro air vehicles. Exp. Fluids 46, 777–798.CrossRefGoogle Scholar
Beam, R. & Warming, R. 1978 An implicit factored scheme for the compressible Navier–Stokes equations. AIAA J. 16 (4), 393–402.Google Scholar
Birch, J. & Dickinson, M. 2003 The influence of wing-wake interactions on the production of aerodynamic forces in flapping flight. J. Expl Biol. 206, 2257–2272.CrossRefGoogle ScholarPubMed
Birch, J., Dickson, W. & Dickinson, M. 2004 Force production and flow structure of the leading edge vortex on flapping wings at high and low Reynolds numbers. J. Expl Biol. 207, 1063–1072.CrossRefGoogle Scholar
Carr, Z. R., Chen, C. & Ringuette, M. J. 2013 Finite-span rotating wings: three-dimensional vortex formation and variations with aspect ratio. Exp. Fluids 54 (2), 1–26.Google Scholar
Cheng, B., Sane, S. P., Barbera, G., Troolin, D. R., Strand, T. & Deng, X. 2013 Three-dimensional flow visualization and vorticity dynamics in revolving wings. Exp. Fluids 54, 1423.Google Scholar
Dickinson, M. 1994 The effects of wing rotation on unsteady aerodynamic performance at low Reynolds numbers. J. Expl Biol. 192, 179–206.CrossRefGoogle ScholarPubMed
Eldredge, J., Wang, C. & Ol, M.2009 A computational study of a canonical pitch-up, pitch-down, wing maneuver. AIAA Paper 2009-3687. AIAA.Google Scholar
Ellington, C. 1999 The novel aerodynamics of insect flight: applications to micro-air vehicles. J. Expl Biol. 202, 3439–3448.Google Scholar
Ellington, C., van den Berg, C., Willmott, A. P. & Thomas, A. L. R. 1996 Leading-edge vortices in insect flight. Nature 384, 626–630.CrossRefGoogle Scholar
Gaitonde, D. & Visbal, M.1998 High-order schemes for Navier–Stokes equations: algorithm and implementation into FDL3DI. Technical Report AFRL-VA-WP-TR-1998-3060. Air Force Research Laboratory, Wright-Patterson AFB.Google Scholar
Gaitonde, D. & Visbal, M.1999 Further development of a Navier–Stokes solution procedure based on higher-order formulas. AIAA Paper 99-0557. AIAA.CrossRefGoogle Scholar
Garmann, D., Visbal, M. & Orkwis, P. 2013a Comparative study of implicit and subgrid-scale model large-eddy simulation techniques for low-Reynolds number airfoil applications. Intl J. Numer. Meth. Fluids 71 (12), 1546–1565.Google Scholar
Garmann, D., Visbal, M. & Orkwis, P. 2013b Three-dimensional flow structure and aerodynamic loading on a revolving wing. Phys. Fluids 25, 034101.Google Scholar
Gordnier, R. E. & Visbal, M. R.1993 Numerical simulation of delta-wing roll. AIAA Paper 1993-0554. AIAA.Google Scholar
Harbig, R. R., Sheridan, J. & Thompson, M. C. 2013 Reynolds number and aspect ratio effects on the leading-edge vortex for rotating insect wing planforms. J. Fluid Mech. 717, 166–192.CrossRefGoogle Scholar
Jameson, A., Schmidt, W. & Turkel, E.1981 Numerical solutions of the Euler equations by finite volume methods using Runge–Kutta time stepping schemes. AIAA Paper 1981-1259. AIAA.Google Scholar
Kim, D. & Gharib, M. 2010 Experimental study of three-dimensional vortex structures in translating and rotating plates. Exp. Fluids 49, 329–339.CrossRefGoogle Scholar
Lehmann, F. 2004 The mechanisms of lift enhancement in insect flight. Naturwissenschaften 91, 101–122.Google Scholar
Lehmann, F. & Pick, S. 2007 The aerodynamic benefit of wing–wing interaction depends on stroke trajectory in flapping insect wings. J. Expl Biol. 210, 1362–1377.Google Scholar
Lele, S. 1992 Compact finite difference schemes with spectral-like resolution. J. Comput. Phys. 103 (1), 16–42.Google Scholar
Lentink, D. & Dickinson, M. H. 2009a Biofluiddynamic scaling of flapping, spinning and translating fins and wings. J. Expl Biol. 212, 2691–2704.Google Scholar
Lentink, D. & Dickinson, M. 2009b Rotational accelerations stabilize leading edge vortices on revolving fly wings. J. Expl Biol. 212, 2705–2719.Google Scholar
Mao, S. & Jianghao, W. 2004 Large aerodynamic forces on a sweeping wing at low Reynolds number. Acta Mechanica Sin. 20 (1), 24–31.Google Scholar
Matthew, J., Lechner, R., Foysi, H., Sesterhenn, J. & Friedrich, R. 2003 An explicit filtering method for LES of compressible flows. Phys. Fluids 15 (8), 2279–2289.Google Scholar
Maxworthy, T. 2007 The formation and maintenance of a leading-edge vortex during the forward motion of an animal wing. J. Fluid Mech. 587, 471–475.Google Scholar
Ozen, C. & Rockwell, D. 2012a Flow structure on a rotating plate. Exp. Fluids 52 (1), 207–223.Google Scholar
Ozen, C. A. & Rockwell, D. 2012b Three-dimensional flow structure on a rotating wing. J. Fluid Mech. 707, 541–550.CrossRefGoogle Scholar
Poelma, C., Dickson, W. & Dickinson, M. 2006 Time-resolved reconstruction of the full velocity field around a dynamically-scaled flapping wing. Exp. Fluids 41, 213–225.CrossRefGoogle Scholar
Pulliam, T. 1986 Artificial dissipation models for the Euler equations. AIAA J. 24 (12), 1931–1940.Google Scholar
Pulliam, T. & Chaussee, D. 1981 A diagonal form of an implicit approximate-factorization algorithm. J. Comput. Phys. 17 (10), 347–363.Google Scholar
Rizzetta, D. P., Visbal, M. R. & Morgan, P. E.2008 A high-order compact finite-difference scheme for large-eddy simulation of active flow control (invited). AIAA Paper 2008-526. AIAA.CrossRefGoogle Scholar
Sane, S. 2003 The aerodynamics of insect flight. J. Expl Biol. 206 (23), 4191–4208.CrossRefGoogle ScholarPubMed
Shyy, W., Aono, H., Kang, C. & Liu, H. 2013 An Introduction to Flapping Wing Aerodynamics. Cambridge University Press.Google Scholar
Shyy, W., Lian, Y., Tang, J., Viieru, D. & Liu, Hao. 2008 Aerodynamics of Low Reynolds Number Flyers. Cambridge University Press.Google Scholar
Stolz, S. & Adams, N. 1999 An approximate deconvolution procedure for large-eddy simulation. Phys. Fluids 11 (7), 1699–1701.CrossRefGoogle Scholar
Tannehill, J. C., Anderson, D. A. & Pletcher, R. H. 1997 Computational Fluid Mechanics and Heat Transfer, 2nd edn Series in Computational and Physical Processes in Mechanics and Thermal Sciences. Taylor & Francis.Google Scholar
Usherwood, J. R. & Ellington, C. 2002a The aerodynamics of revolving wings: I. Model hawkmoth wings. J. Expl Biogr. 205, 1547–1564.CrossRefGoogle ScholarPubMed
Usherwood, J. R. & Ellington, C. 2002b The aerodynamics of revolving wings: II. Propeller force coefficients from mayfly to quail. J. Expl Biogr. 205, 1565–1576.CrossRefGoogle ScholarPubMed
Visbal, M. 1994 Onset of vortex breakdown above a pitching delta wing. AIAA J. 32 (8), 1568–1575.CrossRefGoogle Scholar
Visbal, M. R. & Gaitonde, D. V. 1999 High-order accurate methods for complex unsteady subsonic flows. AIAA J. 37 (10), 1231–1239.Google Scholar
Visbal, M. R. & Gaitonde, D. V. 2002 On the use of high-order finite-difference schemes on curvilinear and deforming meshes. J. Comput. Phys. 181, 155–185.Google Scholar
Visbal, M. & Gordnier, R.2003 On the structure of the shear layer emanating from a swept leading edge at angle of attack. AIAA Paper 2003-4016. AIAA.Google Scholar
Visbal, M. R., Morgan, P. E. & Rizzetta, D. P.2003 An implicit LES approach based on high-order compact differencing and filtering schemes. AIAA Paper 2003-4098. AIAA.CrossRefGoogle Scholar
Visbal, M. R. & Rizzetta, D. P. 2002 Large-eddy simulation on curvilinear grids using compact differencing and filtering schemes. Trans. ASME J. Fluids Engng 124, 836–847.Google Scholar