Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Wang, Qiqi
Hu, Rui
and
Blonigan, Patrick
2014.
Least Squares Shadowing sensitivity analysis of chaotic limit cycle oscillations.
Journal of Computational Physics,
Vol. 267,
Issue. ,
p.
210.
Larsson, Johan
and
Wang, Qiqi
2014.
The prospect of using large eddy and detached eddy simulations in engineering design, and the research required to get there.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 372,
Issue. 2022,
p.
20130329.
Meliga, Philippe
Boujo, Edouard
Pujals, Gregory
and
Gallaire, François
2014.
Sensitivity of aerodynamic forces in laminar and turbulent flow past a square cylinder.
Physics of Fluids,
Vol. 26,
Issue. 10,
Wei, Mingjun
and
Xu, Min
2014.
A continuous adjoint-based approach for the optimization of wing flapping.
Hoffman, Johan
Jansson, Johan
Jansson, Niclas
and
De Abreu, Rodrigo Vilela
2015.
Towards a parameter-free method for high Reynolds number turbulent flow simulation based on adaptive finite element approximation.
Computer Methods in Applied Mechanics and Engineering,
Vol. 288,
Issue. ,
p.
60.
Mao, X.
2015.
Sensitivity of forces to wall transpiration in flow past an aerofoil.
Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 471,
Issue. 2184,
p.
20150618.
Talnikar, Chaitanya
Blonigan, Patrick J.
Bodart, Julien
and
Wang, Qiqi
2015.
Optimization with LES -- algorithms for dealing with sampling error of turbulence statistics.
Xu, Min
and
Wei, Mingjun
2016.
Using adjoint-based optimization to study kinematics and deformation of flapping wings.
Journal of Fluid Mechanics,
Vol. 799,
Issue. ,
p.
56.
Alexeev, Alexey Kirillovich
and
Bondarev, Alexander Evgenyevich
2016.
On important information for inverse problems and its visualization.
Keldysh Institute Preprints,
p.
1.
Önder, Asim
and
Meyers, Johan
2016.
Optimal control of a transitional jet using a continuous adjoint method.
Computers & Fluids,
Vol. 126,
Issue. ,
p.
12.
Meliga, Philippe
2017.
Computing the sensitivity of drag and lift in flow past a circular cylinder: Time-stepping versus self-consistent analysis.
Physical Review Fluids,
Vol. 2,
Issue. 7,
Talnikar, Chaitanya
Wang, Qiqi
and
Laskowski, Gregory M.
2017.
Unsteady Adjoint of Pressure Loss for a Fundamental Transonic Turbine Vane.
Journal of Turbomachinery,
Vol. 139,
Issue. 3,
Yi, Junsok
and
Capone, Luigi
2017.
Adjoint-Based Sensitivity Analysis for Unsteady Bladerow Interaction Using Space–Time Gradient Method.
Journal of Turbomachinery,
Vol. 139,
Issue. 11,
Günther, Stefanie
Gauger, Nicolas R.
and
Wang, Qiqi
2017.
A framework for simultaneous aerodynamic design optimization in the presence of chaos.
Journal of Computational Physics,
Vol. 328,
Issue. ,
p.
387.
Hoffman, Johan
Jansson, Johan
Jansson, Niclas
De Abreu, Rodrigo Vilela
and
Johnson, Claes
2017.
Encyclopedia of Computational Mechanics Second Edition.
p.
1.
Koehler, Christopher
Durscher, Ryan
Beran, Philip
and
Bhagat, Nitin
2018.
Adjoint-enhanced flow visualization.
Journal of Visualization,
Vol. 21,
Issue. 5,
p.
819.
Meliga, P.
Boujo, E.
Meldi, M.
and
Gallaire, F.
2018.
Revisiting the drag reduction problem using adjoint-based distributed forcing of laminar and turbulent flows over a circular cylinder.
European Journal of Mechanics - B/Fluids,
Vol. 72,
Issue. ,
p.
123.
Warnakulasuriya, Suneth
Andre, Michael
Wüchner, Roland
and
Bletzinger, Kai‐Uwe
2018.
Stabilization of a Time‐Dependent Discrete Adjoint Solver for Chaotic Incompressible Flows.
PAMM,
Vol. 18,
Issue. 1,
Mao, Xuerui
and
Pearson, Emily
2018.
Drag reduction and thrust generation by tangential surface motion in flow past a cylinder.
Theoretical and Computational Fluid Dynamics,
Vol. 32,
Issue. 3,
p.
307.
Ashley, Anthony S.
Crean, Jared
and
Hicken, Jason E.
2019.
Towards Aerodynamic Shape Optimization of Unsteady Turbulent Flows.
 ${\mathit{Re}}_{D} = 20, 100$ and
${\mathit{Re}}_{D} = 20, 100$ and 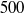 $500$. The quantity of interest in the adjoint formulation is the drag on the cylinder. We use classical fluid mechanics approaches to analyse the adjoint solution, which is a vector field similar to a flow field. Production and dissipation of kinetic energy of the adjoint field is discussed. We also derive the evolution of circulation of the adjoint field along a closed material contour. These analytical results are used to explain three numerical solutions of the adjoint equations presented in this paper. The adjoint solution at
$500$. The quantity of interest in the adjoint formulation is the drag on the cylinder. We use classical fluid mechanics approaches to analyse the adjoint solution, which is a vector field similar to a flow field. Production and dissipation of kinetic energy of the adjoint field is discussed. We also derive the evolution of circulation of the adjoint field along a closed material contour. These analytical results are used to explain three numerical solutions of the adjoint equations presented in this paper. The adjoint solution at 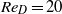 ${\mathit{Re}}_{D} = 20$, a viscous steady state flow, exhibits a downstream suction and an upstream jet, the opposite of the expected behaviour of a flow field. The adjoint solution at
${\mathit{Re}}_{D} = 20$, a viscous steady state flow, exhibits a downstream suction and an upstream jet, the opposite of the expected behaviour of a flow field. The adjoint solution at 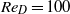 ${\mathit{Re}}_{D} = 100$, a periodic two-dimensional unsteady flow, exhibits periodic, bean-shaped circulation in the near-wake region. The adjoint solution at
${\mathit{Re}}_{D} = 100$, a periodic two-dimensional unsteady flow, exhibits periodic, bean-shaped circulation in the near-wake region. The adjoint solution at 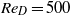 ${\mathit{Re}}_{D} = 500$, a turbulent three-dimensional unsteady flow, has complex dynamics created by the shear layer in the near wake. The magnitude of the adjoint solution increases exponentially at the rate of the first Lyapunov exponent. These numerical results correlate well with the theoretical analysis presented in this paper.
${\mathit{Re}}_{D} = 500$, a turbulent three-dimensional unsteady flow, has complex dynamics created by the shear layer in the near wake. The magnitude of the adjoint solution increases exponentially at the rate of the first Lyapunov exponent. These numerical results correlate well with the theoretical analysis presented in this paper.

