Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-22T08:22:02.709Z Has data issue: false hasContentIssue false
  • English
  • Français

Drag and diffusion coefficients of a spherical particle attached to a fluid–fluid interface

Published online by Cambridge University Press:  10 February 2016

Aaron Dörr ,
Steffen Hardt ,
Hassan Masoud  and
Howard A. Stone
Aaron Dörr*
Affiliation:
Institute for Nano- and Microfluidics, Center of Smart Interfaces, Technische Universität Darmstadt, Alarich-Weiss-Straße 10, 64287 Darmstadt, Germany
Steffen Hardt
Affiliation:
Institute for Nano- and Microfluidics, Center of Smart Interfaces, Technische Universität Darmstadt, Alarich-Weiss-Straße 10, 64287 Darmstadt, Germany
Hassan Masoud
Affiliation:
Department of Mechanical Engineering, University of Nevada, Reno, NV 89557, USA Department of Mechanical and Aerospace Engineering, Princeton University, Princeton, NJ 08544, USA
Howard A. Stone
Affiliation:
Department of Mechanical and Aerospace Engineering, Princeton University, Princeton, NJ 08544, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Explicit analytical expressions for the drag and diffusion coefficients of a spherical particle attached to the flat interface between two immiscible fluids are constructed for the case of a vanishing viscosity ratio between the fluid phases. The model is designed to account explicitly for the dependence on the contact angle between the two fluids and the solid surface. 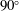The Lorentz reciprocal theorem is applied in the context of geometric perturbations from the limiting cases of $90^{\circ }$ and 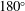$180^{\circ }$ contact angles. The model agrees well with the experimental and numerical data from the literature. Also, an advantage of the method utilized is that the drag and diffusion coefficients can be calculated up to one order higher in the perturbation parameter than the known velocity and pressure fields. Extensions to other particle shapes with known velocity and pressure fields are straightforward.

JFM classification

Low-Reynolds-number flows: Low-Reynolds-number flows Multiphase and Particle-laden Flows: Particle/fluid flow
Type
Papers
Information
Journal of Fluid Mechanics , Volume 790 , 10 March 2016 , pp. 607 - 618
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ally, J. & Amirfazli, A. 2010 Magnetophoretic measurement of the drag force on partially immersed microparticles at air–liquid interfaces. Colloids Surf. A 360, 120–128.CrossRefGoogle Scholar
Bławzdziewicz, J., Ekiel-Jeżewska, M. L. & Wajnryb, E. 2010 Motion of a spherical particle near a planar fluid–fluid interface: the effect of surface incompressibility. J. Chem. Phys. 133, 114702.Google Scholar
Brenner, H. 1964 The Stokes resistance of a slightly deformed sphere. Chem. Engng Sci. 19, 519–539.Google Scholar
Brenner, H. & Leal, L. G. 1978 A micromechanical derivation of Fick’s law for interfacial diffusion of surfactant molecules. J. Colloid Interface Sci. 65 (2), 191–209.Google Scholar
Chen, W. & Tong, P. 2008 Short-time self-diffusion of weakly charged silica spheres at aqueous interfaces. Eur. Phys. Lett. 84, 28003.Google Scholar
Cichocki, B., Ekiel-Jeewska, M. L., Nägele, G. & Wajnryb, E. 2004 Motion of spheres along a fluid–gas interface. J. Chem. Phys. 121, 2305–2316.Google Scholar
Danov, K., Aust, R., Durst, F. & Lange, U. 1995 Influence of the surface viscosity on the hydrodynamic resistance and surface diffusivity of a large Brownian particle. J. Colloid Interface Sci. 175, 36–45.Google Scholar
Danov, K. D., Gurkov, T. D., Raszillier, H. & Durst, F. 1998 Stokes flow caused by the motion of a rigid sphere close to a viscous interface. Chem. Engng Sci. 53 (19), 3413–3434.CrossRefGoogle Scholar
Davis, A. M. J. 1990 Stokes drag on a disk sedimenting toward a plane or with other disks; additional effects of a side wall or free surface. Phys. Fluids A 2, 301–312.CrossRefGoogle Scholar
Dörr, A. & Hardt, S. 2015 Driven particles at fluid interfaces acting as capillary dipoles. J. Fluid Mech. 770, 5–26.Google Scholar
Du, K., Liddle, J. A. & Berglund, A. J. 2012 Three-dimensional real-time tracking of nanoparticles at an oil–water interface. Langmuir 28, 9181–9188.CrossRefGoogle ScholarPubMed
Fischer, T. M., Dhar, P. & Heinig, P. 2006 The viscous drag of spheres and filaments moving in membranes or monolayers. J. Fluid Mech. 558, 451–475.Google Scholar
Fulford, G. R. & Blake, J. R. 1986 Force distribution along a slender body straddling an interface. J. Austral. Math. Soc. B 27, 295–315.Google Scholar
Happel, J. & Brenner, H. 1983 Low Reynolds Number Hydrodynamics. Martinus Nijhoff.Google Scholar
Jeffrey, D. J. & Onishi, Y. 1984 Calculation of the resistance and mobility functions for two unequal rigid spheres in low-Reynolds-number flow. J. Fluid Mech. 139, 261–290.Google Scholar
Larmour, I. A., Saunders, G. C. & Bell, S. E. J. 2008 Sheets of large superhydrophobic metal particles self assembled on water by the cheerios effect. Angew. Chem. Intl Ed. Engl. 47, 5043–5045.CrossRefGoogle ScholarPubMed
Leal, L. G. 1980 Particle motions in a viscous fluid. Annu Rev. Fluid Mech. 12, 435–476.CrossRefGoogle Scholar
Lorentz, H. A. 1896 Eene algemeene stelling omtrent de beweging eerier vloeistof met wrijving en eenige daamit afgeleide gevolgen. Zittingsverslag van de Koninklijke Akademie van Wetenschappen te Amsterdam 5, 168–175 (translation into English: J. Engng Maths 30, 1996, 19–24).Google Scholar
Masoud, H. & Stone, H. A. 2014 A reciprocal theorem for Marangoni propulsion. J. Fluid Mech. 741 R4, 1–7.Google Scholar
O’Neill, M. E., Ranger, K. B. & Brenner, H. 1986 Slip at the surface of a translating-rotating sphere bisected by a free surface bounding a semiinfinite viscous fluid: removal of the contactline singularity. Phys. Fluids 29, 913–924.Google Scholar
Peng, Y., Chen, W., Fischer, Th. M., Weitz, D. A. & Tong, P. 2009 Short-time self-diffusion of nearly hard spheres at an oil–water interface. J. Fluid Mech. 618, 243–261.CrossRefGoogle Scholar
Petkov, J. T., Denkov, N. D., Danov, K. D., Velev, O. D., Aust, R. & Durst, F. 1995 Measurement of the drag coefficient of spherical particles attached to fluid interfaces. J. Colloid Interface Sci. 172, 147–154.Google Scholar
Pozrikidis, C. 2007 Particle motion near and inside an interface. J. Fluid Mech. 575, 333–357.Google Scholar
Radoev, B., Nedjalkov, M. & Djakovich, V. 1992 Brownian motion at liquid–gas interfaces. 1. Diffusion coefficients of macroparticles at pure interfaces. Langmuir 8, 2962–2965.Google Scholar
Ranger, K. B. 1978 The circular disk straddling the interface of a two-phase flow. Intl J. Multiphase Flow 4, 263–277.Google Scholar
Schönecker, C. & Hardt, S. 2014 Electro-osmotic flow along superhydrophobic surfaces with embedded electrodes. Phys. Rev. E 89, 063005.Google Scholar
Sriram, I., Walder, R. & Schwartz, D. K. 2012 Stokes–Einstein and desorption-mediated diffusion of protein molecules at the oil–water interface. Soft Matt. 8, 6000–6003.Google Scholar
Stone, H. A. & Masoud, H. 2015 Mobility of membrane-trapped particles. J. Fluid Mech. 781, 494–505.CrossRefGoogle Scholar
Stone, H. A. & Samuel, A. D. T. 1996 Propulsion of microorganisms by surface distortions. Phys. Rev. Lett. 77 (19), 4102–4104.Google Scholar
Tanzosh, J. P. & Stone, H. A. 1996 A general approach for analyzing the arbitrary motion of a circular disk in a stokes flow. Chem. Engng Commun. 148–150, 333–346.CrossRefGoogle Scholar
Walder, R. B., Honciuc, A. & Schwartz, D. K. 2010 Phospholipid diffusion at the oil–water interface. J. Phys. Chem. B 114, 11484–11488.Google Scholar
Wang, D., Yordanov, S., Paroor, H. M., Mukhopadhyay, A., Li, C. Y., Butt, H.-J. & Koynov, K. 2011 Probing diffusion of single nanoparticles at water-oil interfaces. Small 7 (24), 3502–3507.Google Scholar
Zabarankin, M. 2007 Asymmetric three-dimensional Stokes flow about two fused equal spheres. Proc. R. Soc. Lond. A 463, 2329–2349.Google Scholar
Zhang, L., Wu, J., Wang, Y., Long, Y., Zhao, N. & Xu, J. 2012 Combination of bioinspiration: a general route to superhydrophobic particles. J. Am. Chem. Soc. 134, 9879–9881.Google Scholar
Supplementary material: File

Dörr supplementary material

Dörr supplementary material 1

Download Dörr supplementary material(File)
File 210.9 KB