Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Volk, Andreas
Rossi, Massimiliano
Kähler, Christian J.
Hilgenfeldt, Sascha
and
Marin, Alvaro
2015.
Growth control of sessile microbubbles in PDMS devices.
Lab on a Chip,
Vol. 15,
Issue. 24,
p.
4607.
Dietrich, Erik
Wildeman, Sander
Visser, Claas Willem
Hofhuis, Kevin
Kooij, E. Stefan
Zandvliet, Harold J. W.
and
Lohse, Detlef
2016.
Role of natural convection in the dissolution of sessile droplets.
Journal of Fluid Mechanics,
Vol. 794,
Issue. ,
p.
45.
Peñas-López, Pablo
Parrales, Miguel A.
Rodríguez-Rodríguez, Javier
and
van der Meer, Devaraj
2016.
The history effect in bubble growth and dissolution. Part 1. Theory.
Journal of Fluid Mechanics,
Vol. 800,
Issue. ,
p.
180.
Peñas-López, Pablo
van Elburg, Benjamin
Parrales, Miguel A.
and
Rodríguez-Rodríguez, Javier
2017.
Diffusion of dissolved
CO2
in water propagating from a cylindrical bubble in a horizontal Hele-Shaw cell.
Physical Review Fluids,
Vol. 2,
Issue. 6,
Domingues, Eddy M.
Arunachalam, Sankara
and
Mishra, Himanshu
2017.
Doubly Reentrant Cavities Prevent Catastrophic Wetting Transitions on Intrinsically Wetting Surfaces.
ACS Applied Materials & Interfaces,
Vol. 9,
Issue. 25,
p.
21532.
Lv, Pengyu
Le The, Hai
Eijkel, Jan
Van den Berg, Albert
Zhang, Xuehua
and
Lohse, Detlef
2017.
Growth and Detachment of Oxygen Bubbles Induced by Gold-Catalyzed Decomposition of Hydrogen Peroxide.
The Journal of Physical Chemistry C,
Vol. 121,
Issue. 38,
p.
20769.
Yamashita, Tatsuya
and
Ando, Keita
2017.
Aeration of water with oxygen microbubbles and its purging effect.
Journal of Fluid Mechanics,
Vol. 825,
Issue. ,
p.
16.
Peñas-López, Pablo
Moreno Soto, Álvaro
Parrales, Miguel A.
van der Meer, Devaraj
Lohse, Detlef
and
Rodríguez-Rodríguez, Javier
2017.
The history effect on bubble growth and dissolution. Part 2. Experiments and simulations of a spherical bubble attached to a horizontal flat plate.
Journal of Fluid Mechanics,
Vol. 820,
Issue. ,
p.
479.
Lv, Pengyu
Xiang, Yaolei
Xue, Yahui
Lin, Hao
and
Duan, Huiling
2017.
Morphological bubble evolution induced by air diffusion on submerged hydrophobic structures.
Physics of Fluids,
Vol. 29,
Issue. 3,
Michelin, Sébastien
Guérin, Etienne
and
Lauga, Eric
2018.
Collective dissolution of microbubbles.
Physical Review Fluids,
Vol. 3,
Issue. 4,
Hwang, Gi Byoung
Page, Kristopher
Patir, Adnan
Nair, Sean P.
Allan, Elaine
and
Parkin, Ivan P.
2018.
The Anti-Biofouling Properties of Superhydrophobic Surfaces are Short-Lived.
ACS Nano,
Vol. 12,
Issue. 6,
p.
6050.
Fernández-Pérez, A.
Hyuk Yoo, T. Sang
Fernández-Luna, J.L
Moreno, F.
García-Caurel, E.
and
Saiz, J.M.
2020.
Polarimetric analysis of a fused sphere as a model for adherent particles.
Optik,
Vol. 207,
Issue. ,
p.
164371.
Shim, Suin
Khodaparast, Sepideh
Lai, Ching-Yao
Yan, Jing
Ault, Jesse T.
Rallabandi, Bhargav
Shardt, Orest
and
Stone, Howard A.
2021.
CO2-Driven diffusiophoresis for maintaining a bacteria-free surface.
Soft Matter,
Vol. 17,
Issue. 9,
p.
2568.
Rivero-Rodríguez, Javier
Pérez-Saborid, Miguel
and
Scheid, Benoit
2021.
An alternative choice of the boundary condition for the arbitrary Lagrangian-Eulerian method.
Journal of Computational Physics,
Vol. 443,
Issue. ,
p.
110494.
Joewondo, Nerine
Garbin, Valeria
and
Pini, Ronny
2022.
Nonuniform Collective Dissolution of Bubbles in Regular Pore Networks.
Transport in Porous Media,
Vol. 141,
Issue. 3,
p.
649.
Gao, Dan
Yang, Jiaxi
Qi, Youwei
Guo, Chang
and
Zhang, Heng
2023.
Review and Perspectives on CO2 Bubble Dynamic Characteristics in Different Liquids during Carbon Capture, Utilization, and Storage Process.
Energy & Fuels,
Vol. 37,
Issue. 1,
p.
58.
Joewondo, Nerine
Garbin, Valeria
and
Pini, Ronny
2023.
Experimental evidence of the effect of solute concentration on the collective evolution of bubbles in a regular pore-network.
Chemical Engineering Research and Design,
Vol. 192,
Issue. ,
p.
82.
Arunachalam, Sankara
and
Mishra, Himanshu
2024.
Collective wetting transitions of submerged gas‐entrapping microtextured surfaces.
Droplet,
Vol. 3,
Issue. 3,
Shi, Hongbo
Zhang, Hang
Geng, Linlin
Qu, Sen
Wang, Xikun
and
Nikrityuk, Petr A.
2024.
Dynamic behaviors of cavitation bubbles near biomimetic surfaces: A numerical study.
Ocean Engineering,
Vol. 292,
Issue. ,
p.
116628.
Xu, Ning
Fu, Xin
Su, Rui
Xu, Wen-Ping
and
Chen, Wen-Yu
2024.
Dissolution process of CO2 bubble adhered to a flat plate in a flow fluid.
AIP Advances,
Vol. 14,
Issue. 6,
 $\text{CO}_{2}$ spherical cap bubble adhered to a flat surface in air-saturated water
$\text{CO}_{2}$ spherical cap bubble adhered to a flat surface in air-saturated water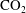 $\text{CO}_{2}$ bubbles (with initial radii
$\text{CO}_{2}$ bubbles (with initial radii 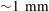 ${\sim}1~\text{mm}$) immersed in air-saturated water adhered to two substrates with different levels of hydrophobicity. It is found that the contact angle dynamics plays an important role in the bubble dissolution rate. A dissolution model for a multicomponent SCB in an isothermal and uniform pressure environment is then devised. The model is based on the quasi-stationary approximation. It includes the effect of the contact angle dynamics, whose behaviour is predicted by means of a simplified model based on the results obtained from adhesion hysteresis. The presence of an impermeable substrate hinders the overall rate of mass transfer. Two approaches are considered in its determination: (a) the inclusion of a diffusion boundary layer–plate interaction model and (b) a finite-difference solution. The model solutions are compared with the experimental results, yielding fairly good agreement.
${\sim}1~\text{mm}$) immersed in air-saturated water adhered to two substrates with different levels of hydrophobicity. It is found that the contact angle dynamics plays an important role in the bubble dissolution rate. A dissolution model for a multicomponent SCB in an isothermal and uniform pressure environment is then devised. The model is based on the quasi-stationary approximation. It includes the effect of the contact angle dynamics, whose behaviour is predicted by means of a simplified model based on the results obtained from adhesion hysteresis. The presence of an impermeable substrate hinders the overall rate of mass transfer. Two approaches are considered in its determination: (a) the inclusion of a diffusion boundary layer–plate interaction model and (b) a finite-difference solution. The model solutions are compared with the experimental results, yielding fairly good agreement.


