Published online by Cambridge University Press: 28 August 2013
A significant amount of research effort has been made to understand the boundary layer instability and the generation and evolution of turbulence subject to periodic/oscillatory flows. However, little is known about bottom boundary layers driven by highly transient and intermittent free-stream flow forcing, such as solitary wave motion. To better understand the nature of the instability mechanisms and turbulent flow characteristics subject to solitary wave motion, a large number of direct numerical simulations are conducted. Different amplitudes of random initial fluctuating velocity field are imposed. Two different instability mechanisms are observed within the range of Reynolds number studied. The first is a short-lived, nonlinear, long-wave instability which is observed during the acceleration phase, and the second is a broadband instability that occurs during the deceleration phase. Transition from a laminar to turbulent state is observed to follow two different breakdown pathways: the first follows the sequence of 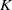 $K$-type secondary instability of a near-wall boundary layer at comparatively lower Reynolds number and the second one follows a breakdown path similar to that of free shear layers. Overall characteristics of the flow are categorized into four regimes as: (i) laminar; (ii) disturbed laminar; (iii) transitional; and (iv) turbulent. Our categorization into four regimes is consistent with earlier works. However, this study is able to provide more specific definitions through the instability characteristics and the turbulence breakdown process.
$K$-type secondary instability of a near-wall boundary layer at comparatively lower Reynolds number and the second one follows a breakdown path similar to that of free shear layers. Overall characteristics of the flow are categorized into four regimes as: (i) laminar; (ii) disturbed laminar; (iii) transitional; and (iv) turbulent. Our categorization into four regimes is consistent with earlier works. However, this study is able to provide more specific definitions through the instability characteristics and the turbulence breakdown process.