Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Lluesma-Rodríguez, Federico
Álcantara-Ávila, Francisco
Pérez-Quiles, María Jezabel
and
Hoyas, Sergio
2021.
A Code for Simulating Heat Transfer in Turbulent Channel Flow.
Mathematics,
Vol. 9,
Issue. 7,
p.
756.
Tang, S.L.
and
Antonia, R.A.
2022.
Scaling of small-scale wall turbulence.
Journal of Fluid Mechanics,
Vol. 948,
Issue. ,
Zhang, Peng
Song, Yubin
Liu, Yilang
and
Xia, Zhenhua
2022.
Equivalence of three thermal boundary conditions in compressible turbulent channel flows.
Physical Review E,
Vol. 105,
Issue. 6,
Kawata, T.
and
Tsukahara, T.
2022.
Spectral analysis on dissimilarity between turbulent momentum and heat transfers in plane Couette turbulence.
Physics of Fluids,
Vol. 34,
Issue. 7,
Hoyas, Sergio
Oberlack, Martin
Alcántara-Ávila, Francisco
Kraheberger, Stefanie V.
and
Laux, Jonathan
2022.
Wall turbulence at high friction Reynolds numbers.
Physical Review Fluids,
Vol. 7,
Issue. 1,
Modesti, Davide
and
Pirozzoli, Sergio
2022.
Direct numerical simulation of forced thermal convection in square ducts up to .
Journal of Fluid Mechanics,
Vol. 941,
Issue. ,
Zonta, Francesco
Hadi Sichani, Pejman
and
Soldati, Alfredo
2022.
Interaction between thermal stratification and turbulence in channel flow.
Journal of Fluid Mechanics,
Vol. 945,
Issue. ,
Yu, Linqi
Yousif, Mustafa Z.
Zhang, Meng
Hoyas, Sergio
Vinuesa, Ricardo
and
Lim, Hee-Chang
2022.
Three-dimensional ESRGAN for super-resolution reconstruction of turbulent flows with tricubic interpolation-based transfer learning.
Physics of Fluids,
Vol. 34,
Issue. 12,
Kawata, Takuya
and
Tsukahara, Takahiro
2022.
Spectral Analysis on Transport Budgets of Turbulent Heat Fluxes in Plane Couette Turbulence.
Energies,
Vol. 15,
Issue. 14,
p.
5258.
Pirozzoli, Sergio
Romero, Joshua
Fatica, Massimiliano
Verzicco, Roberto
and
Orlandi, Paolo
2022.
DNS of passive scalars in turbulent pipe flow.
Journal of Fluid Mechanics,
Vol. 940,
Issue. ,
Chen, Peng E.S.
Huang, George P.
Shi, Yipeng
Yang, Xiang I.A.
and
Lv, Yu
2022.
A unified temperature transformation for high-Mach-number flows above adiabatic and isothermal walls.
Journal of Fluid Mechanics,
Vol. 951,
Issue. ,
Klein, Marten
Schmidt, Heiko
and
Lignell, David O.
2022.
Stochastic modeling of surface scalar-flux fluctuations in turbulent channel flow using one-dimensional turbulence.
International Journal of Heat and Fluid Flow,
Vol. 93,
Issue. ,
p.
108889.
Schmekel, D.
Alcántara-Ávila, F.
Hoyas, S.
and
Vinuesa, R.
2022.
Predicting Coherent Turbulent Structures via Deep Learning.
Frontiers in Physics,
Vol. 10,
Issue. ,
Zhong, Kevin
Hutchins, Nicholas
and
Chung, Daniel
2023.
Heat-transfer scaling at moderate Prandtl numbers in the fully rough regime.
Journal of Fluid Mechanics,
Vol. 959,
Issue. ,
2023.
Effect of wall temperature in streamwise supersonic corner flow.
Physics of Fluids,
Vol. 35,
Issue. 6,
Pirozzoli, Sergio
and
Modesti, Davide
2023.
Direct numerical simulation of one-sided forced thermal convection in plane channels.
Journal of Fluid Mechanics,
Vol. 957,
Issue. ,
Pirozzoli, Sergio
2023.
Prandtl number effects on passive scalars in turbulent pipe flow.
Journal of Fluid Mechanics,
Vol. 965,
Issue. ,
Vigdorovich, Igor
2023.
Supersonic turbulent boundary layer on a plate. II. Flow in the wall region and the Crocco integral.
Physics of Fluids,
Vol. 35,
Issue. 11,
Balasubramanian, Arivazhagan G.
Guastoni, Luca
Schlatter, Philipp
and
Vinuesa, Ricardo
2023.
Direct numerical simulation of a zero-pressure-gradient turbulent boundary layer with passive scalars up to Prandtl number Pr = 6.
Journal of Fluid Mechanics,
Vol. 974,
Issue. ,
Yousif, Mustafa Z.
Yu, Linqi
Hoyas, Sergio
Vinuesa, Ricardo
and
Lim, HeeChang
2023.
A deep-learning approach for reconstructing 3D turbulent flows from 2D observation data.
Scientific Reports,
Vol. 13,
Issue. 1,
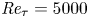 ${\textit {Re}}_\tau =5000$ and
${\textit {Re}}_\tau =5000$ and 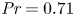 ${\textit {Pr}} = 0.71$
${\textit {Pr}} = 0.71$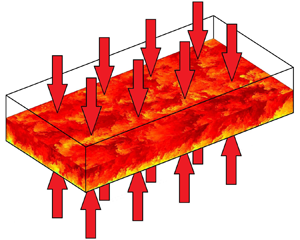
 $5000$ and the Prandtl number of air,
$5000$ and the Prandtl number of air,  $0.71$. The mixed boundary condition has been used as the boundary condition of the thermal field. The computational domain has been set to
$0.71$. The mixed boundary condition has been used as the boundary condition of the thermal field. The computational domain has been set to  $3.2 {\rm \pi}h$,
$3.2 {\rm \pi}h$,  $2h$ and
$2h$ and  $1.6 {\rm \pi}h$ in the
$1.6 {\rm \pi}h$ in the  $x$,
$x$,  $y$ and
$y$ and  $z$ directions, respectively. This domain is large enough to accurately compute the statistics of the flow. Mean values and intensities of the temperature have been obtained. Derived parameters from the average thermal field, such as the von Kármán constant and the Nusselt number have been calculated. An asymptotic behaviour of the von Kármán constant is observed when
$z$ directions, respectively. This domain is large enough to accurately compute the statistics of the flow. Mean values and intensities of the temperature have been obtained. Derived parameters from the average thermal field, such as the von Kármán constant and the Nusselt number have been calculated. An asymptotic behaviour of the von Kármán constant is observed when  ${\textit {Re}}_\tau$ is increased. A correlation for the Nusselt number is proposed. Also, the turbulent Prandtl number has been calculated and it does not present significant changes when
${\textit {Re}}_\tau$ is increased. A correlation for the Nusselt number is proposed. Also, the turbulent Prandtl number has been calculated and it does not present significant changes when  ${\textit {Re}}_\tau$ is increased. Finally, the turbulent budgets are presented. A relation between the increment of the inner peak of the temperature intensities and the scaling failure of the dissipation and viscous diffusion terms is provided. The statistics of all simulations can be downloaded from the web page of our group: http://personales.upv.es/serhocal/.
${\textit {Re}}_\tau$ is increased. Finally, the turbulent budgets are presented. A relation between the increment of the inner peak of the temperature intensities and the scaling failure of the dissipation and viscous diffusion terms is provided. The statistics of all simulations can be downloaded from the web page of our group: http://personales.upv.es/serhocal/.


