Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-25T23:04:24.807Z Has data issue: false hasContentIssue false
  • English
  • Français

Direct numerical simulation of the turbulent kinetic energy and energy dissipation rate in a cylinder wake

Published online by Cambridge University Press:  02 August 2022

Hongyi Jiang Open the ORCID record for Hongyi Jiang [Opens in a new window] ,
Xiaoyuan Hu ,
Liang Cheng  and
Tongming Zhou
Hongyi Jiang
Affiliation:
School of Engineering, The University of Western Australia, 35 Stirling Highway, Perth, WA 6009, Australia
Xiaoyuan Hu
Affiliation:
School of Marine Science and Engineering, Guangzhou International Campus, South China University of Technology, Guangzhou 511442, PR China
Liang Cheng*
Affiliation:
School of Engineering, The University of Western Australia, 35 Stirling Highway, Perth, WA 6009, Australia School of Marine Science and Engineering, Guangzhou International Campus, South China University of Technology, Guangzhou 511442, PR China
Tongming Zhou
Affiliation:
School of Engineering, The University of Western Australia, 35 Stirling Highway, Perth, WA 6009, Australia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

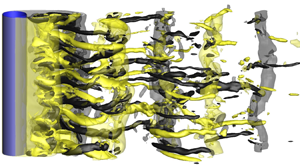
The turbulent kinetic energy and energy dissipation rate in the wake of a circular cylinder are examined at a Reynolds number of 1000. The turbulence characteristics are quantified using direct numerical simulation, which provides a comprehensive dataset that is almost impossible to acquire from physical experiments. The energy dissipation rate is decomposed into the components due to the mean flow, the coherent primary vortices and the remainder. It is found that the remainder component, which develops only in a three-dimensional turbulent wake and resides mainly in the regions of vortices, accounts for 95 % and 97 % of the total dissipation rate for 10 and 20 cylinder diameters downstream of the cylinder, respectively (while the remainder accounts for 62 % and 83 % of the total turbulent kinetic energy). Based on the remainder component, the validity of local isotropy, local axisymmetry, local homogeneity and homogeneity in the y–z plane for the turbulent dissipation in the wake is examined. The analysis reveals that the turbulent dissipation is largely locally homogeneous, but not locally isotropic or axisymmetric, even after the annihilation of the primary vortex street. In addition, the performances of the four corresponding surrogates to the true dissipation rate are evaluated. Owing to the general validity of local homogeneity, the surrogates of local homogeneity and homogeneity in the y–z plane perform well. Although local axisymmetry does not hold, the corresponding surrogate performs well, because errors from different terms largely cancel out. However, the surrogate of local isotropy generally underestimates the true dissipation rate.

JFM classification

Wakes/Jets: Wakes Wakes/Jets: Vortex streets
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 946 , 10 September 2022 , A11
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Antonia, R.A., Djenidi, L. & Danaila, L. 2014 Collapse of the turbulent dissipative range on Kolmogorov scales. Phys. Fluids 26, 045105.CrossRefGoogle Scholar
Antonia, R.A., Zhou, T. & Zhu, Y. 1998 Three-component vorticity measurements in a turbulent grid flow. J. Fluid Mech. 374, 29–57.CrossRefGoogle Scholar
Browne, L.W.B., Antonia, R.A. & Shah, D.A. 1987 Turbulent energy dissipation in a wake. J. Fluid Mech. 179, 307–326.CrossRefGoogle Scholar
Browne, L.W.B., Antonia, R.A. & Shah, D.A. 1989 On the origin of the organised motion in the turbulent far-wake of a cylinder. Exp. Fluids 7, 475–480.CrossRefGoogle Scholar
Cantwell, B. & Coles, D. 1983 An experimental study of entrainment and transport in the turbulent near wake of a circular cylinder. J. Fluid Mech. 136, 321–374.Google Scholar
Cantwell, C.D., et al. 2015 Nektar++: An open-source spectral/hp element framework. Comput. Phys. Commun. 192, 205–219.CrossRefGoogle Scholar
Chassaing, P., 2000 Turbulence en mécanique des fluids.Google Scholar
Chen, J.G., Zhou, Y., Antonia, R.A. & Zhou, T.M. 2018 Characteristics of the turbulent energy dissipation rate in a cylinder wake. J. Fluid Mech. 835, 271–300.CrossRefGoogle Scholar
Chen, J.G., Zhou, Y., Zhou, T.M. & Antonia, R.A. 2016 Three-dimensional vorticity, momentum and heat transport in a turbulent cylinder wake. J. Fluid Mech. 809, 135–167.CrossRefGoogle Scholar
Cimbala, J.M., Nagib, H.M. & Roshko, A. 1988 Large structure in the far wakes of two-dimensional bluff bodies. J. Fluid Mech. 190, 265–298.CrossRefGoogle Scholar
Ducci, A., Konstantinidis, E., Balabani, S. & Yianneskis, M. 2005 Single- and two-point LDA measurements in the turbulent near wake of a circular cylinder. Engineering Turbulence Modelling and Experiments 6: Procedings of the ERCOFTAC International Symposium on Engineering Turbulence Modelling and Measurements; ETMM6, Sardinia, Italy, 451–460.Google Scholar
Elsner, J.W. & Elsner, W. 1996 On the measurement of turbulence energy dissipation. Meas. Sci. Technol. 7, 1334–1348.CrossRefGoogle Scholar
George, W.K. & Hussein, H.J. 1991 Locally axisymmetric turbulence. J. Fluid Mech. 233, 1–23.Google Scholar
Hussain, A.K.M.F. & Hayakawa, M. 1987 Eduction of large-scale organized structures in a turbulent plane wake. J. Fluid Mech. 180, 193–229.CrossRefGoogle Scholar
Jiang, H. & Cheng, L. 2017 Strouhal–Reynolds number relationship for flow past a circular cylinder. J. Fluid Mech. 832, 170–188.Google Scholar
Jiang, H. & Cheng, L. 2019 Transition to the secondary vortex street in the wake of a circular cylinder. J. Fluid Mech. 867, 691–722.CrossRefGoogle Scholar
Jiang, H. & Cheng, L. 2021 Large-eddy simulation of flow past a circular cylinder for Reynolds numbers 400 to 3900. Phys. Fluids 33, 034119.CrossRefGoogle Scholar
Karniadakis, G.E. 1990 Spectral element-Fourier methods for incompressible turbulent flows. Comput. Meth. Appl. Mech. Engng 80, 367–380.CrossRefGoogle Scholar
Karniadakis, G.E., Israeli, M. & Orszag, S.A. 1991 High-order splitting methods for the incompressible Navier-Stokes equations. J. Comput. Phys. 97, 414–443.CrossRefGoogle Scholar
Karniadakis, G.E. & Sherwin, S.J. 2005 Spectral/hp Element Methods for CFD. Oxford University Press.Google Scholar
Kirby, R.M. & Sherwin, S.J. 2006 Stabilisation of spectral/hp element methods through spectral vanishing viscosity: application to fluid mechanics modelling. Comput. Meth. Appl. Mech. Engng 195, 3128–3144.Google Scholar
Lefeuvre, N., Thiesset, F., Djenidi, L. & Antonia, R.A. 2014 Statistics of the turbulent kinetic energy dissipation rate and its surrogates in a square cylinder wake flow. Phys. Fluids 26, 095104.CrossRefGoogle Scholar
Matsumura, M. & Antonia, R.A. 1993 Momentum and heat transport in the turbulent intermediate wake of a circular cylinder. J. Fluid Mech. 250, 651–668.CrossRefGoogle Scholar
Moxey, D., et al. 2020 Nektar++: Enhancing the capability and application of high-fidelity spectral/hp element methods. Comput. Phys. Commun. 249, 107110.CrossRefGoogle Scholar
Noca, F., Park, H. & Gharib, M. 1998 Vortex formation length of a circular cylinder (300 < Re < 4000) using DPIV. Proceedings on Bluff Body Wakes and Vortex-Induced Vibration. ASME Fluids Engineering Division.Google Scholar
Norberg, C. 1994 An experimental investigation of the flow around a circular cylinder: influence of aspect ratio. J. Fluid Mech. 258, 287–316.CrossRefGoogle Scholar
Norberg, C. 2003 Fluctuating lift on a circular cylinder: review and new measurements. J. Fluids Struct. 17, 57–96.CrossRefGoogle Scholar
Pope, S.B. 2000 Turbulent Flows, p. 186. Cambridge University Press.CrossRefGoogle Scholar
Reynolds, W.C. & Hussain, A.K.M.F. 1972 The mechanics of an organized wave in turbulent shear flow. Part 3. Theoretical models and comparisons with experiments. J. Fluid Mech. 54, 263–288.CrossRefGoogle Scholar
Roshko, A. 1954 On the development of turbulent wakes from vortex streets. NACA Tech. Rep. 1191.Google Scholar
Saddoughi, S.G. & Veeravalli, S.V. 1994 Local isotropy in turbulent boundary layers at high Reynolds number. J. Fluid Mech. 268, 333–372.CrossRefGoogle Scholar
Tang, S.L., Antonia, R.A., Djenidi, L. & Zhou, Y. 2016 Complete self-preservation along the axis of a circular cylinder far wake. J. Fluid Mech. 786, 253–274.CrossRefGoogle Scholar
Taylor, G.I. 1935 Statistical theory of turbulence. Proc. R. Soc. A: Math. Phys. Engng Sci. 151, 421–444.Google Scholar
Wieselsberger, C. 1922 New data on the laws of fluid resistance. NACA Tech. Note 84. National Advisory Committee for Aeronautics.Google Scholar
Williamson, C.H.K. 1996 Vortex dynamics in the cylinder wake. Annu. Rev. Fluid Mech. 28, 477–539.Google Scholar
Williamson, C.H.K. & Prasad, A. 1993 A new mechanism for oblique wave resonance in the ‘natural’ far wake. J. Fluid Mech. 256, 269–313.CrossRefGoogle Scholar
Williamson, C.H.K. & Roshko, A. 1990 Measurements of base pressure in the wake of a cylinder at low Reynolds numbers. Z. Flugwiss. Weltraumforsch. 14, 38–46.Google Scholar
Zdravkovich, M.M. 1997 Flow Around Circular Cylinders, Volume 1: Fundamentals. Oxford University Press.Google Scholar
Zhang, H.J., Zhou, Y. & Antonia, R.A. 2000 Longitudinal and spanwise vortical structures in a turbulent near wake. Phys. Fluids 12, 2954–2964.Google Scholar
Zhou, T. & Antonia, R.A. 2000 Reynolds number dependence of the small-scale structure of grid turbulence. J. Fluid Mech. 406, 81–107.CrossRefGoogle Scholar
Zhou, T., Zhou, Y., Yiu, M.W. & Chua, L.P. 2003 Three-dimensional vorticity in a turbulent cylinder wake. Exp. Fluids 35, 459–471.CrossRefGoogle Scholar
Zhou, Y., Zhang, H.J. & Yiu, M.W. 2002 The turbulent wake of two side-by-side circular cylinders. J. Fluid Mech. 458, 303–332.CrossRefGoogle Scholar
Zhu, Y. & Antonia, R.A. 1997 On the correlation between enstrophy and energy dissipation rate in a turbulent wake. Appl. Sci. Res. 57, 337–347.CrossRefGoogle Scholar