Hostname: page-component-745bb68f8f-b6zl4 Total loading time: 0 Render date: 2025-01-09T18:24:00.122Z Has data issue: false hasContentIssue false
  • English
  • Français

A direct numerical simulation analysis of coherent structures in bubble-laden channel flows

Published online by Cambridge University Press:  04 November 2020

Josef Hasslberger Open the ORCID record for Josef Hasslberger [Opens in a new window] ,
Paolo Cifani Open the ORCID record for Paolo Cifani [Opens in a new window] ,
Nilanjan Chakraborty  and
Markus Klein Open the ORCID record for Markus Klein [Opens in a new window]
Josef Hasslberger*
Affiliation:
Institute of Applied Mathematics and Scientific Computing, Bundeswehr University Munich, Werner-Heisenberg-Weg 39, 85577Neubiberg, Germany
Paolo Cifani
Affiliation:
Bernoulli Institute for Mathematics, Computer Science and Artificial Intelligence, University of Groningen, Nijenborgh 9, 9747 AGGroningen, Netherlands
Nilanjan Chakraborty
Affiliation:
School of Engineering, Newcastle University, Claremont Road, Newcastle-Upon-TyneNE1 7RU, UK
Markus Klein
Affiliation:
Institute of Applied Mathematics and Scientific Computing, Bundeswehr University Munich, Werner-Heisenberg-Weg 39, 85577Neubiberg, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

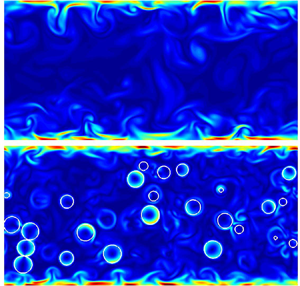
To investigate the coherent structures in turbulent wall-bounded bubbly flows, a local flow topology analysis has been performed in this work. Using the invariants of the velocity-gradient tensor, all possible small-scale flow structures can be categorized into two nodal and two focal topologies for incompressible flows. The analysed direct numerical simulation database of freely moving and deforming bubble swarms is based on a multi-marker geometrical volume-of-fluid method. It appears that bubbles are acting as mixing elements and fragmenting the large topology structures. Since the behaviour in both phases is quite different, key quantities were evaluated in a conditional manner, i.e. separately for the gaseous dispersed phase and the liquid carrier phase. Several similarities with an analytical solution for laminar creeping flows have been observed although the investigated channel flow is turbulent. It has been concluded that the inclusion of bubbles results in a partial shift from focal to nodal flow character whereas the share of stable and unstable flow types remains fairly constant at approximately 50 % in both phases. No clear analogy can be discerned in terms of the global effects of bubble inclusion and wall confinement on flow topologies. At least with respect to flow topologies, regions in the vicinity of the interface show a clearly different behaviour depending on the phase. The size distribution of coherent structures in the bubble-laden channel flow has also been analysed. The measured flow topology size spectrum in the liquid phase can be approximated by a simple model with reasonable accuracy.

JFM classification

Mathematical Foundations: Topological fluid dynamics Multiphase and Particle-laden Flows: Multiphase flow
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 905 , 25 December 2020 , A37
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Blackburn, H. M., Mansour, N. N. & Cantwell, B. J. 1996 Topology of fine-scale motions in turbulent channel flow. J. Fluid Mech. 310, 269–292.CrossRefGoogle Scholar
Chacin, J. M. & Cantwell, B. J. 2000 Dynamics of a low Reynolds number turbulent boundary layer. J. Fluid Mech. 404, 87–115.CrossRefGoogle Scholar
Chakraborty, N, Wacks, D. H., Ketterl, S, Klein, M & Im, H. G. 2019 Scalar dissipation rate transport conditional on flow topologies in different regimes of premixed turbulent combustion. Proc. Combust. Inst. 37 (2), 2353–2361.CrossRefGoogle Scholar
Chong, M. S., Perry, A. E. & Cantwell, B. J. 1990 A general classification of three-dimensional flow fields. Phys. Fluids A 2 (5), 765–777.CrossRefGoogle Scholar
Chong, M. S., Soria, J., Perry, A. E., Chacin, J., Cantwell, B. J. & Na, Y. 1998 Turbulence structures of wall-bounded shear flows found using DNS data. J. Fluid Mech. 357, 225–247.CrossRefGoogle Scholar
Cifani, P. 2017 DNS of turbulent bubble-laden channel flows. PhD thesis, University of Twente.Google Scholar
Cifani, P. 2019 Analysis of a constant-coefficient pressure equation method for fast computations of two-phase flows at high density ratios. J. Comput. Phys. 398, 108904.CrossRefGoogle Scholar
Cifani, P., Kuerten, J. G. M. & Geurts, B. J. 2018 Highly scalable DNS solver for turbulent bubble-laden channel flow. Comput. Fluids 172, 67–83.CrossRefGoogle Scholar
Cifani, P., Kuerten, J. G. M. & Geurts, B. J. 2020 Flow and bubble statistics of turbulent bubble-laden downflow channel. Intl J. Multiphase Flow 126, 103244.CrossRefGoogle Scholar
Cifani, P., Michalek, W. R., Priems, G. J. M., Kuerten, J. G. M., van der Geld, C. W. M. & Geurts, B. J. 2016 A comparison between the surface compression method and an interface reconstruction method for the VOF approach. Comput. Fluids 136, 421–435.CrossRefGoogle Scholar
Dabiri, S., Lu, J. & Tryggvason, G. 2013 Transition between regimes of a vertical channel bubbly upflow due to bubble deformability. Phys. Fluids 25 (10), 102110.CrossRefGoogle Scholar
Dixit, H. N. & Govindarajan, R. 2010 Vortex-induced instabilities and accelerated collapse due to inertial effects of density stratification. J. Fluid Mech. 646, 415–439.CrossRefGoogle Scholar
Dodd, M. S. & Jofre, L. 2019 Small-scale flow topologies in decaying isotropic turbulence laden with finite-size droplets. Phys. Rev. Fluids 4 (6), 064303.CrossRefGoogle Scholar
Elsinga, G. E. & Marusic, I. 2010 Universal aspects of small-scale motions in turbulence. J. Fluid Mech. 662, 514–539.CrossRefGoogle Scholar
Fang, X., Wang, B. C. & Bergstrom, D. J. 2019 Using vortex identifiers to build eddy-viscosity subgrid-scale models for large-eddy simulation. Phys. Rev. Fluids 4 (3), 034606.CrossRefGoogle Scholar
Fulgosi, M., Lakehal, D., Banerjee, S. & De Angelis, V. 2003 Direct numerical simulation of turbulence in a sheared air–water flow with a deformable interface. J. Fluid Mech. 482, 319–345.CrossRefGoogle Scholar
Han, W., Scholtissek, A., Dietzsch, F., Jahanbakhshi, R. & Hasse, C. 2019 Influence of flow topology and scalar structure on flame-tangential diffusion in turbulent non-premixed combustion. Combust. Flame 206, 21–36.CrossRefGoogle Scholar
Hasslberger, J., Ketterl, S., Klein, M. & Chakraborty, N. 2019 a Flow topologies in primary atomization of liquid jets: a direct numerical simulation analysis. J. Fluid Mech. 859, 819–838.CrossRefGoogle Scholar
Hasslberger, J., Klein, M. & Chakraborty, N. 2018 Flow topologies in bubble-induced turbulence: a direct numerical simulation analysis. J. Fluid Mech. 857, 270–290.CrossRefGoogle Scholar
Hasslberger, J., Marten, S. & Klein, M. 2019 b A theoretical investigation of flow topologies in bubble- and droplet-affected flows. Fluids 4 (3), 117.CrossRefGoogle Scholar
Kim, J., Moin, P. & Moser, R. 1987 Turbulence statistics in fully developed channel flow at low Reynolds number. J. Fluid Mech. 177, 133–166.CrossRefGoogle Scholar
Kobayashi, H. 2005 The subgrid-scale models based on coherent structures for rotating homogeneous turbulence and turbulent channel flow. Phys. Fluids 17 (4), 045104.CrossRefGoogle Scholar
Kwakkel, M., Breugem, W. P. & Boersma, B. J. 2013 Extension of a CLSVOF method for droplet-laden flows with a coalescence/breakup model. J. Comput. Phys. 253, 166–188.CrossRefGoogle Scholar
Lakehal, D. 2018 Status and future developments of large-eddy simulation of turbulent multi-fluid flows (LEIS and LESS). Intl J. Multiphase Flow 104, 322–337.CrossRefGoogle Scholar
Lau, Y. M., Deen, N. G. & Kuipers, J. A. M. 2013 Development of an image measurement technique for size distribution in dense bubbly flows. Chem. Engng Sci. 94, 20–29.CrossRefGoogle Scholar
Lu, J. & Tryggvason, G. 2006 Numerical study of turbulent bubbly downflows in a vertical channel. Phys. Fluids 18 (10), 103302.CrossRefGoogle Scholar
Lu, J. & Tryggvason, G. 2007 Effect of bubble size in turbulent bubbly downflow in a vertical channel. Chem. Engng Sci. 62 (11), 3008–3018.CrossRefGoogle Scholar
Lu, J. & Tryggvason, G. 2008 Effect of bubble deformability in turbulent bubbly upflow in a vertical channel. Phys. Fluids 20 (4), 040701.CrossRefGoogle Scholar
Lu, J. & Tryggvason, G. 2013 Dynamics of nearly spherical bubbles in a turbulent channel upflow. J. Fluid Mech. 732, 166–189.CrossRefGoogle Scholar
Mercado, J. M., Gomez, D. C., Van Gils, D., Sun, C. & Lohse, D. 2010 On bubble clustering and energy spectra in pseudo-turbulence. J. Fluid Mech. 650, 287–306.CrossRefGoogle Scholar
Moser, R. D., Kim, J. & Mansour, N. N. 1999 Direct numerical simulation of turbulent channel flow up to ${Re}_\tau =590$. Phys. Fluids 11 (4), 943–945.CrossRefGoogle Scholar
Ooi, A., Martin, J., Soria, J. & Chong, M. S. 1999 A study of the evolution and characteristics of the invariants of the velocity-gradient tensor in isotropic turbulence. J. Fluid Mech. 381, 141–174.CrossRefGoogle Scholar
Perry, A. E. & Chong, M. S. 1987 A description of eddying motions and flow patterns using critical-point concepts. Annu. Rev. Fluid Mech. 19 (1), 125–155.CrossRefGoogle Scholar
Popinet, S. 2009 An accurate adaptive solver for surface-tension-driven interfacial flows. J. Comput. Phys. 228 (16), 5838–5866.CrossRefGoogle Scholar
Sadhal, S. S., Ayyaswamy, P. S. & Chung, J. N. 2012 Transport Phenomena with Drops and Bubbles. Springer Science and Business Media.Google Scholar
Santarelli, C. & Fröhlich, J. 2015 Direct numerical simulations of spherical bubbles in vertical turbulent channel flow. Intl J. Multiphase Flow 75, 174–193.CrossRefGoogle Scholar
Santarelli, C. & Fröhlich, J. 2016 Direct numerical simulations of spherical bubbles in vertical turbulent channel flow. Influence of bubble size and bidispersity. Intl J. Multiphase Flow 81, 27–45.CrossRefGoogle Scholar
Satapathy, R. & Smith, W. 1961 The motion of single immiscible drops through a liquid. J. Fluid Mech. 10 (4), 561–570.CrossRefGoogle Scholar
Trias, F. X., Folch, D., Gorobets, A. & Oliva, A. 2015 Building proper invariants for eddy-viscosity subgrid-scale models. Phys. Fluids 27 (6), 065103.CrossRefGoogle Scholar
Tripathi, M. K., Sahu, K. C. & Govindarajan, R. 2014 Why a falling drop does not in general behave like a rising bubble. Nat. Sci. Rep. 4, 4771.CrossRefGoogle Scholar
Tryggvason, G., Dabiri, S., Aboulhasanzadeh, B. & Lu, J. 2013 Multiscale considerations in direct numerical simulations of multiphase flows. Phys. Fluids 25 (3), 031302.CrossRefGoogle Scholar
Uhlmann, M. 2008 Interface-resolved direct numerical simulation of vertical particulate channel flow in the turbulent regime. Phys. Fluids 20 (5), 053305.CrossRefGoogle Scholar
Vreman, A. W. & Kuerten, J. G. M. 2014 Comparison of direct numerical simulation databases of turbulent channel flow at $Re_{\tau }=180$. Phys. Fluids 26 (1), 015102.CrossRefGoogle Scholar
Wacks, D. H., Chakraborty, N., Klein, M., Arias, P. G. & Im, H. G. 2016 Flow topologies in different regimes of premixed turbulent combustion: a direct numerical simulation analysis. Phys. Rev. Fluids 1 (8), 083401.CrossRefGoogle Scholar