Published online by Cambridge University Press: 05 February 2021
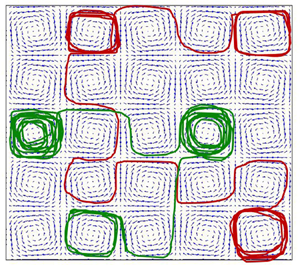
We numerically investigated the phenomenon of non-Gaussian normal diffusion of a Brownian colloidal particle in a periodic array of planar counter-rotating convection rolls. At high Péclet numbers, normal diffusion is observed to occur at all times with non-Gaussian transient statistics. This effect vanishes with increasing the observation time. The displacement distributions decay either slower or faster than a Gaussian function, depending on the flow parameters. The sign of their excess kurtosis is related to the difference between two dynamical time scales, namely, the mean exit time of the particle out of a convection roll and its circulation period inside it.