Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Lawson, J. M.
and
Dawson, J. R.
2013.
The formation of turbulent vortex rings by synthetic jets.
Physics of Fluids,
Vol. 25,
Issue. 10,
Liu, Weiguang
Xu, Ying
Zhang, Tao
and
Qi, Fengfeng
2015.
Experimental optimization for dual support structures cone flow meters based on cone wake flow field characteristics.
Sensors and Actuators A: Physical,
Vol. 232,
Issue. ,
p.
115.
Gao, L.
and
Yu, S. C. M.
2015.
Vortex Rings and Jets.
Vol. 111,
Issue. ,
p.
1.
Ghasemi, A.
Roussinova, V.
Barron, R. M.
and
Balachandar, R.
2016.
Large eddy simulation of the near-field vortex dynamics in starting square jet transitioning into steady state.
Physics of Fluids,
Vol. 28,
Issue. 8,
Gao, Lei
and
Yu, S. C. M.
2016.
Formation of Leading Vortex Pair in Two-Dimensional Starting Jets.
AIAA Journal,
Vol. 54,
Issue. 4,
p.
1364.
Schlueter-Kuck, Kristy
and
Dabiri, John O.
2016.
Pressure evolution in the shear layer of forming vortex rings.
Physical Review Fluids,
Vol. 1,
Issue. 1,
Ghasemi, Abbas
Pereira, Aaron
and
Li, Xianguo
2017.
Large Eddy Simulation of Compressible Subsonic Turbulent Jet Starting From a Smooth Contraction Nozzle.
Flow, Turbulence and Combustion,
Vol. 98,
Issue. 1,
p.
83.
Xiang, Yang
Lin, Haiyan
Zhang, Bin
and
Liu, Hong
2018.
Quantitative analysis of vortex added-mass and impulse generation during vortex ring formation based on elliptic Lagrangian coherent structures.
Experimental Thermal and Fluid Science,
Vol. 94,
Issue. ,
p.
295.
Xiang, Yang
Qin, Suyang
and
Liu, Hong
2018.
Patterns for efficient propulsion during the energy evolution of vortex rings.
European Journal of Mechanics - B/Fluids,
Vol. 71,
Issue. ,
p.
47.
Xu, Yang
Wang, Jin-Jun
Feng, Li-Hao
He, Guo-Sheng
and
Wang, Zhong-Yi
2018.
Laminar vortex rings impinging onto porous walls with a constant porosity.
Journal of Fluid Mechanics,
Vol. 837,
Issue. ,
p.
729.
Xiang, Yang
Liu, Hong
and
Qin, Suyang
2018.
A Unified Energy Feature of Vortex Rings for Identifying the Pinch-Off Mechanism.
Journal of Fluids Engineering,
Vol. 140,
Issue. 1,
Hatoum, Hoda
Moore, Brandon L.
and
Dasi, Lakshmi Prasad
2018.
On the Significance of Systolic Flow Waveform on Aortic Valve Energy Loss.
Annals of Biomedical Engineering,
Vol. 46,
Issue. 12,
p.
2102.
Li, Zhi-Yu
Xu, Yang
and
Wang, Jin-Jun
2020.
Similarity Parameter for Synthetic Jet Vortex Rings Impinging onto Porous Walls.
AIAA Journal,
Vol. 58,
Issue. 2,
p.
722.
Lin, Hai-yan
Xiang, Yang
Qin, Su-yang
Xu, Hui
and
Liu, Hong
2020.
Lagrangian analysis of the fluid transport induced by the interaction of two co-axial co-rotating vortex rings.
Journal of Hydrodynamics,
Vol. 32,
Issue. 6,
p.
1080.
Lin, Haiyan
Xiang, Yang
Xu, Hui
Liu, Hong
and
Zhang, Bin
2020.
Passive scalar mixing induced by the formation of compressible vortex rings.
Acta Mechanica Sinica,
Vol. 36,
Issue. 6,
p.
1258.
Qin, Liang
Xiang, Yang
Lin, Haiyan
and
Liu, Hong
2020.
Formation and dynamics of compressible vortex rings generated by a shock tube.
Experiments in Fluids,
Vol. 61,
Issue. 3,
Limbourg, Raphaël
and
Nedić, Jovan
2021.
Formation of an orifice-generated vortex ring.
Journal of Fluid Mechanics,
Vol. 913,
Issue. ,
Lin, Shujia
Wang, Fuxin
Li, Zhuoqi
and
Xiang, Yang
2021.
Proceedings of the International Conference on Aerospace System Science and Engineering 2020.
Vol. 680,
Issue. ,
p.
153.
Khosronejad, Ali
Kang, Seokkoo
Wermelinger, Fabian
Koumoutsakos, Petros
and
Sotiropoulos, Fotis
2021.
A computational study of expiratory particle transport and vortex dynamics during breathing with and without face masks.
Physics of Fluids,
Vol. 33,
Issue. 6,
Thawko, A.
van Hout, R.
Yadav, H.
and
Tartakovsky, L.
2021.
Flow field characteristics of a confined, underexpanded transient round jet.
Physics of Fluids,
Vol. 33,
Issue. 8,
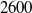 to
to 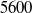 , are conducted so as to investigate the development of the trailing shear layer during the leading vortex ring formation, as well as the corresponding effects on the pinch-off process. Results obtained by digital particle image velocimetry (DPIV) show that secondary vortices start to develop in the trailing jet only after the critical time scale, the ‘formation number’, is achieved. The subsequent growth of the secondary vortices reduces the vorticity flux being fed into the leading vortex ring and, as a consequence, constrains the growth of leading vortex ring with larger circulation. Evolution of perturbation waves into secondary vortices is found to associate with growth and translation of the leading vortex ring during the formation process. A dimensionless parameter ‘
, are conducted so as to investigate the development of the trailing shear layer during the leading vortex ring formation, as well as the corresponding effects on the pinch-off process. Results obtained by digital particle image velocimetry (DPIV) show that secondary vortices start to develop in the trailing jet only after the critical time scale, the ‘formation number’, is achieved. The subsequent growth of the secondary vortices reduces the vorticity flux being fed into the leading vortex ring and, as a consequence, constrains the growth of leading vortex ring with larger circulation. Evolution of perturbation waves into secondary vortices is found to associate with growth and translation of the leading vortex ring during the formation process. A dimensionless parameter ‘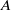 ’, defined as
’, defined as 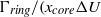 ), is therefore proposed to characterize the effect of the leading vortex ring on suppressing the nonlinear development of instability in the trailing shear layer, i.e. the initial roll-up of the secondary vortices. In a starting jet,
), is therefore proposed to characterize the effect of the leading vortex ring on suppressing the nonlinear development of instability in the trailing shear layer, i.e. the initial roll-up of the secondary vortices. In a starting jet, 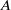 follows a decreasing trend with the formation time
follows a decreasing trend with the formation time 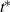 . A critical value
. A critical value  is identified experimentally, which physically coincides with the initiation of the first secondary vortex roll-up and, therefore, indicates the onset of pinch-off process.
is identified experimentally, which physically coincides with the initiation of the first secondary vortex roll-up and, therefore, indicates the onset of pinch-off process.