Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-26T20:16:38.302Z Has data issue: false hasContentIssue false
  • English
  • Français

The decay of a dipolar vortex in a weakly dispersive environment

Published online by Cambridge University Press:  28 April 2021

Edward R. Johnson Open the ORCID record for Edward R. Johnson [Opens in a new window]  and
Matthew N. Crowe Open the ORCID record for Matthew N. Crowe [Opens in a new window]
Edward R. Johnson*
Affiliation:
Department of Mathematics, University College London, LondonWC1E 6BT, UK
Matthew N. Crowe
Affiliation:
Department of Mathematics, University College London, LondonWC1E 6BT, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

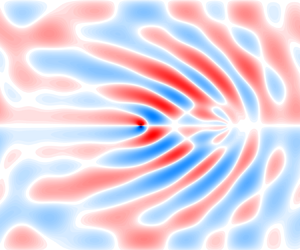
A simple model is presented for the evolution of a dipolar vortex propagating horizontally in a vertical-slice model of a weakly stratified inviscid atmosphere, following the model of Flierl & Haines (Phys. Fluids, vol. 6, 1994, pp. 3487–3497) for a modon on the ${\rm beta}$-plane. The dipole is assumed to evolve to remain within the family of Lamb–Chaplygin dipoles but with varying radius and speed. The dipole loses energy and impulse through internal wave radiation. It is argued, and verified against numerical solutions of the full equations, that an appropriately defined centre vorticity for the dipole is closely conserved throughout the flow evolution. Combining conservation of centre vorticity with the requirement that the dipole energy loss balances the work done on the fluid by internal wave radiation gives a model that captures much of the observed dipole decay. Similar results are noted for a cylindrical dipole propagating along the axis of a rotating fluid when the dipole axis is perpendicular to the axis of rotation and for a spherical vortex propagating horizontally in a weakly stratified fluid. The model extends to fluids of small viscosity and so provides an estimate for the relative importance of wave drag and dissipation in dipole decay.

JFM classification

Geophysical and Geological Flows: Quasi-geostrophic flows Geophysical and Geological Flows: Rotating flows Geophysical and Geological Flows: Stratified flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 917 , 25 June 2021 , A35
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Bretherton, F.P. 1967 The time-dependent motion due to a cylinder moving in an unbounded rotating or stratified fluid. J. Fluid Mech. 28, 545–570.Google Scholar
Brion, V., Sipp, D. & Jacquin, L. 2014 Linear dynamics of the Lamb–Chaplygin dipole in the two-dimensional limit. Phys. Fluids 26, 064103.Google Scholar
Burns, K.J., Vasil, G.M., Oishi, J.S., Lecoanet, D. & Brown, B.P. 2020 Dedalus: a flexible framework for numerical simulations with spectral methods. Phys. Rev. Res. 2, 023068.Google Scholar
Carr, M., Davies, P. & Hoebers, R. 2015 Experiments on the structure and stability of mode-2 internal solitary-like waves propagating on an offset pycnocline. Phys. Fluids 27, 046602.Google Scholar
Couder, Y. & Basdevant, C. 1986 Experimental and numerical study of vortex couples in two-dimensional flows. J. Fluid Mech. 173, 225–251.Google Scholar
Crowe, M.N., Kemp, C.J.D. & Johnson, E.R. 2021 The decay of Hill's vortex in a rotating flow. J. Fluid Mech. (under review).Google Scholar
Delbende, I. & Rossi, M. 2009 The dynamics of a viscous vortex dipole. Phys. Fluids 21, 073605.Google Scholar
Flierl, G.R. 1987 Isolated eddy models in geophysics. Annu. Rev. Fluid Mech. 19, 493–530.Google Scholar
Flierl, G.R. & Haines, K. 1994 The decay of modons due to Rossby wave radiation. Phys. Fluids 6, 3487–3497.Google Scholar
Flierl, G.R., Stern, M.E. & Whitehead, J.A. 1983 The physical significance of modons: laboratory experiments and general integral constraints. Dyn. Atmos. Oceans 7, 233–263.Google Scholar
van de Fliert, B.W. 1996 The viscous modulation of Lamb's dipole vortex. Phys. Fluids 8, 1975–1977.Google Scholar
Flór, J.B. & van Heijst, G.J.F. 1994 An experimental-study of dipolar vortex structures in a stratified fluid. J. Fluid Mech. 279, 101–133.Google Scholar
Flór, J.B., van Heijst, G.J.F. & Delfos, R. 1995 Decay of dipolar vortex structures in a stratified fluid. Phys. Fluids 7, 374–383.Google Scholar
Ford, R., McIntyre, M.E. & Norton, W.A. 2000 Balance and the slow quasimanifold: some explicit results. J. Atmos. Sci. 57, 1236–1254.Google Scholar
van Geffen, J.H.G.M. & van Heijst, G.J.F. 1998 Viscous evolution of 2D dipolar vortices. Fluid Dyn. Res. 22, 191–213.Google Scholar
Gorodtsov, V.A. & Teodorovich, E.V. 1983 Study of internal waves in the case of rapid horizontal motion of cylinders and spheres. Fluid Dyn. 17, 893–898.Google Scholar
Hill, M.J.M. 1894 On a spherical vortex. Phil. Trans. R. Soc. 185, 213–245.Google Scholar
Kamachi, M. & Honji, H. 1982 Steady flow patterns of internal solitary bulges in a stratified fluid. Phys. Fluids 25, 1119–1120.Google Scholar
Lamb, H. 1932 Hydrodynamics, 6th edn. Cambridge University Press.Google Scholar
Larichev, V.D. & Reznik, G.M. 1976 Two-dimensional solitary Rossby waves. Dokl. Akad. Nauk SSSR 231, 12–13.Google Scholar
Long, R.R. 1955 Some aspects of the flow of stratified fluids. III: continuous density gradients. Tellus 7, 341–357.Google Scholar
McWilliams, J.C. & Zabusky, N.J. 1982 Interactions of isolated vortices. 1. Modons colliding with modons. Geophys. Astrophys. Fluid Dyn. 19, 207–227.Google Scholar
Meleshko, V.V. & van Heijst, G.J.F. 1994 On Chaplygin's investigations of two-dimensional vortex structures in an inviscid fluid. J. Fluid Mech. 272, 157–182.Google Scholar
Nguyen Duc, J.-M. & Sommeria, J. 1988 Experimental characterization of steady two-dimensional vortex couples. J. Fluid Mech. 192, 175–192.Google Scholar
Nielsen, A.H. & Rasmussen, J.J 1997 Formation and temporal evolution of the Lamb dipole. Phys. Fluids 9, 982–991.Google Scholar
Nycander, J. & Isichenko, M.B. 1990 Motion of dipole vortices in a weakly inhomogeneous medium and related convective transport. Phys. Fluids 2, 2042–2047.Google Scholar
Salloum, M., Knio, O.M. & Brandt, A. 2012 Numerical simulation of mass transport in internal solitary waves. Phys. Fluids 24, 016602.Google Scholar
Velasco Fuentes, O.U. & van Heijst, G.J.F. 1994 Experimental-study of dipolar vortices on a topographic $\beta$-plane. J. Fluid Mech. 259, 79–106.Google Scholar

Johnson and Crowe supplementary movie 1

See pdf file for movie caption

Download Johnson and Crowe supplementary movie 1(Video)
Video 907.4 KB

Johnson and Crowe supplementary movie 2

See pdf file for movie caption

Download Johnson and Crowe supplementary movie 2(Video)
Video 1 MB
Supplementary material: PDF

Johnson and Crowe supplementary material

Captions for movies 1-2

Download Johnson and Crowe supplementary material(PDF)
PDF 13.1 KB