Published online by Cambridge University Press: 08 July 2013
We analyse the steady-state convection–diffusion mixing of a solute by a creeping circulatory flow in a long sealed rectangular two-dimensional channel with impermeable sidewalls and fixed different solute concentrations at the two opposite edges. Solution circulation is due to a constant velocity slip along the sidewalls and a back flow along the channel axis. This simple model distils the essence of circulation in concentration polarization of an electrolyte solution under a DC electric current in a micro-channel sealed by an ion-selective element (a nano-channel or a cation exchange membrane). It is observed that in the slow circulation regime (small  $Pe$ numbers) the solute flux through the channel is governed by the Taylor–Aris dispersion mechanism, i.e. the flux is driven by the cross-sectional average axial concentration gradient, whereas upon increase in
$Pe$ numbers) the solute flux through the channel is governed by the Taylor–Aris dispersion mechanism, i.e. the flux is driven by the cross-sectional average axial concentration gradient, whereas upon increase in  $Pe$ this mechanism fails. The general question addressed is where the system goes after the breakdown of the Taylor–Aris dispersion regime. In order to find out the answer, the following specific questions have to be addressed. (1) How does the Taylor–Aris dispersion mechanism break down upon increase in
$Pe$ this mechanism fails. The general question addressed is where the system goes after the breakdown of the Taylor–Aris dispersion regime. In order to find out the answer, the following specific questions have to be addressed. (1) How does the Taylor–Aris dispersion mechanism break down upon increase in 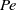 $Pe$? (2) Why does it break down? (3) What is the role of the channel aspect ratio in this breakdown? The answers to these questions are obtained through analysing a hierarchy of suitable auxiliary model problems, including the unidirectional zero discharge channel flow and the circulatory analogue of plane-parallel Couette flow, for which most of the analysis is done. They may be summarized as follows. Upon increase in circulation velocity, the Taylor–Aris dispersion mechanism fails due to the formation of lateral non-uniformities of longitudinal solute concentration gradient driving the dispersion flux. These non-uniformities accumulate in protrusion-like disturbances of the solute concentration (wall fingers) emerging near the channel sidewall at the flow exit from the edge. Wall fingers propagate along the sidewalls with increase in
$Pe$? (2) Why does it break down? (3) What is the role of the channel aspect ratio in this breakdown? The answers to these questions are obtained through analysing a hierarchy of suitable auxiliary model problems, including the unidirectional zero discharge channel flow and the circulatory analogue of plane-parallel Couette flow, for which most of the analysis is done. They may be summarized as follows. Upon increase in circulation velocity, the Taylor–Aris dispersion mechanism fails due to the formation of lateral non-uniformities of longitudinal solute concentration gradient driving the dispersion flux. These non-uniformities accumulate in protrusion-like disturbances of the solute concentration (wall fingers) emerging near the channel sidewall at the flow exit from the edge. Wall fingers propagate along the sidewalls with increase in 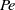 $Pe$ and eventually reach the opposite channel edges, transforming into narrow surface convection layers. These layers, together with the edge diffusion layers, form a closed mass transport pattern carrying most of the mass flux through the channel with the bulk largely excluded from the transport. The formation of this pattern finalizes the transition from Taylor–Aris dispersion to the surface convection regime. For large circulation velocities, concentration distribution in the surface convection layers attains an oscillatory spiral structure reminiscent of thermal waves in heat conduction.
$Pe$ and eventually reach the opposite channel edges, transforming into narrow surface convection layers. These layers, together with the edge diffusion layers, form a closed mass transport pattern carrying most of the mass flux through the channel with the bulk largely excluded from the transport. The formation of this pattern finalizes the transition from Taylor–Aris dispersion to the surface convection regime. For large circulation velocities, concentration distribution in the surface convection layers attains an oscillatory spiral structure reminiscent of thermal waves in heat conduction.