Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-27T02:41:24.010Z Has data issue: false hasContentIssue false
  • English
  • Français

Confined self-propulsion of an isotropic active colloid

Published online by Cambridge University Press:  23 December 2021

Francesco Picella Open the ORCID record for Francesco Picella [Opens in a new window]  and
Sébastien Michelin Open the ORCID record for Sébastien Michelin [Opens in a new window]
Francesco Picella
Affiliation:
LadHyX, CNRS – Ecole Polytechnique, Institut Polytechnique de Paris, 91120 Palaiseau, France
Sébastien Michelin*
Affiliation:
LadHyX, CNRS – Ecole Polytechnique, Institut Polytechnique de Paris, 91120 Palaiseau, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

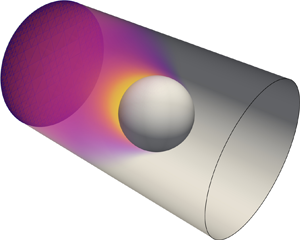
To spontaneously break their intrinsic symmetry and self-propel at the micron scale, isotropic active colloidal particles and droplets exploit the nonlinear convective transport of chemical solutes emitted/consumed at their surface by the surface-driven fluid flows generated by these solutes. Significant progress was recently made to understand the onset of self-propulsion and nonlinear dynamics. Yet, most models ignore a fundamental experimental feature, namely the spatial confinement of the colloid, and its effect on propulsion. In this work the self-propulsion of an isotropic colloid inside a capillary tube is investigated numerically. A flexible computational framework is proposed based on a finite-volume approach on adaptative octree grids and embedded boundary methods. This method is able to account for complex geometric confinement, the nonlinear coupling of chemical transport and flow fields, and the precise resolution of the surface boundary conditions, that drive the system's dynamics. Somewhat counterintuitively, spatial confinement promotes the colloid's spontaneous motion by reducing the minimum advection-to-diffusion ratio or Péclet number, ${Pe}$, required to self-propel; furthermore, self-propulsion velocities are significantly modified as the colloid-to-capillary size ratio $\kappa$ is increased, reaching a maximum at fixed ${Pe}$ for an optimal confinement $0<\kappa <1$. These properties stem from a fundamental change in the dominant chemical transport mechanism with respect to the unbounded problem: with diffusion now restricted in most directions by the confining walls, the excess solute is predominantly convected away downstream from the colloid, enhancing front-back concentration contrasts. These results are confirmed quantitatively using conservation arguments and lubrication analysis of the tightly confined limit, $\kappa \rightarrow 1$.

JFM classification

Complex Fluids: Active matter Drops and Bubbles: Drops
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 933 , 25 February 2022 , A27
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Anderson, J.L. 1989 Colloid transport by interfacial forces. Annu. Rev. Fluid Mech. 21 (1), 61–99.CrossRefGoogle Scholar
Bechinger, C., Leonardo, R.D., Löwen, H., Reichhardt, C., Volpe, G. & Volpe, G. 2016 Active particles in complex and crowded environments. Rev. Mod. Phys. 88, 045006.CrossRefGoogle Scholar
Bell, J.B., Colella, P. & Glaz, H.M. 1989 A second-order projection method for the incompressible Navier–Stokes equations. J. Comput. Phys. 85 (2), 257–283.CrossRefGoogle Scholar
Berg, H.C. 1993 Random Walks in Biology. Princeton University Press.Google Scholar
de Blois, C., Bertin, V., Suda, S., Ichikawa, M., Reyssat, M. & Dauchot, O. 2021 Swimming droplet in 1D geometries, an active Bretherton problem. Soft Matt. 17, 6646–6660.CrossRefGoogle ScholarPubMed
de Blois, C., Reyssat, M., Michelin, S. & Dauchot, O. 2019 Flow field around a confined active droplet. Phys. Rev. Fluids 4 (5), 054001.CrossRefGoogle Scholar
Brooks, A.M. & Strano, M.S. 2020 A conceptual advance that gives microrobots legs. Nature 584 (7822), 530–531.CrossRefGoogle ScholarPubMed
Bunea, A.-I. & Glückstad, J. 2019 Strategies for optical trapping in biological samples: aiming at microrobotic surgeons. Laser Photonics Rev. 13 (4), 1800227.CrossRefGoogle Scholar
Cheon, S.I., Silva, L.B.C., Khair, A.S. & Zarzar, L.D. 2021 Interfacially-adsorbed particles enhance the self-propulsion of oil droplets in aqueous surfactant. Soft Matt. 17 (28), 6742–6750.CrossRefGoogle ScholarPubMed
Delmotte, B., Keaveny, E.E., Plouraboué, F. & Climent, E. 2015 Large-scale simulation of steady and time-dependent active suspensions with the force-coupling method. J. Comput. Phys. 302, 524–547.CrossRefGoogle Scholar
Desaï, N. & Michelin, S. 2021 Instability and self-propulsion of active droplets along a wall. (under review).CrossRefGoogle Scholar
Dreyfus, R., Baudry, J., Roper, M.L., Fermigier, M., Stone, H.A. & Bibette, J. 2005 Microscopic artificial swimmers. Nature 437 (7060), 862–865.CrossRefGoogle ScholarPubMed
Golestanian, R., Liverpool, T.B. & Ajdari, A. 2007 Designing phoretic micro- and nano-swimmers. New J. Phys. 9 (5), 126–126.CrossRefGoogle Scholar
Hokmabad, B.V., Dey, R., Jalaal, M., Mohanty, D., Almukambetova, M., Baldwin, K.A., Lohse, D. & Maass, C.C. 2021 Emergence of bimodal motility in active droplets. Phys. Rev. X 11 (1), 011043.Google Scholar
Hokmabad, B.V., Saha, S., Agudo-Canalejo, J., Golestanian, R. & Maass, C.C. 2020 Quantitative characterization of chemorepulsive alignment-induced interactions in active emulsions. arXiv:2012.05170.Google Scholar
van Hooft, J.A., Popinet, S., van Heerwaarden, C.C., van der Linden, S.J.A., de Roode, S.R. & van de Wiel, B.J.H. 2018 Towards adaptive grids for atmospheric boundary-layer simulations. Boundary-Layer Meteorol. 167 (3), 421–443.CrossRefGoogle ScholarPubMed
Howse, J.R., Jones, R.A.L., Ryan, A.J., Gough, T., Vafabakhsh, R. & Golestanian, R. 2007 Self-motile colloidal particles: from directed propulsion to random walk. Phys. Rev. Lett. 99 (4), 048102.CrossRefGoogle ScholarPubMed
Hu, W.-F., Lin, T.-S., Rafai, S. & Misbah, C. 2019 Chaotic swimming of phoretic particles. Phys. Rev. Lett. 123 (23), 238004.CrossRefGoogle ScholarPubMed
Illien, P., de Blois, C., Liu, Y., van der Linden, M.N. & Dauchot, O. 2020 Speed-dispersion-induced alignment: a one-dimensional model inspired by swimming droplets experiments. Phys. Rev. E 101 (4), 040602.CrossRefGoogle ScholarPubMed
Izri, Z., van der Linden, M.N., Michelin, S. & Dauchot, O. 2014 Self-propulsion of pure water droplets by spontaneous Marangoni-stress-driven motion. Phys. Rev. Lett. 113 (24), 248302.CrossRefGoogle ScholarPubMed
Jin, C., Vachier, J., Bandyopadhyay, S., Macharashvili, T. & Maass, C.C. 2019 Fine balance of chemotactic and hydrodynamic torques: when microswimmers orbit a pillar just once. Phys. Rev. E 100 (4), 040601.CrossRefGoogle ScholarPubMed
Johansen, H. & Colella, P. 1998 A Cartesian grid embedded boundary method for Poisson's equation on irregular domains. J. Comput. Phys. 147 (1), 60–85.CrossRefGoogle Scholar
Kim, S. & Karrila, S.J. 1991 Microhydrodynamics: Principles and Selected Applications. Butterworth- Heinemann.Google Scholar
Koleoso, M., Feng, X., Xue, Y., Li, Q., Munshi, T. & Chen, X. 2020 Micro/nanoscale magnetic robots for biomedical applications. Mater. Today Bio 8, 100085.CrossRefGoogle ScholarPubMed
Krüger, C., Bahr, C., Herminghaus, S. & Maass, C.C. 2016 Dimensionality matters in the collective behaviour of active emulsions. Eur. Phys. J. E 39 (6), 64.CrossRefGoogle ScholarPubMed
Kümmel, F., ten Hagen, B., Wittkowski, R., Buttinoni, I., Eichhorn, R., Volpe, G., Löwen, H. & Bechinger, C. 2013 Circular motion of asymmetric self-propelling particles. Phys. Rev. Lett. 110 (19), 198302.CrossRefGoogle ScholarPubMed
Lauga, E. & Powers, T.R. 2009 The hydrodynamics of swimming microorganisms. Rep. Prog. Phys. 72 (9), 096601.CrossRefGoogle Scholar
Leal, L.G. 2007 Advanced Transport Phenomena. Cambridge University Press.CrossRefGoogle Scholar
Lippera, K., Benzaquen, M. & Michelin, S. 2020 a Bouncing, chasing, or pausing: asymmetric collisions of active droplets. Phys. Rev. Fluids 5 (3), 032201.CrossRefGoogle Scholar
Lippera, K., Morozov, M., Benzaquen, M. & Michelin, S. 2020 b Collisions and rebounds of chemically active droplets. J. Fluid Mech. 886, A17.CrossRefGoogle Scholar
Maass, C.C., Krüger, C., Herminghaus, S. & Bahr, C. 2016 Swimming droplets. Annu. Rev. Condens. Matt. Phys. 7 (1), 171–193.CrossRefGoogle Scholar
Magdanz, V., et al. 2020 IRONSperm: sperm-templated soft magnetic microrobots. Sci. Adv. 6 (28), eaba5855.CrossRefGoogle ScholarPubMed
Marchetti, M.C., Joanny, J.F., Ramaswamy, S., Liverpool, T.B., Prost, J., Rao, M. & Simha, R.A. 2013 Hydrodynamics of soft active matter. Rev. Mod. Phys. 85 (3), 1143–1189.CrossRefGoogle Scholar
Michelin, S. & Lauga, E. 2015 Autophoretic locomotion from geometric asymmetry. Eur. Phys. J. E 38 (2), 7.CrossRefGoogle ScholarPubMed
Michelin, S., Lauga, E. & Bartolo, D. 2013 Spontaneous autophoretic motion of isotropic particles. Phys. Fluids 25 (6), 061701.CrossRefGoogle Scholar
Moerman, P.G., Moyses, H.W., van der Wee, E.B., Grier, D.G., van Blaaderen, A., Kegel, W.K., Groenewold, J. & Brujic, J. 2017 Solute-mediated interactions between active droplets. Phys. Rev. E 96 (3), 032607.CrossRefGoogle ScholarPubMed
Montenegro-Johnson, T.D., Michelin, S. & Lauga, E. 2015 A regularised singularity approach to phoretic problems. Eur. Phys. J. E 38 (12), 139.CrossRefGoogle ScholarPubMed
Moran, J. & Posner, J. 2019 Microswimmers with no moving parts. Phys. Today 72 (5), 44–50.CrossRefGoogle Scholar
Moran, J.L. & Posner, J.D. 2017 Phoretic self-propulsion. Annu. Rev. Fluid Mech. 49 (1), 511–540.CrossRefGoogle Scholar
Morozov, M. 2020 Adsorption inhibition by swollen micelles may cause multistability in active droplets. Soft Matt. 16 (24), 5624–5632.CrossRefGoogle ScholarPubMed
Morozov, M. & Michelin, S. 2019 a Nonlinear dynamics of a chemically-active drop: from steady to chaotic self-propulsion. J. Chem. Phys. 150 (4), 044110.CrossRefGoogle ScholarPubMed
Morozov, M. & Michelin, S. 2019 b Self-propulsion near the onset of Marangoni instability of deformable active droplets. J. Fluid Mech. 860, 711–738.CrossRefGoogle Scholar
Nelson, B.J., Kaliakatsos, I.K. & Abbott, J.J. 2010 Microrobots for minimally invasive medicine. Annu. Rev. Biomed. Engng 12 (1), 55–85.CrossRefGoogle ScholarPubMed
Paxton, W.F., Kistler, K.C., Olmeda, C.C., Sen, A., Angelo, S.K., Cao, Y., Mallouk, T.E., Lammert, P.E. & Crespi, V.H. 2004 Catalytic nanomotors: autonomous movement of striped nanorods. J. Am. Chem. Soc. 126 (41), 13424–13431.CrossRefGoogle ScholarPubMed
Popinet, S. 2003 Gerris: a tree-based adaptive solver for the incompressible Euler equations in complex geometries. J. Comput. Phys. 190 (2), 572–600.CrossRefGoogle Scholar
Popinet, S. 2015 A quadtree-adaptive multigrid solver for the Serre–Green–Naghdi equations. J. Comput. Phys. 302, 336–358.CrossRefGoogle Scholar
Pozrikidis, C. 1992 Boundary Integral and Singularity Methods for Linearized Viscous Flow. Cambridge University Press.CrossRefGoogle Scholar
Purcell, E.M. 1977 Life at low Reynolds number. Am. J. Phys. 45 (1), 3–11.CrossRefGoogle Scholar
Rao, K.J., Li, F., Meng, L., Zheng, H., Cai, F. & Wang, W. 2015 A force to be reckoned with: a review of synthetic microswimmers powered by ultrasound. Small 11 (24), 2836–2846.CrossRefGoogle ScholarPubMed
Rojas-Pérez, F., Delmotte, B. & Michelin, S. 2021 Hydrochemical interactions of phoretic particles: a regularized multipole framework. J. Fluid Mech. 919, A22.CrossRefGoogle Scholar
Sangani, A.S. & Mo, G. 1996 An $O(N)$ algorithm for Stokes and Laplace interactions of particles. Phys. Fluids 8 (8), 1990–2010.CrossRefGoogle Scholar
Schneiders, L, Günther, C., Meinke, M. & Schröder, W. 2016 An efficient conservative cut-cell method for rigid bodies interacting with viscous compressible flows. J. Comput. Phys. 311, 62–86.CrossRefGoogle Scholar
Schwartz, P., Barad, M., Colella, P. & Ligocki, T. 2006 A Cartesian grid embedded boundary method for the heat equation and Poisson's equation in three dimensions. J. Comput. Phys. 211 (2), 531–550.CrossRefGoogle Scholar
Selçuk, C., Ghigo, A.R., Popinet, S. & Wachs, A. 2021 A fictitious domain method with distributed Lagrange multipliers on adaptive quad/octrees for the direct numerical simulation of particle-laden flows. J. Comput. Phys. 430, 109954.CrossRefGoogle Scholar
Sherwood, J.D. & Ghosal, S. 2018 Nonlinear electrophoresis of a tightly fitting sphere in a cylindrical tube. J. Fluid Mech. 843, 847–871.CrossRefGoogle Scholar
Stone, H.A. & Samuel, A.D.T. 1996 Propulsion of microorganisms by surface distortions. Phys. Rev. Lett. 77 (19), 4102–4104.CrossRefGoogle ScholarPubMed
Suga, M., Suda, S., Ichikawa, M. & Kimura, Y. 2018 Self-propelled motion switching in nematic liquid crystal droplets in aqueous surfactant solutions. Phys. Rev. E 97 (6), 062703.CrossRefGoogle ScholarPubMed
Thutupalli, S., Seemann, R. & Herminghaus, S. 2011 Swarming behavior of simple model squirmers. New J. Phys. 13 (7), 073021.CrossRefGoogle Scholar
Varma, A., Montenegro-Johnson, T.D. & Michelin, S. 2018 Clustering-induced self-propulsion of isotropic autophoretic particles. Soft Matt. 14 (35), 7155–7173.CrossRefGoogle ScholarPubMed
Yan, W. & Brady, J.F. 2016 The behavior of active diffusiophoretic suspensions: an accelerated Laplacian dynamics study. J. Chem. Phys. 145 (13), 134902.CrossRefGoogle ScholarPubMed
Yu, T., Chuphal, P., Thakur, S., Reigh, S.Y., Singh, D.P. & Fischer, P. 2018 Chemical micromotors self-assemble and self-propel by spontaneous symmetry breaking. Chem. Commun. 54 (84), 11933–11936.CrossRefGoogle ScholarPubMed
Zhu, L., Lauga, E. & Brandt, L. 2013 Low-Reynolds-number swimming in a capillary tube. J. Fluid Mech. 726, 285–311.CrossRefGoogle Scholar