Article contents
Compressible vapour flow in conduits and fractures
Published online by Cambridge University Press: 10 August 2016
Abstract
We consider the steady flow of a viscous compressible gas through an axisymmetric or two-dimensional porous medium whose properties in the direction of the flow are sufficiently slowly varying. The study is partly motivated by a number of different applications in the Earth sciences, including the release of magmatic volatiles from a magma chamber beneath an active volcano and the discharge of geothermal fluids. The results are also relevant to evaluating the consequences of an accidental release of carbon dioxide from a storage reservoir within the Earth, as might happen at a carbon capture and storage (CCS) site. We consider both slow, thermally equilibrated, flows and fast, adiabatic flows. Because the flow is compressible, it is the mass (and not the volume) flux which is conserved along the flow. We determine this constant mass flux and the velocity and pressure fields, both of which vary with position along the flow, as a function of all the physical parameters. We find that the resultant pressure gradient in the medium is largest at the far, low-pressure end of the conduit because the velocity is largest at that end due to the smallest density being associated with the smallest pressures. This means that the pressure in the permeable conduit is always larger than the linear pressure distribution which joins the given pressures at depth and at the surface, as would be the situation if the flow were incompressible. The detailed pressure distribution is shown to depend on the variation with depth of the quantity 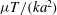 $\unicode[STIX]{x1D707}T/(ka^{2})$, where
$\unicode[STIX]{x1D707}T/(ka^{2})$, where 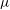 $\unicode[STIX]{x1D707}$ is the dynamic viscosity of the vapour,
$\unicode[STIX]{x1D707}$ is the dynamic viscosity of the vapour, 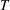 $T$ is the external temperature,
$T$ is the external temperature, 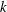 $k$ the permeability and
$k$ the permeability and 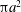 $\unicode[STIX]{x03C0}a^{2}$ the cross-sectional area of the conduit. The resultant mass flux is determined to be proportional to the mean along the flow of
$\unicode[STIX]{x03C0}a^{2}$ the cross-sectional area of the conduit. The resultant mass flux is determined to be proportional to the mean along the flow of 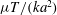 $\unicode[STIX]{x1D707}T/(ka^{2})$. We present two numerical illustrations of the results.
$\unicode[STIX]{x1D707}T/(ka^{2})$. We present two numerical illustrations of the results.
- Type
- Papers
- Information
- Copyright
- © 2016 Cambridge University Press
References
- 5
- Cited by



