Published online by Cambridge University Press: 21 March 2018
Compressible flow varies from ideal-gas behaviour at high pressures where molecular interactions become important. It is widely accepted that density is well described through a cubic equation of state while enthalpy and sound speed are functions of both temperature and pressure, based on two parameters, 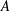 $A$ and
$A$ and 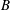 $B$, related to intermolecular attraction and repulsion, respectively. Assuming small variations from ideal-gas behaviour, a closed-form approximate solution is obtained that is valid over a wide range of conditions. An expansion in these molecular interaction parameters simplifies relations for flow variables, elucidating the role of molecular repulsion and attraction in variations from ideal-gas behaviour. Real-gas modifications in density, enthalpy and sound speed for a given pressure and temperature lead to variations in many basic compressible-flow configurations. Sometimes, the variations can be substantial in quantitative or qualitative terms. The new approach is applied to choked-nozzle flow, isentropic flow, nonlinear wave propagation and flow across a shock wave, all for a real gas. Modifications are obtained for allowable mass flow through a choked nozzle, nozzle thrust, sonic wave speed, Riemann invariants, Prandtl’s shock relation and the Rankine–Hugoniot relations. Forced acoustic oscillations can show substantial augmentation of pressure amplitudes when real-gas effects are taken into account. Shocks at higher temperatures and pressures can have larger pressure jumps with real-gas effects. Weak shocks decay to zero strength at sonic speed. The proposed framework can rely on any cubic equation of state and can be applied to multicomponent flows or to more complex flow configurations.
$B$, related to intermolecular attraction and repulsion, respectively. Assuming small variations from ideal-gas behaviour, a closed-form approximate solution is obtained that is valid over a wide range of conditions. An expansion in these molecular interaction parameters simplifies relations for flow variables, elucidating the role of molecular repulsion and attraction in variations from ideal-gas behaviour. Real-gas modifications in density, enthalpy and sound speed for a given pressure and temperature lead to variations in many basic compressible-flow configurations. Sometimes, the variations can be substantial in quantitative or qualitative terms. The new approach is applied to choked-nozzle flow, isentropic flow, nonlinear wave propagation and flow across a shock wave, all for a real gas. Modifications are obtained for allowable mass flow through a choked nozzle, nozzle thrust, sonic wave speed, Riemann invariants, Prandtl’s shock relation and the Rankine–Hugoniot relations. Forced acoustic oscillations can show substantial augmentation of pressure amplitudes when real-gas effects are taken into account. Shocks at higher temperatures and pressures can have larger pressure jumps with real-gas effects. Weak shocks decay to zero strength at sonic speed. The proposed framework can rely on any cubic equation of state and can be applied to multicomponent flows or to more complex flow configurations.