Article contents
Complex solutions of the Dean equations and non-uniqueness at all Reynolds numbers
Published online by Cambridge University Press: 29 March 2017
Abstract
Steady incompressible flow down a slowly curving circular pipe is considered, analytically and numerically. Both real and complex solutions are investigated. Using high-order Hermite–Padé approximants, the Dean series solution is analytically continued outside its circle of convergence, where it predicts a complex solution branch for real positive Dean number, 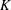 $K$. This is confirmed by numerical solution. It is shown that other previously unknown solution branches exist for all
$K$. This is confirmed by numerical solution. It is shown that other previously unknown solution branches exist for all  $K>0$, which are related to an unforced complex eigensolution. This non-uniqueness is believed to be generic to the Navier–Stokes equations in most geometries. By means of path continuation, numerical solutions are followed around the complex
$K>0$, which are related to an unforced complex eigensolution. This non-uniqueness is believed to be generic to the Navier–Stokes equations in most geometries. By means of path continuation, numerical solutions are followed around the complex 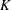 $K$-plane. The standard Dean two-vortex solution is shown to lie on the same hypersurface as the eigensolution and the four-vortex solutions found in the literature. Elliptic pipes are considered and shown to exhibit similar behaviour to the circular case. There is an imaginary singularity limiting convergence of the Dean series, an unforced solution at
$K$-plane. The standard Dean two-vortex solution is shown to lie on the same hypersurface as the eigensolution and the four-vortex solutions found in the literature. Elliptic pipes are considered and shown to exhibit similar behaviour to the circular case. There is an imaginary singularity limiting convergence of the Dean series, an unforced solution at  $K=0$ and non-uniqueness for
$K=0$ and non-uniqueness for 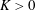 $K>0$, culminating in a real bifurcation.
$K>0$, culminating in a real bifurcation.
- Type
- Papers
- Information
- Copyright
- © 2017 Cambridge University Press
References
- 3
- Cited by



