Hostname: page-component-78c5997874-94fs2 Total loading time: 0 Render date: 2024-11-17T11:23:57.321Z Has data issue: false hasContentIssue false
  • English
  • Français

The common mechanism of turbulent skin-friction drag reduction with superhydrophobic longitudinal microgrooves and riblets

Published online by Cambridge University Press:  10 January 2018

Amirreza Rastegari Open the ORCID record for Amirreza Rastegari [Opens in a new window]  and
Rayhaneh Akhavan Open the ORCID record for Rayhaneh Akhavan [Opens in a new window]
Amirreza Rastegari
Affiliation:
Department of Mechanical Engineering, The University of Michigan, Ann Arbor, MI 48109-2125, USA
Rayhaneh Akhavan*
Affiliation:
Department of Mechanical Engineering, The University of Michigan, Ann Arbor, MI 48109-2125, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Turbulent skin-friction drag reduction with superhydrophobic (SH) longitudinal microgrooves and riblets is investigated by direct numerical simulation (DNS), using lattice Boltzmann methods, in channel flow. The liquid/gas interfaces in the SH longitudinal microgrooves were modelled as stationary, curved, shear-free boundaries, with the meniscus shape determined from the solution of the Young–Laplace equation. Interface protrusion angles of 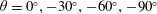$\unicode[STIX]{x1D703}=0^{\circ },-30^{\circ },-60^{\circ },-90^{\circ }$ were investigated. For comparison, the same geometries as those formed by the SH interfaces were also studied as riblets. Drag reductions of up to 61 % and up to 5 % were realized in DNS with SH longitudinal microgrooves and riblets, respectively, in turbulent channel flows at bulk Reynolds numbers of 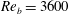$Re_{b}=3600$ (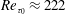$Re_{\unicode[STIX]{x1D70F}_{0}}\approx 222$) and 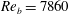$Re_{b}=7860$ (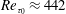$Re_{\unicode[STIX]{x1D70F}_{0}}\approx 442$), with arrays of SH longitudinal microgrooves or riblets of size 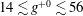$14\lesssim g^{+0}\lesssim 56$ and 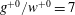$g^{+0}/w^{+0}=7$ on both walls, where 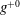$g^{+0}$ and 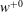$w^{+0}$ denote the widths and spacings of the microgrooves in base flow wall units, respectively. An exact analytical expression is derived which allows the net drag reduction in laminar or turbulent channel flow with any SH or no-slip wall micro-texture to be decomposed into contributions from: (i) the effective slip velocity at the wall, (ii) modifications to the normalized structure of turbulent Reynolds shear stresses due to the presence of this effective slip velocity at the wall, (iii) other modifications to the normalized structure of turbulent Reynolds shear stresses due to the presence of the wall micro-texture, (iv) modifications to the normalized structure of mean flow shear stresses due to the presence of the wall micro-texture and (v) the fraction of the flow rate through the wall micro-texture. Comparison to DNS results shows that SH longitudinal microgrooves and riblets share a common mechanism of drag reduction in which 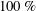$100\,\%$ of the drag reduction arises from effects (i) and (ii). The contributions from (iii)–(v) were always drag enhancing, and followed a common scaling with SH longitudinal microgrooves and riblets when expressed as a function of the square root of the microgroove cross-sectional area in wall units. Extrapolation of drag reduction data from DNS to high Reynolds number flows of practical interest is discussed. It is shown that, for a given geometry and size of the surface micro-texture in wall units, the drag reduction performance of micro-textured surfaces degrades with increasing bulk Reynolds number of the flow. Curved SH interfaces at low protrusion angle (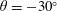$\unicode[STIX]{x1D703}=-30^{\circ }$) were found to enhance the drag reduction by up to 3.6 % compared to flat interfaces, while reducing the instantaneous pressure fluctuations on the SH interfaces by up to a factor of two. This suggests that the longevity of SH interfaces in turbulent flow may be improved by embedding the SH surface within the microgrooves of shallow, scalloped riblets.

JFM classification

Flow Control: Drag reduction Micro-/Nano-fluid dynamics: Micro-/Nano-fluid dynamics Turbulent Flows: Turbulence control
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 838 , 10 March 2018 , pp. 68 - 104
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bechert, D. W. & Bartenwerfer, M. 1989 The viscous flow on surfaces with longitudinal ribs. J. Fluid Mech. 206, 105–129.Google Scholar
Bechert, D. W., Bruse, M., Hage, W., Van Der Hoeven, J. G. T. & Hoppe, G. 1997 Experiments on drag-reducing surfaces and their optimization with an adjustable geometry. J. Fluid Mech. 338, 59–87.Google Scholar
Bushnell, D. & Hefner, J. N. 1990 Viscous drag reduction in boundary layers. In Progress in Astronautics and Aeronautics (ed. Bushnell, D. & Hefner, J. N.), vol. 123. AIAA.Google Scholar
Cha, T., Yi, J. W., Moon, M., Lee, K. & Kim, H. 2010 Nanoscale patterning of microtextured surfaces to control superhydrophobic robustness. Langmuir 26, 8319–8326.Google Scholar
Checco, A., Ocko, B. M., Rhamn, A., Black, C. T., Tasinkevych, M., Giacomello, A. & Dietrich, S. 2014 Collapse and reversibility of the superhydrophobic state on nanotextured surfaces. Phys. Rev. Lett. 112, 216101.Google Scholar
Choi, H., Moin, P. & Kim, J. 1993 Direct numerical simulation of turbulent flow over riblets. J. Fluid Mech. 255, 503–539.Google Scholar
Chu, D. C. & Karniadakis, G. E. 1993 A direct numerical simulation of laminar and turbulent flow over riblet-mounted surfaces. J. Fluid Mech. 250, 1–42.CrossRefGoogle Scholar
Clauser, F. H. 1956 The turbulent boundary layer. Adv. Appl. Mech. 4, 1–51.Google Scholar
Crowdy, D. 2016 Analytical formulae for longitudinal slip lengths over unidirectional superhydrophobic surfaces with curved menisci. J. Fluid Mech. 791, R7.Google Scholar
Dean, R. D. 1978 Reynolds number dependence of skin friction and other bulk flow variables in two dimensional rectangular duct flow. Trans. ASME J. Fluids Engng 100, 215–223.CrossRefGoogle Scholar
Feng, L., Li, S., Li, Y., Li, H., Zhng, L., Zhai, J., Song, Y., Liu, B., Jiang, L. & Zhu, D. 2002 Superhydrophobic surfaces: from natural to artificial. Adv. Mater. 14, 1857–1860.Google Scholar
Fukagata, K., Iwamoto, K. & Kasagi, N. 2002 Contribution of Reynolds stress distribution to the skin-friction in wall-bounded flows. Phys. Fluids 14, L73–L76.Google Scholar
Fukagata, K., Kasagi, N. & Koumoutsakos, P. 2006 A theoretical prediction of friction drag reduction in turbulent flow by superhydrophobic surfaces. Phys. Fluids 18, 051703.Google Scholar
Garcia-Mayoral, R. & Jimenez, J. 2011a Hydrodynamic stability and breakdown of the viscous regime over riblets. J. Fluid Mech. 678, 317–347.CrossRefGoogle Scholar
Garcia-Mayoral, R. & Jimenez, J. 2011b Drag reduction by riblets. Phil. Trans. R. Soc. Lond. A 369, 1412–1427.Google Scholar
Garcia-Mayoral, R. & Jimenez, J. 2012 Scaling of turbulent structures in riblet channels up to Ret = 550. Phys. Fluids 24, 105101.Google Scholar
de Gennes, P., Brochard-Wyart, F. & Quéré, D. 2002 Capillarity and Wetting Phenomena: Drops, Bubbles, Pearls, Waves. Springer.Google Scholar
Ginzburg, I. & Steiner, K. 2003 Lattice Boltzmann model for free-surface flow and its application to filling process in casting. J. Comput. Phys. 185, 61–99.Google Scholar
Ginzburg, I., Verhaeghe, F. & d’Humières, D. 2008 Two-relaxation-time lattice Boltzmann scheme: About parametrization, velocity, pressure and mixed boundary conditions. Commun. Comput. Phys. 3, 427–478.Google Scholar
Goldstein, D. B., Handler, R. & Sirovich, L. 1995 Direct numerical simulation of turbulent flow over a modeled riblet covered surface. J. Fluid Mech. 302, 333–376.CrossRefGoogle Scholar
Goldstein, D. B. & Tuan, T.-C. 1998 Secondary flow induced by riblets. J. Fluid Mech. 363, 115–151.CrossRefGoogle Scholar
Jelly, T. O., Jung, S. Y. & Zaki, T. A. 2014 Turbulence and skin friction modification in channel flow with streamwise-aligned superhydrophobic surface texture. Phys. Fluids 26, 095102.CrossRefGoogle Scholar
Jung, T., Choi, H. & Kim, J. 2016 Effects of the air layer of an idealized superhydrophobic surface on the slip length and skin-friction drag. J. Fluid Mech. 790, R1.Google Scholar
Karatay, E., Tsai, P. A. & Lammertink, R. G. 2013a Rate of gas absorption on a slippery bubble mattress. Soft Matt. 9, 11098–11106.Google Scholar
Karatay, E., Haase, A. S., Visser, C. W., Sun, C., Lohse, D., Tsai, P. A. & Lammertink, R. G. 2013b Control of slippage with tunable bubble mattresses. Proc. Natl Acad. Sci. USA 110, 8422–8426.Google Scholar
Kwon, Y., Patnakar, N., Choi, J. & Lee, J. 2009 Design of surface hierarchy for extreme hydrophobicity. Langmuir 25, 6129–6136.Google Scholar
Lagrava, D., Malaspinas, O., Latt, J. & Chopard, B. 2012 Advances in multi-domain lattice Boltzmann grid refinement. J. Comput. Phys. 231, 4808–4822.Google Scholar
Lammers, P., Beronov, K. N., Volkert, R., Brenner, G. & Durst, F. 2006 Lattice BGK direct numerical simulation of fully developed turbulence in incompressible plane channel flow. Comput. Fluids 35, 1137–1153.CrossRefGoogle Scholar
Lee, C. & Kim, C.-J. 2009 Maximizing the giant liquid slip on superhydrophobic microstructures by nanostructuring their sidewalls. Langmuir 25, 12812–12818.CrossRefGoogle ScholarPubMed
Lee, C. & Kim, C.-J. 2011 Underwater restoration and retention of gases on superhydrophobic surfaces for drag reduction. Phys. Rev. Lett. 106, 014502.Google Scholar
Luchini, P., Manzo, F. & Pozzi, A. 1991 Resistance of a grooved surface to parallel flow and cross-flow. J. Fluid Mech. 228, 87–109.Google Scholar
Martel, M., Perot, J. B. & Rothstein, J. P. 2009 Direct numerical simulations of turbulent flows over superhydrophobic surfaces. J. Fluid Mech. 620, 31–41.CrossRefGoogle Scholar
Martel, M. B., Rothstein, J. P. & Perot, J. B. 2010 An analysis of superhydrophobic turbulent drag reduction mechanisms using direct numerical simulation. Phys. Fluids 22, 065102.Google Scholar
Min, T. & Kim, J. 2004 Effect of superhydrophobic surfaces on skin-friction drag. Phys. Fluids 16, L55.Google Scholar
Nishino, T., Meguro, M., Nakamae, K., Matsushita, M. & Ueda, Y. 1999 The lowest surface free energy based on CF3 alignment. Langmuir 15, 4321–4323.Google Scholar
Ou, J. & Rothstein, J. P. 2005 Direct velocity measurements of the flow past drag reducing ultrahydrophobic surfaces. Phys. Fluids 17, 103606.Google Scholar
Park, H., Park, H. & Kim, J. 2013 A numerical study of the effects of superhydrophobic surface on skin-friction drag in turbulent channel flow. Phys. Fluids 25, 110815.CrossRefGoogle Scholar
Park, H., Sun, G. & Kim, C.-J. 2014 Superhydrophobic turbulent drag reduction as a function of surface grating parameters. J. Fluid Mech. 747, 722–734.CrossRefGoogle Scholar
Peet, Y. & Sagaut, P. 2009 Theoretical prediction of turbulent skin friction on geometrically complex surfaces. Phys. Fluids 21, 105105.Google Scholar
Philip, J. R. 1972 Flows satisfying mixed no-slip and no-shear conditions. Z. Angew. Math. Phys. 23, 353–372.Google Scholar
Rastegari, A. & Akhavan, R. 2013 Lattice Boltzmann simulations of drag reduction by super-hydrophobic surfaces. In Proceedings 14th European Turbulence Conference, 1-4 September, Lyon, France.Google Scholar
Rastegari, A. & Akhavan, R. 2015 On the mechanism of turbulent drag reduction with super-hydrophobic surfaces. J. Fluid Mech. 773, R4.Google Scholar
Rathgen, H. & Mugel, F. 2010 Microscopic shape and contact angle measurement at a superhydrophobic surface. Faraday Discuss. 146, 49–56.Google Scholar
Rosenberg, B. J., Van Buren, T., Fu, M. K. & Smits, A. J. 2016 Turbulent drag reduction over air- and liquid- impregnated surfaces. Phys. Fluids 28, 015103.Google Scholar
Rothstein, J. P. 2010 Slip on superhydrophobic surfaces. Annu. Rev. Fluid Mech. 42, 89–109.Google Scholar
Sbragaglia, M. & Prosperetti, A. 2007 A note on the effective slip properties for microchannel flows with ultrahydrophobic surfaces. Phys. Fluids 19, 043603.Google Scholar
Schellenberger, F., Encinas, N., Vollmer, D. & Butt, H. 2016 How water advances on superhydrophobic surfaces. Phys. Rev. Lett. 116, 096101.Google Scholar
Seo, J., Garcia-Mayoral, R. & Mani, A. 2015 Pressure fluctuations and interfacial robustness in turbulent flows over superhydrophobic surfaces. J. Fluid Mech. 783, 448–473.Google Scholar
Seo, J. & Mani, A. 2016 On the scaling of the slip velocity in turbulent flows over superhydrophobic surfaces. Phys. Fluids 28, 025110.CrossRefGoogle Scholar
Spalart, P. R. & Mclean, J. D. 2011 Drag reduction: enticing turbulence, and then an industry. Phil. Trans. R. Soc. Lond. A 369, 1556–1569.Google Scholar
Steinberger, A., Cottin-Bizonne, C., Kleimann, P. & Charlaix, E. 2007 High friction on a bubble mattress. Nat. Mater. 6, 665–668.Google Scholar
Succi, S. 2001 The Lattice Boltzmann Equation for Fluid Dynamics and Beyond. Oxford University Press.CrossRefGoogle Scholar
Tsai, P., Peters, A. M., Pirat, C., Wessling, M., Lammertink, R. G. H. & Lohse, D. 2009 Quantifying effective slip length over micropatterned hydrophobic surfaces. Phys. Fluids 21, 112002.Google Scholar
Türk, S., Daschiel, G., Stroh, A., Hasegawa, Y. & Frohnapfel, B. 2014 Turbulent flow over superhydrophobic surfaces with streamwise grooves. J. Fluid Mech. 747, 186–217.Google Scholar
Walsh, M. J. 1980 Drag characteristics of V-groove and transverse curvature riblets. In Viscous Flow Drag Reduction (ed. Hough, G. R.), Progress in Astronautics and Aeronautics, vol. 72, pp. 168–184. AIAA.Google Scholar
Walsh, M. J.1982 Turbulent boundary layer drag reduction using riblets. AIAA Paper 82-0169.CrossRefGoogle Scholar
Walsh, M. J. 1990 Riblets. In Viscous Drag Reduction in Boundary Layers (ed. Bushnell, D. & Hefner, J.), Progress in Astronautics and Aeronautics, vol. 123, pp. 203–259. AIAA.Google Scholar
Wang, Z., Koratkar, N., Ci, L. & Ajayan, P. M. 2007 Combined micro-/nanoscale surface roughness for enhanced hydrophobic stability in carbon nanotube arrays. Appl. Phys. Lett. 90, 143117.Google Scholar
Wang, L. P., Teo, C. J. & Khoo, B. C. 2014 Effects of interface deformation on flow through microtubes containing superhydrophobic surfaces with longitudinal ribs and grooves. Microfluid Nanofluid 16, 225–236.Google Scholar
Wong, T. S., Kang, S. H., Tang, S. K. Y., Smythe, E. J., Hatton, B. D., Grinthal, A. & Aizenberg, J. 2011 Bioinspired self-repairing slippery surfaces with pressure-stable omniphobicity. Nature 477, 443–447.Google Scholar