Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-25T17:48:14.925Z Has data issue: false hasContentIssue false
  • English
  • Français

Collision rate of bidisperse spheres settling in a compressional non-continuum gas flow

Published online by Cambridge University Press:  08 January 2021

Johnson Dhanasekaran Open the ORCID record for Johnson Dhanasekaran [Opens in a new window] ,
Anubhab Roy Open the ORCID record for Anubhab Roy [Opens in a new window]  and
Donald L. Koch Open the ORCID record for Donald L. Koch [Opens in a new window]
Johnson Dhanasekaran
Affiliation:
Sibley School of Mechanical and Aerospace Engineering, Cornell University, Ithaca, NY14853, USA
Anubhab Roy
Affiliation:
Department of Applied Mechanics, Indian Institute of Technology Madras, Chennai, Tamil Nadu600036, India
Donald L. Koch*
Affiliation:
Smith School of Chemical and Biomolecular Engineering, Cornell University, Ithaca, NY14853, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

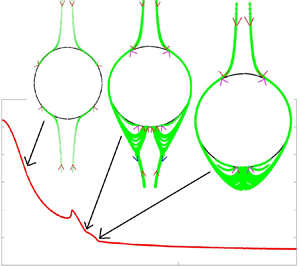
Collisions in a dilute polydisperse suspension of spheres of negligible inertia interacting through non-continuum hydrodynamics and settling in a slow uniaxial compressional flow are studied. The ideal collision rate is evaluated as a function of the relative strength of gravity and uniaxial compressional flow and it deviates significantly from a linear superposition of these driving terms. This non-trivial behaviour is exacerbated by interparticle interactions based on uniformly valid non-continuum hydrodynamics, that capture non-continuum lubrication at small separations and full continuum hydrodynamic interactions at larger separations, retarding collisions driven purely by sedimentation significantly more than those driven purely by the linear flow. While the ideal collision rate is weakly dependent on the orientation of gravity with the axis of compression, the rate including hydrodynamic interactions varies by more than $100\,\%$ with orientation. This dramatic shift can be attributed to complex trajectories driven by interparticle interactions that prevent particle pairs from colliding or enable a circuitous path to collision. These and other important features of the collision process are studied in detail using trajectory analysis at near unity and significantly smaller than unity size ratios of the interacting spheres. For each case analysis is carried for a large range of relative strengths and orientations of gravity to the uniaxial compressional flow, and Knudsen numbers (ratio of mean free path of the media to mean radius).

JFM classification

Drops and Bubbles: Breakup/coalescence Low-Reynolds-number flows: Stokesian dynamics Multiphase and Particle-laden Flows: Particle/fluid flow
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 910 , 10 March 2021 , A10
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Ashurst, W. T., Kerstein, A. R., Kerr, R. M. & Gibson, C. H. 1987 Alignment of vorticity and scalar gradient with strain rate in simulated Navier–Stokes turbulence. Phys. Fluids 30 (8), 2343–2353.CrossRefGoogle Scholar
Bach, G. A., Koch, D. L. & Gopinath, A. 2004 Coalescence and bouncing of small aerosol droplets. J. Fluid Mech. 518, 157–185.CrossRefGoogle Scholar
Balthasar, M., Mauss, F., Knobel, A. & Kraft, M. 2002 Detailed modeling of soot formation in a partially stirred plug flow reactor. Combust. Flame 128 (4), 395–409.CrossRefGoogle Scholar
Batchelor, G. K. 1982 Sedimentation in a dilute polydisperse system of interacting spheres. Part 1. General theory. J. Fluid Mech. 119, 379–408.CrossRefGoogle Scholar
Batchelor, G. K. & Green, J.-T. 1972 The hydrodynamic interaction of two small freely-moving spheres in a linear flow field. J. Fluid Mech. 56 (2), 375–400.CrossRefGoogle Scholar
Brunk, B. K., Koch, D. L. & Lion, L. W. 1998 Turbulent coagulation of colloidal particles. J. Fluid Mech. 364, 81–113.CrossRefGoogle Scholar
Buesser, B. & Pratsinis, S. E. 2012 Design of nanomaterial synthesis by aerosol processes. Annu. Rev. Chem. Biomol. Engng 3, 103–127.CrossRefGoogle ScholarPubMed
Chun, J. & Koch, D. L. 2005 Coagulation of monodisperse aerosol particles by isotropic turbulence. Phys. Fluids 17 (2), 027102.CrossRefGoogle Scholar
Curtis, A. S. G. & Hocking, L. M. 1970 Collision efficiency of equal spherical particles in a shear flow. The influence of London-van der Waals forces. Trans. Faraday Soc. 66, 1381–1390.CrossRefGoogle Scholar
Davis, R. H. 1984 The rate of coagulation of a dilute polydisperse system of sedimenting spheres. J. Fluid Mech. 145, 179–199.CrossRefGoogle Scholar
Davis, R. H., Schonberg, J. A. & Rallison, J. M. 1989 The lubrication force between two viscous drops. Phys. Fluids A 1 (1), 77–81.CrossRefGoogle Scholar
Dhariwal, R. & Bragg, A. D. 2018 Small-scale dynamics of settling, bidisperse particles in turbulence. J. Fluid Mech. 839, 594–620.CrossRefGoogle Scholar
Gopinath, A., Chen, S. B. & Koch, D. L. 1997 Lubrication flows between spherical particles colliding in a compressible non-continuum gas. J. Fluid Mech. 344, 245–269.CrossRefGoogle Scholar
Gopinath, A. & Koch, D. L. 2002 Collision and rebound of small droplets in an incompressible continuum gas. J. Fluid Mech. 454, 145–201.CrossRefGoogle Scholar
Grabowski, W. W. & Wang, L.-P. 2013 Growth of cloud droplets in a turbulent environment. Annu. Rev. Fluid Mech. 45, 293–324.CrossRefGoogle Scholar
Hocking, L. M. & Jonas, P. R. 1970 The collision efficiency of small drops. Q. J. R. Meteorol. Soc. 96 (410), 722–729.CrossRefGoogle Scholar
Ireland, P. J., Bragg, A. D. & Collins, L. R. 2016 a The effect of Reynolds number on inertial particle dynamics in isotropic turbulence. Part 1. Simulations without gravitational effects. J. Fluid Mech. 796, 617–658.CrossRefGoogle Scholar
Ireland, P. J., Bragg, A. D. & Collins, L. R. 2016 b The effect of Reynolds number on inertial particle dynamics in isotropic turbulence. Part 2. Simulations with gravitational effects. J. Fluid Mech. 796, 659–711.CrossRefGoogle Scholar
Jaworek, A., Sobczyk, A. T., Krupa, A., Marchewicz, A., Czech, T. & Śliwiński, L. 2018 Hybrid electrostatic filtration systems for fly ash particles emission control. A review. Sep. Purif. Technol. 213, 283–302.CrossRefGoogle Scholar
Jeffrey, D. J. 1992 The calculation of the low reynolds number resistance functions for two unequal spheres. Phys. Fluids A 4 (1), 16–29.CrossRefGoogle Scholar
Jeffrey, D. J. & Onishi, Y. 1984 Calculation of the resistance and mobility functions for two unequal rigid spheres in low-Reynolds-number flow. J. Fluid Mech. 139, 261–290.CrossRefGoogle Scholar
Kim, S. & Karrila, S. J. 2013 Microhydrodynamics: Principles and Selected Applications. Courier Corporation.Google Scholar
Laurent, F. & Massot, M. 2001 Multi-fluid modelling of laminar polydisperse spray flames: origin, assumptions and comparison of sectional and sampling methods. Combust. Theor. Model. 5 (4), 537–572.CrossRefGoogle Scholar
Malá, H., Rulík, P., Bečková, V., Mihalík, J. & Slezáková, M. 2013 Particle size distribution of radioactive aerosols after the Fukushima and the Chernobyl accidents. J. Environ. Radioactiv. 126, 92–98.CrossRefGoogle Scholar
Qian, J. & Law, C. K. 1997 Regimes of coalescence and separation in droplet collision. J. Fluid Mech. 331, 59–80.CrossRefGoogle Scholar
Rosa, B., Wang, L.-P., Maxey, M. R. & Grabowski, W. W. 2011 An accurate and efficient method for treating aerodynamic interactions of cloud droplets. J. Comput. Phys. 230 (22), 8109–8133.CrossRefGoogle Scholar
Russel, W. B., Russel, W. B., Saville, D. A. & Schowalter, W. R. 1991 Colloidal Dispersions. Cambridge University Press.Google Scholar
Saffman, P. G. F. & Turner, J. S. 1956 On the collision of drops in turbulent clouds. J. Fluid Mech. 1 (1), 16–30.CrossRefGoogle Scholar
Smoluchowski, M. V. 1918 Versuch einer mathematischen theorie der koagulationskinetik kolloider lösungen. Z. Phys. Chem. 92 (1), 129–168.Google Scholar
Sundaram, S. & Collins, L. R. 1997 Collision statistics in an isotropic particle-laden turbulent suspension. Part 1. Direct numerical simulations. J. Fluid Mech. 335, 75–109.CrossRefGoogle Scholar
Sundararajakumar, R. R. & Koch, D. L. 1996 Non-continuum lubrication flows between particles colliding in a gas. J. Fluid Mech. 313, 283–308.CrossRefGoogle Scholar
Wang, H., Zinchenko, A. Z. & Davis, R. H. 1994 The collision rate of small drops in linear flow fields. J. Fluid Mech. 265, 161–188.CrossRefGoogle Scholar
Yeung, P. K. & Pope, S. B. 1989 Lagrangian statistics from direct numerical simulations of isotropic turbulence. J. Fluid Mech. 207, 531–586.CrossRefGoogle Scholar
Zeichner, G. R. & Schowalter, W. R. 1977 Use of trajectory analysis to study stability of colloidal dispersions in flow fields. AIChE J. 23 (3), 243–254.CrossRefGoogle Scholar
Zinchenko, A. Z. & Davis, R. H. 1994 Gravity-induced coalescence of drops at arbitrary Péclet numbers. J. Fluid Mech. 280, 119–148.CrossRefGoogle Scholar