Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-17T07:24:48.389Z Has data issue: false hasContentIssue false
  • English
  • Français

Coalescence dynamics of a compound drop on a deep liquid pool

Published online by Cambridge University Press:  05 March 2019

Hiranya Deka Open the ORCID record for Hiranya Deka [Opens in a new window] ,
Gautam Biswas Open the ORCID record for Gautam Biswas [Opens in a new window] ,
Kirti Chandra Sahu Open the ORCID record for Kirti Chandra Sahu [Opens in a new window] ,
Yash Kulkarni  and
Amaresh Dalal Open the ORCID record for Amaresh Dalal [Opens in a new window]
Hiranya Deka
Affiliation:
Department of Mechanical Engineering, Indian Institute of Technology Guwahati, Guwahati 781 039, Assam, India
Gautam Biswas*
Affiliation:
Department of Mechanical Engineering, Indian Institute of Technology Guwahati, Guwahati 781 039, Assam, India
Kirti Chandra Sahu
Affiliation:
Department of Chemical Engineering, Indian Institute of Technology Hyderabad, Sangareddy 502 285, Telangana, India
Yash Kulkarni
Affiliation:
Department of Mechanical Engineering, Indian Institute of Technology Guwahati, Guwahati 781 039, Assam, India
Amaresh Dalal
Affiliation:
Department of Mechanical Engineering, Indian Institute of Technology Guwahati, Guwahati 781 039, Assam, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

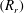The partial coalescence dynamics of a compound drop in a liquid pool is numerically investigated. We study the effect of the ratio of the inner to outer radii $(R_{r})$ of the compound drop while maintaining a constant liquid volume in the outer shell of the compound droplet. It is observed that for small values of the radius ratio, the coalescence dynamics is similar to that of a ‘simple’ drop, but the partial coalescence is suppressed for large values of 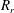$R_{r}$. Increasing the value of 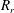$R_{r}$ decreases the distance migrated by the inner bubble in the downward direction inside the pool. The location of the bubble after coalescence is found to play an important role in the pinch-off process of the satellite drop. The influence of the governing dimensionless parameters on the coalescence dynamics has also been investigated.

JFM classification

Drops and Bubbles: Breakup/coalescence
Type
JFM Rapids
Information
Journal of Fluid Mechanics , Volume 866 , 10 May 2019 , R2
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aston, J. G. 1972 Gas-filled hollow drops in aerosols. Colloid Interface Sci. 38, 547–553.Google Scholar
Blanchette, F. & Bigioni, T. P. 2006 Partial coalescence of drops at liquid interfaces. Nat. Phys. 2 (5), 254–257.Google Scholar
Charles, G. E. & Mason, S. G. 1960 The mechanism of partial coalescence of liquid drops at liquid/liquid interfaces. J. Colloid Sci. 15 (2), 105–122.Google Scholar
Chen, X., Mandre, S. & Feng, J. J. 2006a An experimental study of the coalescence between a drop and an interface in Newtonian and polymeric liquids. Phys. Fluids 18, 092103.Google Scholar
Chen, X., Mandre, S. & Feng, J. J. 2006b Partial coalescence between a drop and a liquid–liquid interface. Phys. Fluids 18 (5), 051705.Google Scholar
Deka, H., Biswas, G., Chakraborty, S. & Dalal, A. 2019 Coalescence dynamics of unequal sized drops. Phys. Fluids 31 (1), 012105.Google Scholar
Deka, H., Ray, B., Biswas, G. & Dalal, A. 2018 Dynamics of tongue shaped cavity generated during the impact of high-speed microdrops. Phys. Fluids 30 (4), 042103.Google Scholar
Ding, H., Li, E. Q., Zhang, F. H., Sui, Y., Spelt, P. D. M. & Thoroddsen, S. T. 2012 Propagation of capillary waves and ejection of small droplets in rapid droplet spreading. J. Fluid Mech. 697, 92–114.Google Scholar
Gao, P. & Feng, J. J. 2011 Spreading and breakup of a compound drop on a partially wetting substrate. J. Fluid Mech. 682, 415–433.Google Scholar
Gilet, T., Mulleners, K., Lecomte, J. P., Vandewalle, N. & Dorbolo, S. 2007 Critical parameters for the partial coalescence of a droplet. Phy. Rev. E 75, 036303.Google Scholar
Gulyaev, I. P., Solonenko, O. P., Gulyaev, P. Y. & Smirnov, A. V. 2009 Hydrodynamic features of the impact of a hollow spherical drop on a flat surface. Tech. Phys. Lett. 35 (10), 885–888.Google Scholar
Kavehpour, H. P. 2015 Coalescence of drops. Annu. Rev. Fluid Mech. 47, 245–268.Google Scholar
Kumar, A., Gu, S., Tabbara, H. & Kamnis, S. 2013 Study of impingement of hollow ZrO2 droplets onto a substrate. Surf. Coat. Technol. 220, 164–169.Google Scholar
Li, D., Duan, X., Zheng, Z. & Liu, Y. 2018 Dynamics and heat transfer of a hollow droplet impact on a wetted solid surface. Intl J. Heat Mass Transfer 122, 1014–1023.Google Scholar
Morton, D., Rudman, M. & Jong-Leng, L. 2000 An investigation of the flow regimes resulting from splashing drops. Phys. Fluids 12, 747–763.Google Scholar
Popinet, S. 2009 An accurate adaptive solver for surface-tension-driven interfacial flows. J. Comput. Phys. 228 (16), 5838–5866.Google Scholar
Ray, B., Biswas, G. & Sharma, A. 2010 Generation of secondary droplets in coalescence of a drop at a liquid–liquid interface. J. Fluid Mech. 655, 72–104.Google Scholar
Rayleigh, L. 1878 On the instability of jets. Proc. Lond. Math. Soc. 1 (1), 4–13.Google Scholar
Stone, H. A., Stroock, A. D. & Ajdari, A. 2004 Engineering flows in small devices: microfluidics toward a lab-on-a-chip. Annu. Rev. Fluid Mech. 36, 381–411.Google Scholar
Terwagne, D., Gilet, T., Vandewalle, N. & Dorbolo, S. 2010 From a bouncing compound drop to a double emulsion. Langmuir 26 (14), 11680–11685.Google Scholar
Thomson, J. J. & Newall, H. F. 1886 V. On the formation of vortex rings by drops falling into liquids, and some allied phenomena. Proc. R. Soc. Lond. 39 (239–241), 417–436.Google Scholar
Thoroddsen, S. T., Qian, B., Etoh, T. G. & Takehara, K. 2007 The initial coalescence of miscible drops. Phys. Fluids 19 (7), 072110.Google Scholar
Thoroddsen, S. T. & Takehara, K. 2000 The coalescence cascade of a drop. Phys. Fluids 12, 1265–1267.Google Scholar
Tripathi, M. K., Sahu, K. C. & Govindarajan, R. 2015 Dynamics of an initially spherical bubble rising in quiescent liquid. Nat. Commun. 6, 6268.Google Scholar
Worthington, A. M. 1908 A Study of Splashes. Longmans, Green, and Co.Google Scholar
Yue, P., Zhou, C. & Feng, J. J. 2006 A computational study of the coalescence between a drop and an interface in Newtonian and viscoelastic fluids. Phys. Fluids 18 (10), 102102.Google Scholar
Zhang, F. H., Thoraval, M-J, Thoroddsen, S. T. & Taborek, P. 2015 Partial coalescence from bubbles to drops. J. Fluid Mech. 782, 209–239.Google Scholar
Zheng, Z.-W., Li, D.-S., Qiu, X.-Q. & Cui, Y.-J. 2017 Numerical analysis of hollow droplet impact on a flat surface. Acta Phys. Sinica 66 (1), 14704.Google Scholar